Scaling CLIP: A Comprehensive Analysis of Data, Architecture, and Training Strategies

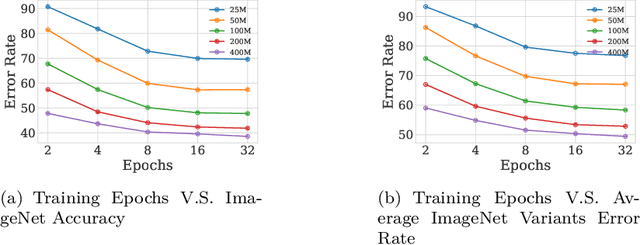
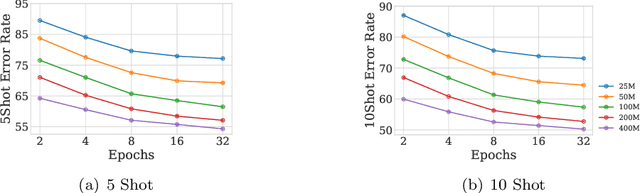
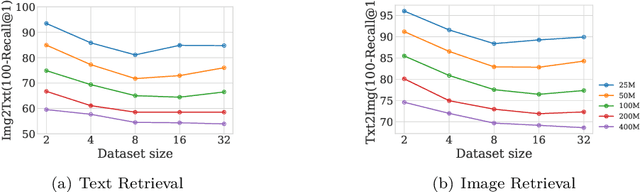
This paper investigates the performance of the Contrastive Language-Image Pre-training (CLIP) when scaled down to limited computation budgets. We explore CLIP along three dimensions: data, architecture, and training strategies. With regards to data, we demonstrate the significance of high-quality training data and show that a smaller dataset of high-quality data can outperform a larger dataset with lower quality. We also examine how model performance varies with different dataset sizes, suggesting that smaller ViT models are better suited for smaller datasets, while larger models perform better on larger datasets with fixed compute. Additionally, we provide guidance on when to choose a CNN-based architecture or a ViT-based architecture for CLIP training. We compare four CLIP training strategies - SLIP, FLIP, CLIP, and CLIP+Data Augmentation - and show that the choice of training strategy depends on the available compute resource. Our analysis reveals that CLIP+Data Augmentation can achieve comparable performance to CLIP using only half of the training data. This work provides practical insights into how to effectively train and deploy CLIP models, making them more accessible and affordable for practical use in various applications.

AutoNumerics-Zero: Automated Discovery of State-of-the-Art Mathematical Functions

Computers calculate transcendental functions by approximating them through the composition of a few limited-precision instructions. For example, an exponential can be calculated with a Taylor series. These approximation methods were developed over the centuries by mathematicians, who emphasized the attainability of arbitrary precision. Computers, however, operate on few limited precision types, such as the popular float32. In this study, we show that when aiming for limited precision, existing approximation methods can be outperformed by programs automatically discovered from scratch by a simple evolutionary algorithm. In particular, over real numbers, our method can approximate the exponential function reaching orders of magnitude more precision for a given number of operations when compared to previous approaches. More practically, over float32 numbers and constrained to less than 1 ULP of error, the same method attains a speedup over baselines by generating code that triggers better XLA/LLVM compilation paths. In other words, in both cases, evolution searched a vast space of possible programs, without knowledge of mathematics, to discover previously unknown optimized approximations to high precision, for the first time. We also give evidence that these results extend beyond the exponential. The ubiquity of transcendental functions suggests that our method has the potential to reduce the cost of scientific computing applications.

Learning and Controlling Silicon Dopant Transitions in Graphene using Scanning Transmission Electron Microscopy

We introduce a machine learning approach to determine the transition dynamics of silicon atoms on a single layer of carbon atoms, when stimulated by the electron beam of a scanning transmission electron microscope (STEM). Our method is data-centric, leveraging data collected on a STEM. The data samples are processed and filtered to produce symbolic representations, which we use to train a neural network to predict transition probabilities. These learned transition dynamics are then leveraged to guide a single silicon atom throughout the lattice to pre-determined target destinations. We present empirical analyses that demonstrate the efficacy and generality of our approach.

Predicting emergence of crystals from amorphous matter with deep learning

Crystallization of the amorphous phases into metastable crystals plays a fundamental role in the formation of new matter, from geological to biological processes in nature to synthesis and development of new materials in the laboratory. Predicting the outcome of such phase transitions reliably would enable new research directions in these areas, but has remained beyond reach with molecular modeling or ab-initio methods. Here, we show that crystallization products of amorphous phases can be predicted in any inorganic chemistry by sampling the crystallization pathways of their local structural motifs at the atomistic level using universal deep learning potentials. We show that this approach identifies the crystal structures of polymorphs that initially nucleate from amorphous precursors with high accuracy across a diverse set of material systems, including polymorphic oxides, nitrides, carbides, fluorides, chlorides, chalcogenides, and metal alloys. Our results demonstrate that Ostwald's rule of stages can be exploited mechanistically at the molecular level to predictably access new metastable crystals from the amorphous phase in material synthesis.

Tied-Augment: Controlling Representation Similarity Improves Data Augmentation

Data augmentation methods have played an important role in the recent advance of deep learning models, and have become an indispensable component of state-of-the-art models in semi-supervised, self-supervised, and supervised training for vision. Despite incurring no additional latency at test time, data augmentation often requires more epochs of training to be effective. For example, even the simple flips-and-crops augmentation requires training for more than 5 epochs to improve performance, whereas RandAugment requires more than 90 epochs. We propose a general framework called Tied-Augment, which improves the efficacy of data augmentation in a wide range of applications by adding a simple term to the loss that can control the similarity of representations under distortions. Tied-Augment can improve state-of-the-art methods from data augmentation (e.g. RandAugment, mixup), optimization (e.g. SAM), and semi-supervised learning (e.g. FixMatch). For example, Tied-RandAugment can outperform RandAugment by 2.0% on ImageNet. Notably, using Tied-Augment, data augmentation can be made to improve generalization even when training for a few epochs and when fine-tuning. We open source our code at https://github.com/ekurtulus/tied-augment/tree/main.

LidarAugment: Searching for Scalable 3D LiDAR Data Augmentations

Data augmentations are important in training high-performance 3D object detectors for point clouds. Despite recent efforts on designing new data augmentations, perhaps surprisingly, most state-of-the-art 3D detectors only use a few simple data augmentations. In particular, different from 2D image data augmentations, 3D data augmentations need to account for different representations of input data and require being customized for different models, which introduces significant overhead. In this paper, we resort to a search-based approach, and propose LidarAugment, a practical and effective data augmentation strategy for 3D object detection. Unlike previous approaches where all augmentation policies are tuned in an exponentially large search space, we propose to factorize and align the search space of each data augmentation, which cuts down the 20+ hyperparameters to 2, and significantly reduces the search complexity. We show LidarAugment can be customized for different model architectures with different input representations by a simple 2D grid search, and consistently improve both convolution-based UPillars/StarNet/RSN and transformer-based SWFormer. Furthermore, LidarAugment mitigates overfitting and allows us to scale up 3D detectors to much larger capacity. In particular, by combining with latest 3D detectors, our LidarAugment achieves a new state-of-the-art 74.8 mAPH L2 on Waymo Open Dataset.

What does a deep neural network confidently perceive? The effective dimension of high certainty class manifolds and their low confidence boundaries

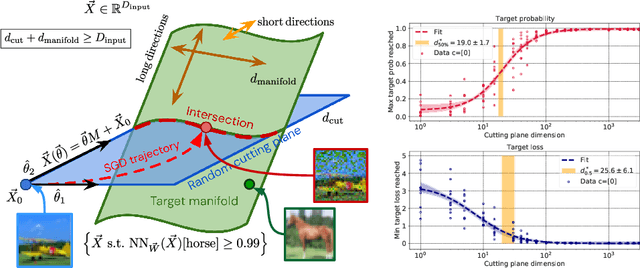
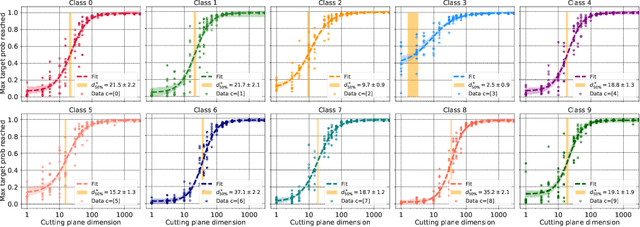
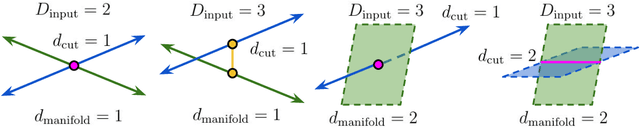
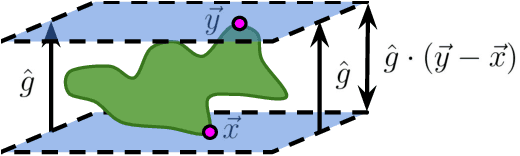
Deep neural network classifiers partition input space into high confidence regions for each class. The geometry of these class manifolds (CMs) is widely studied and intimately related to model performance; for example, the margin depends on CM boundaries. We exploit the notions of Gaussian width and Gordon's escape theorem to tractably estimate the effective dimension of CMs and their boundaries through tomographic intersections with random affine subspaces of varying dimension. We show several connections between the dimension of CMs, generalization, and robustness. In particular we investigate how CM dimension depends on 1) the dataset, 2) architecture (including ResNet, WideResNet \& Vision Transformer), 3) initialization, 4) stage of training, 5) class, 6) network width, 7) ensemble size, 8) label randomization, 9) training set size, and 10) robustness to data corruption. Together a picture emerges that higher performing and more robust models have higher dimensional CMs. Moreover, we offer a new perspective on ensembling via intersections of CMs. Our code is at https://github.com/stanislavfort/slice-dice-optimize/

Beyond the Imitation Game: Quantifying and extrapolating the capabilities of language models

Language models demonstrate both quantitative improvement and new qualitative capabilities with increasing scale. Despite their potentially transformative impact, these new capabilities are as yet poorly characterized. In order to inform future research, prepare for disruptive new model capabilities, and ameliorate socially harmful effects, it is vital that we understand the present and near-future capabilities and limitations of language models. To address this challenge, we introduce the Beyond the Imitation Game benchmark (BIG-bench). BIG-bench currently consists of 204 tasks, contributed by 442 authors across 132 institutions. Task topics are diverse, drawing problems from linguistics, childhood development, math, common-sense reasoning, biology, physics, social bias, software development, and beyond. BIG-bench focuses on tasks that are believed to be beyond the capabilities of current language models. We evaluate the behavior of OpenAI's GPT models, Google-internal dense transformer architectures, and Switch-style sparse transformers on BIG-bench, across model sizes spanning millions to hundreds of billions of parameters. In addition, a team of human expert raters performed all tasks in order to provide a strong baseline. Findings include: model performance and calibration both improve with scale, but are poor in absolute terms (and when compared with rater performance); performance is remarkably similar across model classes, though with benefits from sparsity; tasks that improve gradually and predictably commonly involve a large knowledge or memorization component, whereas tasks that exhibit "breakthrough" behavior at a critical scale often involve multiple steps or components, or brittle metrics; social bias typically increases with scale in settings with ambiguous context, but this can be improved with prompting.

PolyLoss: A Polynomial Expansion Perspective of Classification Loss Functions

Cross-entropy loss and focal loss are the most common choices when training deep neural networks for classification problems. Generally speaking, however, a good loss function can take on much more flexible forms, and should be tailored for different tasks and datasets. Motivated by how functions can be approximated via Taylor expansion, we propose a simple framework, named PolyLoss, to view and design loss functions as a linear combination of polynomial functions. Our PolyLoss allows the importance of different polynomial bases to be easily adjusted depending on the targeting tasks and datasets, while naturally subsuming the aforementioned cross-entropy loss and focal loss as special cases. Extensive experimental results show that the optimal choice within the PolyLoss is indeed dependent on the task and dataset. Simply by introducing one extra hyperparameter and adding one line of code, our Poly-1 formulation outperforms the cross-entropy loss and focal loss on 2D image classification, instance segmentation, object detection, and 3D object detection tasks, sometimes by a large margin.

Learn2Hop: Learned Optimization on Rough Landscapes

Optimization of non-convex loss surfaces containing many local minima remains a critical problem in a variety of domains, including operations research, informatics, and material design. Yet, current techniques either require extremely high iteration counts or a large number of random restarts for good performance. In this work, we propose adapting recent developments in meta-learning to these many-minima problems by learning the optimization algorithm for various loss landscapes. We focus on problems from atomic structural optimization--finding low energy configurations of many-atom systems--including widely studied models such as bimetallic clusters and disordered silicon. We find that our optimizer learns a 'hopping' behavior which enables efficient exploration and improves the rate of low energy minima discovery. Finally, our learned optimizers show promising generalization with efficiency gains on never before seen tasks (e.g. new elements or compositions). Code will be made available shortly.