Ordered Topological Deep Learning: a Network Modeling Case Study

Computer networks are the foundation of modern digital infrastructure, facilitating global communication and data exchange. As demand for reliable high-bandwidth connectivity grows, advanced network modeling techniques become increasingly essential to optimize performance and predict network behavior. Traditional modeling methods, such as packet-level simulators and queueing theory, have notable limitations --either being computationally expensive or relying on restrictive assumptions that reduce accuracy. In this context, the deep learning-based RouteNet family of models has recently redefined network modeling by showing an unprecedented cost-performance trade-off. In this work, we revisit RouteNet's sophisticated design and uncover its hidden connection to Topological Deep Learning (TDL), an emerging field that models higher-order interactions beyond standard graph-based methods. We demonstrate that, although originally formulated as a heterogeneous Graph Neural Network, RouteNet serves as the first instantiation of a new form of TDL. More specifically, this paper presents OrdGCCN, a novel TDL framework that introduces the notion of ordered neighbors in arbitrary discrete topological spaces, and shows that RouteNet's architecture can be naturally described as an ordered topological neural network. To the best of our knowledge, this marks the first successful real-world application of state-of-the-art TDL principles --which we confirm through extensive testbed experiments--, laying the foundation for the next generation of ordered TDL-driven applications.

From superposition to sparse codes: interpretable representations in neural networks

Understanding how information is represented in neural networks is a fundamental challenge in both neuroscience and artificial intelligence. Despite their nonlinear architectures, recent evidence suggests that neural networks encode features in superposition, meaning that input concepts are linearly overlaid within the network's representations. We present a perspective that explains this phenomenon and provides a foundation for extracting interpretable representations from neural activations. Our theoretical framework consists of three steps: (1) Identifiability theory shows that neural networks trained for classification recover latent features up to a linear transformation. (2) Sparse coding methods can extract disentangled features from these representations by leveraging principles from compressed sensing. (3) Quantitative interpretability metrics provide a means to assess the success of these methods, ensuring that extracted features align with human-interpretable concepts. By bridging insights from theoretical neuroscience, representation learning, and interpretability research, we propose an emerging perspective on understanding neural representations in both artificial and biological systems. Our arguments have implications for neural coding theories, AI transparency, and the broader goal of making deep learning models more interpretable.

When Machine Learning Gets Personal: Understanding Fairness of Personalized Models

Personalization in machine learning involves tailoring models to individual users by incorporating personal attributes such as demographic or medical data. While personalization can improve prediction accuracy, it may also amplify biases and reduce explainability. This work introduces a unified framework to evaluate the impact of personalization on both prediction accuracy and explanation quality across classification and regression tasks. We derive novel upper bounds for the number of personal attributes that can be used to reliably validate benefits of personalization. Our analysis uncovers key trade-offs. We show that regression models can potentially utilize more personal attributes than classification models. We also demonstrate that improvements in prediction accuracy due to personalization do not necessarily translate to enhanced explainability -- underpinning the importance to evaluate both metrics when personalizing machine learning models in critical settings such as healthcare. Validated with a real-world dataset, this framework offers practical guidance for balancing accuracy, fairness, and interpretability in personalized models.

TopoTune : A Framework for Generalized Combinatorial Complex Neural Networks

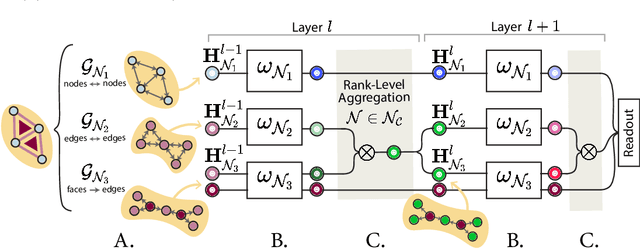
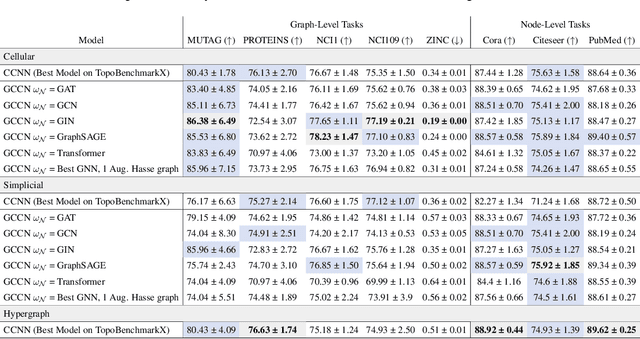
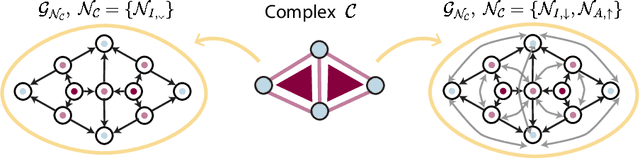
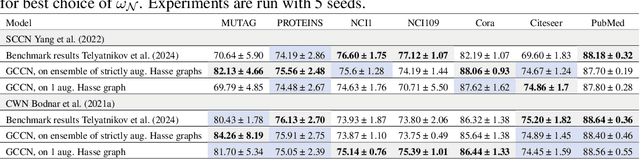
Graph Neural Networks (GNNs) excel in learning from relational datasets, processing node and edge features in a way that preserves the symmetries of the graph domain. However, many complex systems--such as biological or social networks--involve multiway complex interactions that are more naturally represented by higher-order topological spaces. The emerging field of Topological Deep Learning (TDL) aims to accommodate and leverage these higher-order structures. Combinatorial Complex Neural Networks (CCNNs), fairly general TDL models, have been shown to be more expressive and better performing than GNNs. However, differently from the graph deep learning ecosystem, TDL lacks a principled and standardized framework for easily defining new architectures, restricting its accessibility and applicability. To address this issue, we introduce Generalized CCNNs (GCCNs), a novel simple yet powerful family of TDL models that can be used to systematically transform any (graph) neural network into its TDL counterpart. We prove that GCCNs generalize and subsume CCNNs, while extensive experiments on a diverse class of GCCNs show that these architectures consistently match or outperform CCNNs, often with less model complexity. In an effort to accelerate and democratize TDL, we introduce TopoTune, a lightweight software that allows practitioners to define, build, and train GCCNs with unprecedented flexibility and ease.

ICML Topological Deep Learning Challenge 2024: Beyond the Graph Domain

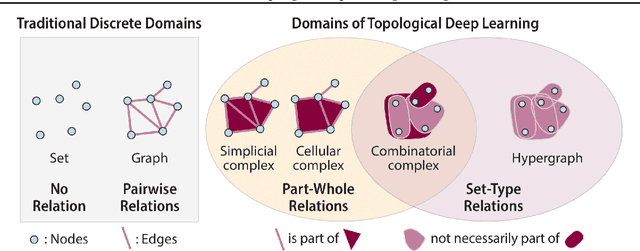
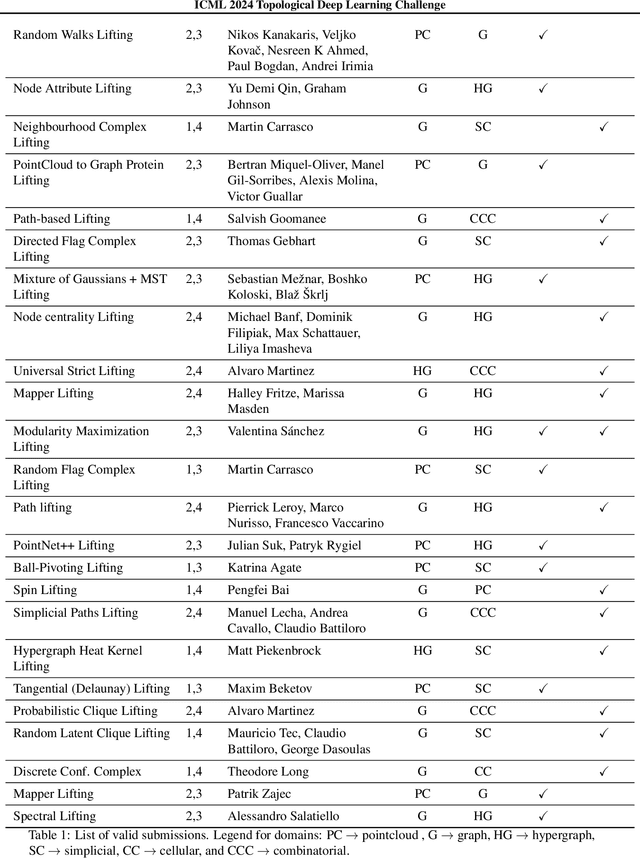
This paper describes the 2nd edition of the ICML Topological Deep Learning Challenge that was hosted within the ICML 2024 ELLIS Workshop on Geometry-grounded Representation Learning and Generative Modeling (GRaM). The challenge focused on the problem of representing data in different discrete topological domains in order to bridge the gap between Topological Deep Learning (TDL) and other types of structured datasets (e.g. point clouds, graphs). Specifically, participants were asked to design and implement topological liftings, i.e. mappings between different data structures and topological domains --like hypergraphs, or simplicial/cell/combinatorial complexes. The challenge received 52 submissions satisfying all the requirements. This paper introduces the main scope of the challenge, and summarizes the main results and findings.

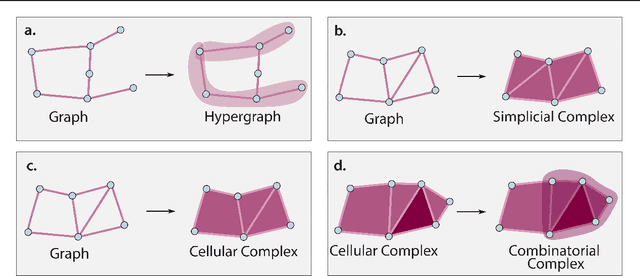
Beyond Euclid: An Illustrated Guide to Modern Machine Learning with Geometric, Topological, and Algebraic Structures

The enduring legacy of Euclidean geometry underpins classical machine learning, which, for decades, has been primarily developed for data lying in Euclidean space. Yet, modern machine learning increasingly encounters richly structured data that is inherently nonEuclidean. This data can exhibit intricate geometric, topological and algebraic structure: from the geometry of the curvature of space-time, to topologically complex interactions between neurons in the brain, to the algebraic transformations describing symmetries of physical systems. Extracting knowledge from such non-Euclidean data necessitates a broader mathematical perspective. Echoing the 19th-century revolutions that gave rise to non-Euclidean geometry, an emerging line of research is redefining modern machine learning with non-Euclidean structures. Its goal: generalizing classical methods to unconventional data types with geometry, topology, and algebra. In this review, we provide an accessible gateway to this fast-growing field and propose a graphical taxonomy that integrates recent advances into an intuitive unified framework. We subsequently extract insights into current challenges and highlight exciting opportunities for future development in this field.

The Selective G-Bispectrum and its Inversion: Applications to G-Invariant Networks

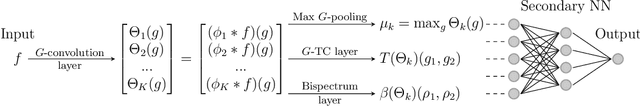
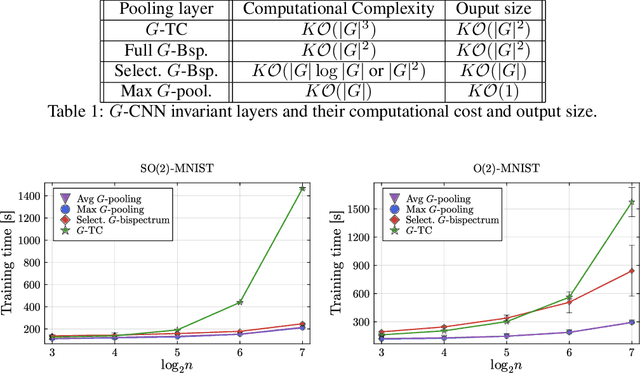
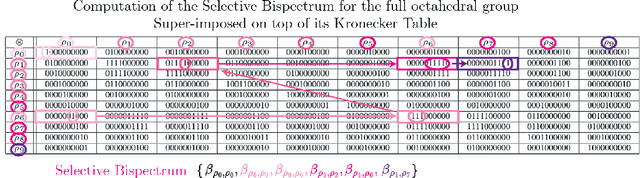
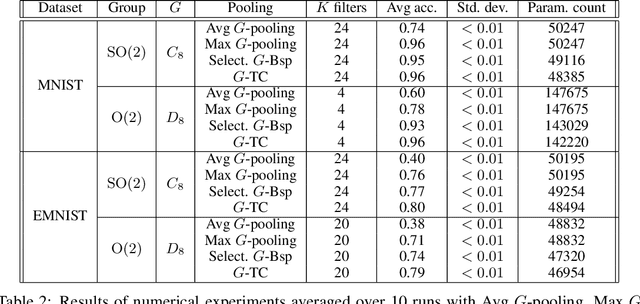
An important problem in signal processing and deep learning is to achieve \textit{invariance} to nuisance factors not relevant for the task. Since many of these factors are describable as the action of a group $G$ (e.g. rotations, translations, scalings), we want methods to be $G$-invariant. The $G$-Bispectrum extracts every characteristic of a given signal up to group action: for example, the shape of an object in an image, but not its orientation. Consequently, the $G$-Bispectrum has been incorporated into deep neural network architectures as a computational primitive for $G$-invariance\textemdash akin to a pooling mechanism, but with greater selectivity and robustness. However, the computational cost of the $G$-Bispectrum ($\mathcal{O}(|G|^2)$, with $|G|$ the size of the group) has limited its widespread adoption. Here, we show that the $G$-Bispectrum computation contains redundancies that can be reduced into a \textit{selective $G$-Bispectrum} with $\mathcal{O}(|G|)$ complexity. We prove desirable mathematical properties of the selective $G$-Bispectrum and demonstrate how its integration in neural networks enhances accuracy and robustness compared to traditional approaches, while enjoying considerable speeds-up compared to the full $G$-Bispectrum.

TopoBenchmarkX: A Framework for Benchmarking Topological Deep Learning

This work introduces TopoBenchmarkX, a modular open-source library designed to standardize benchmarking and accelerate research in Topological Deep Learning (TDL). TopoBenchmarkX maps the TDL pipeline into a sequence of independent and modular components for data loading and processing, as well as model training, optimization, and evaluation. This modular organization provides flexibility for modifications and facilitates the adaptation and optimization of various TDL pipelines. A key feature of TopoBenchmarkX is that it allows for the transformation and lifting between topological domains. This enables, for example, to obtain richer data representations and more fine-grained analyses by mapping the topology and features of a graph to higher-order topological domains such as simplicial and cell complexes. The range of applicability of TopoBenchmarkX is demonstrated by benchmarking several TDL architectures for various tasks and datasets.

Attending to Topological Spaces: The Cellular Transformer

Topological Deep Learning seeks to enhance the predictive performance of neural network models by harnessing topological structures in input data. Topological neural networks operate on spaces such as cell complexes and hypergraphs, that can be seen as generalizations of graphs. In this work, we introduce the Cellular Transformer (CT), a novel architecture that generalizes graph-based transformers to cell complexes. First, we propose a new formulation of the usual self- and cross-attention mechanisms, tailored to leverage incidence relations in cell complexes, e.g., edge-face and node-edge relations. Additionally, we propose a set of topological positional encodings specifically designed for cell complexes. By transforming three graph datasets into cell complex datasets, our experiments reveal that CT not only achieves state-of-the-art performance, but it does so without the need for more complex enhancements such as virtual nodes, in-domain structural encodings, or graph rewiring.

Position Paper: Challenges and Opportunities in Topological Deep Learning

Topological deep learning (TDL) is a rapidly evolving field that uses topological features to understand and design deep learning models. This paper posits that TDL may complement graph representation learning and geometric deep learning by incorporating topological concepts, and can thus provide a natural choice for various machine learning settings. To this end, this paper discusses open problems in TDL, ranging from practical benefits to theoretical foundations. For each problem, it outlines potential solutions and future research opportunities. At the same time, this paper serves as an invitation to the scientific community to actively participate in TDL research to unlock the potential of this emerging field.