Beyond Euclid: An Illustrated Guide to Modern Machine Learning with Geometric, Topological, and Algebraic Structures

The enduring legacy of Euclidean geometry underpins classical machine learning, which, for decades, has been primarily developed for data lying in Euclidean space. Yet, modern machine learning increasingly encounters richly structured data that is inherently nonEuclidean. This data can exhibit intricate geometric, topological and algebraic structure: from the geometry of the curvature of space-time, to topologically complex interactions between neurons in the brain, to the algebraic transformations describing symmetries of physical systems. Extracting knowledge from such non-Euclidean data necessitates a broader mathematical perspective. Echoing the 19th-century revolutions that gave rise to non-Euclidean geometry, an emerging line of research is redefining modern machine learning with non-Euclidean structures. Its goal: generalizing classical methods to unconventional data types with geometry, topology, and algebra. In this review, we provide an accessible gateway to this fast-growing field and propose a graphical taxonomy that integrates recent advances into an intuitive unified framework. We subsequently extract insights into current challenges and highlight exciting opportunities for future development in this field.

An Embedding-Dynamic Approach to Self-supervised Learning

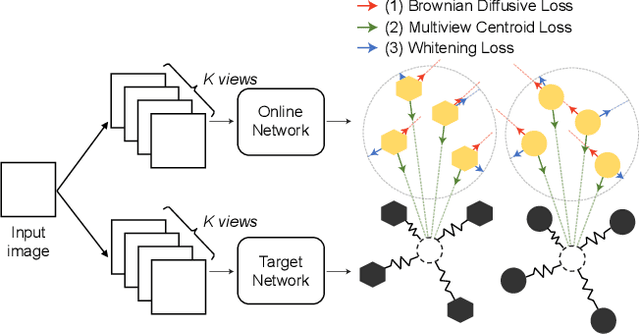
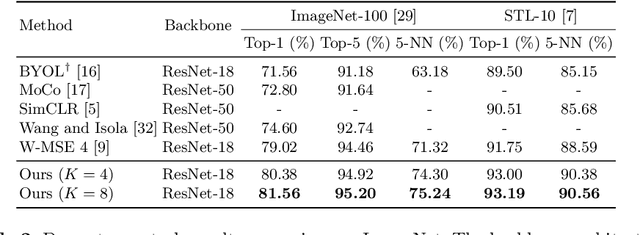
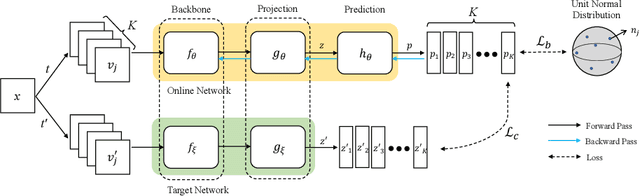
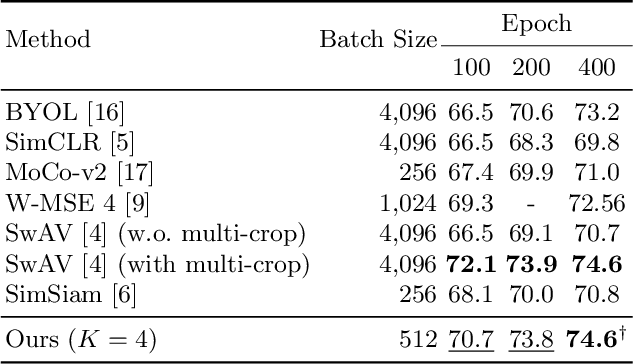
A number of recent self-supervised learning methods have shown impressive performance on image classification and other tasks. A somewhat bewildering variety of techniques have been used, not always with a clear understanding of the reasons for their benefits, especially when used in combination. Here we treat the embeddings of images as point particles and consider model optimization as a dynamic process on this system of particles. Our dynamic model combines an attractive force for similar images, a locally dispersive force to avoid local collapse, and a global dispersive force to achieve a globally-homogeneous distribution of particles. The dynamic perspective highlights the advantage of using a delayed-parameter image embedding (a la BYOL) together with multiple views of the same image. It also uses a purely-dynamic local dispersive force (Brownian motion) that shows improved performance over other methods and does not require knowledge of other particle coordinates. The method is called MSBReg which stands for (i) a Multiview centroid loss, which applies an attractive force to pull different image view embeddings toward their centroid, (ii) a Singular value loss, which pushes the particle system toward spatially homogeneous density, (iii) a Brownian diffusive loss. We evaluate downstream classification performance of MSBReg on ImageNet as well as transfer learning tasks including fine-grained classification, multi-class object classification, object detection, and instance segmentation. In addition, we also show that applying our regularization term to other methods further improves their performance and stabilize the training by preventing a mode collapse.