TopoTune : A Framework for Generalized Combinatorial Complex Neural Networks

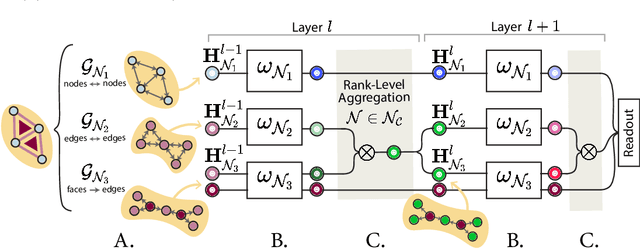
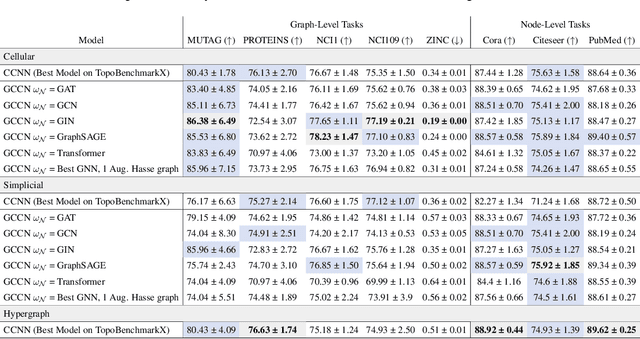
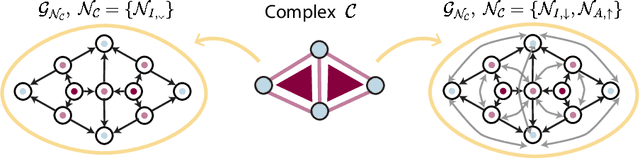
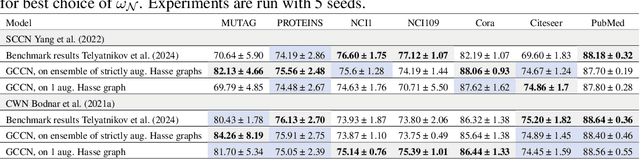
Graph Neural Networks (GNNs) excel in learning from relational datasets, processing node and edge features in a way that preserves the symmetries of the graph domain. However, many complex systems--such as biological or social networks--involve multiway complex interactions that are more naturally represented by higher-order topological spaces. The emerging field of Topological Deep Learning (TDL) aims to accommodate and leverage these higher-order structures. Combinatorial Complex Neural Networks (CCNNs), fairly general TDL models, have been shown to be more expressive and better performing than GNNs. However, differently from the graph deep learning ecosystem, TDL lacks a principled and standardized framework for easily defining new architectures, restricting its accessibility and applicability. To address this issue, we introduce Generalized CCNNs (GCCNs), a novel simple yet powerful family of TDL models that can be used to systematically transform any (graph) neural network into its TDL counterpart. We prove that GCCNs generalize and subsume CCNNs, while extensive experiments on a diverse class of GCCNs show that these architectures consistently match or outperform CCNNs, often with less model complexity. In an effort to accelerate and democratize TDL, we introduce TopoTune, a lightweight software that allows practitioners to define, build, and train GCCNs with unprecedented flexibility and ease.

ICML Topological Deep Learning Challenge 2024: Beyond the Graph Domain

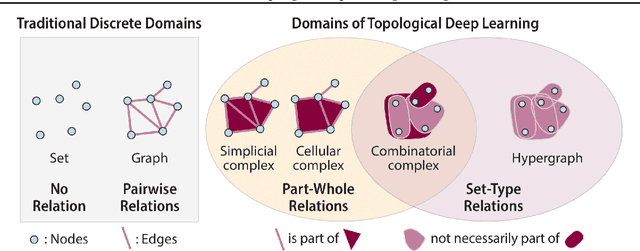
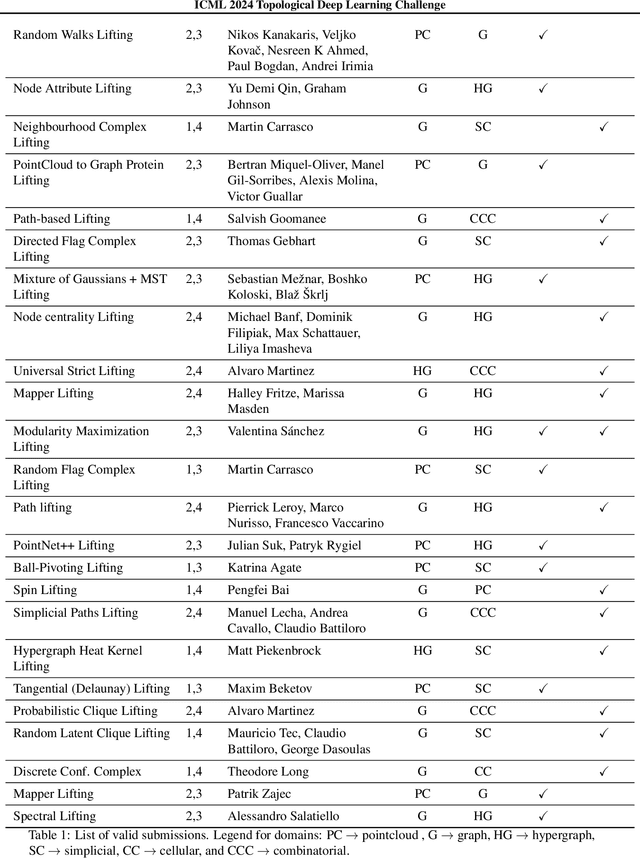
This paper describes the 2nd edition of the ICML Topological Deep Learning Challenge that was hosted within the ICML 2024 ELLIS Workshop on Geometry-grounded Representation Learning and Generative Modeling (GRaM). The challenge focused on the problem of representing data in different discrete topological domains in order to bridge the gap between Topological Deep Learning (TDL) and other types of structured datasets (e.g. point clouds, graphs). Specifically, participants were asked to design and implement topological liftings, i.e. mappings between different data structures and topological domains --like hypergraphs, or simplicial/cell/combinatorial complexes. The challenge received 52 submissions satisfying all the requirements. This paper introduces the main scope of the challenge, and summarizes the main results and findings.

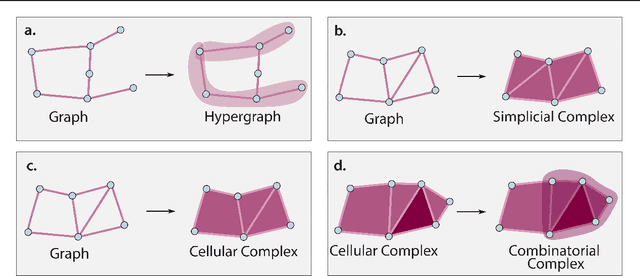
Beyond Euclid: An Illustrated Guide to Modern Machine Learning with Geometric, Topological, and Algebraic Structures

The enduring legacy of Euclidean geometry underpins classical machine learning, which, for decades, has been primarily developed for data lying in Euclidean space. Yet, modern machine learning increasingly encounters richly structured data that is inherently nonEuclidean. This data can exhibit intricate geometric, topological and algebraic structure: from the geometry of the curvature of space-time, to topologically complex interactions between neurons in the brain, to the algebraic transformations describing symmetries of physical systems. Extracting knowledge from such non-Euclidean data necessitates a broader mathematical perspective. Echoing the 19th-century revolutions that gave rise to non-Euclidean geometry, an emerging line of research is redefining modern machine learning with non-Euclidean structures. Its goal: generalizing classical methods to unconventional data types with geometry, topology, and algebra. In this review, we provide an accessible gateway to this fast-growing field and propose a graphical taxonomy that integrates recent advances into an intuitive unified framework. We subsequently extract insights into current challenges and highlight exciting opportunities for future development in this field.

Attending to Topological Spaces: The Cellular Transformer

Topological Deep Learning seeks to enhance the predictive performance of neural network models by harnessing topological structures in input data. Topological neural networks operate on spaces such as cell complexes and hypergraphs, that can be seen as generalizations of graphs. In this work, we introduce the Cellular Transformer (CT), a novel architecture that generalizes graph-based transformers to cell complexes. First, we propose a new formulation of the usual self- and cross-attention mechanisms, tailored to leverage incidence relations in cell complexes, e.g., edge-face and node-edge relations. Additionally, we propose a set of topological positional encodings specifically designed for cell complexes. By transforming three graph datasets into cell complex datasets, our experiments reveal that CT not only achieves state-of-the-art performance, but it does so without the need for more complex enhancements such as virtual nodes, in-domain structural encodings, or graph rewiring.

TopoX: A Suite of Python Packages for Machine Learning on Topological Domains

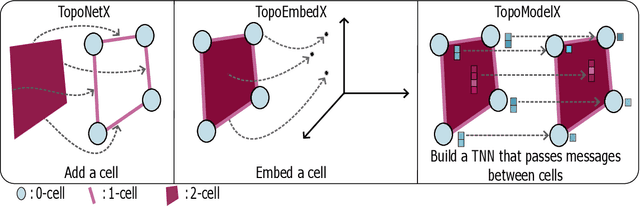
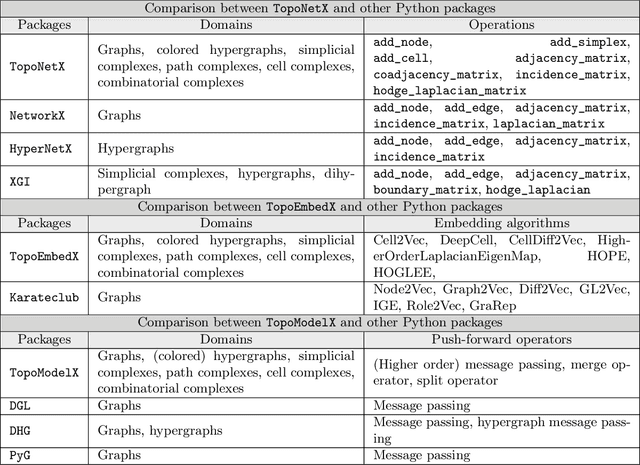
We introduce topox, a Python software suite that provides reliable and user-friendly building blocks for computing and machine learning on topological domains that extend graphs: hypergraphs, simplicial, cellular, path and combinatorial complexes. topox consists of three packages: toponetx facilitates constructing and computing on these domains, including working with nodes, edges and higher-order cells; topoembedx provides methods to embed topological domains into vector spaces, akin to popular graph-based embedding algorithms such as node2vec; topomodelx is built on top of PyTorch and offers a comprehensive toolbox of higher-order message passing functions for neural networks on topological domains. The extensively documented and unit-tested source code of topox is available under MIT license at https://github.com/pyt-team.

ICML 2023 Topological Deep Learning Challenge : Design and Results

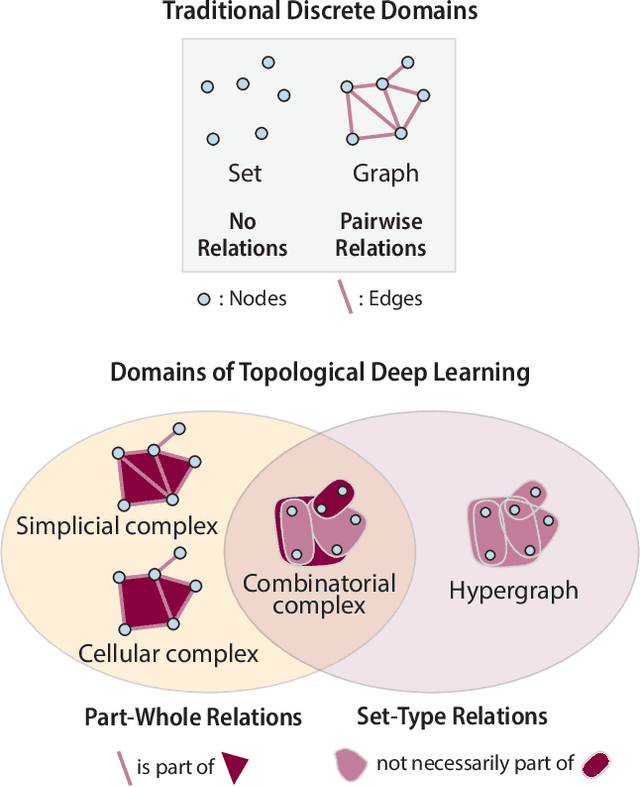
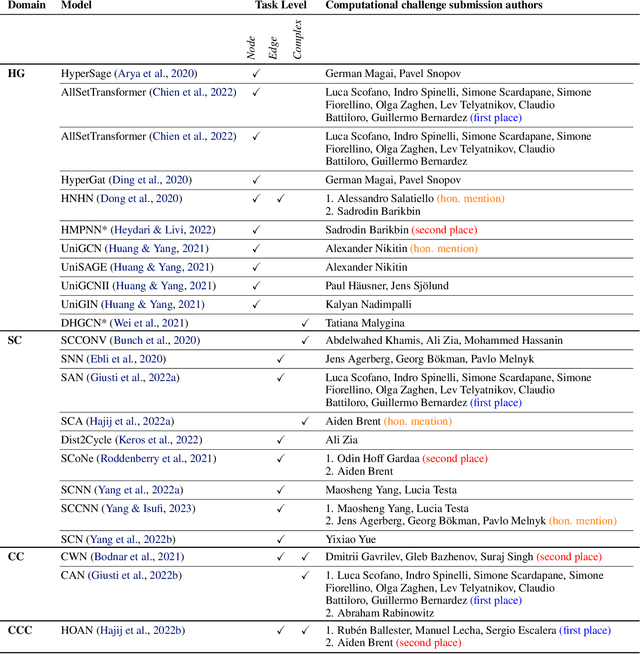
This paper presents the computational challenge on topological deep learning that was hosted within the ICML 2023 Workshop on Topology and Geometry in Machine Learning. The competition asked participants to provide open-source implementations of topological neural networks from the literature by contributing to the python packages TopoNetX (data processing) and TopoModelX (deep learning). The challenge attracted twenty-eight qualifying submissions in its two-month duration. This paper describes the design of the challenge and summarizes its main findings.

Architectures of Topological Deep Learning: A Survey on Topological Neural Networks

The natural world is full of complex systems characterized by intricate relations between their components: from social interactions between individuals in a social network to electrostatic interactions between atoms in a protein. Topological Deep Learning (TDL) provides a comprehensive framework to process and extract knowledge from data associated with these systems, such as predicting the social community to which an individual belongs or predicting whether a protein can be a reasonable target for drug development. TDL has demonstrated theoretical and practical advantages that hold the promise of breaking ground in the applied sciences and beyond. However, the rapid growth of the TDL literature has also led to a lack of unification in notation and language across Topological Neural Network (TNN) architectures. This presents a real obstacle for building upon existing works and for deploying TNNs to new real-world problems. To address this issue, we provide an accessible introduction to TDL, and compare the recently published TNNs using a unified mathematical and graphical notation. Through an intuitive and critical review of the emerging field of TDL, we extract valuable insights into current challenges and exciting opportunities for future development.

Intentional Choreography with Semi-Supervised Recurrent VAEs

We summarize the model and results of PirouNet, a semi-supervised recurrent variational autoencoder. Given a small amount of dance sequences labeled with qualitative choreographic annotations, PirouNet conditionally generates dance sequences in the style of the choreographer.

PirouNet: Creating Intentional Dance with Semi-Supervised Conditional Recurrent Variational Autoencoders

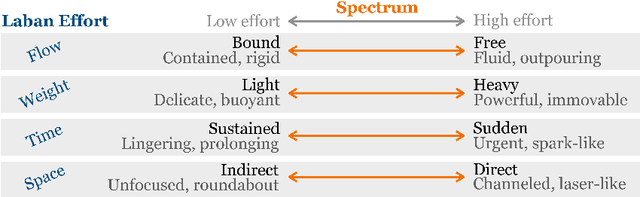
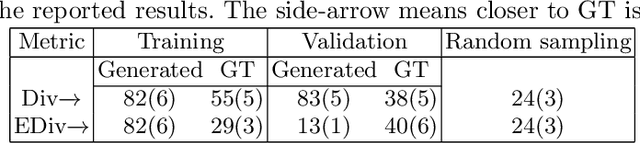
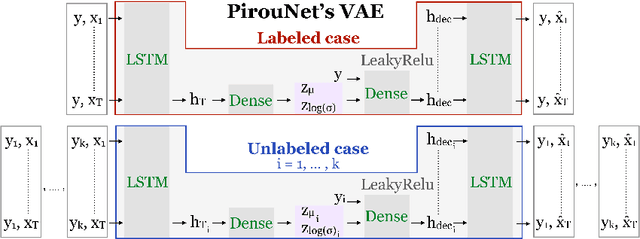
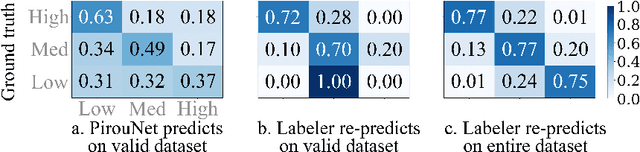
Using Artificial Intelligence (AI) to create dance choreography with intention is still at an early stage. Methods that conditionally generate dance sequences remain limited in their ability to follow choreographer-specific creative intentions, often relying on external prompts or supervised learning. In the same vein, fully annotated dance datasets are rare and labor intensive. To fill this gap and help leverage deep learning as a meaningful tool for choreographers, we propose "PirouNet", a semi-supervised conditional recurrent variational autoencoder together with a dance labeling web application. PirouNet allows dance professionals to annotate data with their own subjective creative labels and subsequently generate new bouts of choreography based on their aesthetic criteria. Thanks to the proposed semi-supervised approach, PirouNet only requires a small portion of the dataset to be labeled, typically on the order of 1%. We demonstrate PirouNet's capabilities as it generates original choreography based on the "Laban Time Effort", an established dance notion describing intention for a movement's time dynamics. We extensively evaluate PirouNet's dance creations through a series of qualitative and quantitative metrics, validating its applicability as a tool for choreographers.