Analytic DAG Constraints for Differentiable DAG Learning

Recovering the underlying Directed Acyclic Graph (DAG) structures from observational data presents a formidable challenge, partly due to the combinatorial nature of the DAG-constrained optimization problem. Recently, researchers have identified gradient vanishing as one of the primary obstacles in differentiable DAG learning and have proposed several DAG constraints to mitigate this issue. By developing the necessary theory to establish a connection between analytic functions and DAG constraints, we demonstrate that analytic functions from the set $\{f(x) = c_0 + \sum_{i=1}^{\infty}c_ix^i | \forall i > 0, c_i > 0; r = \lim_{i\rightarrow \infty}c_{i}/c_{i+1} > 0\}$ can be employed to formulate effective DAG constraints. Furthermore, we establish that this set of functions is closed under several functional operators, including differentiation, summation, and multiplication. Consequently, these operators can be leveraged to create novel DAG constraints based on existing ones. Using these properties, we design a series of DAG constraints and develop an efficient algorithm to evaluate them. Experiments in various settings demonstrate that our DAG constraints outperform previous state-of-the-art comparators. Our implementation is available at https://github.com/zzhang1987/AnalyticDAGLearning.

When Selection Meets Intervention: Additional Complexities in Causal Discovery

We address the common yet often-overlooked selection bias in interventional studies, where subjects are selectively enrolled into experiments. For instance, participants in a drug trial are usually patients of the relevant disease; A/B tests on mobile applications target existing users only, and gene perturbation studies typically focus on specific cell types, such as cancer cells. Ignoring this bias leads to incorrect causal discovery results. Even when recognized, the existing paradigm for interventional causal discovery still fails to address it. This is because subtle differences in when and where interventions happen can lead to significantly different statistical patterns. We capture this dynamic by introducing a graphical model that explicitly accounts for both the observed world (where interventions are applied) and the counterfactual world (where selection occurs while interventions have not been applied). We characterize the Markov property of the model, and propose a provably sound algorithm to identify causal relations as well as selection mechanisms up to the equivalence class, from data with soft interventions and unknown targets. Through synthetic and real-world experiments, we demonstrate that our algorithm effectively identifies true causal relations despite the presence of selection bias.

Permutation-Based Rank Test in the Presence of Discretization and Application in Causal Discovery with Mixed Data

Recent advances have shown that statistical tests for the rank of cross-covariance matrices play an important role in causal discovery. These rank tests include partial correlation tests as special cases and provide further graphical information about latent variables. Existing rank tests typically assume that all the continuous variables can be perfectly measured, and yet, in practice many variables can only be measured after discretization. For example, in psychometric studies, the continuous level of certain personality dimensions of a person can only be measured after being discretized into order-preserving options such as disagree, neutral, and agree. Motivated by this, we propose Mixed data Permutation-based Rank Test (MPRT), which properly controls the statistical errors even when some or all variables are discretized. Theoretically, we establish the exchangeability and estimate the asymptotic null distribution by permutations; as a consequence, MPRT can effectively control the Type I error in the presence of discretization while previous methods cannot. Empirically, our method is validated by extensive experiments on synthetic data and real-world data to demonstrate its effectiveness as well as applicability in causal discovery.

Differentiable Causal Discovery For Latent Hierarchical Causal Models

Discovering causal structures with latent variables from observational data is a fundamental challenge in causal discovery. Existing methods often rely on constraint-based, iterative discrete searches, limiting their scalability to large numbers of variables. Moreover, these methods frequently assume linearity or invertibility, restricting their applicability to real-world scenarios. We present new theoretical results on the identifiability of nonlinear latent hierarchical causal models, relaxing previous assumptions in literature about the deterministic nature of latent variables and exogenous noise. Building on these insights, we develop a novel differentiable causal discovery algorithm that efficiently estimates the structure of such models. To the best of our knowledge, this is the first work to propose a differentiable causal discovery method for nonlinear latent hierarchical models. Our approach outperforms existing methods in both accuracy and scalability. We demonstrate its practical utility by learning interpretable hierarchical latent structures from high-dimensional image data and demonstrate its effectiveness on downstream tasks.

Revisiting Differentiable Structure Learning: Inconsistency of $\ell_1$ Penalty and Beyond

Recent advances in differentiable structure learning have framed the combinatorial problem of learning directed acyclic graphs as a continuous optimization problem. Various aspects, including data standardization, have been studied to identify factors that influence the empirical performance of these methods. In this work, we investigate critical limitations in differentiable structure learning methods, focusing on settings where the true structure can be identified up to Markov equivalence classes, particularly in the linear Gaussian case. While Ng et al. (2024) highlighted potential non-convexity issues in this setting, we demonstrate and explain why the use of $\ell_1$-penalized likelihood in such cases is fundamentally inconsistent, even if the global optimum of the optimization problem can be found. To resolve this limitation, we develop a hybrid differentiable structure learning method based on $\ell_0$-penalized likelihood with hard acyclicity constraint, where the $\ell_0$ penalty can be approximated by different techniques including Gumbel-Softmax. Specifically, we first estimate the underlying moral graph, and use it to restrict the search space of the optimization problem, which helps alleviate the non-convexity issue. Experimental results show that the proposed method enhances empirical performance both before and after data standardization, providing a more reliable path for future advancements in differentiable structure learning, especially for learning Markov equivalence classes.

A Skewness-Based Criterion for Addressing Heteroscedastic Noise in Causal Discovery

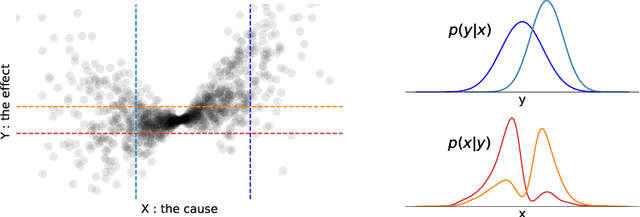
Real-world data often violates the equal-variance assumption (homoscedasticity), making it essential to account for heteroscedastic noise in causal discovery. In this work, we explore heteroscedastic symmetric noise models (HSNMs), where the effect $Y$ is modeled as $Y = f(X) + \sigma(X)N$, with $X$ as the cause and $N$ as independent noise following a symmetric distribution. We introduce a novel criterion for identifying HSNMs based on the skewness of the score (i.e., the gradient of the log density) of the data distribution. This criterion establishes a computationally tractable measurement that is zero in the causal direction but nonzero in the anticausal direction, enabling the causal direction discovery. We extend this skewness-based criterion to the multivariate setting and propose SkewScore, an algorithm that handles heteroscedastic noise without requiring the extraction of exogenous noise. We also conduct a case study on the robustness of SkewScore in a bivariate model with a latent confounder, providing theoretical insights into its performance. Empirical studies further validate the effectiveness of the proposed method.

On the Identifiability of Sparse ICA without Assuming Non-Gaussianity

Independent component analysis (ICA) is a fundamental statistical tool used to reveal hidden generative processes from observed data. However, traditional ICA approaches struggle with the rotational invariance inherent in Gaussian distributions, often necessitating the assumption of non-Gaussianity in the underlying sources. This may limit their applicability in broader contexts. To accommodate Gaussian sources, we develop an identifiability theory that relies on second-order statistics without imposing further preconditions on the distribution of sources, by introducing novel assumptions on the connective structure from sources to observed variables. Different from recent work that focuses on potentially restrictive connective structures, our proposed assumption of structural variability is both considerably less restrictive and provably necessary. Furthermore, we propose two estimation methods based on second-order statistics and sparsity constraint. Experimental results are provided to validate our identifiability theory and estimation methods.

Continual Learning of Nonlinear Independent Representations

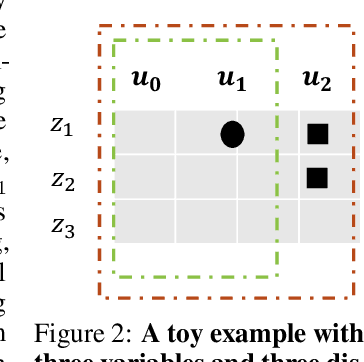
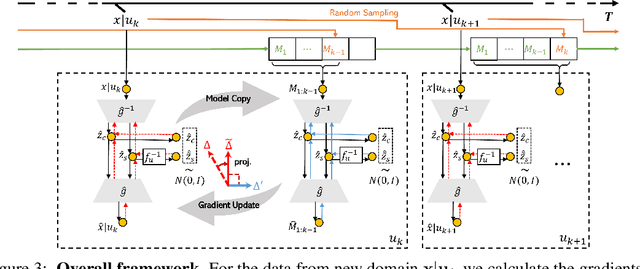
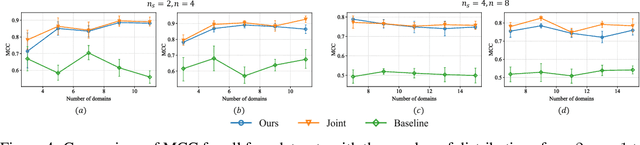
Identifying the causal relations between interested variables plays a pivotal role in representation learning as it provides deep insights into the dataset. Identifiability, as the central theme of this approach, normally hinges on leveraging data from multiple distributions (intervention, distribution shift, time series, etc.). Despite the exciting development in this field, a practical but often overlooked problem is: what if those distribution shifts happen sequentially? In contrast, any intelligence possesses the capacity to abstract and refine learned knowledge sequentially -- lifelong learning. In this paper, with a particular focus on the nonlinear independent component analysis (ICA) framework, we move one step forward toward the question of enabling models to learn meaningful (identifiable) representations in a sequential manner, termed continual causal representation learning. We theoretically demonstrate that model identifiability progresses from a subspace level to a component-wise level as the number of distributions increases. Empirically, we show that our method achieves performance comparable to nonlinear ICA methods trained jointly on multiple offline distributions and, surprisingly, the incoming new distribution does not necessarily benefit the identification of all latent variables.

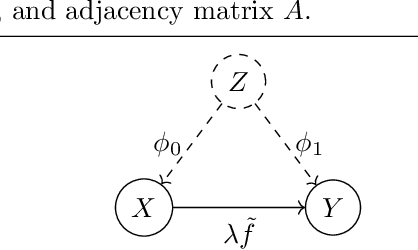
On the Parameter Identifiability of Partially Observed Linear Causal Models

Linear causal models are important tools for modeling causal dependencies and yet in practice, only a subset of the variables can be observed. In this paper, we examine the parameter identifiability of these models by investigating whether the edge coefficients can be recovered given the causal structure and partially observed data. Our setting is more general than that of prior research - we allow all variables, including both observed and latent ones, to be flexibly related, and we consider the coefficients of all edges, whereas most existing works focus only on the edges between observed variables. Theoretically, we identify three types of indeterminacy for the parameters in partially observed linear causal models. We then provide graphical conditions that are sufficient for all parameters to be identifiable and show that some of them are provably necessary. Methodologically, we propose a novel likelihood-based parameter estimation method that addresses the variance indeterminacy of latent variables in a specific way and can asymptotically recover the underlying parameters up to trivial indeterminacy. Empirical studies on both synthetic and real-world datasets validate our identifiability theory and the effectiveness of the proposed method in the finite-sample regime.

Local Causal Discovery with Linear non-Gaussian Cyclic Models

Local causal discovery is of great practical significance, as there are often situations where the discovery of the global causal structure is unnecessary, and the interest lies solely on a single target variable. Most existing local methods utilize conditional independence relations, providing only a partially directed graph, and assume acyclicity for the ground-truth structure, even though real-world scenarios often involve cycles like feedback mechanisms. In this work, we present a general, unified local causal discovery method with linear non-Gaussian models, whether they are cyclic or acyclic. We extend the application of independent component analysis from the global context to independent subspace analysis, enabling the exact identification of the equivalent local directed structures and causal strengths from the Markov blanket of the target variable. We also propose an alternative regression-based method in the particular acyclic scenarios. Our identifiability results are empirically validated using both synthetic and real-world datasets.