LLM-Mediated Guidance of MARL Systems

In complex multi-agent environments, achieving efficient learning and desirable behaviours is a significant challenge for Multi-Agent Reinforcement Learning (MARL) systems. This work explores the potential of combining MARL with Large Language Model (LLM)-mediated interventions to guide agents toward more desirable behaviours. Specifically, we investigate how LLMs can be used to interpret and facilitate interventions that shape the learning trajectories of multiple agents. We experimented with two types of interventions, referred to as controllers: a Natural Language (NL) Controller and a Rule-Based (RB) Controller. The NL Controller, which uses an LLM to simulate human-like interventions, showed a stronger impact than the RB Controller. Our findings indicate that agents particularly benefit from early interventions, leading to more efficient training and higher performance. Both intervention types outperform the baseline without interventions, highlighting the potential of LLM-mediated guidance to accelerate training and enhance MARL performance in challenging environments.

Re-evaluating Open-ended Evaluation of Large Language Models

Evaluation has traditionally focused on ranking candidates for a specific skill. Modern generalist models, such as Large Language Models (LLMs), decidedly outpace this paradigm. Open-ended evaluation systems, where candidate models are compared on user-submitted prompts, have emerged as a popular solution. Despite their many advantages, we show that the current Elo-based rating systems can be susceptible to and even reinforce biases in data, intentional or accidental, due to their sensitivity to redundancies. To address this issue, we propose evaluation as a 3-player game, and introduce novel game-theoretic solution concepts to ensure robustness to redundancy. We show that our method leads to intuitive ratings and provide insights into the competitive landscape of LLM development.

Deviation Ratings: A General, Clone-Invariant Rating Method

Many real-world multi-agent or multi-task evaluation scenarios can be naturally modelled as normal-form games due to inherent strategic (adversarial, cooperative, and mixed motive) interactions. These strategic interactions may be agentic (e.g. players trying to win), fundamental (e.g. cost vs quality), or complementary (e.g. niche finding and specialization). In such a formulation, it is the strategies (actions, policies, agents, models, tasks, prompts, etc.) that are rated. However, the rating problem is complicated by redundancy and complexity of N-player strategic interactions. Repeated or similar strategies can distort ratings for those that counter or complement them. Previous work proposed ``clone invariant'' ratings to handle such redundancies, but this was limited to two-player zero-sum (i.e. strictly competitive) interactions. This work introduces the first N-player general-sum clone invariant rating, called deviation ratings, based on coarse correlated equilibria. The rating is explored on several domains including LLMs evaluation.

Charting the Shapes of Stories with Game Theory

Stories are records of our experiences and their analysis reveals insights into the nature of being human. Successful analyses are often interdisciplinary, leveraging mathematical tools to extract structure from stories and insights from structure. Historically, these tools have been restricted to one dimensional charts and dynamic social networks; however, modern AI offers the possibility of identifying more fully the plot structure, character incentives, and, importantly, counterfactual plot lines that the story could have taken but did not take. In this work, we use AI to model the structure of stories as game-theoretic objects, amenable to quantitative analysis. This allows us to not only interrogate each character's decision making, but also possibly peer into the original author's conception of the characters' world. We demonstrate our proposed technique on Shakespeare's famous Romeo and Juliet. We conclude with a discussion of how our analysis could be replicated in broader contexts, including real-life scenarios.

Nash Equilibria via Stochastic Eigendecomposition

This work proposes a novel set of techniques for approximating a Nash equilibrium in a finite, normal-form game. It achieves this by constructing a new reformulation as solving a parameterized system of multivariate polynomials with tunable complexity. In doing so, it forges an itinerant loop from game theory to machine learning and back. We show a Nash equilibrium can be approximated with purely calls to stochastic, iterative variants of singular value decomposition and power iteration, with implications for biological plausibility. We provide pseudocode and experiments demonstrating solving for all equilibria of a general-sum game using only these readily available linear algebra tools.

Soft Condorcet Optimization for Ranking of General Agents

A common way to drive progress of AI models and agents is to compare their performance on standardized benchmarks. Comparing the performance of general agents requires aggregating their individual performances across a potentially wide variety of different tasks. In this paper, we describe a novel ranking scheme inspired by social choice frameworks, called Soft Condorcet Optimization (SCO), to compute the optimal ranking of agents: the one that makes the fewest mistakes in predicting the agent comparisons in the evaluation data. This optimal ranking is the maximum likelihood estimate when evaluation data (which we view as votes) are interpreted as noisy samples from a ground truth ranking, a solution to Condorcet's original voting system criteria. SCO ratings are maximal for Condorcet winners when they exist, which we show is not necessarily true for the classical rating system Elo. We propose three optimization algorithms to compute SCO ratings and evaluate their empirical performance. When serving as an approximation to the Kemeny-Young voting method, SCO rankings are on average 0 to 0.043 away from the optimal ranking in normalized Kendall-tau distance across 865 preference profiles from the PrefLib open ranking archive. In a simulated noisy tournament setting, SCO achieves accurate approximations to the ground truth ranking and the best among several baselines when 59\% or more of the preference data is missing. Finally, SCO ranking provides the best approximation to the optimal ranking, measured on held-out test sets, in a problem containing 52,958 human players across 31,049 games of the classic seven-player game of Diplomacy.

Convex Markov Games: A Framework for Fairness, Imitation, and Creativity in Multi-Agent Learning

Expert imitation, behavioral diversity, and fairness preferences give rise to preferences in sequential decision making domains that do not decompose additively across time. We introduce the class of convex Markov games that allow general convex preferences over occupancy measures. Despite infinite time horizon and strictly higher generality than Markov games, pure strategy Nash equilibria exist under strict convexity. Furthermore, equilibria can be approximated efficiently by performing gradient descent on an upper bound of exploitability. Our experiments imitate human choices in ultimatum games, reveal novel solutions to the repeated prisoner's dilemma, and find fair solutions in a repeated asymmetric coordination game. In the prisoner's dilemma, our algorithm finds a policy profile that deviates from observed human play only slightly, yet achieves higher per-player utility while also being three orders of magnitude less exploitable.

States as Strings as Strategies: Steering Language Models with Game-Theoretic Solvers

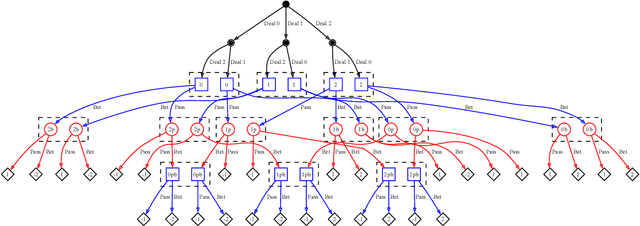
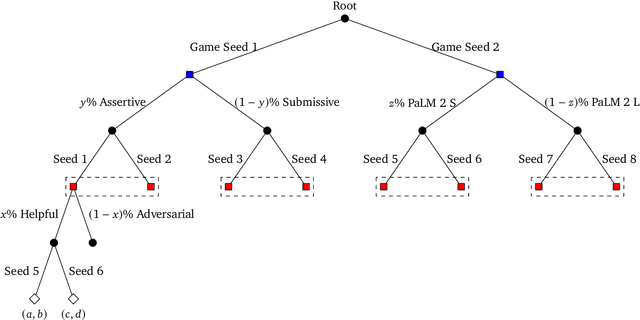
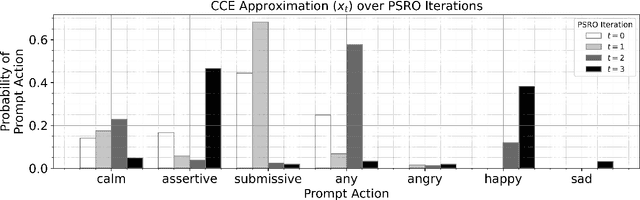
Game theory is the study of mathematical models of strategic interactions among rational agents. Language is a key medium of interaction for humans, though it has historically proven difficult to model dialogue and its strategic motivations mathematically. A suitable model of the players, strategies, and payoffs associated with linguistic interactions (i.e., a binding to the conventional symbolic logic of game theory) would enable existing game-theoretic algorithms to provide strategic solutions in the space of language. In other words, a binding could provide a route to computing stable, rational conversational strategies in dialogue. Large language models (LLMs) have arguably reached a point where their generative capabilities can enable realistic, human-like simulations of natural dialogue. By prompting them in various ways, we can steer their responses towards different output utterances. Leveraging the expressivity of natural language, LLMs can also help us quickly generate new dialogue scenarios, which are grounded in real world applications. In this work, we present one possible binding from dialogue to game theory as well as generalizations of existing equilibrium finding algorithms to this setting. In addition, by exploiting LLMs generation capabilities along with our proposed binding, we can synthesize a large repository of formally-defined games in which one can study and test game-theoretic solution concepts. We also demonstrate how one can combine LLM-driven game generation, game-theoretic solvers, and imitation learning to construct a process for improving the strategic capabilities of LLMs.

Combining Tree-Search, Generative Models, and Nash Bargaining Concepts in Game-Theoretic Reinforcement Learning

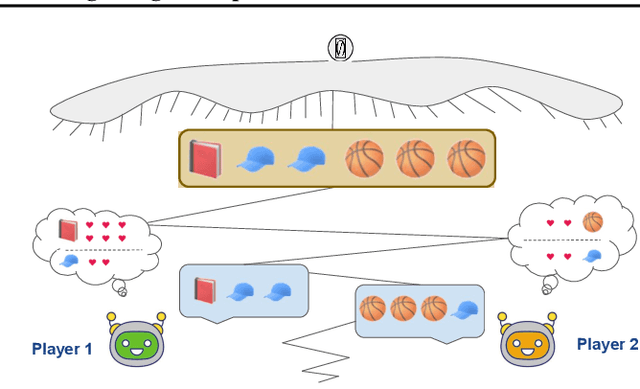
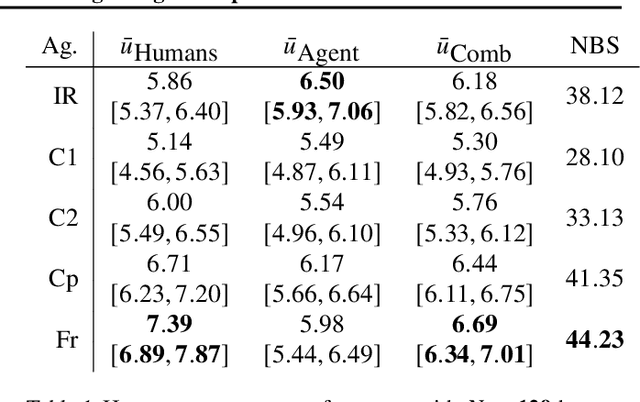
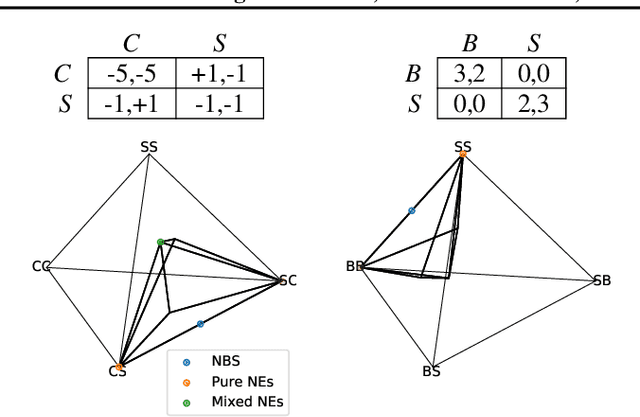
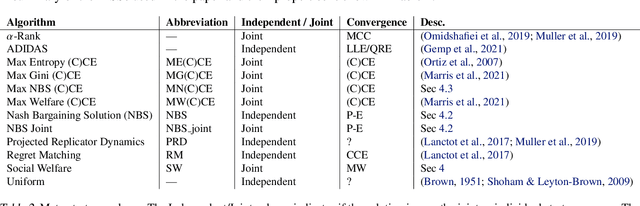
Multiagent reinforcement learning (MARL) has benefited significantly from population-based and game-theoretic training regimes. One approach, Policy-Space Response Oracles (PSRO), employs standard reinforcement learning to compute response policies via approximate best responses and combines them via meta-strategy selection. We augment PSRO by adding a novel search procedure with generative sampling of world states, and introduce two new meta-strategy solvers based on the Nash bargaining solution. We evaluate PSRO's ability to compute approximate Nash equilibrium, and its performance in two negotiation games: Colored Trails, and Deal or No Deal. We conduct behavioral studies where human participants negotiate with our agents ($N = 346$). We find that search with generative modeling finds stronger policies during both training time and test time, enables online Bayesian co-player prediction, and can produce agents that achieve comparable social welfare negotiating with humans as humans trading among themselves.

AlphaSnake: Policy Iteration on a Nondeterministic NP-hard Markov Decision Process

Reinforcement learning has recently been used to approach well-known NP-hard combinatorial problems in graph theory. Among these problems, Hamiltonian cycle problems are exceptionally difficult to analyze, even when restricted to individual instances of structurally complex graphs. In this paper, we use Monte Carlo Tree Search (MCTS), the search algorithm behind many state-of-the-art reinforcement learning algorithms such as AlphaZero, to create autonomous agents that learn to play the game of Snake, a game centered on properties of Hamiltonian cycles on grid graphs. The game of Snake can be formulated as a single-player discounted Markov Decision Process (MDP) where the agent must behave optimally in a stochastic environment. Determining the optimal policy for Snake, defined as the policy that maximizes the probability of winning - or win rate - with higher priority and minimizes the expected number of time steps to win with lower priority, is conjectured to be NP-hard. Performance-wise, compared to prior work in the Snake game, our algorithm is the first to achieve a win rate over $0.5$ (a uniform random policy achieves a win rate $< 2.57 \times 10^{-15}$), demonstrating the versatility of AlphaZero in approaching NP-hard environments.