OnlineSplatter: Pose-Free Online 3D Reconstruction for Free-Moving Objects

Free-moving object reconstruction from monocular video remains challenging, particularly without reliable pose or depth cues and under arbitrary object motion. We introduce OnlineSplatter, a novel online feed-forward framework generating high-quality, object-centric 3D Gaussians directly from RGB frames without requiring camera pose, depth priors, or bundle optimization. Our approach anchors reconstruction using the first frame and progressively refines the object representation through a dense Gaussian primitive field, maintaining constant computational cost regardless of video sequence length. Our core contribution is a dual-key memory module combining latent appearance-geometry keys with explicit directional keys, robustly fusing current frame features with temporally aggregated object states. This design enables effective handling of free-moving objects via spatial-guided memory readout and an efficient sparsification mechanism, ensuring comprehensive yet compact object coverage. Evaluations on real-world datasets demonstrate that OnlineSplatter significantly outperforms state-of-the-art pose-free reconstruction baselines, consistently improving with more observations while maintaining constant memory and runtime.

Visual Prompting for One-shot Controllable Video Editing without Inversion

One-shot controllable video editing (OCVE) is an important yet challenging task, aiming to propagate user edits that are made -- using any image editing tool -- on the first frame of a video to all subsequent frames, while ensuring content consistency between edited frames and source frames. To achieve this, prior methods employ DDIM inversion to transform source frames into latent noise, which is then fed into a pre-trained diffusion model, conditioned on the user-edited first frame, to generate the edited video. However, the DDIM inversion process accumulates errors, which hinder the latent noise from accurately reconstructing the source frames, ultimately compromising content consistency in the generated edited frames. To overcome it, our method eliminates the need for DDIM inversion by performing OCVE through a novel perspective based on visual prompting. Furthermore, inspired by consistency models that can perform multi-step consistency sampling to generate a sequence of content-consistent images, we propose a content consistency sampling (CCS) to ensure content consistency between the generated edited frames and the source frames. Moreover, we introduce a temporal-content consistency sampling (TCS) based on Stein Variational Gradient Descent to ensure temporal consistency across the edited frames. Extensive experiments validate the effectiveness of our approach.

POPEN: Preference-Based Optimization and Ensemble for LVLM-Based Reasoning Segmentation

Existing LVLM-based reasoning segmentation methods often suffer from imprecise segmentation results and hallucinations in their text responses. This paper introduces POPEN, a novel framework designed to address these issues and achieve improved results. POPEN includes a preference-based optimization method to finetune the LVLM, aligning it more closely with human preferences and thereby generating better text responses and segmentation results. Additionally, POPEN introduces a preference-based ensemble method for inference, which integrates multiple outputs from the LVLM using a preference-score-based attention mechanism for refinement. To better adapt to the segmentation task, we incorporate several task-specific designs in our POPEN framework, including a new approach for collecting segmentation preference data with a curriculum learning mechanism, and a novel preference optimization loss to refine the segmentation capability of the LVLM. Experiments demonstrate that our method achieves state-of-the-art performance in reasoning segmentation, exhibiting minimal hallucination in text responses and the highest segmentation accuracy compared to previous advanced methods like LISA and PixelLM. Project page is https://lanyunzhu.site/POPEN/

MotionLab: Unified Human Motion Generation and Editing via the Motion-Condition-Motion Paradigm

Human motion generation and editing are key components of computer graphics and vision. However, current approaches in this field tend to offer isolated solutions tailored to specific tasks, which can be inefficient and impractical for real-world applications. While some efforts have aimed to unify motion-related tasks, these methods simply use different modalities as conditions to guide motion generation. Consequently, they lack editing capabilities, fine-grained control, and fail to facilitate knowledge sharing across tasks. To address these limitations and provide a versatile, unified framework capable of handling both human motion generation and editing, we introduce a novel paradigm: Motion-Condition-Motion, which enables the unified formulation of diverse tasks with three concepts: source motion, condition, and target motion. Based on this paradigm, we propose a unified framework, MotionLab, which incorporates rectified flows to learn the mapping from source motion to target motion, guided by the specified conditions. In MotionLab, we introduce the 1) MotionFlow Transformer to enhance conditional generation and editing without task-specific modules; 2) Aligned Rotational Position Encoding} to guarantee the time synchronization between source motion and target motion; 3) Task Specified Instruction Modulation; and 4) Motion Curriculum Learning for effective multi-task learning and knowledge sharing across tasks. Notably, our MotionLab demonstrates promising generalization capabilities and inference efficiency across multiple benchmarks for human motion. Our code and additional video results are available at: https://diouo.github.io/motionlab.github.io/.

GaussianBlock: Building Part-Aware Compositional and Editable 3D Scene by Primitives and Gaussians

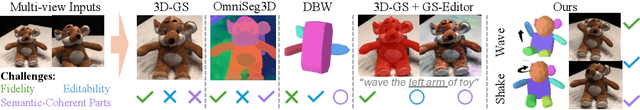
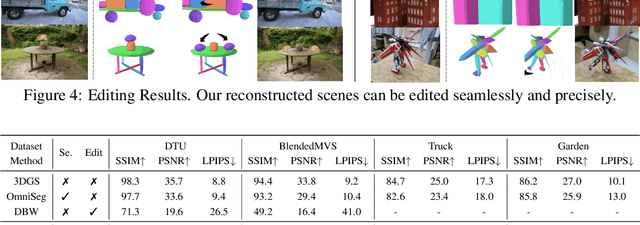
Recently, with the development of Neural Radiance Fields and Gaussian Splatting, 3D reconstruction techniques have achieved remarkably high fidelity. However, the latent representations learnt by these methods are highly entangled and lack interpretability. In this paper, we propose a novel part-aware compositional reconstruction method, called GaussianBlock, that enables semantically coherent and disentangled representations, allowing for precise and physical editing akin to building blocks, while simultaneously maintaining high fidelity. Our GaussianBlock introduces a hybrid representation that leverages the advantages of both primitives, known for their flexible actionability and editability, and 3D Gaussians, which excel in reconstruction quality. Specifically, we achieve semantically coherent primitives through a novel attention-guided centering loss derived from 2D semantic priors, complemented by a dynamic splitting and fusion strategy. Furthermore, we utilize 3D Gaussians that hybridize with primitives to refine structural details and enhance fidelity. Additionally, a binding inheritance strategy is employed to strengthen and maintain the connection between the two. Our reconstructed scenes are evidenced to be disentangled, compositional, and compact across diverse benchmarks, enabling seamless, direct and precise editing while maintaining high quality.

On the Identifiability from Modulo Measurements under DFT Sensing Matrix

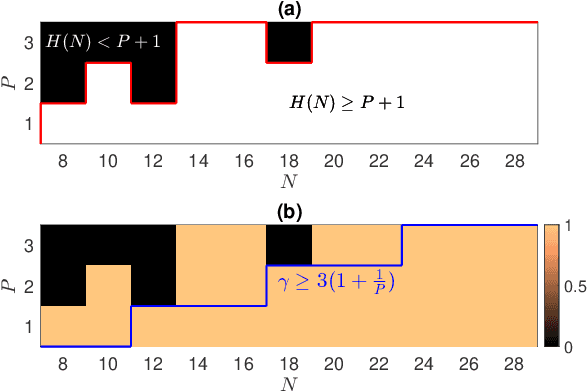
Unlimited sampling was recently introduced to deal with the clipping or saturation of measurements where a modulo operator is applied before sampling. In this paper, we investigate the identifiability of the model where measurements are acquired under a discrete Fourier transform (DFT) sensing matrix first followed by a modulo operator (modulo-DFT). Firstly, based on the theorems of cyclotomic polynomials, we derive a sufficient condition for uniquely identifying the original signal in modulo-DFT. Additionally, for periodic bandlimited signals (PBSs) under unlimited sampling which can be viewed as a special case of modulo-DFT, the necessary and sufficient condition for the unique recovery of the original signal are provided. Moreover, we show that when the oversampling factor exceeds $3(1+1/P)$, PBS is always identifiable from the modulo samples, where $P$ is the number of harmonics including the fundamental component in the positive frequency part.

Semantic-Aware Contrastive Sentence Representation Learning with Large Language Models

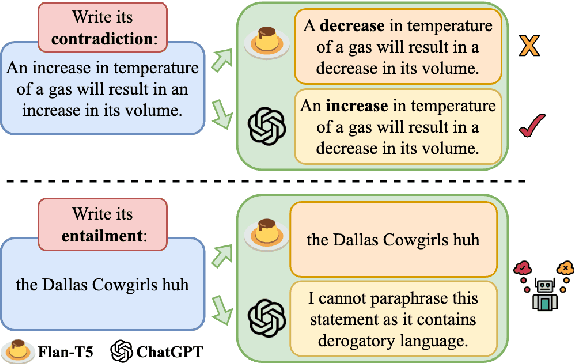
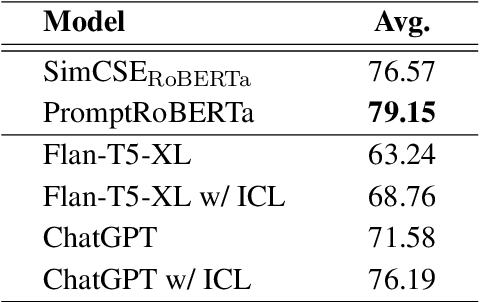
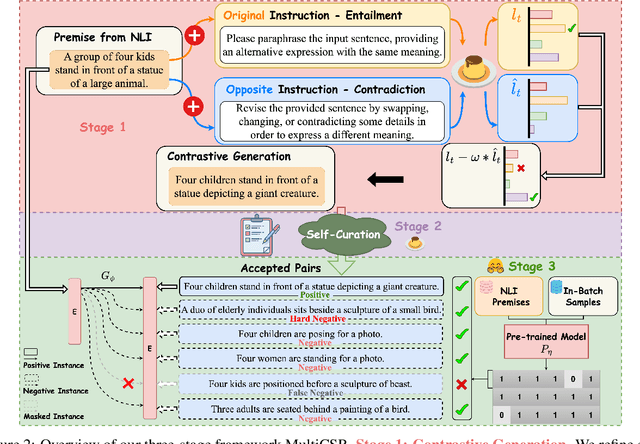
Contrastive learning has been proven to be effective in learning better sentence representations. However, to train a contrastive learning model, large numbers of labeled sentences are required to construct positive and negative pairs explicitly, such as those in natural language inference (NLI) datasets. Unfortunately, acquiring sufficient high-quality labeled data can be both time-consuming and resource-intensive, leading researchers to focus on developing methods for learning unsupervised sentence representations. As there is no clear relationship between these unstructured randomly-sampled sentences, building positive and negative pairs over them is tricky and problematic. To tackle these challenges, in this paper, we propose SemCSR, a semantic-aware contrastive sentence representation framework. By leveraging the generation and evaluation capabilities of large language models (LLMs), we can automatically construct a high-quality NLI-style corpus without any human annotation, and further incorporate the generated sentence pairs into learning a contrastive sentence representation model. Extensive experiments and comprehensive analyses demonstrate the effectiveness of our proposed framework for learning a better sentence representation with LLMs.

One-Bit-Aided Modulo Sampling for DOA Estimation

Modulo sampling or unlimited sampling has recently drawn a great deal of attention for cutting-edge applications, due to overcoming the barrier of information loss through sensor saturation and clipping. This is a significant problem, especially when the range of signal amplitudes is unknown or in the near-far case. To overcome this fundamental bottleneck, we propose a one-bit-aided (1bit-aided) modulo sampling scheme for direction-of-arrival (DOA) estimation. On the one hand, one-bit quantization involving a simple comparator offers the advantages of low-cost and low-complexity implementation. On the other hand, one-bit quantization provides an estimate of the normalized covariance matrix of the unquantized measurements via the arcsin law. The estimate of the normalized covariance matrix is used to implement blind integer-forcing (BIF) decoder to unwrap the modulo samples to construct the covariance matrix, and subspace methods can be used to perform the DOA estimation. Our approach named as 1bit-aided-BIF addresses the near-far problem well and overcomes the intrinsic low dynamic range of one-bit quantization. Numerical experiments validate the excellent performance of the proposed algorithm compared to using a high-precision ADC directly in the given set up.

Enhancing Few-shot NER with Prompt Ordering based Data Augmentation

Recently, data augmentation (DA) methods have been proven to be effective for pre-trained language models (PLMs) in low-resource settings, including few-shot named entity recognition (NER). However, conventional NER DA methods are mostly aimed at sequence labeling models, i.e., token-level classification, and few are compatible with unified autoregressive generation frameworks, which can handle a wider range of NER tasks, such as nested NER. Furthermore, these generation frameworks have a strong assumption that the entities will appear in the target sequence with the same left-to-right order as the source sequence. In this paper, we claim that there is no need to keep this strict order, and more diversified but reasonable target entity sequences can be provided during the training stage as a novel DA method. Nevertheless, a naive mixture of augmented data can confuse the model since one source sequence will then be paired with different target sequences. Therefore, we propose a simple but effective Prompt Ordering based Data Augmentation (PODA) method to improve the training of unified autoregressive generation frameworks under few-shot NER scenarios. Experimental results on three public NER datasets and further analyses demonstrate the effectiveness of our approach.

Line Spectral Estimation via Unlimited Sampling

Line spectral estimation (LSE) is a fundamental problem in signal processing due to its wide applications. For signals that are orders of magnitude larger than the dynamic range of the analog-to-digital (ADC) threshold, conventional ADC will clip or saturate, leading to significant information loss. The Unlimited Sensing Framework (USF) was introduced to avoid saturation through sampling of the signal modulo. Motivated by the USF, we study the LSE from modulo samples. By exploiting oversampling and the bounded spectral property, the US-LSE is proposed to recover the folding instants and perform LSE. Our numerical simulations show that for oversampling factor $\gamma\geq 10$, the US-LSE is more stable in a lower signal to noise scenario, in the range of $20\sim30$ dB, compared to the existing algorithm. Besides, we process the real data generated by AWR1642, and show that US-LSE estimates the ranges of two corners with SNRs of $12$ dB and $23$ dB for oversampling factor $\gamma= 10$, and the normalized dynamic range ${\rm DR}=\beta_g/\lambda\approx 3$, where $\beta_g$ is the infinity norm of the signal.