Deep Optimal Sensor Placement for Black Box Stochastic Simulations

Selecting cost-effective optimal sensor configurations for subsequent inference of parameters in black-box stochastic systems faces significant computational barriers. We propose a novel and robust approach, modelling the joint distribution over input parameters and solution with a joint energy-based model, trained on simulation data. Unlike existing simulation-based inference approaches, which must be tied to a specific set of point evaluations, we learn a functional representation of parameters and solution. This is used as a resolution-independent plug-and-play surrogate for the joint distribution, which can be conditioned over any set of points, permitting an efficient approach to sensor placement. We demonstrate the validity of our framework on a variety of stochastic problems, showing that our method provides highly informative sensor locations at a lower computational cost compared to conventional approaches.

Modelling Global Trade with Optimal Transport

Global trade is shaped by a complex mix of factors beyond supply and demand, including tangible variables like transport costs and tariffs, as well as less quantifiable influences such as political and economic relations. Traditionally, economists model trade using gravity models, which rely on explicit covariates but often struggle to capture these subtler drivers of trade. In this work, we employ optimal transport and a deep neural network to learn a time-dependent cost function from data, without imposing a specific functional form. This approach consistently outperforms traditional gravity models in accuracy while providing natural uncertainty quantification. Applying our framework to global food and agricultural trade, we show that the global South suffered disproportionately from the war in Ukraine's impact on wheat markets. We also analyze the effects of free-trade agreements and trade disputes with China, as well as Brexit's impact on British trade with Europe, uncovering hidden patterns that trade volumes alone cannot reveal.

Towards Multilevel Modelling of Train Passing Events on the Staffordshire Bridge

We suggest a multilevel model, to represent aggregate train-passing events from the Staffordshire bridge monitoring system. We formulate a combined model from simple units, representing strain envelopes (of each train passing) for two types of commuter train. The measurements are treated as a longitudinal dataset and represented with a (low-rank approximation) hierarchical Gaussian process. For each unit in the combined model, we encode domain expertise as boundary condition constraints and work towards a general representation of the strain response. Looking forward, this should allow for the simulation of train types that were previously unobserved in the training data. For example, trains with more passengers or freights with a heavier payload. The strain event simulations are valuable since they can inform further experiments (including FEM calibration, fatigue analysis, or design) to test the bridge in hypothesised scenarios.

Encoding Domain Expertise into Multilevel Models for Source Location

Data from populations of systems are prevalent in many industrial applications. Machines and infrastructure are increasingly instrumented with sensing systems, emitting streams of telemetry data with complex interdependencies. In practice, data-centric monitoring procedures tend to consider these assets (and respective models) as distinct -- operating in isolation and associated with independent data. In contrast, this work captures the statistical correlations and interdependencies between models of a group of systems. Utilising a Bayesian multilevel approach, the value of data can be extended, since the population can be considered as a whole, rather than constituent parts. Most interestingly, domain expertise and knowledge of the underlying physics can be encoded in the model at the system, subgroup, or population level. We present an example of acoustic emission (time-of-arrival) mapping for source location, to illustrate how multilevel models naturally lend themselves to representing aggregate systems in engineering. In particular, we focus on constraining the combined models with domain knowledge to enhance transfer learning and enable further insights at the population level.

Expanding Accurate Person Recognition to New Altitudes and Ranges: The BRIAR Dataset

Face recognition technology has advanced significantly in recent years due largely to the availability of large and increasingly complex training datasets for use in deep learning models. These datasets, however, typically comprise images scraped from news sites or social media platforms and, therefore, have limited utility in more advanced security, forensics, and military applications. These applications require lower resolution, longer ranges, and elevated viewpoints. To meet these critical needs, we collected and curated the first and second subsets of a large multi-modal biometric dataset designed for use in the research and development (R&D) of biometric recognition technologies under extremely challenging conditions. Thus far, the dataset includes more than 350,000 still images and over 1,300 hours of video footage of approximately 1,000 subjects. To collect this data, we used Nikon DSLR cameras, a variety of commercial surveillance cameras, specialized long-rage R&D cameras, and Group 1 and Group 2 UAV platforms. The goal is to support the development of algorithms capable of accurately recognizing people at ranges up to 1,000 m and from high angles of elevation. These advances will include improvements to the state of the art in face recognition and will support new research in the area of whole-body recognition using methods based on gait and anthropometry. This paper describes methods used to collect and curate the dataset, and the dataset's characteristics at the current stage.

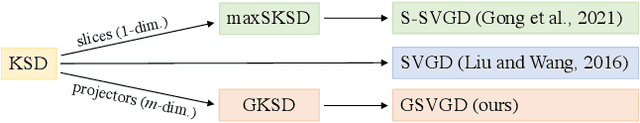
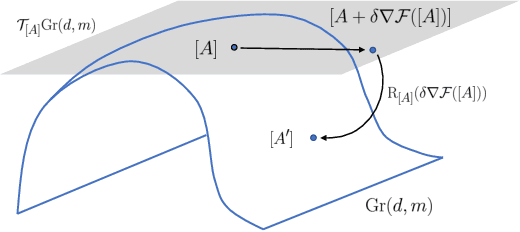
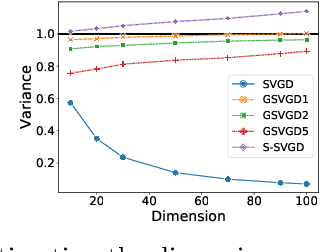
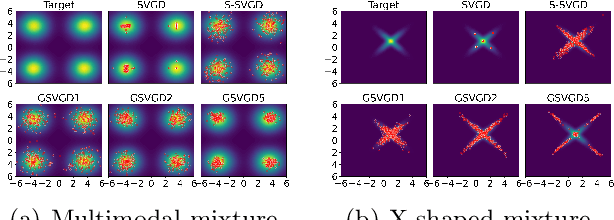
Grassmann Stein Variational Gradient Descent

Stein variational gradient descent (SVGD) is a deterministic particle inference algorithm that provides an efficient alternative to Markov chain Monte Carlo. However, SVGD has been found to suffer from variance underestimation when the dimensionality of the target distribution is high. Recent developments have advocated projecting both the score function and the data onto real lines to sidestep this issue, although this can severely overestimate the epistemic (model) uncertainty. In this work, we propose Grassmann Stein variational gradient descent (GSVGD) as an alternative approach, which permits projections onto arbitrary dimensional subspaces. Compared with other variants of SVGD that rely on dimensionality reduction, GSVGD updates the projectors simultaneously for the score function and the data, and the optimal projectors are determined through a coupled Grassmann-valued diffusion process which explores favourable subspaces. Both our theoretical and experimental results suggest that GSVGD enjoys efficient state-space exploration in high-dimensional problems that have an intrinsic low-dimensional structure.

$\mathcal{F}$-EBM: Energy Based Learning of Functional Data

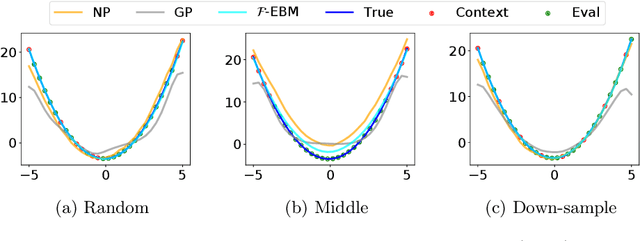
Energy-Based Models (EBMs) have proven to be a highly effective approach for modelling densities on finite-dimensional spaces. Their ability to incorporate domain-specific choices and constraints into the structure of the model through composition make EBMs an appealing candidate for applications in physics, biology and computer vision and various other fields. In this work, we present a novel class of EBM which is able to learn distributions of functions (such as curves or surfaces) from functional samples evaluated at finitely many points. Two unique challenges arise in the functional context. Firstly, training data is often not evaluated along a fixed set of points. Secondly, steps must be taken to control the behaviour of the model between evaluation points, to mitigate overfitting. The proposed infinite-dimensional EBM employs a latent Gaussian process, which is weighted spectrally by an energy function parameterised with a neural network. The resulting EBM has the ability to utilize irregularly sampled training data and can output predictions at any resolution, providing an effective approach to up-scaling functional data. We demonstrate the efficacy of our proposed approach for modelling a range of datasets, including data collected from Standard and Poor's 500 (S\&P) and UK National grid.

Density Estimation from Schlieren Images through Machine Learning

This study proposes a radically alternate approach for extracting quantitative information from schlieren images. The method uses a scaled, derivative enhanced Gaussian process model to obtain true density estimates from two corresponding schlieren images with the knife-edge at horizontal and vertical orientations. We illustrate our approach on schlieren images taken from a wind tunnel sting model, and a supersonic aircraft in flight.