AtlasD: Automatic Local Symmetry Discovery

Existing symmetry discovery methods predominantly focus on global transformations across the entire system or space, but they fail to consider the symmetries in local neighborhoods. This may result in the reported symmetry group being a misrepresentation of the true symmetry. In this paper, we formalize the notion of local symmetry as atlas equivariance. Our proposed pipeline, automatic local symmetry discovery (AtlasD), recovers the local symmetries of a function by training local predictor networks and then learning a Lie group basis to which the predictors are equivariant. We demonstrate AtlasD is capable of discovering local symmetry groups with multiple connected components in top-quark tagging and partial differential equation experiments. The discovered local symmetry is shown to be a useful inductive bias that improves the performance of downstream tasks in climate segmentation and vision tasks.

IAEmu: Learning Galaxy Intrinsic Alignment Correlations

The intrinsic alignments (IA) of galaxies, a key contaminant in weak lensing analyses, arise from correlations in galaxy shapes driven by tidal interactions and galaxy formation processes. Accurate IA modeling is essential for robust cosmological inference, but current approaches rely on perturbative methods that break down on nonlinear scales or on expensive simulations. We introduce IAEmu, a neural network-based emulator that predicts the galaxy position-position ($\xi$), position-orientation ($\omega$), and orientation-orientation ($\eta$) correlation functions and their uncertainties using mock catalogs based on the halo occupation distribution (HOD) framework. Compared to simulations, IAEmu achieves ~3% average error for $\xi$ and ~5% for $\omega$, while capturing the stochasticity of $\eta$ without overfitting. The emulator provides both aleatoric and epistemic uncertainties, helping identify regions where predictions may be less reliable. We also demonstrate generalization to non-HOD alignment signals by fitting to IllustrisTNG hydrodynamical simulation data. As a fully differentiable neural network, IAEmu enables $\sim$10,000$\times$ speed-ups in mapping HOD parameters to correlation functions on GPUs, compared to CPU-based simulations. This acceleration facilitates inverse modeling via gradient-based sampling, making IAEmu a powerful surrogate model for galaxy bias and IA studies with direct applications to Stage IV weak lensing surveys.

Denoising Hamiltonian Network for Physical Reasoning

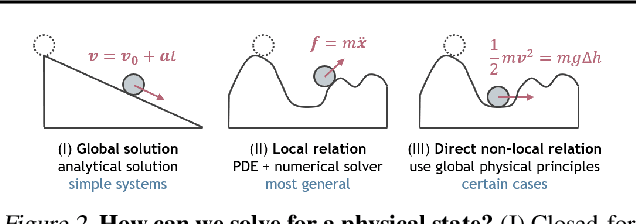
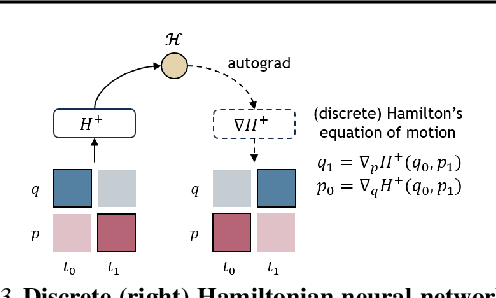
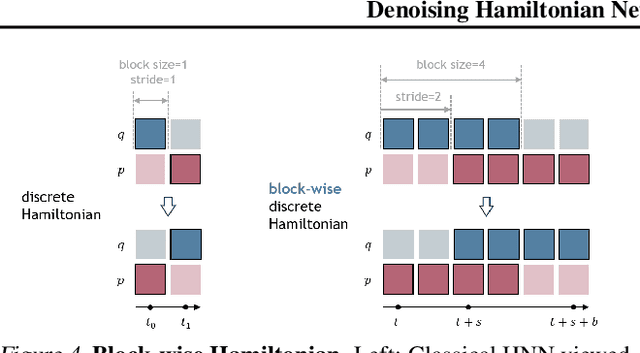
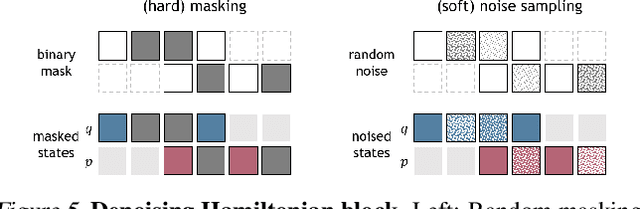
Machine learning frameworks for physical problems must capture and enforce physical constraints that preserve the structure of dynamical systems. Many existing approaches achieve this by integrating physical operators into neural networks. While these methods offer theoretical guarantees, they face two key limitations: (i) they primarily model local relations between adjacent time steps, overlooking longer-range or higher-level physical interactions, and (ii) they focus on forward simulation while neglecting broader physical reasoning tasks. We propose the Denoising Hamiltonian Network (DHN), a novel framework that generalizes Hamiltonian mechanics operators into more flexible neural operators. DHN captures non-local temporal relationships and mitigates numerical integration errors through a denoising mechanism. DHN also supports multi-system modeling with a global conditioning mechanism. We demonstrate its effectiveness and flexibility across three diverse physical reasoning tasks with distinct inputs and outputs.

Hierarchical Equivariant Policy via Frame Transf

Recent advances in hierarchical policy learning highlight the advantages of decomposing systems into high-level and low-level agents, enabling efficient long-horizon reasoning and precise fine-grained control. However, the interface between these hierarchy levels remains underexplored, and existing hierarchical methods often ignore domain symmetry, resulting in the need for extensive demonstrations to achieve robust performance. To address these issues, we propose Hierarchical Equivariant Policy (HEP), a novel hierarchical policy framework. We propose a frame transfer interface for hierarchical policy learning, which uses the high-level agent's output as a coordinate frame for the low-level agent, providing a strong inductive bias while retaining flexibility. Additionally, we integrate domain symmetries into both levels and theoretically demonstrate the system's overall equivariance. HEP achieves state-of-the-art performance in complex robotic manipulation tasks, demonstrating significant improvements in both simulation and real-world settings.

Coarse-to-Fine 3D Keyframe Transporter

Recent advances in Keyframe Imitation Learning (IL) have enabled learning-based agents to solve a diverse range of manipulation tasks. However, most approaches ignore the rich symmetries in the problem setting and, as a consequence, are sample-inefficient. This work identifies and utilizes the bi-equivariant symmetry within Keyframe IL to design a policy that generalizes to transformations of both the workspace and the objects grasped by the gripper. We make two main contributions: First, we analyze the bi-equivariance properties of the keyframe action scheme and propose a Keyframe Transporter derived from the Transporter Networks, which evaluates actions using cross-correlation between the features of the grasped object and the features of the scene. Second, we propose a computationally efficient coarse-to-fine SE(3) action evaluation scheme for reasoning the intertwined translation and rotation action. The resulting method outperforms strong Keyframe IL baselines by an average of >10% on a wide range of simulation tasks, and by an average of 55% in 4 physical experiments.

Reducing the Sensitivity of Neural Physics Simulators to Mesh Topology via Pretraining

Meshes are used to represent complex objects in high fidelity physics simulators across a variety of domains, such as radar sensing and aerodynamics. There is growing interest in using neural networks to accelerate physics simulations, and also a growing body of work on applying neural networks directly to irregular mesh data. Since multiple mesh topologies can represent the same object, mesh augmentation is typically required to handle topological variation when training neural networks. Due to the sensitivity of physics simulators to small changes in mesh shape, it is challenging to use these augmentations when training neural network-based physics simulators. In this work, we show that variations in mesh topology can significantly reduce the performance of neural network simulators. We evaluate whether pretraining can be used to address this issue, and find that employing an established autoencoder pretraining technique with graph embedding models reduces the sensitivity of neural network simulators to variations in mesh topology. Finally, we highlight future research directions that may further reduce neural simulator sensitivity to mesh topology.

MatrixNet: Learning over symmetry groups using learned group representations

Group theory has been used in machine learning to provide a theoretically grounded approach for incorporating known symmetry transformations in tasks from robotics to protein modeling. In these applications, equivariant neural networks use known symmetry groups with predefined representations to learn over geometric input data. We propose MatrixNet, a neural network architecture that learns matrix representations of group element inputs instead of using predefined representations. MatrixNet achieves higher sample efficiency and generalization over several standard baselines in prediction tasks over the several finite groups and the Artin braid group. We also show that MatrixNet respects group relations allowing generalization to group elements of greater word length than in the training set.

Equivariant Action Sampling for Reinforcement Learning and Planning

Reinforcement learning (RL) algorithms for continuous control tasks require accurate sampling-based action selection. Many tasks, such as robotic manipulation, contain inherent problem symmetries. However, correctly incorporating symmetry into sampling-based approaches remains a challenge. This work addresses the challenge of preserving symmetry in sampling-based planning and control, a key component for enhancing decision-making efficiency in RL. We introduce an action sampling approach that enforces the desired symmetry. We apply our proposed method to a coordinate regression problem and show that the symmetry aware sampling method drastically outperforms the naive sampling approach. We furthermore develop a general framework for sampling-based model-based planning with Model Predictive Path Integral (MPPI). We compare our MPPI approach with standard sampling methods on several continuous control tasks. Empirical demonstrations across multiple continuous control environments validate the effectiveness of our approach, showcasing the importance of symmetry preservation in sampling-based action selection.

Approximate Equivariance in Reinforcement Learning

Equivariant neural networks have shown great success in reinforcement learning, improving sample efficiency and generalization when there is symmetry in the task. However, in many problems, only approximate symmetry is present, which makes imposing exact symmetry inappropriate. Recently, approximately equivariant networks have been proposed for supervised classification and modeling physical systems. In this work, we develop approximately equivariant algorithms in reinforcement learning (RL). We define approximately equivariant MDPs and theoretically characterize the effect of approximate equivariance on the optimal Q function. We propose novel RL architectures using relaxed group convolutions and experiment on several continuous control domains and stock trading with real financial data. Our results demonstrate that approximate equivariance matches prior work when exact symmetries are present, and outperforms them when domains exhibit approximate symmetry. As an added byproduct of these techniques, we observe increased robustness to noise at test time.

Relaxed Equivariant Graph Neural Networks

3D Euclidean symmetry equivariant neural networks have demonstrated notable success in modeling complex physical systems. We introduce a framework for relaxed $E(3)$ graph equivariant neural networks that can learn and represent symmetry breaking within continuous groups. Building on the existing e3nn framework, we propose the use of relaxed weights to allow for controlled symmetry breaking. We show empirically that these relaxed weights learn the correct amount of symmetry breaking.