A Self-Ensemble Inspired Approach for Effective Training of Binary-Weight Spiking Neural Networks

Spiking Neural Networks (SNNs) are a promising approach to low-power applications on neuromorphic hardware due to their energy efficiency. However, training SNNs is challenging because of the non-differentiable spike generation function. To address this issue, the commonly used approach is to adopt the backpropagation through time framework, while assigning the gradient of the non-differentiable function with some surrogates. Similarly, Binary Neural Networks (BNNs) also face the non-differentiability problem and rely on approximating gradients. However, the deep relationship between these two fields and how their training techniques can benefit each other has not been systematically researched. Furthermore, training binary-weight SNNs is even more difficult. In this work, we present a novel perspective on the dynamics of SNNs and their close connection to BNNs through an analysis of the backpropagation process. We demonstrate that training a feedforward SNN can be viewed as training a self-ensemble of a binary-activation neural network with noise injection. Drawing from this new understanding of SNN dynamics, we introduce the Self-Ensemble Inspired training method for (Binary-Weight) SNNs (SEI-BWSNN), which achieves high-performance results with low latency even for the case of the 1-bit weights. Specifically, we leverage a structure of multiple shortcuts and a knowledge distillation-based training technique to improve the training of (binary-weight) SNNs. Notably, by binarizing FFN layers in a Transformer architecture, our approach achieves 82.52% accuracy on ImageNet with only 2 time steps, indicating the effectiveness of our methodology and the potential of binary-weight SNNs.

DNT: a Deeply Normalized Transformer that can be trained by Momentum SGD

Transformers have become the de facto backbone of modern deep learning, yet their training typically demands an advanced optimizer with adaptive learning rate like AdamW, rather than a momentum SGDW (mSGDW). Previous works show that it is mainly due to a heavy-tailed distribution of the gradients. In this paper, we introduce a Deeply Normalized Transformer (DNT), which is meticulously engineered to overcome this limitation enabling seamless training with vanilla mSGDW while yielding comparable performance to the Transformers trained via AdamW. To be specific, in DNT, we strategically integrate normalization techniques at proper positions in the Transformers to effectively modulate the Jacobian matrices of each layer, balance the influence of weights, activations, and their interactions, and thus enable the distributions of gradients concentrated. We provide both theoretical justifications of the normalization technique used in our DNT and extensive empirical evaluation on two popular Transformer architectures to validate that: a) DNT outperforms its counterparts (\ie, ViT and GPT), and b) DNT can be effectively trained with vanilla mSGDW.

Stepsize anything: A unified learning rate schedule for budgeted-iteration training

The expanding computational costs and limited resources underscore the critical need for budgeted-iteration training, which aims to achieve optimal learning within predetermined iteration budgets.While learning rate schedules fundamentally govern the performance of different networks and tasks, particularly in budgeted-iteration scenarios, their design remains largely heuristic, lacking theoretical foundations.In addition, the optimal learning rate schedule requires extensive trial-and-error selection, making the training process inefficient.In this work, we propose the Unified Budget-Aware (UBA) schedule, a theoretically grounded learning rate schedule that consistently outperforms commonly-used schedules among diverse architectures and tasks under different constrained training budgets.First, we bridge the gap by constructing a novel training budget-aware optimization framework, which explicitly accounts for the robustness to landscape curvature variations.From this framework, we derive the UBA schedule, controlled by a single hyper-parameter $\varphi$ that provides a trade-off between flexibility and simplicity, eliminating the need for per-network numerical optimization. Moreover, we establish a theoretical connection between $\varphi$ and the condition number, adding interpretation and justification to our approach. Besides, we prove the convergence for different values of $\varphi$.We offer practical guidelines for its selection via theoretical analysis and empirical results.xtensive experimental results show that UBA \textit{consistently surpasses} the commonly-used schedules across diverse vision and language tasks, spanning network architectures (e.g., ResNet, OLMo) and scales, under different training-iteration budgets.

Governing Equation Discovery from Data Based on Differential Invariants

The explicit governing equation is one of the simplest and most intuitive forms for characterizing physical laws. However, directly discovering partial differential equations (PDEs) from data poses significant challenges, primarily in determining relevant terms from a vast search space. Symmetry, as a crucial prior knowledge in scientific fields, has been widely applied in tasks such as designing equivariant networks and guiding neural PDE solvers. In this paper, we propose a pipeline for governing equation discovery based on differential invariants, which can losslessly reduce the search space of existing equation discovery methods while strictly adhering to symmetry. Specifically, we compute the set of differential invariants corresponding to the infinitesimal generators of the symmetry group and select them as the relevant terms for equation discovery. Taking DI-SINDy (SINDy based on Differential Invariants) as an example, we demonstrate that its success rate and accuracy in PDE discovery surpass those of other symmetry-informed governing equation discovery methods across a series of PDEs.

TransDF: Time-Series Forecasting Needs Transformed Label Alignment

Training time-series forecasting models presents unique challenges in designing effective learning objectives. Existing methods predominantly utilize the temporal mean squared error, which faces two critical challenges: (1) label autocorrelation, which leads to bias from the label sequence likelihood; (2) excessive amount of tasks, which increases with the forecast horizon and complicates optimization. To address these challenges, we propose Transform-enhanced Direct Forecast (TransDF), which transforms the label sequence into decorrelated components with discriminated significance. Models are trained to align the most significant components, thereby effectively mitigating label autocorrelation and reducing task amount. Extensive experiments demonstrate that TransDF achieves state-of-the-art performance and is compatible with various forecasting models. Code is available at https://anonymous.4open.science/r/TransDF-88CF.

On the $O(\frac{\sqrt{d}}{K^{1/4}})$ Convergence Rate of AdamW Measured by $\ell_1$ Norm

As the default optimizer for training large language models, AdamW has achieved remarkable success in deep learning. However, its convergence behavior is not theoretically well-understood. This paper establishes the convergence rate $\frac{1}{K}\sum_{k=1}^KE\left[\|\nabla f(x^k)\|_1\right]\leq O(\frac{\sqrt{d}C}{K^{1/4}})$ for AdamW measured by $\ell_1$ norm, where $K$ represents the iteration number, $d$ denotes the model dimension, and $C$ matches the constant in the optimal convergence rate of SGD. Theoretically, we have $E\left[\|\nabla f(x)\|_1\right]\geq\sqrt{\frac{2d}{\pi}}E\left[\|\nabla f(x)\|_2\right]$ when each element of $\nabla f(x)$ is generated from Gaussian distribution $\mathcal N(0,1)$. Empirically, our experimental results on real-world deep learning tasks reveal $\|\nabla f(x)\|_1=\varTheta(\sqrt{d})\|\nabla f(x)\|_2$. Both support that our convergence rate can be considered to be analogous to the optimal $\frac{1}{K}\sum_{k=1}^KE\left[\|\nabla f(x^k)\|_2\right]\leq O(\frac{C}{K^{1/4}})$ convergence rate of SGD.

VACT: A Video Automatic Causal Testing System and a Benchmark

With the rapid advancement of text-conditioned Video Generation Models (VGMs), the quality of generated videos has significantly improved, bringing these models closer to functioning as ``*world simulators*'' and making real-world-level video generation more accessible and cost-effective. However, the generated videos often contain factual inaccuracies and lack understanding of fundamental physical laws. While some previous studies have highlighted this issue in limited domains through manual analysis, a comprehensive solution has not yet been established, primarily due to the absence of a generalized, automated approach for modeling and assessing the causal reasoning of these models across diverse scenarios. To address this gap, we propose VACT: an **automated** framework for modeling, evaluating, and measuring the causal understanding of VGMs in real-world scenarios. By combining causal analysis techniques with a carefully designed large language model assistant, our system can assess the causal behavior of models in various contexts without human annotation, which offers strong generalization and scalability. Additionally, we introduce multi-level causal evaluation metrics to provide a detailed analysis of the causal performance of VGMs. As a demonstration, we use our framework to benchmark several prevailing VGMs, offering insight into their causal reasoning capabilities. Our work lays the foundation for systematically addressing the causal understanding deficiencies in VGMs and contributes to advancing their reliability and real-world applicability.

Empowering LLMs with Logical Reasoning: A Comprehensive Survey

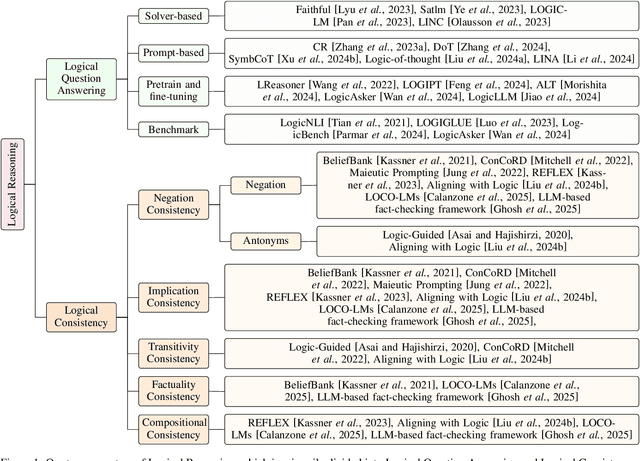
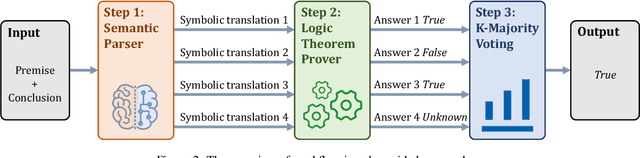
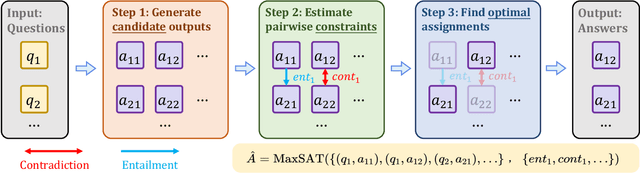
Large language models (LLMs) have achieved remarkable successes on various natural language tasks. However, recent studies have found that there are still significant challenges to the logical reasoning abilities of LLMs. This paper summarizes and categorizes the main challenges into two aspects: (1) Logical question answering, LLMs often fail to generate the correct answer within complex logical problem which requires sophisticated deductive, inductive or abductive reasoning given a collection of premises and constrains. (2) Logical consistency, LLMs are prone to producing responses contradicting themselves across different questions. For example, a state-of-the-art Macaw question-answering LLM answers Yes to both questions Is a magpie a bird? and Does a bird have wings? but answers No to Does a magpie have wings?. To facilitate this research direction, we comprehensively investigate the most cutting-edge methods and propose detailed taxonomies of these methods. Specifically, to accurately answer complex logic questions, previous methods can be categorized based on reliance on external solvers, prompts, pretraining, and fine-tuning. To avoid logical contradictions, we discuss concepts and solutions of various logical consistencies, including implication, negation, transitivity, factuality consistency, and their composites. In addition, we review commonly used benchmark datasets and evaluation metrics, and discuss promising research directions, such as extensions to modal logic to account for uncertainty, and efficient algorithms satisfying multiple logical consistencies simultaneously.

High-Rank Irreducible Cartesian Tensor Decomposition and Bases of Equivariant Spaces

Irreducible Cartesian tensors (ICTs) play a crucial role in the design of equivariant graph neural networks, as well as in theoretical chemistry and chemical physics. Meanwhile, the design space of available linear operations on tensors that preserve symmetry presents a significant challenge. The ICT decomposition and a basis of this equivariant space are difficult to obtain for high-order tensors. After decades of research, we recently achieve an explicit ICT decomposition for $n=5$ \citep{bonvicini2024irreducible} with factorial time/space complexity. This work, for the first time, obtains decomposition matrices for ICTs up to rank $n=9$ with reduced and affordable complexity, by constructing what we call path matrices. The path matrices are obtained via performing chain-like contraction with Clebsch-Gordan matrices following the parentage scheme. We prove and leverage that the concatenation of path matrices is an orthonormal change-of-basis matrix between the Cartesian tensor product space and the spherical direct sum spaces. Furthermore, we identify a complete orthogonal basis for the equivariant space, rather than a spanning set \citep{pearce2023brauer}, through this path matrices technique. We further extend our result to the arbitrary tensor product and direct sum spaces, enabling free design between different spaces while keeping symmetry. The Python code is available in https://github.com/ShihaoShao-GH/ICT-decomposition-and-equivariant-bases where the $n=6,\dots,9$ ICT decomposition matrices are obtained in 1s, 3s, 11s, and 4m32s, respectively.

Free the Design Space of Equivariant Graph Neural Networks: High-Rank Irreducible Cartesian Tensor Decomposition and Bases of Equivariant Spaces

Irreducible Cartesian tensors (ICTs) play a crucial role in the design of equivariant graph neural networks, as well as in theoretical chemistry and chemical physics. Meanwhile, the design space of available linear operations on tensors that preserve symmetry presents a significant challenge. The ICT decomposition and a basis of this equivariant space are difficult to obtain for high-order tensors. After decades of research, we recently achieve an explicit ICT decomposition for $n=5$ \citep{bonvicini2024irreducible} with factorial time/space complexity. This work, for the first time, obtains decomposition matrices for ICTs up to rank $n=9$ with reduced and affordable complexity, by constructing what we call path matrices. The path matrices are obtained via performing chain-like contraction with Clebsch-Gordan matrices following the parentage scheme. We prove and leverage that the concatenation of path matrices is an orthonormal change-of-basis matrix between the Cartesian tensor product space and the spherical direct sum spaces. Furthermore, we identify a complete orthogonal basis for the equivariant space, rather than a spanning set \citep{pearce2023brauer}, through this path matrices technique. We further extend our result to the arbitrary tensor product and direct sum spaces, enabling free design between different spaces while keeping symmetry. The Python code is available in the appendix where the $n=6,\dots,9$ ICT decomposition matrices are obtained in <0.1s, 0.5s, 1s, 3s, 11s, and 4m32s, respectively.