First-Choice Maximality Meets Ex-ante and Ex-post Fairness

For the assignment problem where multiple indivisible items are allocated to a group of agents given their ordinal preferences, we design randomized mechanisms that satisfy first-choice maximality (FCM), i.e., maximizing the number of agents assigned their first choices, together with Pareto efficiency (PE). Our mechanisms also provide guarantees of ex-ante and ex-post fairness. The generalized eager Boston mechanism is ex-ante envy-free, and ex-post envy-free up to one item (EF1). The generalized probabilistic Boston mechanism is also ex-post EF1, and satisfies ex-ante efficiency instead of fairness. We also show that no strategyproof mechanism satisfies ex-post PE, EF1, and FCM simultaneously. In doing so, we expand the frontiers of simultaneously providing efficiency and both ex-ante and ex-post fairness guarantees for the assignment problem.

Anti-Malware Sandbox Games

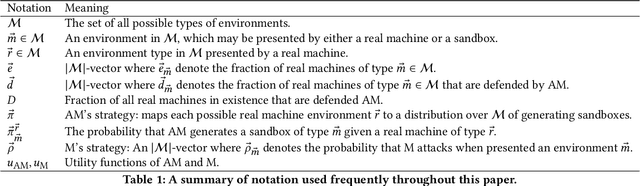
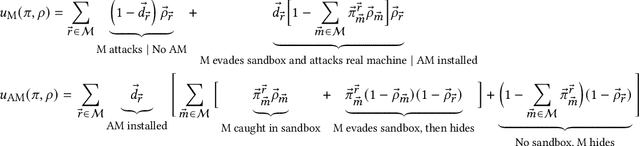
We develop a game theoretic model of malware protection using the state-of-the-art sandbox method, to characterize and compute optimal defense strategies for anti-malware. We model the strategic interaction between developers of malware (M) and anti-malware (AM) as a two player game, where AM commits to a strategy of generating sandbox environments, and M responds by choosing to either attack or hide malicious activity based on the environment it senses. We characterize the condition for AM to protect all its machines, and identify conditions under which an optimal AM strategy can be computed efficiently. For other cases, we provide a quadratically constrained quadratic program (QCQP)-based optimization framework to compute the optimal AM strategy. In addition, we identify a natural and easy to compute strategy for AM, which as we show empirically, achieves AM utility that is close to the optimal AM utility, in equilibrium.

Favoring Eagerness for Remaining Items: Achieving Efficient and Fair Assignments

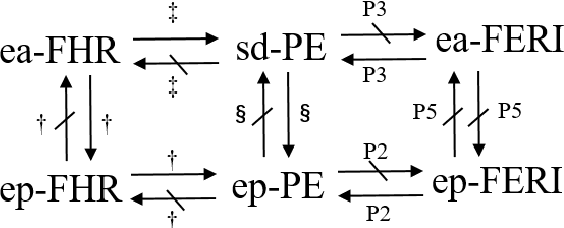
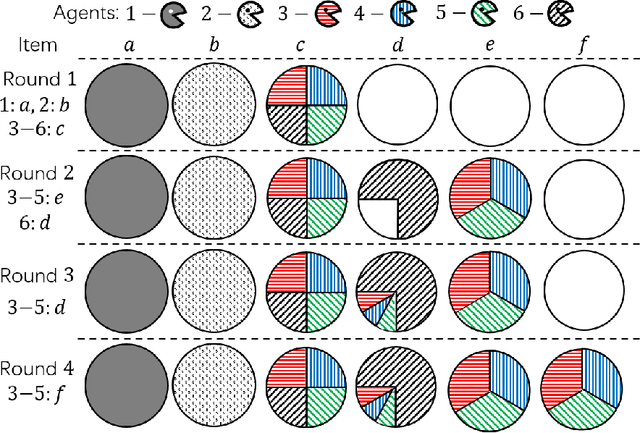
In the assignment problem, items must be assigned to agents who have unit demands, based on agents' ordinal preferences. Often the goal is to design a mechanism that is both fair and efficient. In this paper, we first prove that, unfortunately, the desirable efficiency notions rank-maximality, ex-post favoring-higher-ranks, and ex-ante favoring-higher-ranks, which aim to allocate each item to agents who rank it highest over all the items, are incompatible with the desirable fairness notions strong equal treatment of equals (SETE) and sd-weak-envy-freeness (sd-WEF) simultaneously. In light of this, we propose novel properties of efficiency based on a subtly different notion to favoring higher ranks, by favoring "eagerness" for remaining items and aiming to guarantee that each item is allocated to agents who rank it highest among remaining items. Specifically, we propose ex-post favoring-eagerness-for-remaining-items (ep-FERI) and ex-ante favoring-eagerness-for-remaining-items (ea-FERI). We prove that the eager Boston mechanism satisfies ep-FERI and sd-WSP and that the uniform probabilistic respecting eagerness mechanism satisfies ea-FERI. We also prove that both mechanisms satisfy SETE and sd-WEF, and show that no mechanism can satisfy stronger versions of envy-freeness and strategyproofness while simultaneously maintaining SETE, and either ep-FERI or ea-FERI.

Sequential Mechanisms for Multi-type Resource Allocation

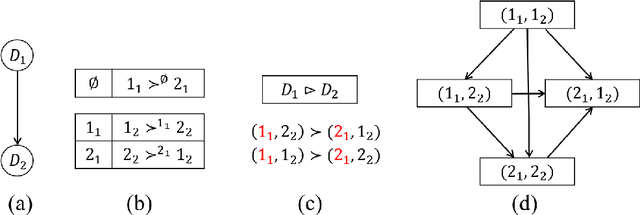
Several resource allocation problems involve multiple types of resources, with a different agency being responsible for "locally" allocating the resources of each type, while a central planner wishes to provide a guarantee on the properties of the final allocation given agents' preferences. We study the relationship between properties of the local mechanisms, each responsible for assigning all of the resources of a designated type, and the properties of a sequential mechanism which is composed of these local mechanisms, one for each type, applied sequentially, under lexicographic preferences, a well studied model of preferences over multiple types of resources in artificial intelligence and economics. We show that when preferences are O-legal, meaning that agents share a common importance order on the types, sequential mechanisms satisfy the desirable properties of anonymity, neutrality, non-bossiness, or Pareto-optimality if and only if every local mechanism also satisfies the same property, and they are applied sequentially according to the order O. Our main results are that under O-legal lexicographic preferences, every mechanism satisfying strategyproofness and a combination of these properties must be a sequential composition of local mechanisms that are also strategyproof, and satisfy the same combinations of properties.

Learning Desirable Matchings From Partial Preferences

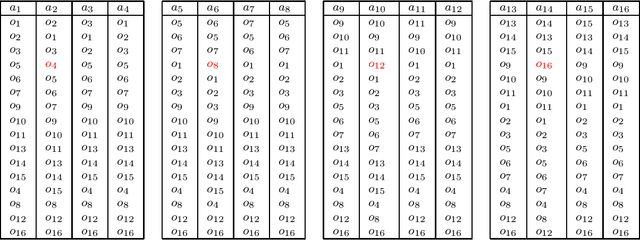
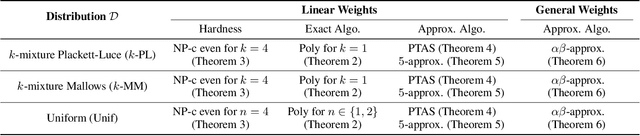
We study the classic problem of matching $n$ agents to $n$ objects, where the agents have ranked preferences over the objects. We focus on two popular desiderata from the matching literature: Pareto optimality and rank-maximality. Instead of asking the agents to report their complete preferences, our goal is to learn a desirable matching from partial preferences, specifically a matching that is necessarily Pareto optimal (NPO) or necessarily rank-maximal (NRM) under any completion of the partial preferences. We focus on the top-$k$ model in which agents reveal a prefix of their preference rankings. We design efficient algorithms to check if a given matching is NPO or NRM, and to check whether such a matching exists given top-$k$ partial preferences. We also study online algorithms to elicit partial preferences adaptively, and prove bounds on their competitive ratio.

Probabilistic Serial Mechanism for Multi-Type Resource Allocation

In multi-type resource allocation (MTRA) problems, there are p $\ge$ 2 types of items, and n agents, who each demand one unit of items of each type, and have strict linear preferences over bundles consisting of one item of each type. For MTRAs with indivisible items, our first result is an impossibility theorem that is in direct contrast to the single type (p = 1) setting: No mechanism, the output of which is always decomposable into a probability distribution over discrete assignments (where no item is split between agents), can satisfy both sd-efficiency and sd-envy-freeness. To circumvent this impossibility result, we consider the natural assumption of lexicographic preference, and provide an extension of the probabilistic serial (PS), called lexicographic probabilistic serial (LexiPS).We prove that LexiPS satisfies sd-efficiency and sd-envy-freeness, retaining the desirable properties of PS. Moreover, LexiPS satisfies sd-weak-strategyproofness when agents are not allowed to misreport their importance orders. For MTRAs with divisible items, we show that the existing multi-type probabilistic serial (MPS) mechanism satisfies the stronger efficiency notion of lexi-efficiency, and is sd-envy-free under strict linear preferences, and sd-weak-strategyproof under lexicographic preferences. We also prove that MPS can be characterized both by leximin-ptimality and by item-wise ordinal fairness, and the family of eating algorithms which MPS belongs to can be characterized by no-generalized-cycle condition.

Multi-type Resource Allocation with Partial Preferences

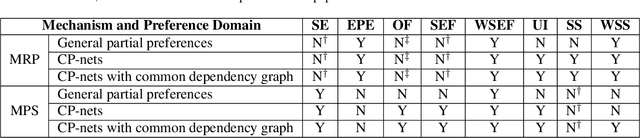
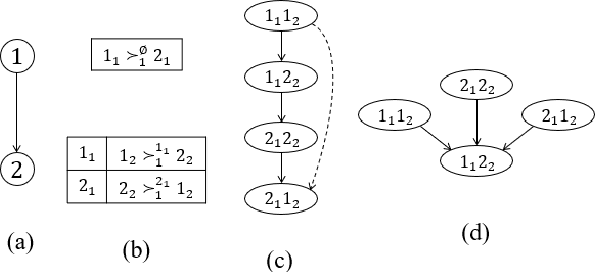
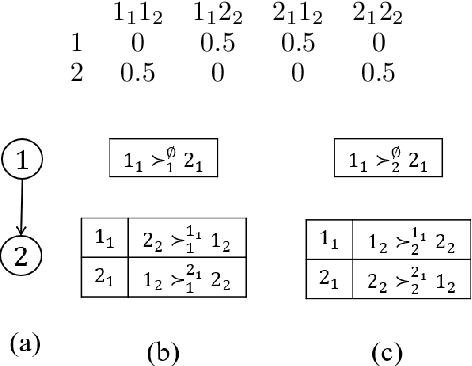
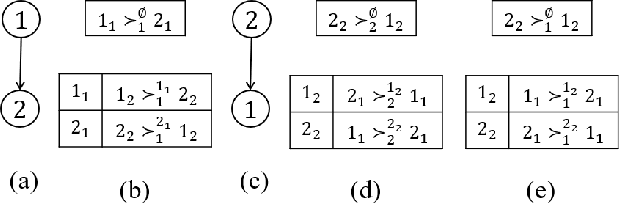
We propose multi-type probabilistic serial (MPS) and multi-type random priority (MRP) as extensions of the well known PS and RP mechanisms to the multi-type resource allocation problem (MTRA) with partial preferences. In our setting, there are multiple types of divisible items, and a group of agents who have partial order preferences over bundles consisting of one item of each type. We show that for the unrestricted domain of partial order preferences, no mechanism satisfies both sd-efficiency and sd-envy-freeness. Notwithstanding this impossibility result, our main message is positive: When agents' preferences are represented by acyclic CP-nets, MPS satisfies sd-efficiency, sd-envy-freeness, ordinal fairness, and upper invariance, while MRP satisfies ex-post-efficiency, sd-strategy-proofness, and upper invariance, recovering the properties of PS and RP.

Minimizing Time-to-Rank: A Learning and Recommendation Approach

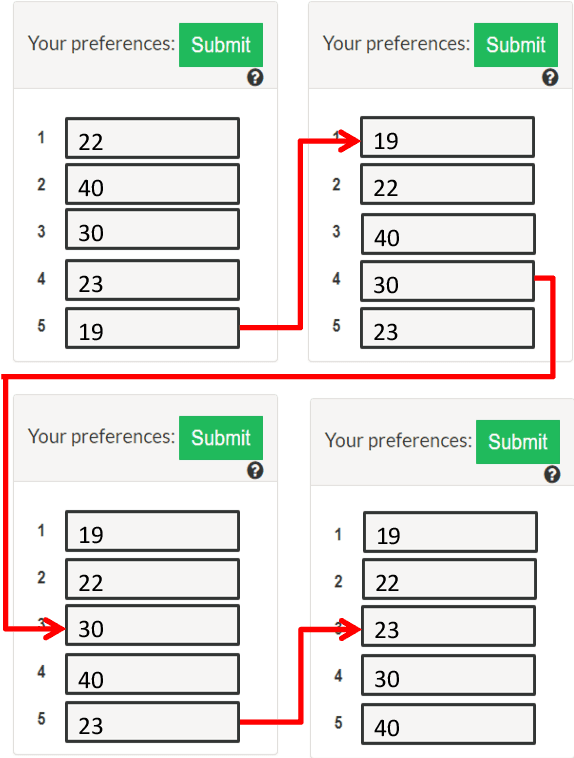
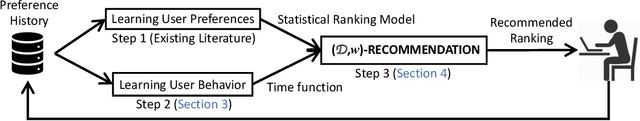
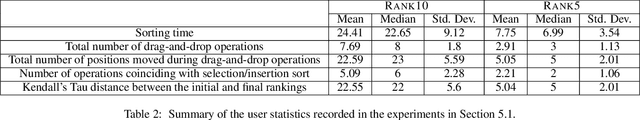
Consider the following problem faced by an online voting platform: A user is provided with a list of alternatives, and is asked to rank them in order of preference using only drag-and-drop operations. The platform's goal is to recommend an initial ranking that minimizes the time spent by the user in arriving at her desired ranking. We develop the first optimization framework to address this problem, and make theoretical as well as practical contributions. On the practical side, our experiments on Amazon Mechanical Turk provide two interesting insights about user behavior: First, that users' ranking strategies closely resemble selection or insertion sort, and second, that the time taken for a drag-and-drop operation depends linearly on the number of positions moved. These insights directly motivate our theoretical model of the optimization problem. We show that computing an optimal recommendation is NP-hard, and provide exact and approximation algorithms for a variety of special cases of the problem. Experimental evaluation on MTurk shows that, compared to a random recommendation strategy, the proposed approach reduces the (average) time-to-rank by up to 50%.

Practical Algorithms for Multi-Stage Voting Rules with Parallel Universes Tiebreaking

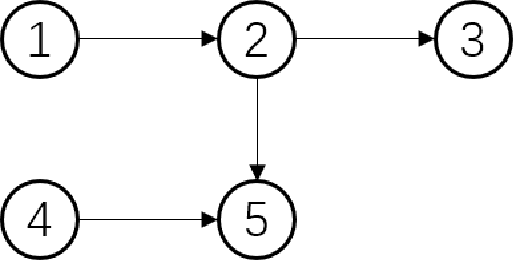
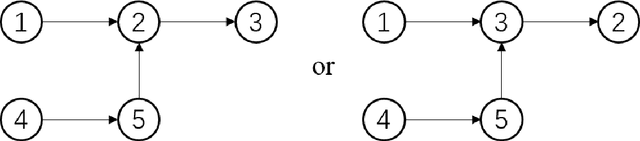
STV and ranked pairs (RP) are two well-studied voting rules for group decision-making. They proceed in multiple rounds, and are affected by how ties are broken in each round. However, the literature is surprisingly vague about how ties should be broken. We propose the first algorithms for computing the set of alternatives that are winners under some tiebreaking mechanism under STV and RP, which is also known as parallel-universes tiebreaking (PUT). Unfortunately, PUT-winners are NP-complete to compute under STV and RP, and standard search algorithms from AI do not apply. We propose multiple DFS-based algorithms along with pruning strategies, heuristics, sampling and machine learning to prioritize search direction to significantly improve the performance. We also propose novel ILP formulations for PUT-winners under STV and RP, respectively. Experiments on synthetic and real-world data show that our algorithms are overall faster than ILP.

Practical Algorithms for STV and Ranked Pairs with Parallel Universes Tiebreaking

STV and ranked pairs (RP) are two well-studied voting rules for group decision-making. They proceed in multiple rounds, and are affected by how ties are broken in each round. However, the literature is surprisingly vague about how ties should be broken. We propose the first algorithms for computing the set of alternatives that are winners under some tiebreaking mechanism under STV and RP, which is also known as parallel-universes tiebreaking (PUT). Unfortunately, PUT-winners are NP-complete to compute under STV and RP, and standard search algorithms from AI do not apply. We propose multiple DFS-based algorithms along with pruning strategies and heuristics to prioritize search direction to significantly improve the performance using machine learning. We also propose novel ILP formulations for PUT-winners under STV and RP, respectively. Experiments on synthetic and real-world data show that our algorithms are overall significantly faster than ILP, while there are a few cases where ILP is significantly faster for RP.