Generalization error of min-norm interpolators in transfer learning

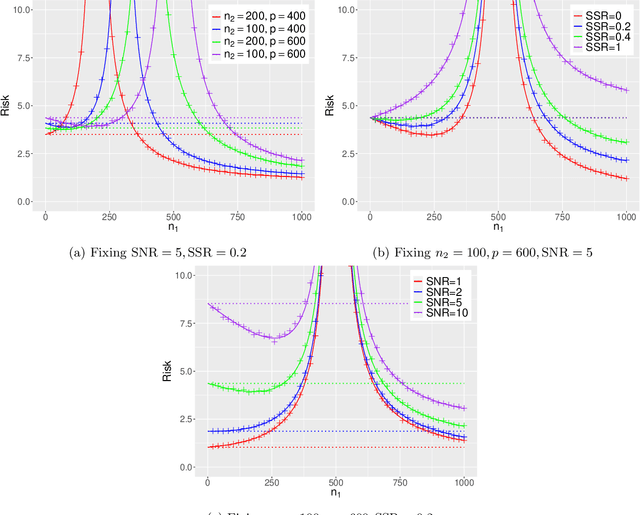
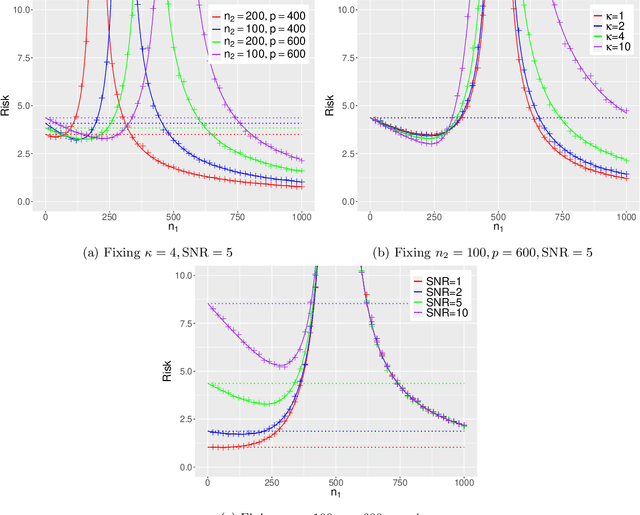
This paper establishes the generalization error of pooled min-$\ell_2$-norm interpolation in transfer learning where data from diverse distributions are available. Min-norm interpolators emerge naturally as implicit regularized limits of modern machine learning algorithms. Previous work characterized their out-of-distribution risk when samples from the test distribution are unavailable during training. However, in many applications, a limited amount of test data may be available during training, yet properties of min-norm interpolation in this setting are not well-understood. We address this gap by characterizing the bias and variance of pooled min-$\ell_2$-norm interpolation under covariate and model shifts. The pooled interpolator captures both early fusion and a form of intermediate fusion. Our results have several implications: under model shift, for low signal-to-noise ratio (SNR), adding data always hurts. For higher SNR, transfer learning helps as long as the shift-to-signal (SSR) ratio lies below a threshold that we characterize explicitly. By consistently estimating these ratios, we provide a data-driven method to determine: (i) when the pooled interpolator outperforms the target-based interpolator, and (ii) the optimal number of target samples that minimizes the generalization error. Under covariate shift, if the source sample size is small relative to the dimension, heterogeneity between between domains improves the risk, and vice versa. We establish a novel anisotropic local law to achieve these characterizations, which may be of independent interest in random matrix theory. We supplement our theoretical characterizations with comprehensive simulations that demonstrate the finite-sample efficacy of our results.

ROTI-GCV: Generalized Cross-Validation for right-ROTationally Invariant Data

Two key tasks in high-dimensional regularized regression are tuning the regularization strength for good predictions and estimating the out-of-sample risk. It is known that the standard approach -- $k$-fold cross-validation -- is inconsistent in modern high-dimensional settings. While leave-one-out and generalized cross-validation remain consistent in some high-dimensional cases, they become inconsistent when samples are dependent or contain heavy-tailed covariates. To model structured sample dependence and heavy tails, we use right-rotationally invariant covariate distributions - a crucial concept from compressed sensing. In the common modern proportional asymptotics regime where the number of features and samples grow comparably, we introduce a new framework, ROTI-GCV, for reliably performing cross-validation. Along the way, we propose new estimators for the signal-to-noise ratio and noise variance under these challenging conditions. We conduct extensive experiments that demonstrate the power of our approach and its superiority over existing methods.

Predictive Inference in Multi-environment Scenarios

We address the challenge of constructing valid confidence intervals and sets in problems of prediction across multiple environments. We investigate two types of coverage suitable for these problems, extending the jackknife and split-conformal methods to show how to obtain distribution-free coverage in such non-traditional, hierarchical data-generating scenarios. Our contributions also include extensions for settings with non-real-valued responses and a theory of consistency for predictive inference in these general problems. We demonstrate a novel resizing method to adapt to problem difficulty, which applies both to existing approaches for predictive inference with hierarchical data and the methods we develop; this reduces prediction set sizes using limited information from the test environment, a key to the methods' practical performance, which we evaluate through neurochemical sensing and species classification datasets.

Spectrum-Aware Adjustment: A New Debiasing Framework with Applications to Principal Components Regression

We introduce a new debiasing framework for high-dimensional linear regression that bypasses the restrictions on covariate distributions imposed by modern debiasing technology. We study the prevalent setting where the number of features and samples are both large and comparable. In this context, state-of-the-art debiasing technology uses a degrees-of-freedom correction to remove shrinkage bias of regularized estimators and conduct inference. However, this method requires that the observed samples are i.i.d., the covariates follow a mean zero Gaussian distribution, and reliable covariance matrix estimates for observed features are available. This approach struggles when (i) covariates are non-Gaussian with heavy tails or asymmetric distributions, (ii) rows of the design exhibit heterogeneity or dependencies, and (iii) reliable feature covariance estimates are lacking. To address these, we develop a new strategy where the debiasing correction is a rescaled gradient descent step (suitably initialized) with step size determined by the spectrum of the sample covariance matrix. Unlike prior work, we assume that eigenvectors of this matrix are uniform draws from the orthogonal group. We show this assumption remains valid in diverse situations where traditional debiasing fails, including designs with complex row-column dependencies, heavy tails, asymmetric properties, and latent low-rank structures. We establish asymptotic normality of our proposed estimator (centered and scaled) under various convergence notions. Moreover, we develop a consistent estimator for its asymptotic variance. Lastly, we introduce a debiased Principal Component Regression (PCR) technique using our Spectrum-Aware approach. In varied simulations and real data experiments, we observe that our method outperforms degrees-of-freedom debiasing by a margin.

Multi-Study Boosting: Theoretical Considerations for Merging vs. Ensembling

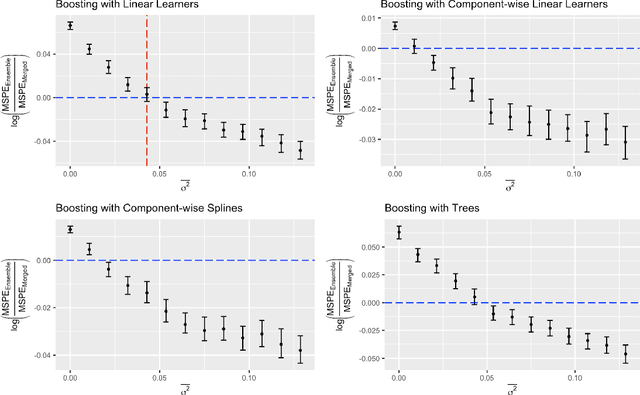
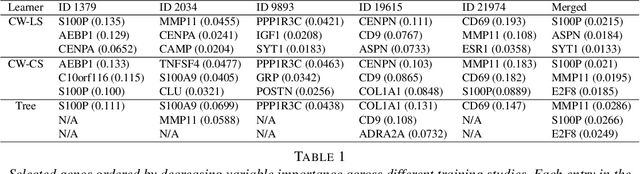
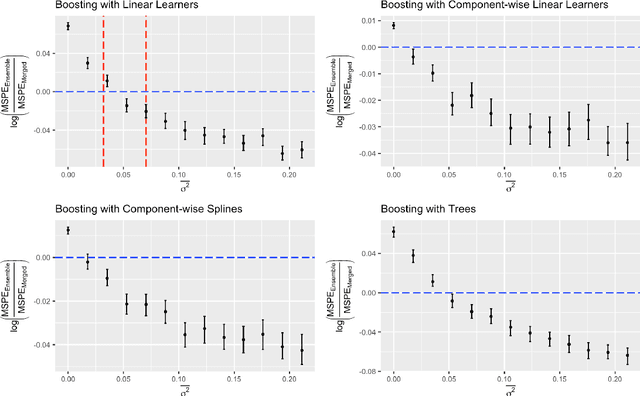
Cross-study replicability is a powerful model evaluation criterion that emphasizes generalizability of predictions. When training cross-study replicable prediction models, it is critical to decide between merging and treating the studies separately. We study boosting algorithms in the presence of potential heterogeneity in predictor-outcome relationships across studies and compare two multi-study learning strategies: 1) merging all the studies and training a single model, and 2) multi-study ensembling, which involves training a separate model on each study and ensembling the resulting predictions. In the regression setting, we provide theoretical guidelines based on an analytical transition point to determine whether it is more beneficial to merge or to ensemble for boosting with linear learners. In addition, we characterize a bias-variance decomposition of estimation error for boosting with component-wise linear learners. We verify the theoretical transition point result in simulation and illustrate how it can guide the decision on merging vs. ensembling in an application to breast cancer gene expression data.

A New Central Limit Theorem for the Augmented IPW Estimator: Variance Inflation, Cross-Fit Covariance and Beyond

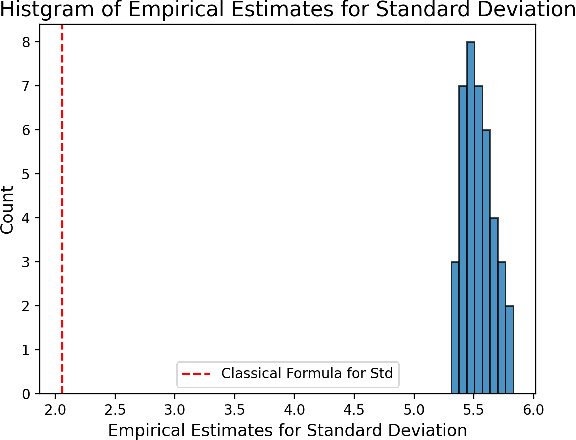
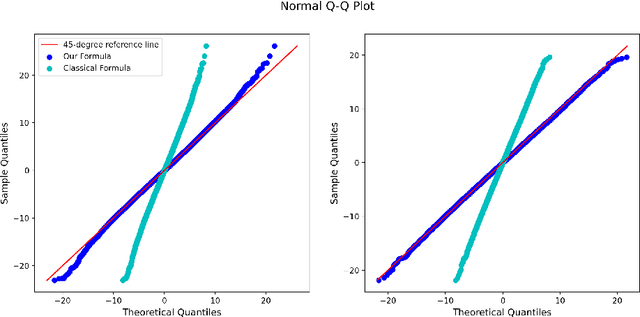
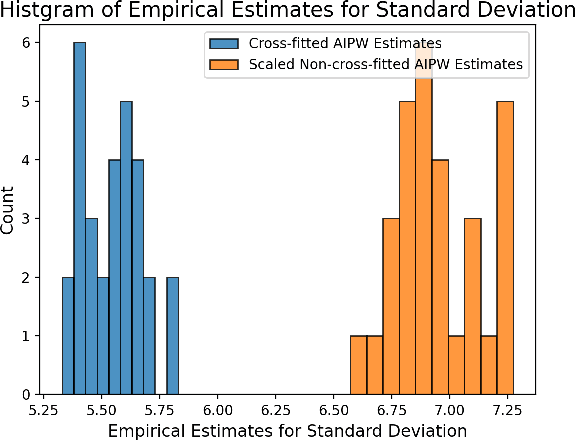
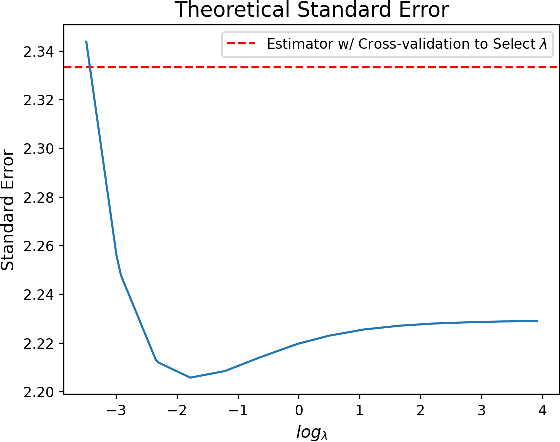
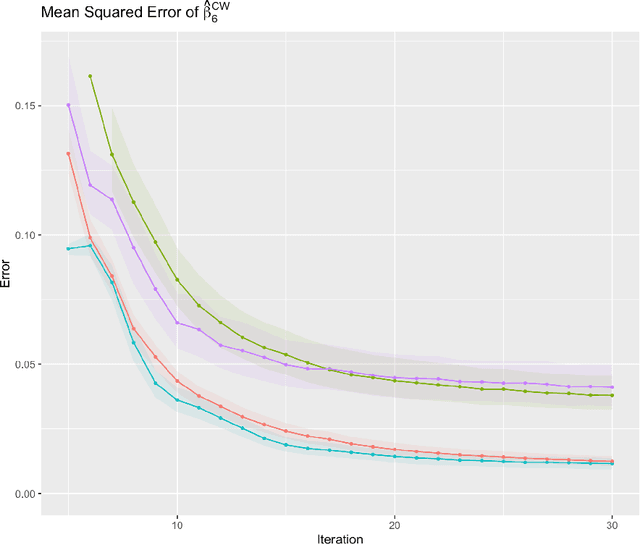
Estimation of the average treatment effect (ATE) is a central problem in causal inference. In recent times, inference for the ATE in the presence of high-dimensional covariates has been extensively studied. Among the diverse approaches that have been proposed, augmented inverse probability weighting (AIPW) with cross-fitting has emerged as a popular choice in practice. In this work, we study this cross-fit AIPW estimator under well-specified outcome regression and propensity score models in a high-dimensional regime where the number of features and samples are both large and comparable. Under assumptions on the covariate distribution, we establish a new CLT for the suitably scaled cross-fit AIPW that applies without any sparsity assumptions on the underlying high-dimensional parameters. Our CLT uncovers two crucial phenomena among others: (i) the AIPW exhibits a substantial variance inflation that can be precisely quantified in terms of the signal-to-noise ratio and other problem parameters, (ii) the asymptotic covariance between the pre-cross-fit estimates is non-negligible even on the root-n scale. In fact, these cross-covariances turn out to be negative in our setting. These findings are strikingly different from their classical counterparts. On the technical front, our work utilizes a novel interplay between three distinct tools--approximate message passing theory, the theory of deterministic equivalents, and the leave-one-out approach. We believe our proof techniques should be useful for analyzing other two-stage estimators in this high-dimensional regime. Finally, we complement our theoretical results with simulations that demonstrate both the finite sample efficacy of our CLT and its robustness to our assumptions.

High-dimensional Asymptotics of Langevin Dynamics in Spiked Matrix Models

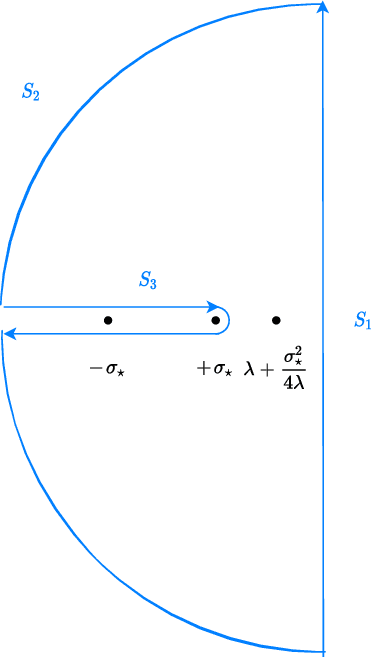
We study Langevin dynamics for recovering the planted signal in the spiked matrix model. We provide a "path-wise" characterization of the overlap between the output of the Langevin algorithm and the planted signal. This overlap is characterized in terms of a self-consistent system of integro-differential equations, usually referred to as the Crisanti-Horner-Sommers-Cugliandolo-Kurchan (CHSCK) equations in the spin glass literature. As a second contribution, we derive an explicit formula for the limiting overlap in terms of the signal-to-noise ratio and the injected noise in the diffusion. This uncovers a sharp phase transition -- in one regime, the limiting overlap is strictly positive, while in the other, the injected noise overcomes the signal, and the limiting overlap is zero.

Representation via Representations: Domain Generalization via Adversarially Learned Invariant Representations

We investigate the power of censoring techniques, first developed for learning {\em fair representations}, to address domain generalization. We examine {\em adversarial} censoring techniques for learning invariant representations from multiple "studies" (or domains), where each study is drawn according to a distribution on domains. The mapping is used at test time to classify instances from a new domain. In many contexts, such as medical forecasting, domain generalization from studies in populous areas (where data are plentiful), to geographically remote populations (for which no training data exist) provides fairness of a different flavor, not anticipated in previous work on algorithmic fairness. We study an adversarial loss function for $k$ domains and precisely characterize its limiting behavior as $k$ grows, formalizing and proving the intuition, backed by experiments, that observing data from a larger number of domains helps. The limiting results are accompanied by non-asymptotic learning-theoretic bounds. Furthermore, we obtain sufficient conditions for good worst-case prediction performance of our algorithm on previously unseen domains. Finally, we decompose our mappings into two components and provide a complete characterization of invariance in terms of this decomposition. To our knowledge, our results provide the first formal guarantees of these kinds for adversarial invariant domain generalization.

Abstracting Fairness: Oracles, Metrics, and Interpretability

It is well understood that classification algorithms, for example, for deciding on loan applications, cannot be evaluated for fairness without taking context into account. We examine what can be learned from a fairness oracle equipped with an underlying understanding of ``true'' fairness. The oracle takes as input a (context, classifier) pair satisfying an arbitrary fairness definition, and accepts or rejects the pair according to whether the classifier satisfies the underlying fairness truth. Our principal conceptual result is an extraction procedure that learns the underlying truth; moreover, the procedure can learn an approximation to this truth given access to a weak form of the oracle. Since every ``truly fair'' classifier induces a coarse metric, in which those receiving the same decision are at distance zero from one another and those receiving different decisions are at distance one, this extraction process provides the basis for ensuring a rough form of metric fairness, also known as individual fairness. Our principal technical result is a higher fidelity extractor under a mild technical constraint on the weak oracle's conception of fairness. Our framework permits the scenario in which many classifiers, with differing outcomes, may all be considered fair. Our results have implications for interpretablity -- a highly desired but poorly defined property of classification systems that endeavors to permit a human arbiter to reject classifiers deemed to be ``unfair'' or illegitimately derived.

A Precise High-Dimensional Asymptotic Theory for Boosting and Min-L1-Norm Interpolated Classifiers

This paper establishes a precise high-dimensional asymptotic theory for Boosting on separable data, taking statistical and computational perspectives. We consider the setting where the number of features (weak learners) p scales with the sample size n, in an over-parametrized regime. On the statistical front, we provide an exact analysis of the generalization error of Boosting, when the algorithm interpolates the training data and maximizes an empirical L1 margin. The angle between the Boosting solution and the ground truth is characterized explicitly. On the computational front, we provide a sharp analysis of the stopping time when Boosting approximately maximizes the empirical L1 margin. Furthermore, we discover that, the larger the margin, the smaller the proportion of active features (with zero initialization). At the heart of our theory lies a detailed study of the maximum L1 margin, using tools from convex geometry. The maximum L1 margin can be precisely described by a new system of non-linear equations, which we study using a novel uniform deviation argument. Preliminary numerical results are presented to demonstrate the accuracy of our theory.