Stock Type Prediction Model Based on Hierarchical Graph Neural Network

This paper introduces a novel approach to stock data analysis by employing a Hierarchical Graph Neural Network (HGNN) model that captures multi-level information and relational structures in the stock market. The HGNN model integrates stock relationship data and hierarchical attributes to predict stock types effectively. The paper discusses the construction of a stock industry relationship graph and the extraction of temporal information from historical price sequences. It also highlights the design of a graph convolution operation and a temporal attention aggregator to model the macro market state. The integration of these features results in a comprehensive stock prediction model that addresses the challenges of utilizing stock relationship data and modeling hierarchical attributes in the stock market.

Advanced RAG Models with Graph Structures: Optimizing Complex Knowledge Reasoning and Text Generation

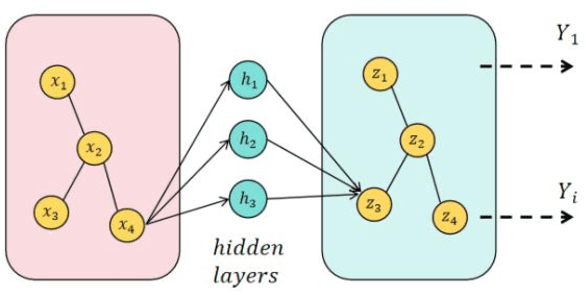
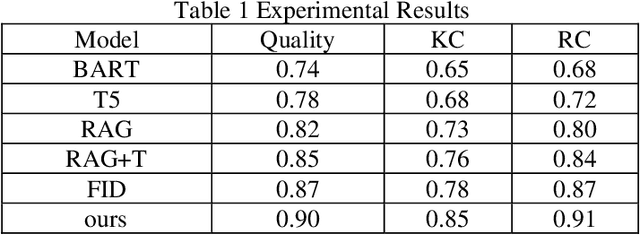
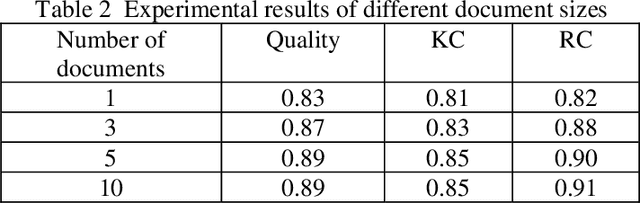
This study aims to optimize the existing retrieval-augmented generation model (RAG) by introducing a graph structure to improve the performance of the model in dealing with complex knowledge reasoning tasks. The traditional RAG model has the problem of insufficient processing efficiency when facing complex graph structure information (such as knowledge graphs, hierarchical relationships, etc.), which affects the quality and consistency of the generated results. This study proposes a scheme to process graph structure data by combining graph neural network (GNN), so that the model can capture the complex relationship between entities, thereby improving the knowledge consistency and reasoning ability of the generated text. The experiment used the Natural Questions (NQ) dataset and compared it with multiple existing generation models. The results show that the graph-based RAG model proposed in this paper is superior to the traditional generation model in terms of quality, knowledge consistency, and reasoning ability, especially when dealing with tasks that require multi-dimensional reasoning. Through the combination of the enhancement of the retrieval module and the graph neural network, the model in this study can better handle complex knowledge background information and has broad potential value in multiple practical application scenarios.

Automated Genre-Aware Article Scoring and Feedback Using Large Language Models

This paper focuses on the development of an advanced intelligent article scoring system that not only assesses the overall quality of written work but also offers detailed feature-based scoring tailored to various article genres. By integrating the pre-trained BERT model with the large language model Chat-GPT, the system gains a deep understanding of both the content and structure of the text, enabling it to provide a thorough evaluation along with targeted suggestions for improvement. Experimental results demonstrate that this system outperforms traditional scoring methods across multiple public datasets, particularly in feature-based assessments, offering a more accurate reflection of the quality of different article types. Moreover, the system generates personalized feedback to assist users in enhancing their writing skills, underscoring the potential and practical value of automated scoring technologies in educational contexts.

Dynamic Fraud Detection: Integrating Reinforcement Learning into Graph Neural Networks

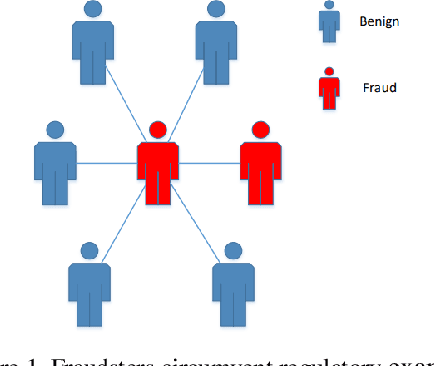
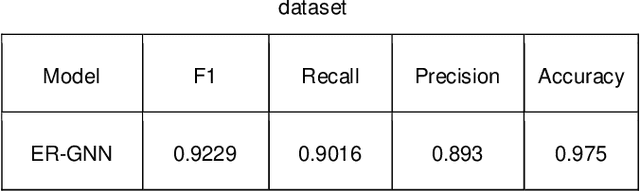
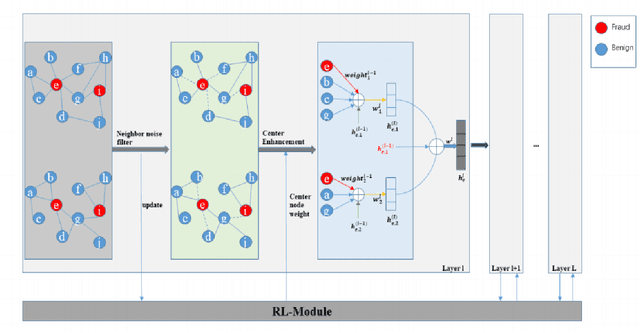
Financial fraud refers to the act of obtaining financial benefits through dishonest means. Such behavior not only disrupts the order of the financial market but also harms economic and social development and breeds other illegal and criminal activities. With the popularization of the internet and online payment methods, many fraudulent activities and money laundering behaviors in life have shifted from offline to online, posing a great challenge to regulatory authorities. How to efficiently detect these financial fraud activities has become an urgent issue that needs to be resolved. Graph neural networks are a type of deep learning model that can utilize the interactive relationships within graph structures, and they have been widely applied in the field of fraud detection. However, there are still some issues. First, fraudulent activities only account for a very small part of transaction transfers, leading to an inevitable problem of label imbalance in fraud detection. At the same time, fraudsters often disguise their behavior, which can have a negative impact on the final prediction results. In addition, existing research has overlooked the importance of balancing neighbor information and central node information. For example, when the central node has too many neighbors, the features of the central node itself are often neglected. Finally, fraud activities and patterns are constantly changing over time, so considering the dynamic evolution of graph edge relationships is also very important.

How Does Distribution Matching Help Domain Generalization: An Information-theoretic Analysis

Domain generalization aims to learn invariance across multiple training domains, thereby enhancing generalization against out-of-distribution data. While gradient or representation matching algorithms have achieved remarkable success, these methods generally lack generalization guarantees or depend on strong assumptions, leaving a gap in understanding the underlying mechanism of distribution matching. In this work, we formulate domain generalization from a novel probabilistic perspective, ensuring robustness while avoiding overly conservative solutions. Through comprehensive information-theoretic analysis, we provide key insights into the roles of gradient and representation matching in promoting generalization. Our results reveal the complementary relationship between these two components, indicating that existing works focusing solely on either gradient or representation alignment are insufficient to solve the domain generalization problem. In light of these theoretical findings, we introduce IDM to simultaneously align the inter-domain gradients and representations. Integrated with the proposed PDM method for complex distribution matching, IDM achieves superior performance over various baseline methods.

Understanding the Generalization Ability of Deep Learning Algorithms: A Kernelized Renyi's Entropy Perspective

Recently, information theoretic analysis has become a popular framework for understanding the generalization behavior of deep neural networks. It allows a direct analysis for stochastic gradient/Langevin descent (SGD/SGLD) learning algorithms without strong assumptions such as Lipschitz or convexity conditions. However, the current generalization error bounds within this framework are still far from optimal, while substantial improvements on these bounds are quite challenging due to the intractability of high-dimensional information quantities. To address this issue, we first propose a novel information theoretical measure: kernelized Renyi's entropy, by utilizing operator representation in Hilbert space. It inherits the properties of Shannon's entropy and can be effectively calculated via simple random sampling, while remaining independent of the input dimension. We then establish the generalization error bounds for SGD/SGLD under kernelized Renyi's entropy, where the mutual information quantities can be directly calculated, enabling evaluation of the tightness of each intermediate step. We show that our information-theoretical bounds depend on the statistics of the stochastic gradients evaluated along with the iterates, and are rigorously tighter than the current state-of-the-art (SOTA) results. The theoretical findings are also supported by large-scale empirical studies1.

Robust and Fast Measure of Information via Low-rank Representation

The matrix-based R\'enyi's entropy allows us to directly quantify information measures from given data, without explicit estimation of the underlying probability distribution. This intriguing property makes it widely applied in statistical inference and machine learning tasks. However, this information theoretical quantity is not robust against noise in the data, and is computationally prohibitive in large-scale applications. To address these issues, we propose a novel measure of information, termed low-rank matrix-based R\'enyi's entropy, based on low-rank representations of infinitely divisible kernel matrices. The proposed entropy functional inherits the specialty of of the original definition to directly quantify information from data, but enjoys additional advantages including robustness and effective calculation. Specifically, our low-rank variant is more sensitive to informative perturbations induced by changes in underlying distributions, while being insensitive to uninformative ones caused by noises. Moreover, low-rank R\'enyi's entropy can be efficiently approximated by random projection and Lanczos iteration techniques, reducing the overall complexity from $\mathcal{O}(n^3)$ to $\mathcal{O}(n^2 s)$ or even $\mathcal{O}(ns^2)$, where $n$ is the number of data samples and $s \ll n$. We conduct large-scale experiments to evaluate the effectiveness of this new information measure, demonstrating superior results compared to matrix-based R\'enyi's entropy in terms of both performance and computational efficiency.

Optimal Randomized Approximations for Matrix based Renyi's Entropy

The Matrix-based Renyi's entropy enables us to directly measure information quantities from given data without the costly probability density estimation of underlying distributions, thus has been widely adopted in numerous statistical learning and inference tasks. However, exactly calculating this new information quantity requires access to the eigenspectrum of a semi-positive definite (SPD) matrix $A$ which grows linearly with the number of samples $n$, resulting in a $O(n^3)$ time complexity that is prohibitive for large-scale applications. To address this issue, this paper takes advantage of stochastic trace approximations for matrix-based Renyi's entropy with arbitrary $\alpha \in R^+$ orders, lowering the complexity by converting the entropy approximation to a matrix-vector multiplication problem. Specifically, we develop random approximations for integer order $\alpha$ cases and polynomial series approximations (Taylor and Chebyshev) for non-integer $\alpha$ cases, leading to a $O(n^2sm)$ overall time complexity, where $s,m \ll n$ denote the number of vector queries and the polynomial order respectively. We theoretically establish statistical guarantees for all approximation algorithms and give explicit order of s and m with respect to the approximation error $\varepsilon$, showing optimal convergence rate for both parameters up to a logarithmic factor. Large-scale simulations and real-world applications validate the effectiveness of the developed approximations, demonstrating remarkable speedup with negligible loss in accuracy.

Computationally Efficient Approximations for Matrix-based Renyi's Entropy

The recently developed matrix based Renyi's entropy enables measurement of information in data simply using the eigenspectrum of symmetric positive semi definite (PSD) matrices in reproducing kernel Hilbert space, without estimation of the underlying data distribution. This intriguing property makes the new information measurement widely adopted in multiple statistical inference and learning tasks. However, the computation of such quantity involves the trace operator on a PSD matrix $G$ to power $\alpha$(i.e., $tr(G^\alpha)$), with a normal complexity of nearly $O(n^3)$, which severely hampers its practical usage when the number of samples (i.e., $n$) is large. In this work, we present computationally efficient approximations to this new entropy functional that can reduce its complexity to even significantly less than $O(n^2)$. To this end, we first develop randomized approximations to $\tr(\G^\alpha)$ that transform the trace estimation into matrix-vector multiplications problem. We extend such strategy for arbitrary values of $\alpha$ (integer or non-integer). We then establish the connection between the matrix-based Renyi's entropy and PSD matrix approximation, which enables us to exploit both clustering and block low-rank structure of $\G$ to further reduce the computational cost. We theoretically provide approximation accuracy guarantees and illustrate the properties of different approximations. Large-scale experimental evaluations on both synthetic and real-world data corroborate our theoretical findings, showing promising speedup with negligible loss in accuracy.

Markov subsampling based Huber Criterion

Subsampling is an important technique to tackle the computational challenges brought by big data. Many subsampling procedures fall within the framework of importance sampling, which assigns high sampling probabilities to the samples appearing to have big impacts. When the noise level is high, those sampling procedures tend to pick many outliers and thus often do not perform satisfactorily in practice. To tackle this issue, we design a new Markov subsampling strategy based on Huber criterion (HMS) to construct an informative subset from the noisy full data; the constructed subset then serves as a refined working data for efficient processing. HMS is built upon a Metropolis-Hasting procedure, where the inclusion probability of each sampling unit is determined using the Huber criterion to prevent over scoring the outliers. Under mild conditions, we show that the estimator based on the subsamples selected by HMS is statistically consistent with a sub-Gaussian deviation bound. The promising performance of HMS is demonstrated by extensive studies on large scale simulations and real data examples.