Higher-Order Topological Directionality and Directed Simplicial Neural Networks

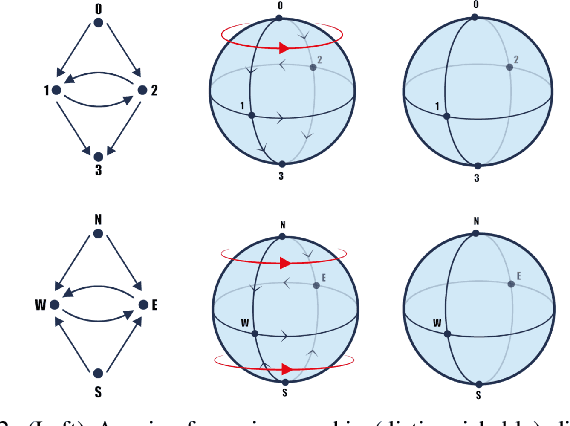
Topological Deep Learning (TDL) has emerged as a paradigm to process and learn from signals defined on higher-order combinatorial topological spaces, such as simplicial or cell complexes. Although many complex systems have an asymmetric relational structure, most TDL models forcibly symmetrize these relationships. In this paper, we first introduce a novel notion of higher-order directionality and we then design Directed Simplicial Neural Networks (Dir-SNNs) based on it. Dir-SNNs are message-passing networks operating on directed simplicial complexes able to leverage directed and possibly asymmetric interactions among the simplices. To our knowledge, this is the first TDL model using a notion of higher-order directionality. We theoretically and empirically prove that Dir-SNNs are more expressive than their directed graph counterpart in distinguishing isomorphic directed graphs. Experiments on a synthetic source localization task demonstrate that Dir-SNNs outperform undirected SNNs when the underlying complex is directed, and perform comparably when the underlying complex is undirected.

ICML Topological Deep Learning Challenge 2024: Beyond the Graph Domain

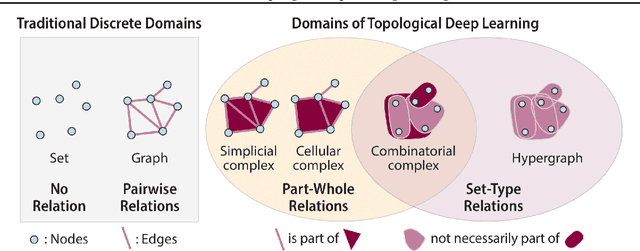
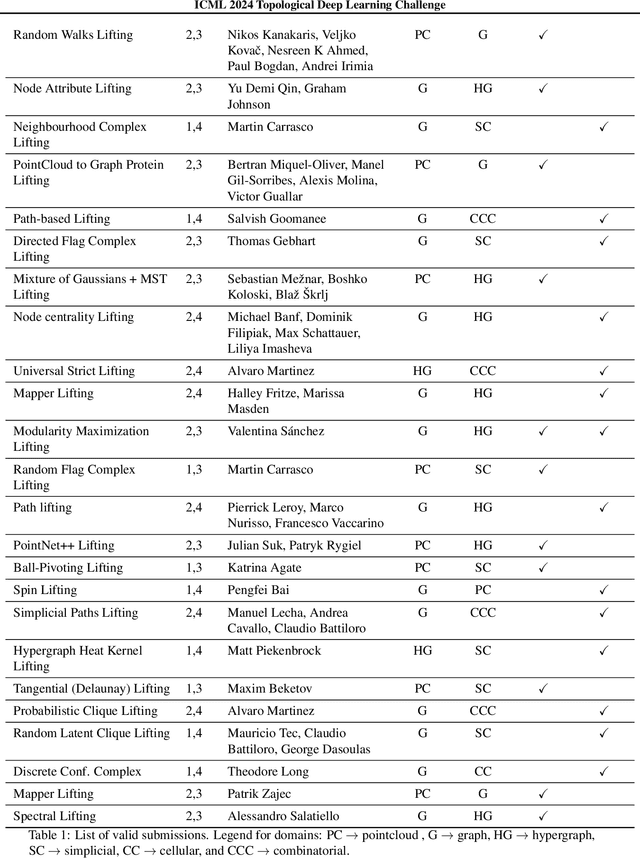
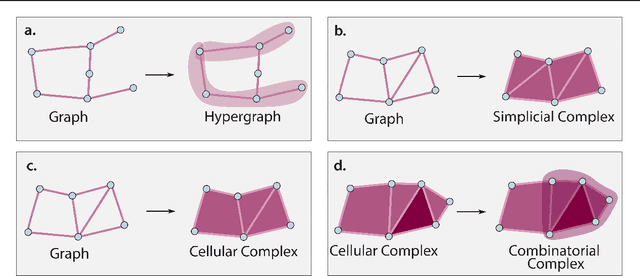
This paper describes the 2nd edition of the ICML Topological Deep Learning Challenge that was hosted within the ICML 2024 ELLIS Workshop on Geometry-grounded Representation Learning and Generative Modeling (GRaM). The challenge focused on the problem of representing data in different discrete topological domains in order to bridge the gap between Topological Deep Learning (TDL) and other types of structured datasets (e.g. point clouds, graphs). Specifically, participants were asked to design and implement topological liftings, i.e. mappings between different data structures and topological domains --like hypergraphs, or simplicial/cell/combinatorial complexes. The challenge received 52 submissions satisfying all the requirements. This paper introduces the main scope of the challenge, and summarizes the main results and findings.

TopoX: A Suite of Python Packages for Machine Learning on Topological Domains

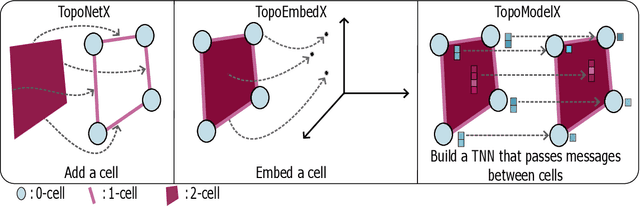
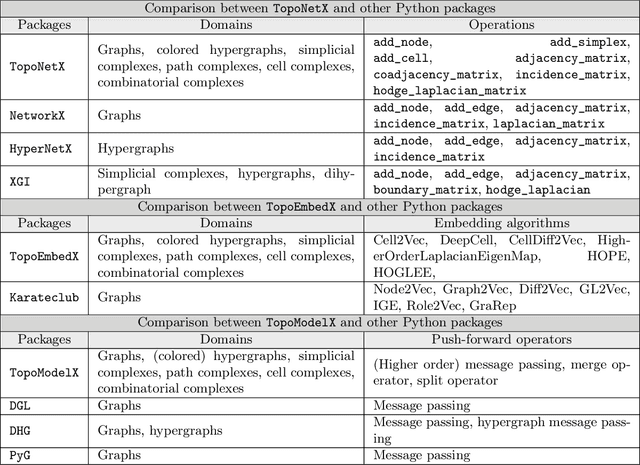
We introduce topox, a Python software suite that provides reliable and user-friendly building blocks for computing and machine learning on topological domains that extend graphs: hypergraphs, simplicial, cellular, path and combinatorial complexes. topox consists of three packages: toponetx facilitates constructing and computing on these domains, including working with nodes, edges and higher-order cells; topoembedx provides methods to embed topological domains into vector spaces, akin to popular graph-based embedding algorithms such as node2vec; topomodelx is built on top of PyTorch and offers a comprehensive toolbox of higher-order message passing functions for neural networks on topological domains. The extensively documented and unit-tested source code of topox is available under MIT license at https://github.com/pyt-team.

ICML 2023 Topological Deep Learning Challenge : Design and Results

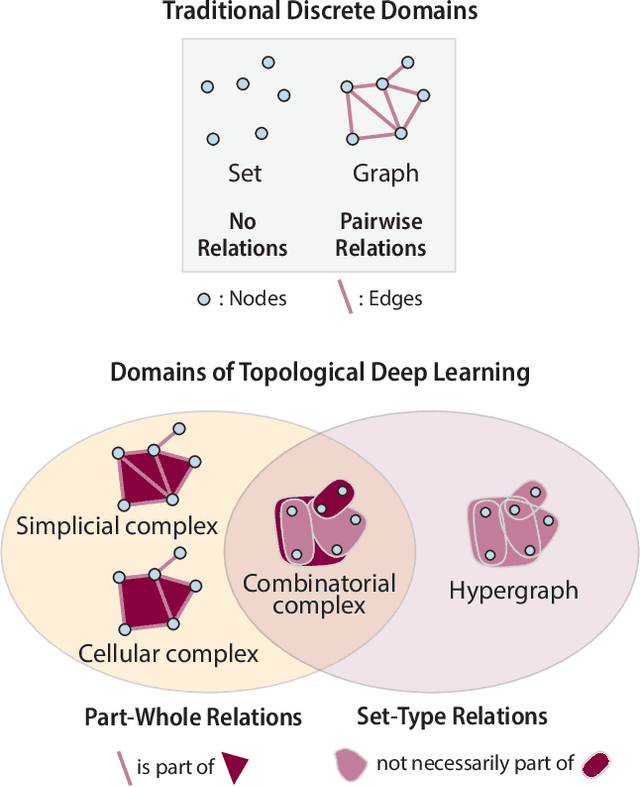
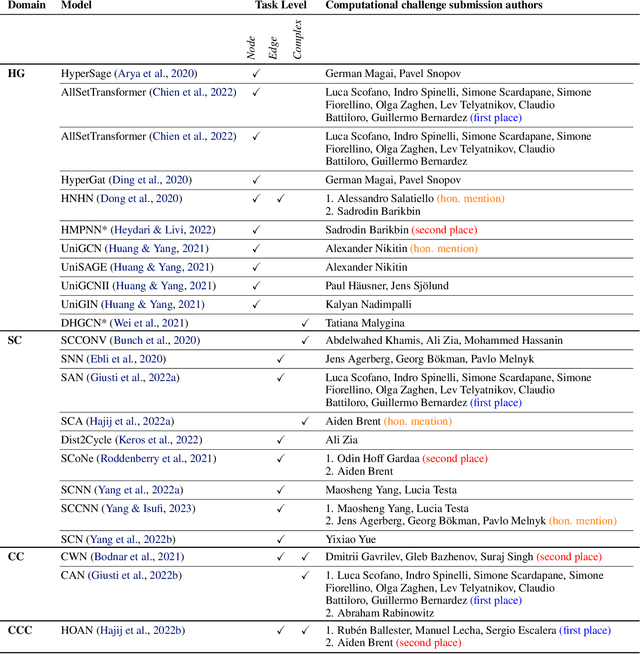
This paper presents the computational challenge on topological deep learning that was hosted within the ICML 2023 Workshop on Topology and Geometry in Machine Learning. The competition asked participants to provide open-source implementations of topological neural networks from the literature by contributing to the python packages TopoNetX (data processing) and TopoModelX (deep learning). The challenge attracted twenty-eight qualifying submissions in its two-month duration. This paper describes the design of the challenge and summarizes its main findings.