Gemini 1.5: Unlocking multimodal understanding across millions of tokens of context

In this report, we present the latest model of the Gemini family, Gemini 1.5 Pro, a highly compute-efficient multimodal mixture-of-experts model capable of recalling and reasoning over fine-grained information from millions of tokens of context, including multiple long documents and hours of video and audio. Gemini 1.5 Pro achieves near-perfect recall on long-context retrieval tasks across modalities, improves the state-of-the-art in long-document QA, long-video QA and long-context ASR, and matches or surpasses Gemini 1.0 Ultra's state-of-the-art performance across a broad set of benchmarks. Studying the limits of Gemini 1.5 Pro's long-context ability, we find continued improvement in next-token prediction and near-perfect retrieval (>99%) up to at least 10M tokens, a generational leap over existing models such as Claude 2.1 (200k) and GPT-4 Turbo (128k). Finally, we highlight surprising new capabilities of large language models at the frontier; when given a grammar manual for Kalamang, a language with fewer than 200 speakers worldwide, the model learns to translate English to Kalamang at a similar level to a person who learned from the same content.

Gemini: A Family of Highly Capable Multimodal Models

This report introduces a new family of multimodal models, Gemini, that exhibit remarkable capabilities across image, audio, video, and text understanding. The Gemini family consists of Ultra, Pro, and Nano sizes, suitable for applications ranging from complex reasoning tasks to on-device memory-constrained use-cases. Evaluation on a broad range of benchmarks shows that our most-capable Gemini Ultra model advances the state of the art in 30 of 32 of these benchmarks - notably being the first model to achieve human-expert performance on the well-studied exam benchmark MMLU, and improving the state of the art in every one of the 20 multimodal benchmarks we examined. We believe that the new capabilities of Gemini models in cross-modal reasoning and language understanding will enable a wide variety of use cases and we discuss our approach toward deploying them responsibly to users.

Automatically Composing Representation Transformations as a Means for Generalization

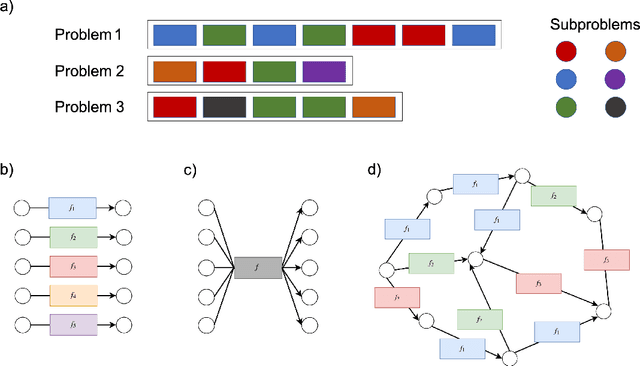
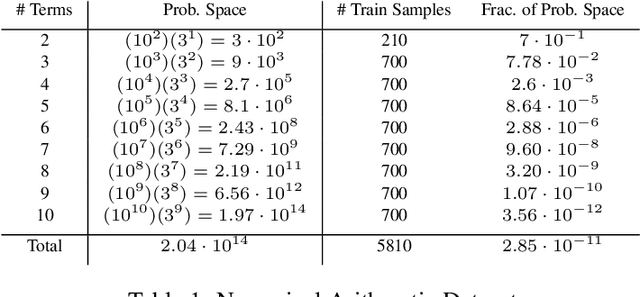
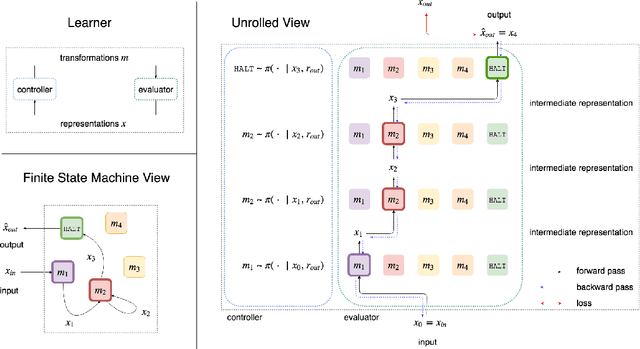
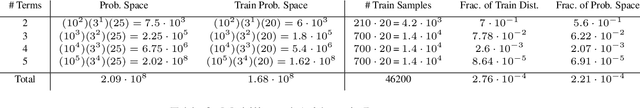
How can we build a learner that can capture the essence of what makes a hard problem more complex than a simple one, break the hard problem along characteristic lines into smaller problems it knows how to solve, and sequentially solve the smaller problems until the larger one is solved? To work towards this goal, we focus on learning to generalize in a particular family of problems that exhibit compositional and recursive structure: their solutions can be found by composing in sequence a set of reusable partial solutions. Our key idea is to recast the problem of generalization as a problem of learning algorithmic procedures: we can formulate a solution to this family as a sequential decision-making process over transformations between representations. Our formulation enables the learner to learn the structure and parameters of its own computation graph with sparse supervision, make analogies between problems by transforming one problem representation to another, and exploit modularity and reuse to scale to problems of varying complexity. Experiments on solving a variety of multilingual arithmetic problems demonstrate that our method discovers the hierarchical decomposition of a problem into its subproblems, generalizes out of distribution to unseen problem classes, and extrapolates to harder versions of the same problem, yielding a 10-fold reduction in sample complexity compared to a monolithic recurrent neural network.

A Compositional Object-Based Approach to Learning Physical Dynamics

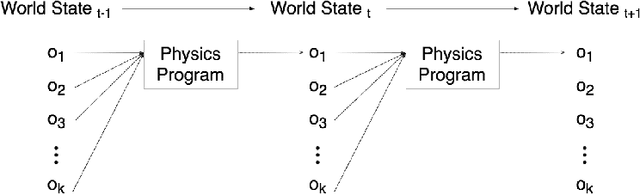
We present the Neural Physics Engine (NPE), a framework for learning simulators of intuitive physics that naturally generalize across variable object count and different scene configurations. We propose a factorization of a physical scene into composable object-based representations and a neural network architecture whose compositional structure factorizes object dynamics into pairwise interactions. Like a symbolic physics engine, the NPE is endowed with generic notions of objects and their interactions; realized as a neural network, it can be trained via stochastic gradient descent to adapt to specific object properties and dynamics of different worlds. We evaluate the efficacy of our approach on simple rigid body dynamics in two-dimensional worlds. By comparing to less structured architectures, we show that the NPE's compositional representation of the structure in physical interactions improves its ability to predict movement, generalize across variable object count and different scene configurations, and infer latent properties of objects such as mass.