Trading-Off Payments and Accuracy in Online Classification with Paid Stochastic Experts

We investigate online classification with paid stochastic experts. Here, before making their prediction, each expert must be paid. The amount that we pay each expert directly influences the accuracy of their prediction through some unknown Lipschitz "productivity" function. In each round, the learner must decide how much to pay each expert and then make a prediction. They incur a cost equal to a weighted sum of the prediction error and upfront payments for all experts. We introduce an online learning algorithm whose total cost after $T$ rounds exceeds that of a predictor which knows the productivity of all experts in advance by at most $\mathcal{O}(K^2(\log T)\sqrt{T})$ where $K$ is the number of experts. In order to achieve this result, we combine Lipschitz bandits and online classification with surrogate losses. These tools allow us to improve upon the bound of order $T^{2/3}$ one would obtain in the standard Lipschitz bandit setting. Our algorithm is empirically evaluated on synthetic data

Delayed Bandits: When Do Intermediate Observations Help?

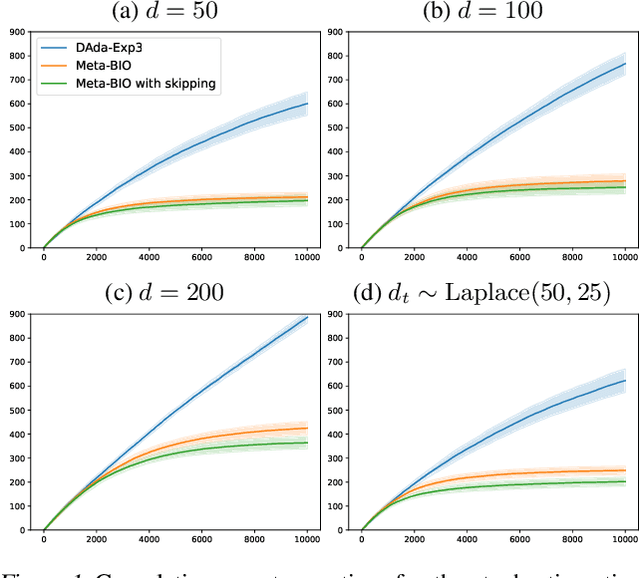
We study a $K$-armed bandit with delayed feedback and intermediate observations. We consider a model where intermediate observations have a form of a finite state, which is observed immediately after taking an action, whereas the loss is observed after an adversarially chosen delay. We show that the regime of the mapping of states to losses determines the complexity of the problem, irrespective of whether the mapping of actions to states is stochastic or adversarial. If the mapping of states to losses is adversarial, then the regret rate is of order $\sqrt{(K+d)T}$ (within log factors), where $T$ is the time horizon and $d$ is a fixed delay. This matches the regret rate of a $K$-armed bandit with delayed feedback and without intermediate observations, implying that intermediate observations are not helpful. However, if the mapping of states to losses is stochastic, we show that the regret grows at a rate of $\sqrt{\big(K+\min\{|\mathcal{S}|,d\}\big)T}$ (within log factors), implying that if the number $|\mathcal{S}|$ of states is smaller than the delay, then intermediate observations help. We also provide refined high-probability regret upper bounds for non-uniform delays, together with experimental validation of our algorithms.

Black-box adversarial attacks using Evolution Strategies

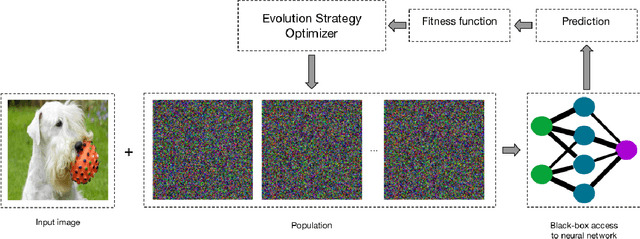
In the last decade, deep neural networks have proven to be very powerful in computer vision tasks, starting a revolution in the computer vision and machine learning fields. However, deep neural networks, usually, are not robust to perturbations of the input data. In fact, several studies showed that slightly changing the content of the images can cause a dramatic decrease in the accuracy of the attacked neural network. Several methods able to generate adversarial samples make use of gradients, which usually are not available to an attacker in real-world scenarios. As opposed to this class of attacks, another class of adversarial attacks, called black-box adversarial attacks, emerged, which does not make use of information on the gradients, being more suitable for real-world attack scenarios. In this work, we compare three well-known evolution strategies on the generation of black-box adversarial attacks for image classification tasks. While our results show that the attacked neural networks can be, in most cases, easily fooled by all the algorithms under comparison, they also show that some black-box optimization algorithms may be better in "harder" setups, both in terms of attack success rate and efficiency (i.e., number of queries).

The Channel Attention based Context Encoder Network for Inner Limiting Membrane Detection

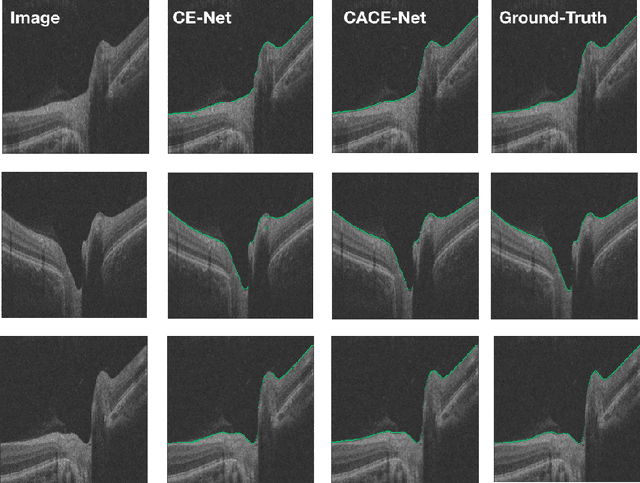
The optic disc segmentation is an important step for retinal image-based disease diagnosis such as glaucoma. The inner limiting membrane (ILM) is the first boundary in the OCT, which can help to extract the retinal pigment epithelium (RPE) through gradient edge information to locate the boundary of the optic disc. Thus, the ILM layer segmentation is of great importance for optic disc localization. In this paper, we build a new optic disc centered dataset from 20 volunteers and manually annotated the ILM boundary in each OCT scan as ground-truth. We also propose a channel attention based context encoder network modified from the CE-Net to segment the optic disc. It mainly contains three phases: the encoder module, the channel attention based context encoder module, and the decoder module. Finally, we demonstrate that our proposed method achieves state-of-the-art disc segmentation performance on our dataset mentioned above.