Bongard in Wonderland: Visual Puzzles that Still Make AI Go Mad?

Recently, newly developed Vision-Language Models (VLMs), such as OpenAI's GPT-4o, have emerged, seemingly demonstrating advanced reasoning capabilities across text and image modalities. Yet, the depth of these advances in language-guided perception and abstract reasoning remains underexplored, and it is unclear whether these models can truly live up to their ambitious promises. To assess the progress and identify shortcomings, we enter the wonderland of Bongard problems, a set of classical visual reasoning puzzles that require human-like abilities of pattern recognition and abstract reasoning. While VLMs occasionally succeed in identifying discriminative concepts and solving some of the problems, they frequently falter, failing to understand and reason about visual concepts. Surprisingly, even elementary concepts that may seem trivial to humans, such as simple spirals, pose significant challenges. Moreover, even when asked to explicitly focus on and analyze these concepts, they continue to falter, suggesting not only a lack of understanding of these elementary visual concepts but also an inability to generalize to unseen concepts. These observations underscore the current limitations of VLMs, emphasize that a significant gap remains between human-like visual reasoning and machine cognition, and highlight the ongoing need for innovation in this area.

Inverse decision-making using neural amortized Bayesian actors

Bayesian observer and actor models have provided normative explanations for many behavioral phenomena in perception, sensorimotor control, and other areas of cognitive science and neuroscience. They attribute behavioral variability and biases to different interpretable entities such as perceptual and motor uncertainty, prior beliefs, and behavioral costs. However, when extending these models to more complex tasks with continuous actions, solving the Bayesian decision-making problem is often analytically intractable. Moreover, inverting such models to perform inference over their parameters given behavioral data is computationally even more difficult. Therefore, researchers typically constrain their models to easily tractable components, such as Gaussian distributions or quadratic cost functions, or resort to numerical methods. To overcome these limitations, we amortize the Bayesian actor using a neural network trained on a wide range of different parameter settings in an unsupervised fashion. Using the pre-trained neural network enables performing gradient-based Bayesian inference of the Bayesian actor model's parameters. We show on synthetic data that the inferred posterior distributions are in close alignment with those obtained using analytical solutions where they exist. Where no analytical solution is available, we recover posterior distributions close to the ground truth. We then show that identifiability problems between priors and costs can arise in more complex cost functions. Finally, we apply our method to empirical data and show that it explains systematic individual differences of behavioral patterns.

What Matters for Active Texture Recognition With Vision-Based Tactile Sensors

This paper explores active sensing strategies that employ vision-based tactile sensors for robotic perception and classification of fabric textures. We formalize the active sampling problem in the context of tactile fabric recognition and provide an implementation of information-theoretic exploration strategies based on minimizing predictive entropy and variance of probabilistic models. Through ablation studies and human experiments, we investigate which components are crucial for quick and reliable texture recognition. Along with the active sampling strategies, we evaluate neural network architectures, representations of uncertainty, influence of data augmentation, and dataset variability. By evaluating our method on a previously published Active Clothing Perception Dataset and on a real robotic system, we establish that the choice of the active exploration strategy has only a minor influence on the recognition accuracy, whereas data augmentation and dropout rate play a significantly larger role. In a comparison study, while humans achieve 66.9% recognition accuracy, our best approach reaches 90.0% in under 5 touches, highlighting that vision-based tactile sensors are highly effective for fabric texture recognition.

Probabilistic inverse optimal control with local linearization for non-linear partially observable systems

Inverse optimal control methods can be used to characterize behavior in sequential decision-making tasks. Most existing work, however, requires the control signals to be known, or is limited to fully-observable or linear systems. This paper introduces a probabilistic approach to inverse optimal control for stochastic non-linear systems with missing control signals and partial observability that unifies existing approaches. By using an explicit model of the noise characteristics of the sensory and control systems of the agent in conjunction with local linearization techniques, we derive an approximate likelihood for the model parameters, which can be computed within a single forward pass. We evaluate our proposed method on stochastic and partially observable version of classic control tasks, a navigation task, and a manual reaching task. The proposed method has broad applicability, ranging from imitation learning to sensorimotor neuroscience.

Speaking Multiple Languages Affects the Moral Bias of Language Models

Pre-trained multilingual language models (PMLMs) are commonly used when dealing with data from multiple languages and cross-lingual transfer. However, PMLMs are trained on varying amounts of data for each language. In practice this means their performance is often much better on English than many other languages. We explore to what extent this also applies to moral norms. Do the models capture moral norms from English and impose them on other languages? Do the models exhibit random and thus potentially harmful beliefs in certain languages? Both these issues could negatively impact cross-lingual transfer and potentially lead to harmful outcomes. In this paper, we (1) apply the MoralDirection framework to multilingual models, comparing results in German, Czech, Arabic, Mandarin Chinese, and English, (2) analyse model behaviour on filtered parallel subtitles corpora, and (3) apply the models to a Moral Foundations Questionnaire, comparing with human responses from different countries. Our experiments demonstrate that, indeed, PMLMs encode differing moral biases, but these do not necessarily correspond to cultural differences or commonalities in human opinions.

Reinforcement Learning with Non-Exponential Discounting

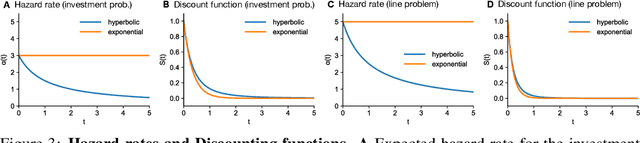
Commonly in reinforcement learning (RL), rewards are discounted over time using an exponential function to model time preference, thereby bounding the expected long-term reward. In contrast, in economics and psychology, it has been shown that humans often adopt a hyperbolic discounting scheme, which is optimal when a specific task termination time distribution is assumed. In this work, we propose a theory for continuous-time model-based reinforcement learning generalized to arbitrary discount functions. This formulation covers the case in which there is a non-exponential random termination time. We derive a Hamilton-Jacobi-Bellman (HJB) equation characterizing the optimal policy and describe how it can be solved using a collocation method, which uses deep learning for function approximation. Further, we show how the inverse RL problem can be approached, in which one tries to recover properties of the discount function given decision data. We validate the applicability of our proposed approach on two simulated problems. Our approach opens the way for the analysis of human discounting in sequential decision-making tasks.

Improving saliency models' predictions of the next fixation with humans' intrinsic cost of gaze shifts

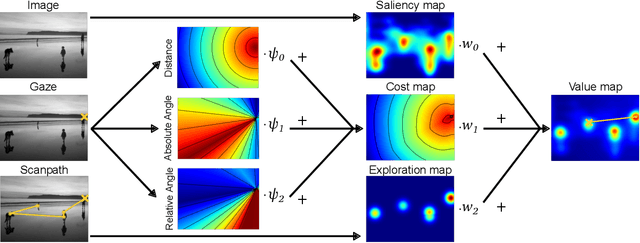
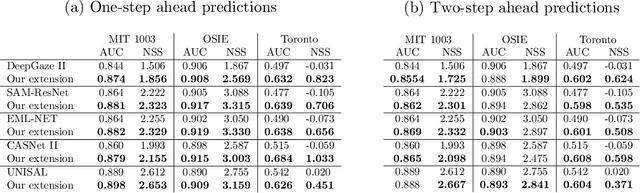
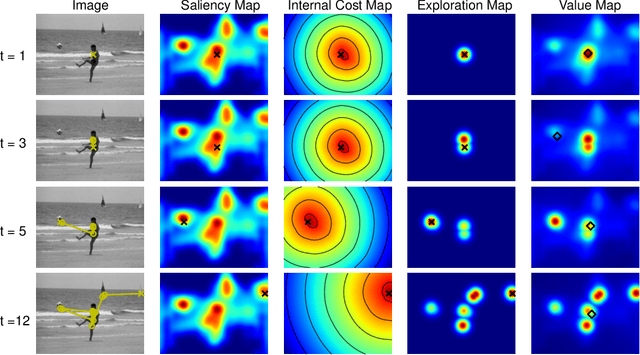
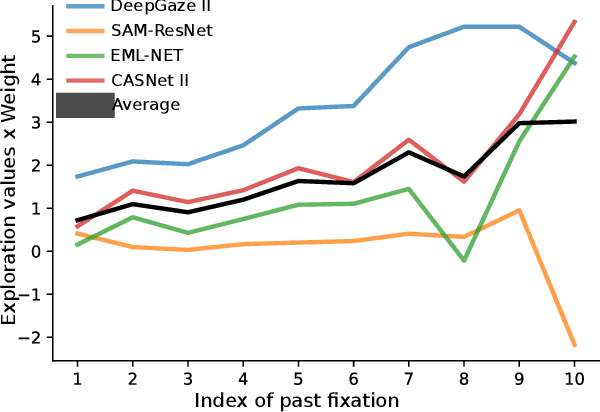
The human prioritization of image regions can be modeled in a time invariant fashion with saliency maps or sequentially with scanpath models. However, while both types of models have steadily improved on several benchmarks and datasets, there is still a considerable gap in predicting human gaze. Here, we leverage two recent developments to reduce this gap: theoretical analyses establishing a principled framework for predicting the next gaze target and the empirical measurement of the human cost for gaze switches independently of image content. We introduce an algorithm in the framework of sequential decision making, which converts any static saliency map into a sequence of dynamic history-dependent value maps, which are recomputed after each gaze shift. These maps are based on 1) a saliency map provided by an arbitrary saliency model, 2) the recently measured human cost function quantifying preferences in magnitude and direction of eye movements, and 3) a sequential exploration bonus, which changes with each subsequent gaze shift. The parameters of the spatial extent and temporal decay of this exploration bonus are estimated from human gaze data. The relative contributions of these three components were optimized on the MIT1003 dataset for the NSS score and are sufficient to significantly outperform predictions of the next gaze target on NSS and AUC scores for five state of the art saliency models on three image data sets. Thus, we provide an implementation of human gaze preferences, which can be used to improve arbitrary saliency models' predictions of humans' next gaze targets.

Inferring perceptual decision making parameters from behavior in production and reproduction tasks

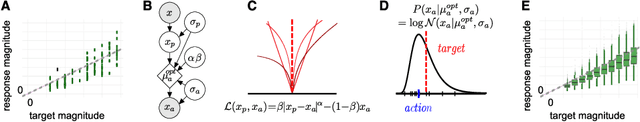
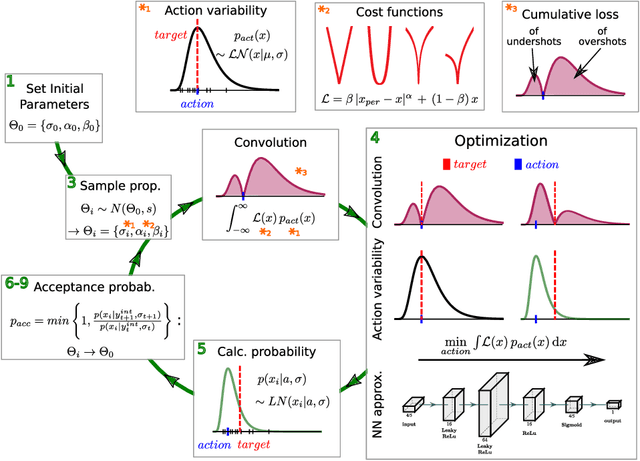
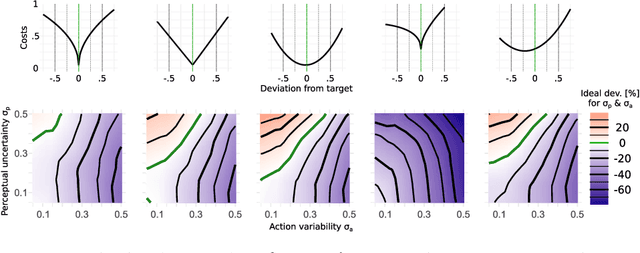
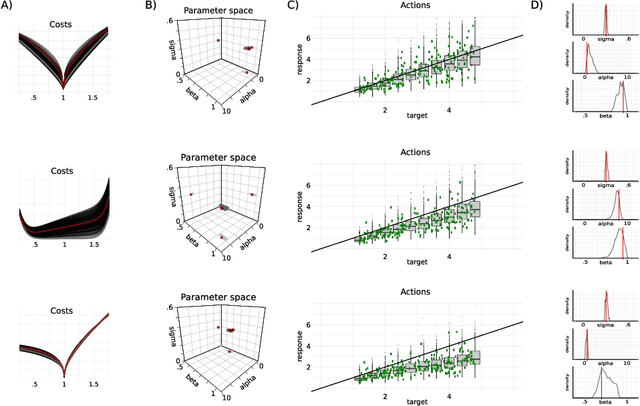
Bayesian models of behavior have provided computational level explanations in a range of psychophysical tasks. One fundamental experimental paradigm is the production or reproduction task, in which subjects are instructed to generate an action that either reproduces a previously sensed stimulus magnitude or achieves a target response. This type of task therefore distinguishes itself from other psychophysical tasks in that the responses are on a continuum and effort plays an important role with increasing response magnitude. Based on Bayesian decision theory we present an inference method to recover perceptual uncertainty, response variability, and the cost function underlying human responses. Crucially, the cost function is parameterized such that effort is explicitly included. We present a hybrid inference method employing MCMC sampling utilizing appropriate proposal distributions and an inner loop utilizing amortized inference with a neural network that approximates the mode of the optimal response distribution. We show how this model can be utilized to avoid unidentifiability of experimental designs and that parameters can be recovered through validation on synthetic and application to experimental data. Our approach will enable behavioral scientists to perform Bayesian inference of decision making parameters in production and reproduction tasks.

Inverse Optimal Control Adapted to the Noise Characteristics of the Human Sensorimotor System

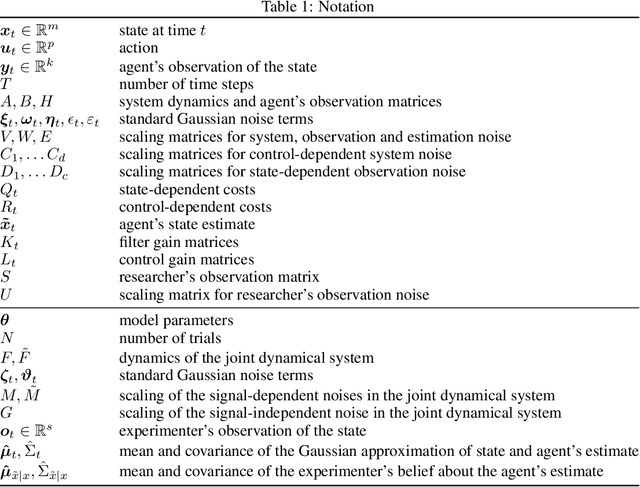
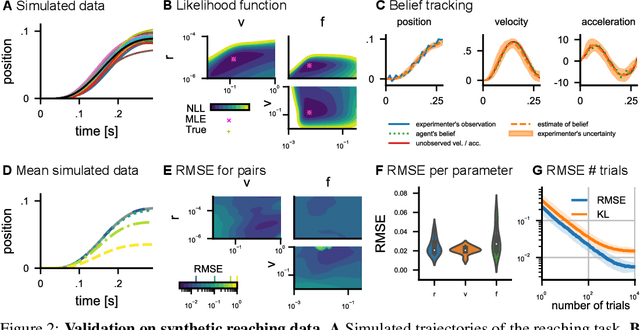
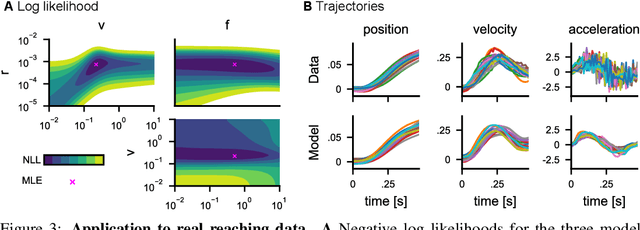
Computational level explanations based on optimal feedback control with signal-dependent noise have been able to account for a vast array of phenomena in human sensorimotor behavior. However, commonly a cost function needs to be assumed for a task and the optimality of human behavior is evaluated by comparing observed and predicted trajectories. Here, we introduce inverse optimal control with signal-dependent noise, which allows inferring the cost function from observed behavior. To do so, we formalize the problem as a partially observable Markov decision process and distinguish between the agent's and the experimenter's inference problems. Specifically, we derive a probabilistic formulation of the evolution of states and belief states and an approximation to the propagation equation in the linear-quadratic Gaussian problem with signal-dependent noise. We extend the model to the case of partial observability of state variables from the point of view of the experimenter. We show the feasibility of the approach through validation on synthetic data and application to experimental data. Our approach enables recovering the costs and benefits implicit in human sequential sensorimotor behavior, thereby reconciling normative and descriptive approaches in a computational framework.

Structural Causal Interpretation Theorem

Human mental processes allow for qualitative reasoning about causality in terms of mechanistic relations of the variables of interest, which we argue are naturally described by structural causal model (SCM). Since interpretations are being derived from mental models, the same applies for SCM. By defining a metric space on SCM, we provide a theoretical perspective on the comparison of mental models and thereby conclude that interpretations can be used for guiding a learning system towards true causality. To this effect, we present a theoretical analysis from first principles that results in a human-readable interpretation scheme consistent with the provided causality that we name structural causal interpretations (SCI). Going further, we prove that any existing neural induction method (NIM) is in fact interpretable. Our first experiment (E1) assesses the quality of such NIM-based SCI. In (E2) we observe evidence for our conjecture on improved sample-efficiency for SCI-based learning. After conducting a small user study, in (E3) we observe superiority in human-based over NIM-based SCI in support of our initial hypothesis.