Divide and Conquer: Provably Unveiling the Pareto Front with Multi-Objective Reinforcement Learning

A significant challenge in multi-objective reinforcement learning is obtaining a Pareto front of policies that attain optimal performance under different preferences. We introduce Iterated Pareto Referent Optimisation (IPRO), a principled algorithm that decomposes the task of finding the Pareto front into a sequence of single-objective problems for which various solution methods exist. This enables us to establish convergence guarantees while providing an upper bound on the distance to undiscovered Pareto optimal solutions at each step. Empirical evaluations demonstrate that IPRO matches or outperforms methods that require additional domain knowledge. By leveraging problem-specific single-objective solvers, our approach also holds promise for applications beyond multi-objective reinforcement learning, such as in pathfinding and optimisation.

Monte Carlo Tree Search Algorithms for Risk-Aware and Multi-Objective Reinforcement Learning

In many risk-aware and multi-objective reinforcement learning settings, the utility of the user is derived from a single execution of a policy. In these settings, making decisions based on the average future returns is not suitable. For example, in a medical setting a patient may only have one opportunity to treat their illness. Making decisions using just the expected future returns -- known in reinforcement learning as the value -- cannot account for the potential range of adverse or positive outcomes a decision may have. Therefore, we should use the distribution over expected future returns differently to represent the critical information that the agent requires at decision time by taking both the future and accrued returns into consideration. In this paper, we propose two novel Monte Carlo tree search algorithms. Firstly, we present a Monte Carlo tree search algorithm that can compute policies for nonlinear utility functions (NLU-MCTS) by optimising the utility of the different possible returns attainable from individual policy executions, resulting in good policies for both risk-aware and multi-objective settings. Secondly, we propose a distributional Monte Carlo tree search algorithm (DMCTS) which extends NLU-MCTS. DMCTS computes an approximate posterior distribution over the utility of the returns, and utilises Thompson sampling during planning to compute policies in risk-aware and multi-objective settings. Both algorithms outperform the state-of-the-art in multi-objective reinforcement learning for the expected utility of the returns.

Pareto Conditioned Networks

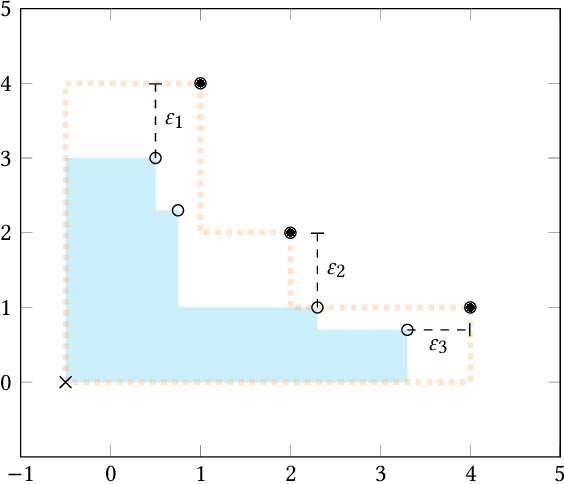
In multi-objective optimization, learning all the policies that reach Pareto-efficient solutions is an expensive process. The set of optimal policies can grow exponentially with the number of objectives, and recovering all solutions requires an exhaustive exploration of the entire state space. We propose Pareto Conditioned Networks (PCN), a method that uses a single neural network to encompass all non-dominated policies. PCN associates every past transition with its episode's return. It trains the network such that, when conditioned on this same return, it should reenact said transition. In doing so we transform the optimization problem into a classification problem. We recover a concrete policy by conditioning the network on the desired Pareto-efficient solution. Our method is stable as it learns in a supervised fashion, thus avoiding moving target issues. Moreover, by using a single network, PCN scales efficiently with the number of objectives. Finally, it makes minimal assumptions on the shape of the Pareto front, which makes it suitable to a wider range of problems than previous state-of-the-art multi-objective reinforcement learning algorithms.

Exploring the Pareto front of multi-objective COVID-19 mitigation policies using reinforcement learning

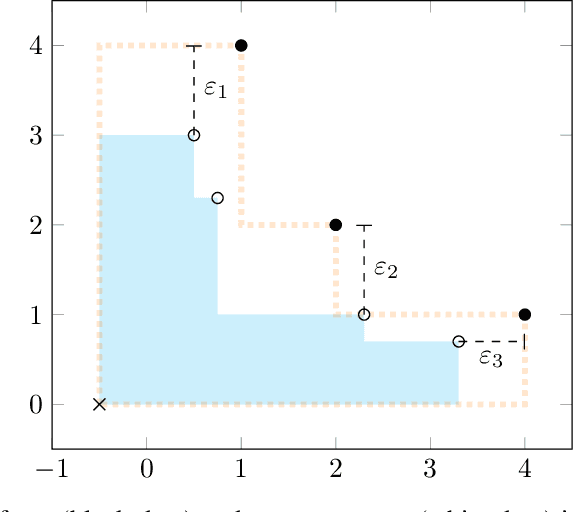
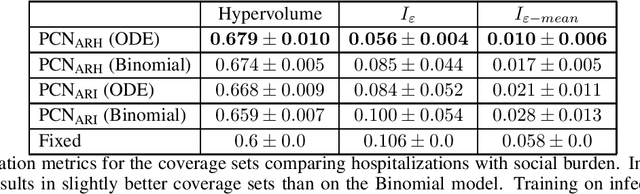
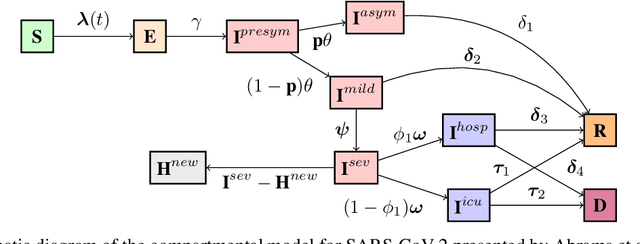
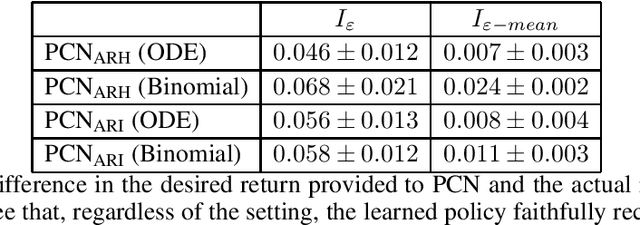
Infectious disease outbreaks can have a disruptive impact on public health and societal processes. As decision making in the context of epidemic mitigation is hard, reinforcement learning provides a methodology to automatically learn prevention strategies in combination with complex epidemic models. Current research focuses on optimizing policies w.r.t. a single objective, such as the pathogen's attack rate. However, as the mitigation of epidemics involves distinct, and possibly conflicting criteria (i.a., prevalence, mortality, morbidity, cost), a multi-objective approach is warranted to learn balanced policies. To lift this decision-making process to real-world epidemic models, we apply deep multi-objective reinforcement learning and build upon a state-of-the-art algorithm, Pareto Conditioned Networks (PCN), to learn a set of solutions that approximates the Pareto front of the decision problem. We consider the first wave of the Belgian COVID-19 epidemic, which was mitigated by a lockdown, and study different deconfinement strategies, aiming to minimize both COVID-19 cases (i.e., infections and hospitalizations) and the societal burden that is induced by the applied mitigation measures. We contribute a multi-objective Markov decision process that encapsulates the stochastic compartment model that was used to inform policy makers during the COVID-19 epidemic. As these social mitigation measures are implemented in a continuous action space that modulates the contact matrix of the age-structured epidemic model, we extend PCN to this setting. We evaluate the solution returned by PCN, and observe that it correctly learns to reduce the social burden whenever the hospitalization rates are sufficiently low. In this work, we thus show that multi-objective reinforcement learning is attainable in complex epidemiological models and provides essential insights to balance complex mitigation policies.

Local Advantage Networks for Cooperative Multi-Agent Reinforcement Learning

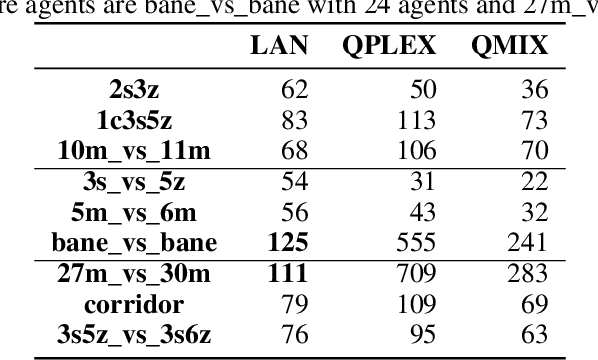
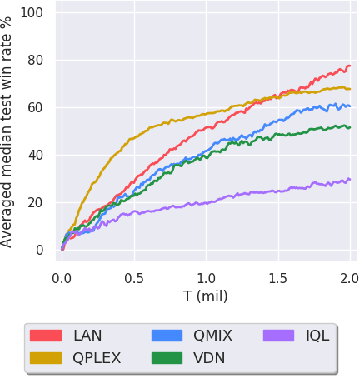
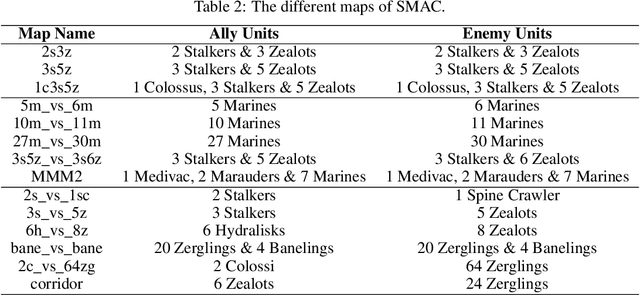
Multi-agent reinforcement learning (MARL) enables us to create adaptive agents in challenging environments, even when the agents have limited observation. Modern MARL methods have hitherto focused on finding factorized value functions. While this approach has proven successful, the resulting methods have convoluted network structures. We take a radically different approach, and build on the structure of independent Q-learners. Inspired by influence-based abstraction, we start from the observation that compact representations of the observation-action histories can be sufficient to learn close to optimal decentralized policies. Combining this observation with a dueling architecture, our algorithm, LAN, represents these policies as separate individual advantage functions w.r.t. a centralized critic. These local advantage networks condition only on a single agent's local observation-action history. The centralized value function conditions on the agents' representations as well as the full state of the environment. The value function, which is cast aside before execution, serves as a stabilizer that coordinates the learning and to formulate DQN targets during learning. In contrast with other methods, this enables LAN to keep the number of network parameters of its centralized network independent in the number of agents, without imposing additional constraints like monotonic value functions. When evaluated on the StarCraft multi-agent challenge benchmark, LAN shows state-of-the-art performance and scores more than 80% wins in two previously unsolved maps `corridor' and `3s5z_vs_3s6z', leading to an improvement of 10% over QPLEX on average performance on the 14 maps. Moreover when the number of agents becomes large, LAN uses significantly fewer parameters than QPLEX or even QMIX. We thus show that LAN's structure forms a key improvement that helps MARL methods remain scalable.

A Practical Guide to Multi-Objective Reinforcement Learning and Planning

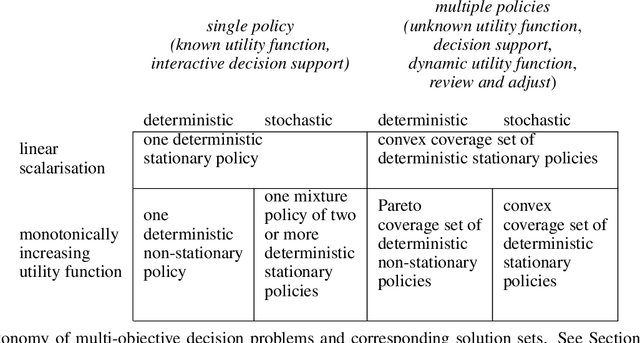
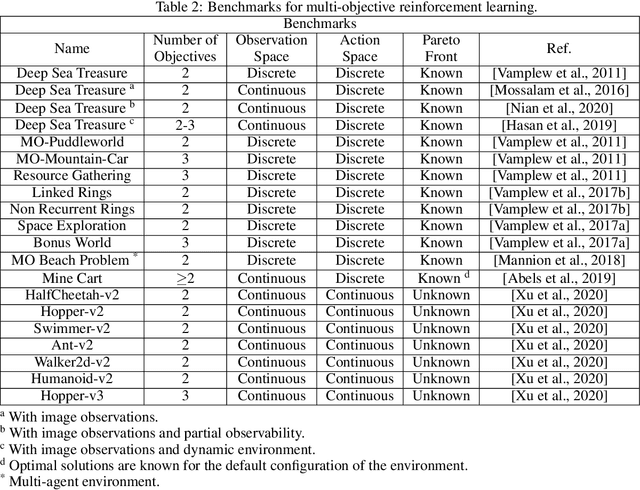
Real-world decision-making tasks are generally complex, requiring trade-offs between multiple, often conflicting, objectives. Despite this, the majority of research in reinforcement learning and decision-theoretic planning either assumes only a single objective, or that multiple objectives can be adequately handled via a simple linear combination. Such approaches may oversimplify the underlying problem and hence produce suboptimal results. This paper serves as a guide to the application of multi-objective methods to difficult problems, and is aimed at researchers who are already familiar with single-objective reinforcement learning and planning methods who wish to adopt a multi-objective perspective on their research, as well as practitioners who encounter multi-objective decision problems in practice. It identifies the factors that may influence the nature of the desired solution, and illustrates by example how these influence the design of multi-objective decision-making systems for complex problems.

Risk Aware and Multi-Objective Decision Making with Distributional Monte Carlo Tree Search

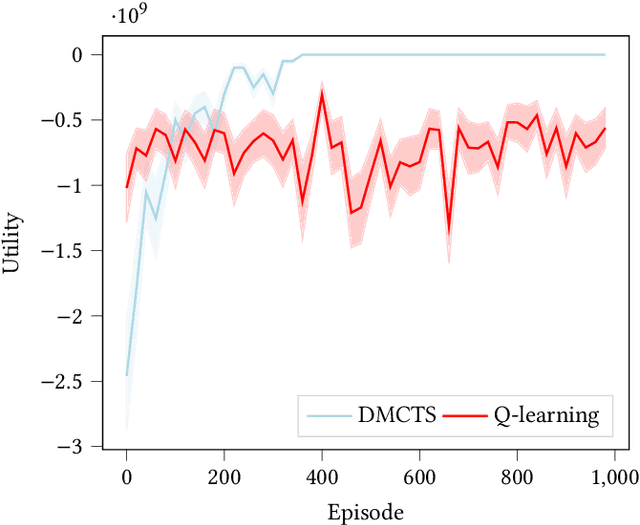
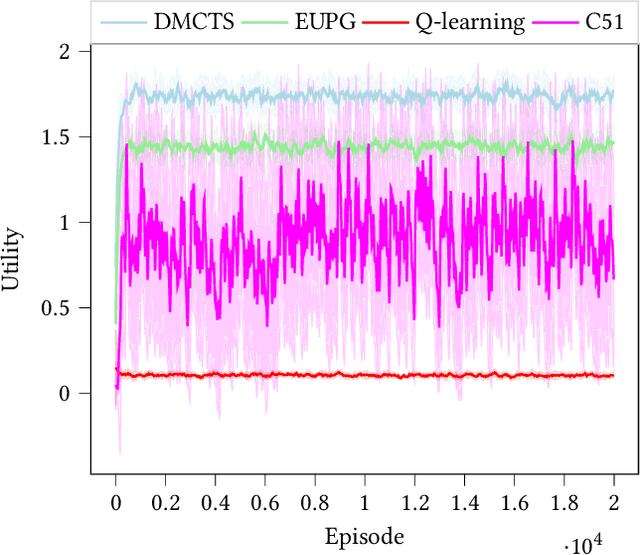
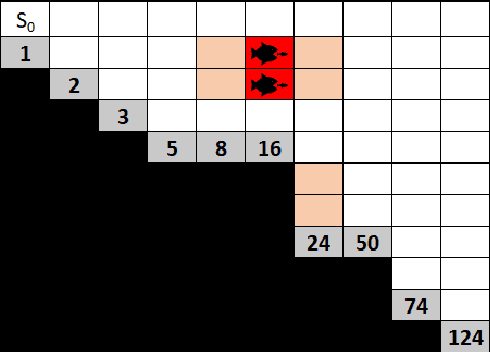
In many risk-aware and multi-objective reinforcement learning settings, the utility of the user is derived from the single execution of a policy. In these settings, making decisions based on the average future returns is not suitable. For example, in a medical setting a patient may only have one opportunity to treat their illness. When making a decision, just the expected return -- known in reinforcement learning as the value -- cannot account for the potential range of adverse or positive outcomes a decision may have. Our key insight is that we should use the distribution over expected future returns differently to represent the critical information that the agent requires at decision time. In this paper, we propose Distributional Monte Carlo Tree Search, an algorithm that learns a posterior distribution over the utility of the different possible returns attainable from individual policy executions, resulting in good policies for both risk-aware and multi-objective settings. Moreover, our algorithm outperforms the state-of-the-art in multi-objective reinforcement learning for the expected utility of the returns.