Doctor Rashomon and the UNIVERSE of Madness: Variable Importance with Unobserved Confounding and the Rashomon Effect

Variable importance (VI) methods are often used for hypothesis generation, feature selection, and scientific validation. In the standard VI pipeline, an analyst estimates VI for a single predictive model with only the observed features. However, the importance of a feature depends heavily on which other variables are included in the model, and essential variables are often omitted from observational datasets. Moreover, the VI estimated for one model is often not the same as the VI estimated for another equally-good model - a phenomenon known as the Rashomon Effect. We address these gaps by introducing UNobservables and Inference for Variable importancE using Rashomon SEts (UNIVERSE). Our approach adapts Rashomon sets - the sets of near-optimal models in a dataset - to produce bounds on the true VI even with missing features. We theoretically guarantee the robustness of our approach, show strong performance on semi-synthetic simulations, and demonstrate its utility in a credit risk task.

Leveraging Predictive Equivalence in Decision Trees

Decision trees are widely used for interpretable machine learning due to their clearly structured reasoning process. However, this structure belies a challenge we refer to as predictive equivalence: a given tree's decision boundary can be represented by many different decision trees. The presence of models with identical decision boundaries but different evaluation processes makes model selection challenging. The models will have different variable importance and behave differently in the presence of missing values, but most optimization procedures will arbitrarily choose one such model to return. We present a boolean logical representation of decision trees that does not exhibit predictive equivalence and is faithful to the underlying decision boundary. We apply our representation to several downstream machine learning tasks. Using our representation, we show that decision trees are surprisingly robust to test-time missingness of feature values; we address predictive equivalence's impact on quantifying variable importance; and we present an algorithm to optimize the cost of reaching predictions.

Data Fusion for Partial Identification of Causal Effects

Data fusion techniques integrate information from heterogeneous data sources to improve learning, generalization, and decision making across data sciences. In causal inference, these methods leverage rich observational data to improve causal effect estimation, while maintaining the trustworthiness of randomized controlled trials. Existing approaches often relax the strong no unobserved confounding assumption by instead assuming exchangeability of counterfactual outcomes across data sources. However, when both assumptions simultaneously fail - a common scenario in practice - current methods cannot identify or estimate causal effects. We address this limitation by proposing a novel partial identification framework that enables researchers to answer key questions such as: Is the causal effect positive or negative? and How severe must assumption violations be to overturn this conclusion? Our approach introduces interpretable sensitivity parameters that quantify assumption violations and derives corresponding causal effect bounds. We develop doubly robust estimators for these bounds and operationalize breakdown frontier analysis to understand how causal conclusions change as assumption violations increase. We apply our framework to the Project STAR study, which investigates the effect of classroom size on students' third-grade standardized test performance. Our analysis reveals that the Project STAR results are robust to simultaneous violations of key assumptions, both on average and across various subgroups of interest. This strengthens confidence in the study's conclusions despite potential unmeasured biases in the data.

Rashomon Sets for Prototypical-Part Networks: Editing Interpretable Models in Real-Time

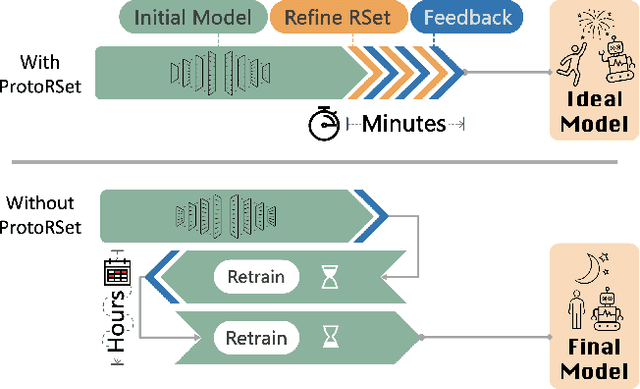
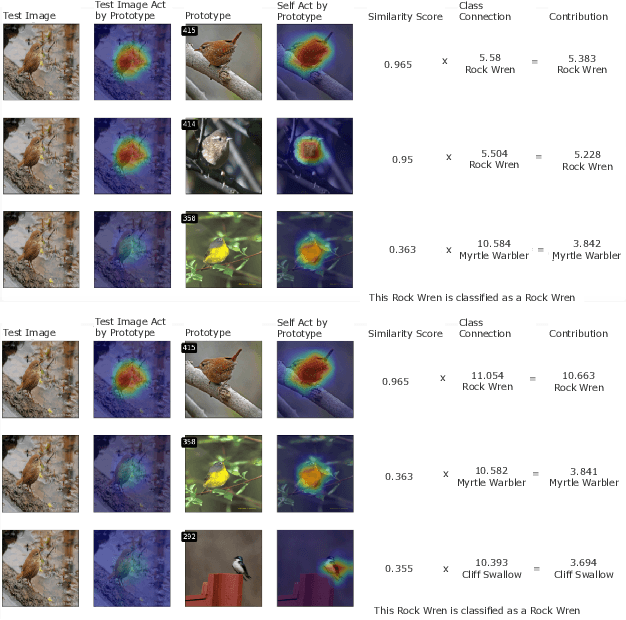
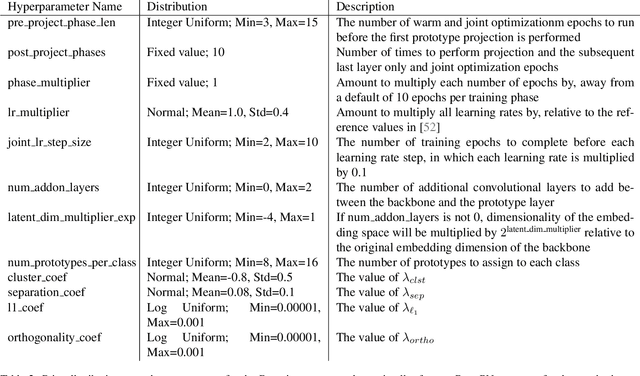
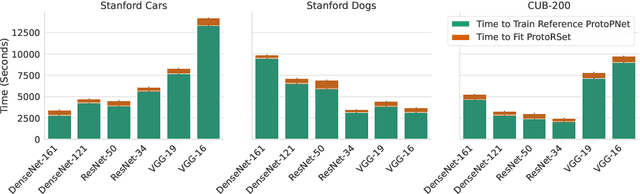
Interpretability is critical for machine learning models in high-stakes settings because it allows users to verify the model's reasoning. In computer vision, prototypical part models (ProtoPNets) have become the dominant model type to meet this need. Users can easily identify flaws in ProtoPNets, but fixing problems in a ProtoPNet requires slow, difficult retraining that is not guaranteed to resolve the issue. This problem is called the "interaction bottleneck." We solve the interaction bottleneck for ProtoPNets by simultaneously finding many equally good ProtoPNets (i.e., a draw from a "Rashomon set"). We show that our framework - called Proto-RSet - quickly produces many accurate, diverse ProtoPNets, allowing users to correct problems in real time while maintaining performance guarantees with respect to the training set. We demonstrate the utility of this method in two settings: 1) removing synthetic bias introduced to a bird identification model and 2) debugging a skin cancer identification model. This tool empowers non-machine-learning experts, such as clinicians or domain experts, to quickly refine and correct machine learning models without repeated retraining by machine learning experts.

Models That Are Interpretable But Not Transparent

Faithful explanations are essential for machine learning models in high-stakes applications. Inherently interpretable models are well-suited for these applications because they naturally provide faithful explanations by revealing their decision logic. However, model designers often need to keep these models proprietary to maintain their value. This creates a tension: we need models that are interpretable--allowing human decision-makers to understand and justify predictions, but not transparent, so that the model's decision boundary is not easily replicated by attackers. Shielding the model's decision boundary is particularly challenging alongside the requirement of completely faithful explanations, since such explanations reveal the true logic of the model for an entire subspace around each query point. This work provides an approach, FaithfulDefense, that creates model explanations for logical models that are completely faithful, yet reveal as little as possible about the decision boundary. FaithfulDefense is based on a maximum set cover formulation, and we provide multiple formulations for it, taking advantage of submodularity.

Near Optimal Decision Trees in a SPLIT Second

Decision tree optimization is fundamental to interpretable machine learning. The most popular approach is to greedily search for the best feature at every decision point, which is fast but provably suboptimal. Recent approaches find the global optimum using branch and bound with dynamic programming, showing substantial improvements in accuracy and sparsity at great cost to scalability. An ideal solution would have the accuracy of an optimal method and the scalability of a greedy method. We introduce a family of algorithms called SPLIT (SParse Lookahead for Interpretable Trees) that moves us significantly forward in achieving this ideal balance. We demonstrate that not all sub-problems need to be solved to optimality to find high quality trees; greediness suffices near the leaves. Since each depth adds an exponential number of possible trees, this change makes our algorithms orders of magnitude faster than existing optimal methods, with negligible loss in performance. We extend this algorithm to allow scalable computation of sets of near-optimal trees (i.e., the Rashomon set).

How Your Location Relates to Health: Variable Importance and Interpretable Machine Learning for Environmental and Sociodemographic Data

Health outcomes depend on complex environmental and sociodemographic factors whose effects change over location and time. Only recently has fine-grained spatial and temporal data become available to study these effects, namely the MEDSAT dataset of English health, environmental, and sociodemographic information. Leveraging this new resource, we use a variety of variable importance techniques to robustly identify the most informative predictors across multiple health outcomes. We then develop an interpretable machine learning framework based on Generalized Additive Models (GAMs) and Multiscale Geographically Weighted Regression (MGWR) to analyze both local and global spatial dependencies of each variable on various health outcomes. Our findings identify NO2 as a global predictor for asthma, hypertension, and anxiety, alongside other outcome-specific predictors related to occupation, marriage, and vegetation. Regional analyses reveal local variations with air pollution and solar radiation, with notable shifts during COVID. This comprehensive approach provides actionable insights for addressing health disparities, and advocates for the integration of interpretable machine learning in public health.

Dimension Reduction with Locally Adjusted Graphs

Dimension reduction (DR) algorithms have proven to be extremely useful for gaining insight into large-scale high-dimensional datasets, particularly finding clusters in transcriptomic data. The initial phase of these DR methods often involves converting the original high-dimensional data into a graph. In this graph, each edge represents the similarity or dissimilarity between pairs of data points. However, this graph is frequently suboptimal due to unreliable high-dimensional distances and the limited information extracted from the high-dimensional data. This problem is exacerbated as the dataset size increases. If we reduce the size of the dataset by selecting points for a specific sections of the embeddings, the clusters observed through DR are more separable since the extracted subgraphs are more reliable. In this paper, we introduce LocalMAP, a new dimensionality reduction algorithm that dynamically and locally adjusts the graph to address this challenge. By dynamically extracting subgraphs and updating the graph on-the-fly, LocalMAP is capable of identifying and separating real clusters within the data that other DR methods may overlook or combine. We demonstrate the benefits of LocalMAP through a case study on biological datasets, highlighting its utility in helping users more accurately identify clusters for real-world problems.

Interpretable Generalized Additive Models for Datasets with Missing Values

Many important datasets contain samples that are missing one or more feature values. Maintaining the interpretability of machine learning models in the presence of such missing data is challenging. Singly or multiply imputing missing values complicates the model's mapping from features to labels. On the other hand, reasoning on indicator variables that represent missingness introduces a potentially large number of additional terms, sacrificing sparsity. We solve these problems with M-GAM, a sparse, generalized, additive modeling approach that incorporates missingness indicators and their interaction terms while maintaining sparsity through l0 regularization. We show that M-GAM provides similar or superior accuracy to prior methods while significantly improving sparsity relative to either imputation or naive inclusion of indicator variables.

Navigating the Effect of Parametrization for Dimensionality Reduction

Parametric dimensionality reduction methods have gained prominence for their ability to generalize to unseen datasets, an advantage that traditional approaches typically lack. Despite their growing popularity, there remains a prevalent misconception among practitioners about the equivalence in performance between parametric and non-parametric methods. Here, we show that these methods are not equivalent -- parametric methods retain global structure but lose significant local details. To explain this, we provide evidence that parameterized approaches lack the ability to repulse negative pairs, and the choice of loss function also has an impact. Addressing these issues, we developed a new parametric method, ParamRepulsor, that incorporates Hard Negative Mining and a loss function that applies a strong repulsive force. This new method achieves state-of-the-art performance on local structure preservation for parametric methods without sacrificing the fidelity of global structural representation. Our code is available at https://github.com/hyhuang00/ParamRepulsor.