Beyond-Diagonal RIS Architecture Design and Optimization under Physics-Consistent Models

Reconfigurable intelligent surface (RIS) is a promising technology for future wireless communication systems. Conventional RIS is constrained to a diagonal scattering matrix, which limits its flexibility. Recently, beyond-diagonal RIS (BD-RIS) has been proposed as a more general RIS architecture class that allows inter-element connections and shows great potential for performance improvement. Despite extensive progress on BD-RIS, most existing studies rely on simplified channel models that ignore practical electromagnetic (EM) effects such as mutual coupling and impedance mismatching. To address this gap, this paper investigates the architecture design and optimization of BD-RIS under the general physics-consistent model derived with multiport network theory in recent literature. Building on a compact reformulation of this model, we show that band-connected RIS achieves the same channel-shaping capability as fully-connected RIS, which extends existing results obtained for conventional channel models. We then develop optimization methods under the general physics-consistent model; specifically, we derive closed-form solutions for single-input single-output (SISO) systems, propose a globally optimal semidefinite relaxation (SDR)-based algorithm for single-stream multi-input multi-output (MIMO) systems, and design an efficient alternating direction method of multipliers (ADMM)-based algorithm for multiuser MIMO systems. Using the proposed algorithms, we conduct comprehensive simulations to evaluate the impact of various EM effects and approximations, including mutual coupling among RIS antennas and the commonly adopted unilateral approximation, on system performance.

Asymptotic Analysis of Nonlinear One-Bit Precoding in Massive MIMO Systems via Approximate Message Passing

Massive multiple-input multiple-output (MIMO) systems employing one-bit digital-to-analog converters offer a hardware-efficient solution for wireless communications. However, the one-bit constraint poses significant challenges for precoding design, as it transforms the problem into a discrete and nonconvex optimization task. In this paper, we investigate a widely adopted ``convex-relaxation-then-quantization" approach for nonlinear symbol-level one-bit precoding. Specifically, we first solve a convex relaxation of the discrete minimum mean square error precoding problem, and then quantize the solution to satisfy the one-bit constraint. To analyze the high-dimensional asymptotic performance of this scheme, we develop a novel analytical framework based on approximate message passing (AMP). This framework enables us to derive a closed-form expression for the symbol error probability (SEP) at the receiver side in the large-system limit, which provides a quantitative characterization of how model and system parameters affect the SEP performance. Our empirical results suggest that the $\ell_\infty^2$ regularizer, when paired with an optimally chosen regularization parameter, achieves optimal SEP performance within a broad class of convex regularization functions. As a first step towards a theoretical justification, we prove the optimality of the $\ell_\infty^2$ regularizer within the mixed $\ell_\infty^2$-$\ell_2^2$ regularization functions.

Lossy Beyond Diagonal Reconfigurable Intelligent Surfaces: Modeling and Optimization

Beyond diagonal reconfigurable intelligent surface (BD-RIS) has emerged as an advancement and generalization of the conventional diagonal RIS (D-RIS) by introducing tunable interconnections between RIS elements, enabling smarter wave manipulation and enlarged coverage. While BD-RIS has demonstrated advantages over D-RIS in various aspects, most existing works rely on the assumption of a lossless model, leaving practical considerations unaddressed. This paper thus proposes a lossy BD-RIS model and develops corresponding optimization algorithms for various BD-RIS-aided communication systems. First, by leveraging admittance parameter analysis, we model each tunable admittance based on a lumped circuit with losses and derive an expression of a circle characterizing the real and imaginary parts of each tunable admittance. We then consider the received signal power maximization in single-user single-input single-output (SISO) systems with the proposed lossy BD-RIS model. To solve the optimization problem, we design an effective algorithm by carefully exploiting the problem structure. Specifically, an alternating direction method of multipliers (ADMM) framework is custom-designed to deal with the complicated constraints associated with lossy BD-RIS. Furthermore, we extend the proposed algorithmic framework to more general multiuser multiple-input single-output (MU-MISO) systems, where the transmit precoder and BD-RIS scattering matrix are jointly designed to maximize the sum-rate of the system. Finally, simulation results demonstrate that all BD-RIS architectures still outperform D-RIS in the presence of losses, but the optimal BD-RIS architectures in the lossless case are not necessarily optimal in the lossy case, e.g. group-connected BD-RIS can outperform fully- and tree-connected BD-RISs in SISO systems with relatively high losses, whereas the opposite always holds true in the lossless case.

Beyond Diagonal RIS in Multiuser MIMO: Graph Theoretic Modeling and Optimal Architectures with Low Complexity

Reconfigurable intelligent surfaces (RIS) is regarded as a key enabler of wave/analog-domain beamforming, processing, and computing in future wireless communication systems. Recently, Beyond Diagonal RIS (BD-RIS) has been proposed as a generalization of conventional RIS, offering enhanced design flexibility thanks to the presence of tunable impedances that connect RIS elements. However, increased interconnections lead to high circuit complexity, which poses a significant practical challenge. In this paper, we address the fundamental open question: What is the class of BD-RIS architectures that achieves the optimal performance in a RIS-aided multiuser multi-input multi-output (MIMO) system? By modeling BD-RIS architectures using graph theory, we identify a class of BD-RIS architectures that achieves the optimal performance--matching that of fully-connected RIS--while maintaining low circuit complexity. Our result holds for a broad class of performance metrics, including the commonly used sum channel gain/sum-rate/energy efficiency maximization, transmit power minimization, and the information-theoretic capacity region. The number of tunable impedances in the proposed class is ${O}(N\min\{D,N/2\})$, where $N$ denotes the number of RIS elements and $D$ is the degree of freedom of the multiuser MIMO channel, i.e., the minimum between the number of transmit antennas and the total number of received antennas across all users. Since $D$ is much smaller than $N$ in practice, the complexity scales as ${O}(ND)$, which is substantially lower than the ${O}(N^2)$ complexity of fully-connected RIS. We further introduce two novel BD-RIS architectures--band-connected RIS and stem-connected RIS--and show that they belong to the optimal architecture class under certain conditions. Simulation results validate the optimality and enhanced performance-complexity tradeoff of our proposed architecture.

Optimization of Beyond Diagonal RIS: A Universal Framework Applicable to Arbitrary Architectures

Reconfigurable intelligent surfaces (RISs) are envisioned as a promising technology for future wireless communication systems due to their ability to control the propagation environment in a hardware- and energy-efficient way. Recently, the concept of RISs has been extended to beyond diagonal RISs (BD-RISs), which unlock the full potential of RISs thanks to the presence of tunable interconnections between RIS elements. While various algorithms have been proposed for specific BD-RIS architectures, a universal optimization framework applicable to arbitrary architectures is still lacking. In this paper, we bridge this research gap by proposing an architecture-independent framework for BD-RIS optimization, with the main focus on sum-rate maximization and transmit power minimization in multiuser multi-input single-output (MU-MISO) systems. Specifically, we first incorporate BD-RIS architectures into the models by connecting the scattering matrix with the admittance matrix and introducing appropriate constraints to the admittance matrix. The formulated problems are then solved by our custom-designed partially proximal alternating direction method of multipliers (pp-ADMM) algorithms. The pp-ADMM algorithms are computationally efficient, with each subproblem either admitting a closed-form solution or being easily solvable. We further explore the extension of the proposed framework to general utility functions and multiuser multi-input multi-output (MU-MIMO) systems. Simulation results demonstrate that the proposed approaches achieve a better trade-off between performance and computational efficiency compared to existing methods. We also compare the performance of various BD-RIS architectures in MU-MISO systems using the proposed approach, which has not been explored before due to the lack of an architecture-independent framework.

An Efficient Convex-Hull Relaxation Based Algorithm for Multi-User Discrete Passive Beamforming

Intelligent reflecting surface (IRS) is an emerging technology to enhance spatial multiplexing in wireless networks. This letter considers the discrete passive beamforming design for IRS in order to maximize the minimum signal-to-interference-plus-noise ratio (SINR) among multiple users in an IRS-assisted downlink network. The main design difficulty lies in the discrete phase-shift constraint. Differing from most existing works, this letter advocates a convex-hull relaxation of the discrete constraints which leads to a continuous reformulated problem equivalent to the original discrete problem. This letter further proposes an efficient alternating projection/proximal gradient descent and ascent algorithm for solving the reformulated problem. Simulation results show that the proposed algorithm outperforms the state-of-the-art methods significantly.

Quantized Constant-Envelope Waveform Design for Massive MIMO DFRC Systems

Both dual-functional radar-communication (DFRC) and massive multiple-input multiple-output (MIMO) have been recognized as enabling technologies for 6G wireless networks. This paper considers the advanced waveform design for hardware-efficient massive MIMO DFRC systems. Specifically, the transmit waveform is imposed with the quantized constant-envelope (QCE) constraint, which facilitates the employment of low-resolution digital-to-analog converters (DACs) and power-efficient amplifiers. The waveform design problem is formulated as the minimization of the mean square error (MSE) between the designed and desired beampatterns subject to the constructive interference (CI)-based communication quality of service (QoS) constraints and the QCE constraint. To solve the formulated problem, we first utilize the penalty technique to transform the discrete problem into an equivalent continuous penalty model. Then, we propose an inexact augmented Lagrangian method (ALM) algorithm for solving the penalty model. In particular, the ALM subproblem at each iteration is solved by a custom-built block successive upper-bound minimization (BSUM) algorithm, which admits closed-form updates, making the proposed inexact ALM algorithm computationally efficient. Simulation results demonstrate the superiority of the proposed approach over existing state-of-the-art ones. In addition, extensive simulations are conducted to examine the impact of various system parameters on the trade-off between communication and radar performances.

A Survey of Advances in Optimization Methods for Wireless Communication System Design

Mathematical optimization is now widely regarded as an indispensable modeling and solution tool for the design of wireless communications systems. While optimization has played a significant role in the revolutionary progress in wireless communication and networking technologies from 1G to 5G and onto the future 6G, the innovations in wireless technologies have also substantially transformed the nature of the underlying mathematical optimization problems upon which the system designs are based and have sparked significant innovations in the development of methodologies to understand, to analyze, and to solve those problems. In this paper, we provide a comprehensive survey of recent advances in mathematical optimization theory and algorithms for wireless communication system design. We begin by illustrating common features of mathematical optimization problems arising in wireless communication system design. We discuss various scenarios and use cases and their associated mathematical structures from an optimization perspective. We then provide an overview of recent advances in mathematical optimization theory and algorithms, from nonconvex optimization, global optimization, and integer programming, to distributed optimization and learning-based optimization. The key to successful solution of mathematical optimization problems is in carefully choosing and/or developing suitable optimization algorithms (or neural network architectures) that can exploit the underlying problem structure. We conclude the paper by identifying several open research challenges and outlining future research directions.

Asymptotic SEP Analysis and Optimization of Linear-Quantized Precoding in Massive MIMO Systems

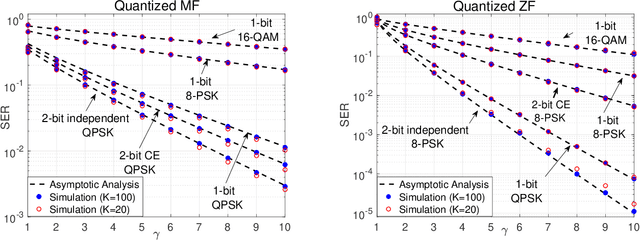
A promising approach to deal with the high hardware cost and energy consumption of massive MIMO transmitters is to use low-resolution digital-to-analog converters (DACs) at each antenna element. This leads to a transmission scheme where the transmitted signals are restricted to a finite set of voltage levels. This paper is concerned with the analysis and optimization of a low-cost quantized precoding strategy, referred to as linear-quantized precoding, for a downlink massive MIMO system under Rayleigh fading. In linear-quantized precoding, the signals are first processed by a linear precoding matrix and subsequently quantized component-wise by the DAC. In this paper, we analyze both the signal-to-interference-plus-noise ratio (SINR) and the symbol error probability (SEP) performances of such linear-quantized precoding schemes in an asymptotic framework where the number of transmit antennas and the number of users grow large with a fixed ratio. Our results provide a rigorous justification for the heuristic arguments based on the Bussgang decomposition that are commonly used in prior works. Based on the asymptotic analysis, we further derive the optimal precoder within a class of linear-quantized precoders that includes several popular precoders as special cases. Our numerical results demonstrate the excellent accuracy of the asymptotic analysis for finite systems and the optimality of the derived precoder.

Linear One-Bit Precoding in Massive MIMO: Asymptotic SEP Analysis and Optimization

This paper focuses on the analysis and optimization of a class of linear one-bit precoding schemes for a downlink massive MIMO system under Rayleigh fading channels. The considered class of linear one-bit precoding is fairly general, including the well-known matched filter (MF) and zero-forcing (ZF) precoding schemes as special cases. Our analysis is based on an asymptotic framework where the numbers of transmit antennas and users in the system grow to infinity with a fixed ratio. We show that, under the asymptotic assumption, the symbol error probability (SEP) of the considered linear one-bit precoding schemes converges to that of a scalar ``signal plus independent Gaussian noise'' model. This result enables us to provide accurate predictions for the SEP of linear one-bit precoding. Additionally, we also derive the optimal linear one-bit precoding scheme within the considered class based on our analytical results. Simulation results demonstrate the excellent accuracy of the SEP prediction and the optimality of the derived precoder.