Asymptotic Analysis of Nonlinear One-Bit Precoding in Massive MIMO Systems via Approximate Message Passing

Massive multiple-input multiple-output (MIMO) systems employing one-bit digital-to-analog converters offer a hardware-efficient solution for wireless communications. However, the one-bit constraint poses significant challenges for precoding design, as it transforms the problem into a discrete and nonconvex optimization task. In this paper, we investigate a widely adopted ``convex-relaxation-then-quantization" approach for nonlinear symbol-level one-bit precoding. Specifically, we first solve a convex relaxation of the discrete minimum mean square error precoding problem, and then quantize the solution to satisfy the one-bit constraint. To analyze the high-dimensional asymptotic performance of this scheme, we develop a novel analytical framework based on approximate message passing (AMP). This framework enables us to derive a closed-form expression for the symbol error probability (SEP) at the receiver side in the large-system limit, which provides a quantitative characterization of how model and system parameters affect the SEP performance. Our empirical results suggest that the $\ell_\infty^2$ regularizer, when paired with an optimally chosen regularization parameter, achieves optimal SEP performance within a broad class of convex regularization functions. As a first step towards a theoretical justification, we prove the optimality of the $\ell_\infty^2$ regularizer within the mixed $\ell_\infty^2$-$\ell_2^2$ regularization functions.

Unifying AMP Algorithms for Rotationally-Invariant Models

This paper presents a unified framework for constructing Approximate Message Passing (AMP) algorithms for rotationally-invariant models. By employing a general iterative algorithm template and reducing it to long-memory Orthogonal AMP (OAMP), we systematically derive the correct Onsager terms of AMP algorithms. This approach allows us to rederive an AMP algorithm introduced by Fan and Opper et al., while shedding new light on the role of free cumulants of the spectral law. The free cumulants arise naturally from a recursive centering operation, potentially of independent interest beyond the scope of AMP. To illustrate the flexibility of our framework, we introduce two novel AMP variants and apply them to estimation in spiked models.

Covariance-Based Device Activity Detection with Massive MIMO for Near-Field Correlated Channels

This paper studies the device activity detection problem in a massive multiple-input multiple-output (MIMO) system for near-field communications (NFC). In this system, active devices transmit their signature sequences to the base station (BS), which detects the active devices based on the received signal. In this paper, we model the near-field channels as correlated Rician fading channels and formulate the device activity detection problem as a maximum likelihood estimation (MLE) problem. Compared to the traditional uncorrelated channel model, the correlation of channels complicates both algorithm design and theoretical analysis of the MLE problem. On the algorithmic side, we propose two computationally efficient algorithms for solving the MLE problem: an exact coordinate descent (CD) algorithm and an inexact CD algorithm. The exact CD algorithm solves the one-dimensional optimization subproblem exactly using matrix eigenvalue decomposition and polynomial root-finding. By approximating the objective function appropriately, the inexact CD algorithm solves the one-dimensional optimization subproblem inexactly with lower complexity and more robust numerical performance. Additionally, we analyze the detection performance of the MLE problem under correlated channels by comparing it with the case of uncorrelated channels. The analysis shows that when the overall number of devices $N$ is large or the signature sequence length $L$ is small, the detection performance of MLE under correlated channels tends to be better than that under uncorrelated channels. Conversely, when $N$ is small or $L$ is large, MLE performs better under uncorrelated channels than under correlated ones. Simulation results demonstrate the computational efficiency of the proposed algorithms and verify the correctness of the analysis.

Precise Analysis of Covariance Identifiability for Activity Detection in Grant-Free Random Access

We consider the identifiability issue of maximum likelihood based activity detection in massive MIMO based grant-free random access. A prior work by Chen et al. indicates that the identifiability undergoes a phase transition for commonly-used random signatures. In this paper, we provide an analytical characterization of the boundary of the phase transition curve. Our theoretical results agree well with the numerical experiments.

Optimality of Approximate Message Passing Algorithms for Spiked Matrix Models with Rotationally Invariant Noise

We study the problem of estimating a rank one signal matrix from an observed matrix generated by corrupting the signal with additive rotationally invariant noise. We develop a new class of approximate message-passing algorithms for this problem and provide a simple and concise characterization of their dynamics in the high-dimensional limit. At each iteration, these algorithms exploit prior knowledge about the noise structure by applying a non-linear matrix denoiser to the eigenvalues of the observed matrix and prior information regarding the signal structure by applying a non-linear iterate denoiser to the previous iterates generated by the algorithm. We exploit our result on the dynamics of these algorithms to derive the optimal choices for the matrix and iterate denoisers. We show that the resulting algorithm achieves the smallest possible asymptotic estimation error among a broad class of iterative algorithms under a fixed iteration budget.

Asymptotic SEP Analysis and Optimization of Linear-Quantized Precoding in Massive MIMO Systems

A promising approach to deal with the high hardware cost and energy consumption of massive MIMO transmitters is to use low-resolution digital-to-analog converters (DACs) at each antenna element. This leads to a transmission scheme where the transmitted signals are restricted to a finite set of voltage levels. This paper is concerned with the analysis and optimization of a low-cost quantized precoding strategy, referred to as linear-quantized precoding, for a downlink massive MIMO system under Rayleigh fading. In linear-quantized precoding, the signals are first processed by a linear precoding matrix and subsequently quantized component-wise by the DAC. In this paper, we analyze both the signal-to-interference-plus-noise ratio (SINR) and the symbol error probability (SEP) performances of such linear-quantized precoding schemes in an asymptotic framework where the number of transmit antennas and the number of users grow large with a fixed ratio. Our results provide a rigorous justification for the heuristic arguments based on the Bussgang decomposition that are commonly used in prior works. Based on the asymptotic analysis, we further derive the optimal precoder within a class of linear-quantized precoders that includes several popular precoders as special cases. Our numerical results demonstrate the excellent accuracy of the asymptotic analysis for finite systems and the optimality of the derived precoder.

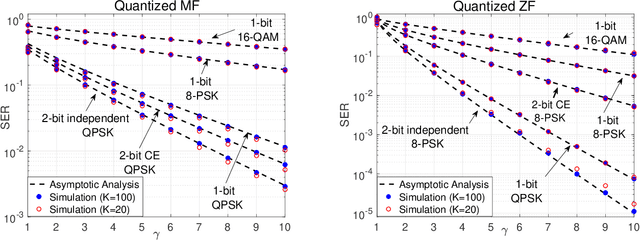
Linear One-Bit Precoding in Massive MIMO: Asymptotic SEP Analysis and Optimization

This paper focuses on the analysis and optimization of a class of linear one-bit precoding schemes for a downlink massive MIMO system under Rayleigh fading channels. The considered class of linear one-bit precoding is fairly general, including the well-known matched filter (MF) and zero-forcing (ZF) precoding schemes as special cases. Our analysis is based on an asymptotic framework where the numbers of transmit antennas and users in the system grow to infinity with a fixed ratio. We show that, under the asymptotic assumption, the symbol error probability (SEP) of the considered linear one-bit precoding schemes converges to that of a scalar ``signal plus independent Gaussian noise'' model. This result enables us to provide accurate predictions for the SEP of linear one-bit precoding. Additionally, we also derive the optimal linear one-bit precoding scheme within the considered class based on our analytical results. Simulation results demonstrate the excellent accuracy of the SEP prediction and the optimality of the derived precoder.

Impact of the Sensing Spectrum on Signal Recovery in Generalized Linear Models

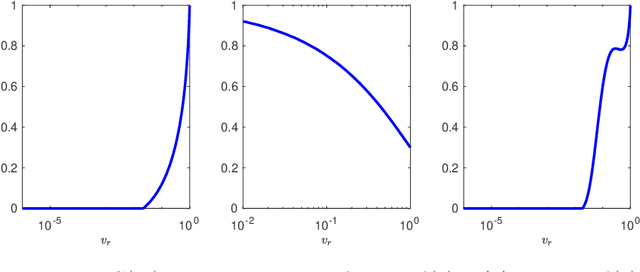
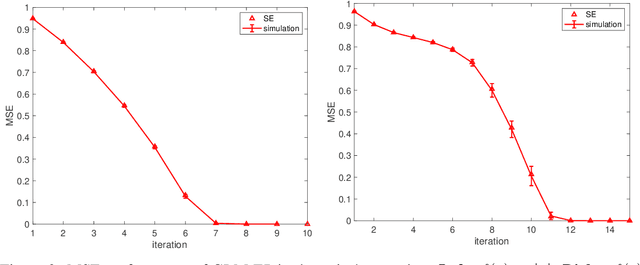
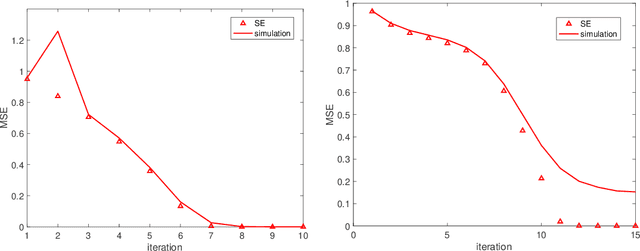
We consider a nonlinear inverse problem $\mathbf{y}= f(\mathbf{Ax})$, where observations $\mathbf{y} \in \mathbb{R}^m$ are the componentwise nonlinear transformation of $\mathbf{Ax} \in \mathbb{R}^m$, $\mathbf{x} \in \mathbb{R}^n$ is the signal of interest and $\mathbf{A}$ is a known linear mapping. By properly specifying the nonlinear processing function, this model can be particularized to many signal processing problems, including compressed sensing and phase retrieval. Our main goal in this paper is to understand the impact of sensing matrices, or more specifically the spectrum of sensing matrices, on the difficulty of recovering $\mathbf{x}$ from $\mathbf{y}$. Towards this goal, we study the performance of one of the most successful recovery methods, i.e. the expectation propagation algorithm (EP). We define a notion for the spikiness of the spectrum of $\mathbf{A}$ and show the importance of this measure in the performance of the EP. Whether the spikiness of the spectrum can hurt or help the recovery performance of EP depends on $f$. We define certain quantities based on the function $f$ that enables us to describe the impact of the spikiness of the spectrum on EP recovery. Based on our framework, we are able to show that for instance, in phase-retrieval problems, matrices with spikier spectrums are better for EP, while in 1-bit compressed sensing problems, less spiky (flatter) spectrums offer better recoveries. Our results unify and substantially generalize the existing results that compare sub-Gaussian and orthogonal matrices, and provide a platform toward designing optimal sensing systems.