AbDiffuser: Full-Atom Generation of In-Vitro Functioning Antibodies

We introduce AbDiffuser, an equivariant and physics-informed diffusion model for the joint generation of antibody 3D structures and sequences. AbDiffuser is built on top of a new representation of protein structure, relies on a novel architecture for aligned proteins, and utilizes strong diffusion priors to improve the denoising process. Our approach improves protein diffusion by taking advantage of domain knowledge and physics-based constraints; handles sequence-length changes; and reduces memory complexity by an order of magnitude enabling backbone and side chain generation. We validate AbDiffuser in silico and in vitro. Numerical experiments showcase the ability of AbDiffuser to generate antibodies that closely track the sequence and structural properties of a reference set. Laboratory experiments confirm that all 16 HER2 antibodies discovered were expressed at high levels and that 57.1% of selected designs were tight binders.

Protein Discovery with Discrete Walk-Jump Sampling

We resolve difficulties in training and sampling from a discrete generative model by learning a smoothed energy function, sampling from the smoothed data manifold with Langevin Markov chain Monte Carlo (MCMC), and projecting back to the true data manifold with one-step denoising. Our Discrete Walk-Jump Sampling formalism combines the maximum likelihood training of an energy-based model and improved sample quality of a score-based model, while simplifying training and sampling by requiring only a single noise level. We evaluate the robustness of our approach on generative modeling of antibody proteins and introduce the distributional conformity score to benchmark protein generative models. By optimizing and sampling from our models for the proposed distributional conformity score, 97-100% of generated samples are successfully expressed and purified and 35% of functional designs show equal or improved binding affinity compared to known functional antibodies on the first attempt in a single round of laboratory experiments. We also report the first demonstration of long-run fast-mixing MCMC chains where diverse antibody protein classes are visited in a single MCMC chain.

PropertyDAG: Multi-objective Bayesian optimization of partially ordered, mixed-variable properties for biological sequence design

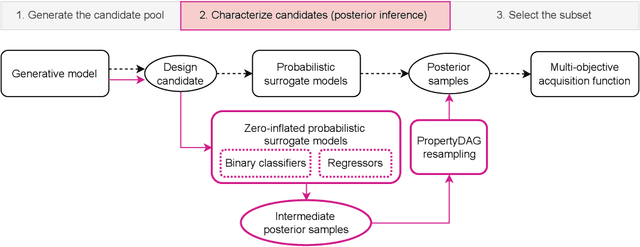
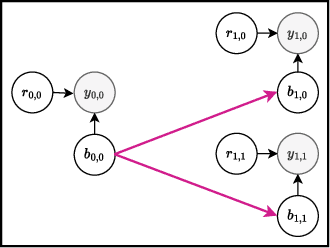
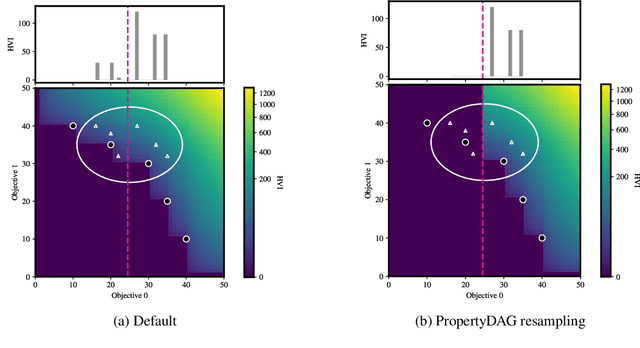
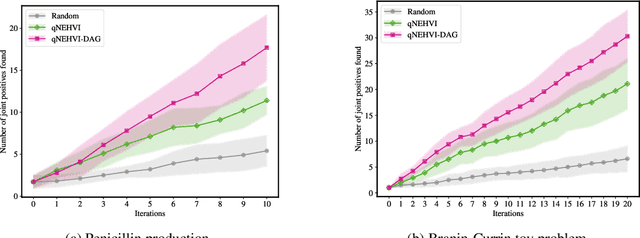
Bayesian optimization offers a sample-efficient framework for navigating the exploration-exploitation trade-off in the vast design space of biological sequences. Whereas it is possible to optimize the various properties of interest jointly using a multi-objective acquisition function, such as the expected hypervolume improvement (EHVI), this approach does not account for objectives with a hierarchical dependency structure. We consider a common use case where some regions of the Pareto frontier are prioritized over others according to a specified $\textit{partial ordering}$ in the objectives. For instance, when designing antibodies, we would like to maximize the binding affinity to a target antigen only if it can be expressed in live cell culture -- modeling the experimental dependency in which affinity can only be measured for antibodies that can be expressed and thus produced in viable quantities. In general, we may want to confer a partial ordering to the properties such that each property is optimized conditioned on its parent properties satisfying some feasibility condition. To this end, we present PropertyDAG, a framework that operates on top of the traditional multi-objective BO to impose this desired ordering on the objectives, e.g. expression $\rightarrow$ affinity. We demonstrate its performance over multiple simulated active learning iterations on a penicillin production task, toy numerical problem, and a real-world antibody design task.

Non-Negative Matrix Factorizations for Multiplex Network Analysis

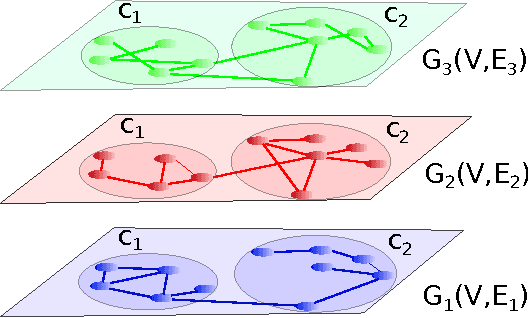
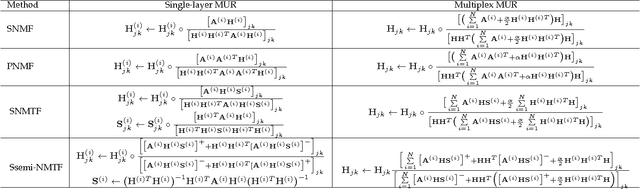
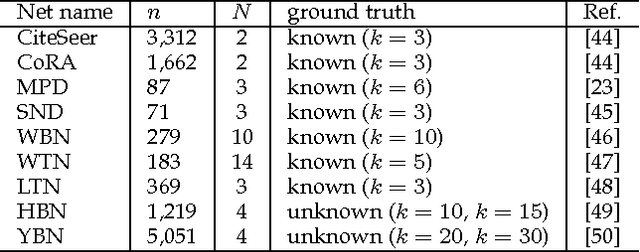
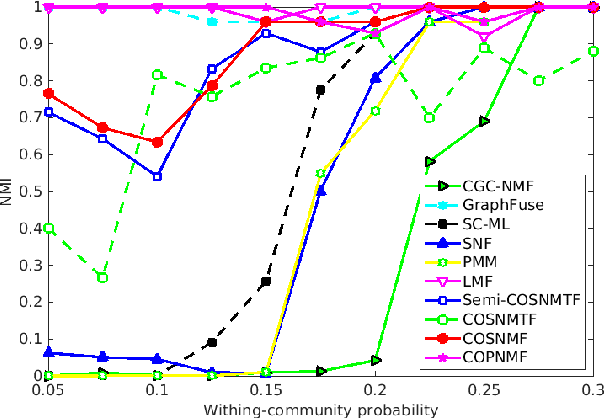
Networks have been a general tool for representing, analyzing, and modeling relational data arising in several domains. One of the most important aspect of network analysis is community detection or network clustering. Until recently, the major focus have been on discovering community structure in single (i.e., monoplex) networks. However, with the advent of relational data with multiple modalities, multiplex networks, i.e., networks composed of multiple layers representing different aspects of relations, have emerged. Consequently, community detection in multiplex network, i.e., detecting clusters of nodes shared by all layers, has become a new challenge. In this paper, we propose Network Fusion for Composite Community Extraction (NF-CCE), a new class of algorithms, based on four different non-negative matrix factorization models, capable of extracting composite communities in multiplex networks. Each algorithm works in two steps: first, it finds a non-negative, low-dimensional feature representation of each network layer; then, it fuses the feature representation of layers into a common non-negative, low-dimensional feature representation via collective factorization. The composite clusters are extracted from the common feature representation. We demonstrate the superior performance of our algorithms over the state-of-the-art methods on various types of multiplex networks, including biological, social, economic, citation, phone communication, and brain multiplex networks.