Urmi Ninad
SpaceTime: Causal Discovery from Non-Stationary Time Series
Jan 17, 2025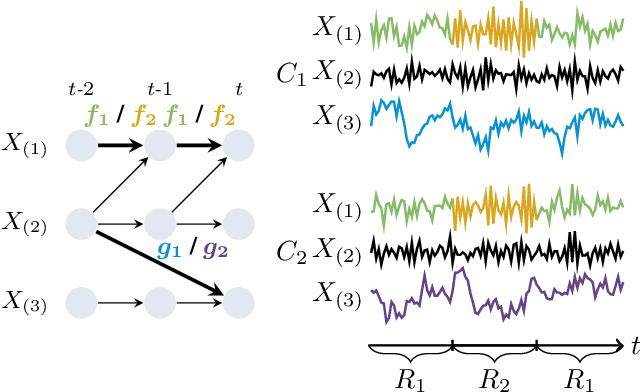
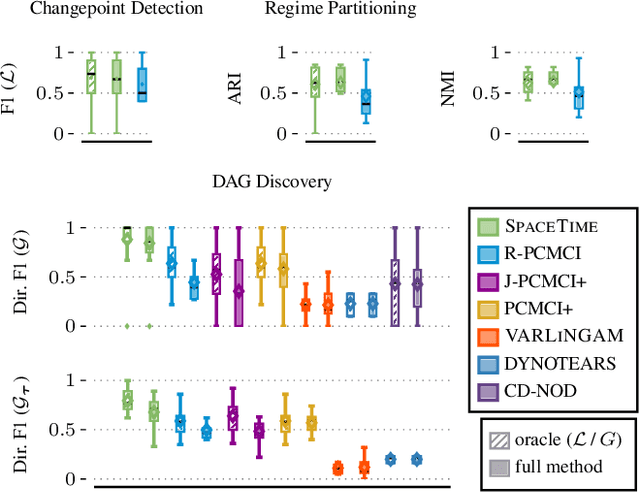
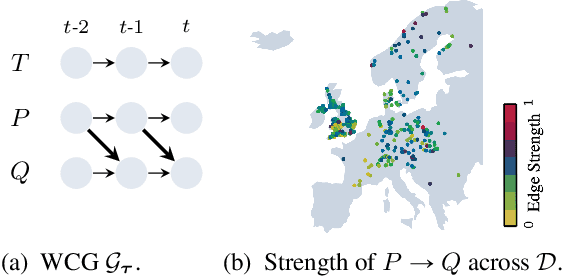
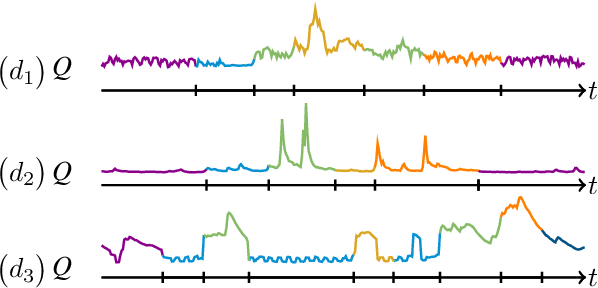
Abstract:Understanding causality is challenging and often complicated by changing causal relationships over time and across environments. Climate patterns, for example, shift over time with recurring seasonal trends, while also depending on geographical characteristics such as ecosystem variability. Existing methods for discovering causal graphs from time series either assume stationarity, do not permit both temporal and spatial distribution changes, or are unaware of locations with the same causal relationships. In this work, we therefore unify the three tasks of causal graph discovery in the non-stationary multi-context setting, of reconstructing temporal regimes, and of partitioning datasets and time intervals into those where invariant causal relationships hold. To construct a consistent score that forms the basis of our method, we employ the Minimum Description Length principle. Our resulting algorithm SPACETIME simultaneously accounts for heterogeneity across space and non-stationarity over time. Given multiple time series, it discovers regime changepoints and a temporal causal graph using non-parametric functional modeling and kernelized discrepancy testing. We also show that our method provides insights into real-world phenomena such as river-runoff measured at different catchments and biosphere-atmosphere interactions across ecosystems.
Causal discovery with endogenous context variables
Dec 06, 2024



Abstract:Causal systems often exhibit variations of the underlying causal mechanisms between the variables of the system. Often, these changes are driven by different environments or internal states in which the system operates, and we refer to context variables as those variables that indicate this change in causal mechanisms. An example are the causal relations in soil moisture-temperature interactions and their dependence on soil moisture regimes: Dry soil triggers a dependence of soil moisture on latent heat, while environments with wet soil do not feature such a feedback, making it a context-specific property. Crucially, a regime or context variable such as soil moisture need not be exogenous and can be influenced by the dynamical system variables - precipitation can make a dry soil wet - leading to joint systems with endogenous context variables. In this work we investigate the assumptions for constraint-based causal discovery of context-specific information in systems with endogenous context variables. We show that naive approaches such as learning different regime graphs on masked data, or pooling all data, can lead to uninformative results. We propose an adaptive constraint-based discovery algorithm and give a detailed discussion on the connection to structural causal models, including sufficiency assumptions, which allow to prove the soundness of our algorithm and to interpret the results causally. Numerical experiments demonstrate the performance of the proposed method over alternative baselines, but they also unveil current limitations of our method.
Invariance & Causal Representation Learning: Prospects and Limitations
Dec 06, 2023Abstract:In causal models, a given mechanism is assumed to be invariant to changes of other mechanisms. While this principle has been utilized for inference in settings where the causal variables are observed, theoretical insights when the variables of interest are latent are largely missing. We assay the connection between invariance and causal representation learning by establishing impossibility results which show that invariance alone is insufficient to identify latent causal variables. Together with practical considerations, we use these theoretical findings to highlight the need for additional constraints in order to identify representations by exploiting invariance.
Identifying Linearly-Mixed Causal Representations from Multi-Node Interventions
Nov 05, 2023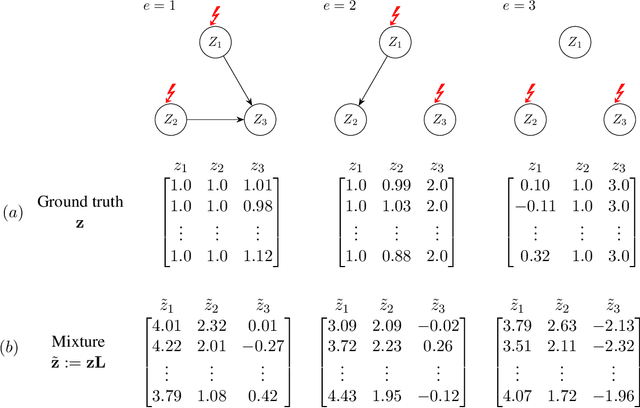
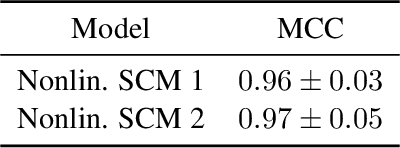

Abstract:The task of inferring high-level causal variables from low-level observations, commonly referred to as causal representation learning, is fundamentally underconstrained. As such, recent works to address this problem focus on various assumptions that lead to identifiability of the underlying latent causal variables. A large corpus of these preceding approaches consider multi-environment data collected under different interventions on the causal model. What is common to virtually all of these works is the restrictive assumption that in each environment, only a single variable is intervened on. In this work, we relax this assumption and provide the first identifiability result for causal representation learning that allows for multiple variables to be targeted by an intervention within one environment. Our approach hinges on a general assumption on the coverage and diversity of interventions across environments, which also includes the shared assumption of single-node interventions of previous works. The main idea behind our approach is to exploit the trace that interventions leave on the variance of the ground truth causal variables and regularizing for a specific notion of sparsity with respect to this trace. In addition to and inspired by our theoretical contributions, we present a practical algorithm to learn causal representations from multi-node interventional data and provide empirical evidence that validates our identifiability results.
Projecting infinite time series graphs to finite marginal graphs using number theory
Oct 09, 2023Abstract:In recent years, a growing number of method and application works have adapted and applied the causal-graphical-model framework to time series data. Many of these works employ time-resolved causal graphs that extend infinitely into the past and future and whose edges are repetitive in time, thereby reflecting the assumption of stationary causal relationships. However, most results and algorithms from the causal-graphical-model framework are not designed for infinite graphs. In this work, we develop a method for projecting infinite time series graphs with repetitive edges to marginal graphical models on a finite time window. These finite marginal graphs provide the answers to $m$-separation queries with respect to the infinite graph, a task that was previously unresolved. Moreover, we argue that these marginal graphs are useful for causal discovery and causal effect estimation in time series, effectively enabling to apply results developed for finite graphs to the infinite graphs. The projection procedure relies on finding common ancestors in the to-be-projected graph and is, by itself, not new. However, the projection procedure has not yet been algorithmically implemented for time series graphs since in these infinite graphs there can be infinite sets of paths that might give rise to common ancestors. We solve the search over these possibly infinite sets of paths by an intriguing combination of path-finding techniques for finite directed graphs and solution theory for linear Diophantine equations. By providing an algorithm that carries out the projection, our paper makes an important step towards a theoretically-grounded and method-agnostic generalization of a range of causal inference methods and results to time series.
Conditional Independence Testing with Heteroskedastic Data and Applications to Causal Discovery
Jun 20, 2023



Abstract:Conditional independence (CI) testing is frequently used in data analysis and machine learning for various scientific fields and it forms the basis of constraint-based causal discovery. Oftentimes, CI testing relies on strong, rather unrealistic assumptions. One of these assumptions is homoskedasticity, in other words, a constant conditional variance is assumed. We frame heteroskedasticity in a structural causal model framework and present an adaptation of the partial correlation CI test that works well in the presence of heteroskedastic noise, given that expert knowledge about the heteroskedastic relationships is available. Further, we provide theoretical consistency results for the proposed CI test which carry over to causal discovery under certain assumptions. Numerical causal discovery experiments demonstrate that the adapted partial correlation CI test outperforms the standard test in the presence of heteroskedasticity and is on par for the homoskedastic case. Finally, we discuss the general challenges and limits as to how expert knowledge about heteroskedasticity can be accounted for in causal discovery.
Discovering Causal Relations and Equations from Data
May 21, 2023Abstract:Physics is a field of science that has traditionally used the scientific method to answer questions about why natural phenomena occur and to make testable models that explain the phenomena. Discovering equations, laws and principles that are invariant, robust and causal explanations of the world has been fundamental in physical sciences throughout the centuries. Discoveries emerge from observing the world and, when possible, performing interventional studies in the system under study. With the advent of big data and the use of data-driven methods, causal and equation discovery fields have grown and made progress in computer science, physics, statistics, philosophy, and many applied fields. All these domains are intertwined and can be used to discover causal relations, physical laws, and equations from observational data. This paper reviews the concepts, methods, and relevant works on causal and equation discovery in the broad field of Physics and outlines the most important challenges and promising future lines of research. We also provide a taxonomy for observational causal and equation discovery, point out connections, and showcase a complete set of case studies in Earth and climate sciences, fluid dynamics and mechanics, and the neurosciences. This review demonstrates that discovering fundamental laws and causal relations by observing natural phenomena is being revolutionised with the efficient exploitation of observational data, modern machine learning algorithms and the interaction with domain knowledge. Exciting times are ahead with many challenges and opportunities to improve our understanding of complex systems.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge