Dynamic Proximal Gradient Algorithms for Schatten-$p$ Quasi-Norm Regularized Problems

This paper investigates numerical solution methods for the Schatten-$p$ quasi-norm regularized problem with $p \in [0,1]$, which has been widely studied for finding low-rank solutions of linear inverse problems and gained successful applications in various mathematics and applied science fields. We propose a dynamic proximal gradient algorithm that, through the use of the Cayley transformation, avoids computationally expensive singular value decompositions at each iteration, thereby significantly reducing the computational complexity. The algorithm incorporates two step size selection strategies: an adaptive backtracking search and an explicit step size rule. We establish the sublinear convergence of the proposed algorithm for all $p \in [0,1]$ within the framework of the Kurdyka-Lojasiewicz property. Notably, under mild assumptions, we show that the generated sequence converges to a stationary point of the objective function of the problem. For the special case when $p=1$, the linear convergence is further proved under the strict complementarity-type regularity condition commonly used in the linear convergence analysis of the forward-backward splitting algorithms. Preliminary numerical results validate the superior computational efficiency of the proposed algorithm.

Dual Quaternion SE(3) Synchronization with Recovery Guarantees

Synchronization over the special Euclidean group SE(3) aims to recover absolute poses from noisy pairwise relative transformations and is a core primitive in robotics and 3D vision. Standard approaches often require multi-step heuristic procedures to recover valid poses, which are difficult to analyze and typically lack theoretical guarantees. This paper adopts a dual quaternion representation and formulates SE(3) synchronization directly over the unit dual quaternion. A two-stage algorithm is developed: A spectral initializer computed via the power method on a Hermitian dual quaternion measurement matrix, followed by a dual quaternion generalized power method (DQGPM) that enforces feasibility through per-iteration projection. The estimation error bounds are established for spectral estimators, and DQGPM is shown to admit a finite-iteration error bound and achieves linear error contraction up to an explicit noise-dependent threshold. Experiments on synthetic benchmarks and real-world multi-scan point-set registration demonstrate that the proposed pipeline improves both accuracy and efficiency over representative matrix-based methods.

Worst-case generation via minimax optimization in Wasserstein space

Worst-case generation plays a critical role in evaluating robustness and stress-testing systems under distribution shifts, in applications ranging from machine learning models to power grids and medical prediction systems. We develop a generative modeling framework for worst-case generation for a pre-specified risk, based on min-max optimization over continuous probability distributions, namely the Wasserstein space. Unlike traditional discrete distributionally robust optimization approaches, which often suffer from scalability issues, limited generalization, and costly worst-case inference, our framework exploits the Brenier theorem to characterize the least favorable (worst-case) distribution as the pushforward of a transport map from a continuous reference measure, enabling a continuous and expressive notion of risk-induced generation beyond classical discrete DRO formulations. Based on the min-max formulation, we propose a Gradient Descent Ascent (GDA)-type scheme that updates the decision model and the transport map in a single loop, establishing global convergence guarantees under mild regularity assumptions and possibly without convexity-concavity. We also propose to parameterize the transport map using a neural network that can be trained simultaneously with the GDA iterations by matching the transported training samples, thereby achieving a simulation-free approach. The efficiency of the proposed method as a risk-induced worst-case generator is validated by numerical experiments on synthetic and image data.

Distributionally Robust Optimization via Iterative Algorithms in Continuous Probability Spaces

We consider a minimax problem motivated by distributionally robust optimization (DRO) when the worst-case distribution is continuous, leading to significant computational challenges due to the infinite-dimensional nature of the optimization problem. Recent research has explored learning the worst-case distribution using neural network-based generative models to address these computational challenges but lacks algorithmic convergence guarantees. This paper bridges this theoretical gap by presenting an iterative algorithm to solve such a minimax problem, achieving global convergence under mild assumptions and leveraging technical tools from vector space minimax optimization and convex analysis in the space of continuous probability densities. In particular, leveraging Brenier's theorem, we represent the worst-case distribution as a transport map applied to a continuous reference measure and reformulate the regularized discrepancy-based DRO as a minimax problem in the Wasserstein space. Furthermore, we demonstrate that the worst-case distribution can be efficiently computed using a modified Jordan-Kinderlehrer-Otto (JKO) scheme with sufficiently large regularization parameters for commonly used discrepancy functions, linked to the radius of the ambiguity set. Additionally, we derive the global convergence rate and quantify the total number of subgradient and inexact modified JKO iterations required to obtain approximate stationary points. These results are potentially applicable to nonconvex and nonsmooth scenarios, with broad relevance to modern machine learning applications.

Locally Differentially Private Online Federated Learning With Correlated Noise

We introduce a locally differentially private (LDP) algorithm for online federated learning that employs temporally correlated noise to improve utility while preserving privacy. To address challenges posed by the correlated noise and local updates with streaming non-IID data, we develop a perturbed iterate analysis that controls the impact of the noise on the utility. Moreover, we demonstrate how the drift errors from local updates can be effectively managed for several classes of nonconvex loss functions. Subject to an $(\epsilon,\delta)$-LDP budget, we establish a dynamic regret bound that quantifies the impact of key parameters and the intensity of changes in the dynamic environment on the learning performance. Numerical experiments confirm the efficacy of the proposed algorithm.

Differentially Private Online Federated Learning with Correlated Noise

We propose a novel differentially private algorithm for online federated learning that employs temporally correlated noise to improve the utility while ensuring the privacy of the continuously released models. To address challenges stemming from DP noise and local updates with streaming noniid data, we develop a perturbed iterate analysis to control the impact of the DP noise on the utility. Moreover, we demonstrate how the drift errors from local updates can be effectively managed under a quasi-strong convexity condition. Subject to an $(\epsilon, \delta)$-DP budget, we establish a dynamic regret bound over the entire time horizon that quantifies the impact of key parameters and the intensity of changes in dynamic environments. Numerical experiments validate the efficacy of the proposed algorithm.

Rotation Group Synchronization via Quotient Manifold

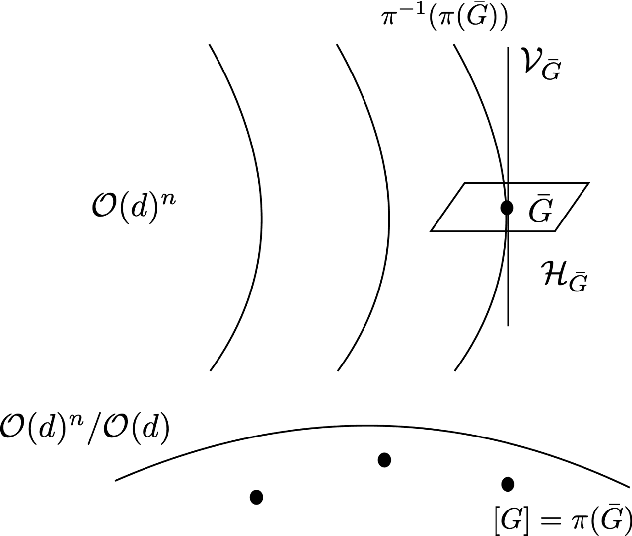
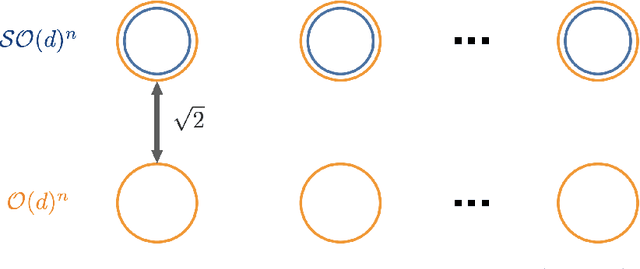
Rotation group $\mathcal{SO}(d)$ synchronization is an important inverse problem and has attracted intense attention from numerous application fields such as graph realization, computer vision, and robotics. In this paper, we focus on the least-squares estimator of rotation group synchronization with general additive noise models, which is a nonconvex optimization problem with manifold constraints. Unlike the phase/orthogonal group synchronization, there are limited provable approaches for solving rotation group synchronization. First, we derive improved estimation results of the least-squares/spectral estimator, illustrating the tightness and validating the existing relaxation methods of solving rotation group synchronization through the optimum of relaxed orthogonal group version under near-optimal noise level for exact recovery. Moreover, departing from the standard approach of utilizing the geometry of the ambient Euclidean space, we adopt an intrinsic Riemannian approach to study orthogonal/rotation group synchronization. Benefiting from a quotient geometric view, we prove the positive definite condition of quotient Riemannian Hessian around the optimum of orthogonal group synchronization problem, and consequently the Riemannian local error bound property is established to analyze the convergence rate properties of various Riemannian algorithms. As a simple and feasible method, the sequential convergence guarantee of the (quotient) Riemannian gradient method for solving orthogonal/rotation group synchronization problem is studied, and we derive its global linear convergence rate to the optimum with the spectral initialization. All results are deterministic without any probabilistic model.

LogSpecT: Feasible Graph Learning Model from Stationary Signals with Recovery Guarantees

Graph learning from signals is a core task in Graph Signal Processing (GSP). One of the most commonly used models to learn graphs from stationary signals is SpecT. However, its practical formulation rSpecT is known to be sensitive to hyperparameter selection and, even worse, to suffer from infeasibility. In this paper, we give the first condition that guarantees the infeasibility of rSpecT and design a novel model (LogSpecT) and its practical formulation (rLogSpecT) to overcome this issue. Contrary to rSpecT, the novel practical model rLogSpecT is always feasible. Furthermore, we provide recovery guarantees of rLogSpecT, which are derived from modern optimization tools related to epi-convergence. These tools could be of independent interest and significant for various learning problems. To demonstrate the advantages of rLogSpecT in practice, a highly efficient algorithm based on the linearized alternating direction method of multipliers (L-ADMM) is proposed. The subproblems of L-ADMM admit closed-form solutions and the convergence is guaranteed. Extensive numerical results on both synthetic and real networks corroborate the stability and superiority of our proposed methods, underscoring their potential for various graph learning applications.

Doubly Smoothed GDA: Global Convergent Algorithm for Constrained Nonconvex-Nonconcave Minimax Optimization

Nonconvex-nonconcave minimax optimization has been the focus of intense research over the last decade due to its broad applications in machine learning and operation research. Unfortunately, most existing algorithms cannot be guaranteed to converge and always suffer from limit cycles. Their global convergence relies on certain conditions that are difficult to check, including but not limited to the global Polyak-\L{}ojasiewicz condition, the existence of a solution satisfying the weak Minty variational inequality and $\alpha$-interaction dominant condition. In this paper, we develop the first provably convergent algorithm called doubly smoothed gradient descent ascent method, which gets rid of the limit cycle without requiring any additional conditions. We further show that the algorithm has an iteration complexity of $\mathcal{O}(\epsilon^{-4})$ for finding a game stationary point, which matches the best iteration complexity of single-loop algorithms under nonconcave-concave settings. The algorithm presented here opens up a new path for designing provable algorithms for nonconvex-nonconcave minimax optimization problems.

Nonsmooth Composite Nonconvex-Concave Minimax Optimization

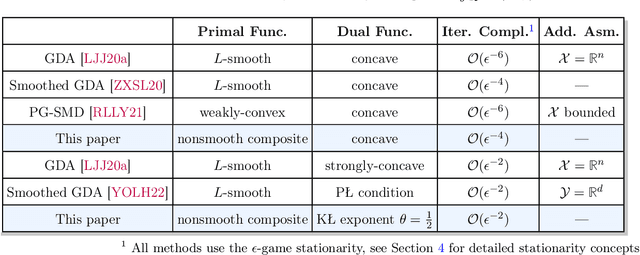
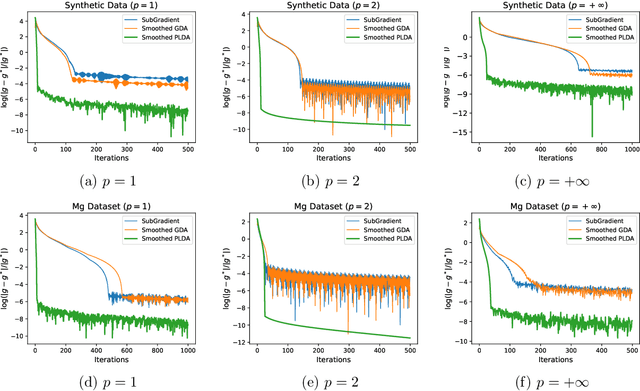
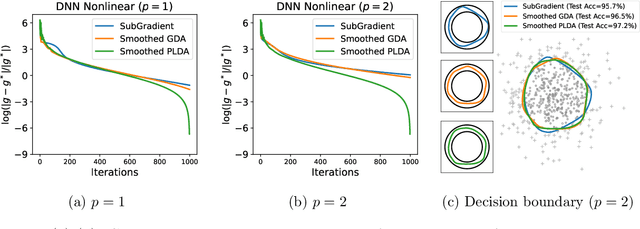
Nonconvex-concave minimax optimization has received intense interest in machine learning, including learning with robustness to data distribution, learning with non-decomposable loss, adversarial learning, to name a few. Nevertheless, most existing works focus on the gradient-descent-ascent (GDA) variants that can only be applied in smooth settings. In this paper, we consider a family of minimax problems whose objective function enjoys the nonsmooth composite structure in the variable of minimization and is concave in the variables of maximization. By fully exploiting the composite structure, we propose a smoothed proximal linear descent ascent (\textit{smoothed} PLDA) algorithm and further establish its $\mathcal{O}(\epsilon^{-4})$ iteration complexity, which matches that of smoothed GDA~\cite{zhang2020single} under smooth settings. Moreover, under the mild assumption that the objective function satisfies the one-sided Kurdyka-\L{}ojasiewicz condition with exponent $\theta \in (0,1)$, we can further improve the iteration complexity to $\mathcal{O}(\epsilon^{-2\max\{2\theta,1\}})$. To the best of our knowledge, this is the first provably efficient algorithm for nonsmooth nonconvex-concave problems that can achieve the optimal iteration complexity $\mathcal{O}(\epsilon^{-2})$ if $\theta \in (0,1/2]$. As a byproduct, we discuss different stationarity concepts and clarify their relationships quantitatively, which could be of independent interest. Empirically, we illustrate the effectiveness of the proposed smoothed PLDA in variation regularized Wasserstein distributionally robust optimization problems.