Graph Pseudotime Analysis and Neural Stochastic Differential Equations for Analyzing Retinal Degeneration Dynamics and Beyond

Understanding disease progression at the molecular pathway level usually requires capturing both structural dependencies between pathways and the temporal dynamics of disease evolution. In this work, we solve the former challenge by developing a biologically informed graph-forming method to efficiently construct pathway graphs for subjects from our newly curated JR5558 mouse transcriptomics dataset. We then develop Graph-level Pseudotime Analysis (GPA) to infer graph-level trajectories that reveal how disease progresses at the population level, rather than in individual subjects. Based on the trajectories estimated by GPA, we identify the most sensitive pathways that drive disease stage transitions. In addition, we measure changes in pathway features using neural stochastic differential equations (SDEs), which enables us to formally define and compute pathway stability and disease bifurcation points (points of no return), two fundamental problems in disease progression research. We further extend our theory to the case when pathways can interact with each other, enabling a more comprehensive and multi-faceted characterization of disease phenotypes. The comprehensive experimental results demonstrate the effectiveness of our framework in reconstructing the dynamics of the pathway, identifying critical transitions, and providing novel insights into the mechanistic understanding of disease evolution.

When Graph Neural Networks Meet Dynamic Mode Decomposition

Graph Neural Networks (GNNs) have emerged as fundamental tools for a wide range of prediction tasks on graph-structured data. Recent studies have drawn analogies between GNN feature propagation and diffusion processes, which can be interpreted as dynamical systems. In this paper, we delve deeper into this perspective by connecting the dynamics in GNNs to modern Koopman theory and its numerical method, Dynamic Mode Decomposition (DMD). We illustrate how DMD can estimate a low-rank, finite-dimensional linear operator based on multiple states of the system, effectively approximating potential nonlinear interactions between nodes in the graph. This approach allows us to capture complex dynamics within the graph accurately and efficiently. We theoretically establish a connection between the DMD-estimated operator and the original dynamic operator between system states. Building upon this foundation, we introduce a family of DMD-GNN models that effectively leverage the low-rank eigenfunctions provided by the DMD algorithm. We further discuss the potential of enhancing our approach by incorporating domain-specific constraints such as symmetry into the DMD computation, allowing the corresponding GNN models to respect known physical properties of the underlying system. Our work paves the path for applying advanced dynamical system analysis tools via GNNs. We validate our approach through extensive experiments on various learning tasks, including directed graphs, large-scale graphs, long-range interactions, and spatial-temporal graphs. We also empirically verify that our proposed models can serve as powerful encoders for link prediction tasks. The results demonstrate that our DMD-enhanced GNNs achieve state-of-the-art performance, highlighting the effectiveness of integrating DMD into GNN frameworks.

Diffusing to the Top: Boost Graph Neural Networks with Minimal Hyperparameter Tuning

Graph Neural Networks (GNNs) are proficient in graph representation learning and achieve promising performance on versatile tasks such as node classification and link prediction. Usually, a comprehensive hyperparameter tuning is essential for fully unlocking GNN's top performance, especially for complicated tasks such as node classification on large graphs and long-range graphs. This is usually associated with high computational and time costs and careful design of appropriate search spaces. This work introduces a graph-conditioned latent diffusion framework (GNN-Diff) to generate high-performing GNNs based on the model checkpoints of sub-optimal hyperparameters selected by a light-tuning coarse search. We validate our method through 166 experiments across four graph tasks: node classification on small, large, and long-range graphs, as well as link prediction. Our experiments involve 10 classic and state-of-the-art target models and 20 publicly available datasets. The results consistently demonstrate that GNN-Diff: (1) boosts the performance of GNNs with efficient hyperparameter tuning; and (2) presents high stability and generalizability on unseen data across multiple generation runs. The code is available at https://github.com/lequanlin/GNN-Diff.

Unleash Graph Neural Networks from Heavy Tuning

Graph Neural Networks (GNNs) are deep-learning architectures designed for graph-type data, where understanding relationships among individual observations is crucial. However, achieving promising GNN performance, especially on unseen data, requires comprehensive hyperparameter tuning and meticulous training. Unfortunately, these processes come with high computational costs and significant human effort. Additionally, conventional searching algorithms such as grid search may result in overfitting on validation data, diminishing generalization accuracy. To tackle these challenges, we propose a graph conditional latent diffusion framework (GNN-Diff) to generate high-performing GNNs directly by learning from checkpoints saved during a light-tuning coarse search. Our method: (1) unleashes GNN training from heavy tuning and complex search space design; (2) produces GNN parameters that outperform those obtained through comprehensive grid search; and (3) establishes higher-quality generation for GNNs compared to diffusion frameworks designed for general neural networks.

Design Your Own Universe: A Physics-Informed Agnostic Method for Enhancing Graph Neural Networks

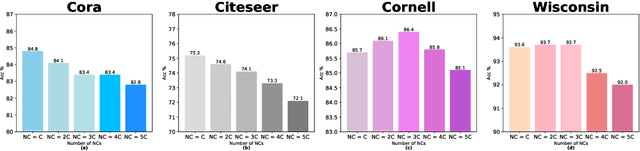
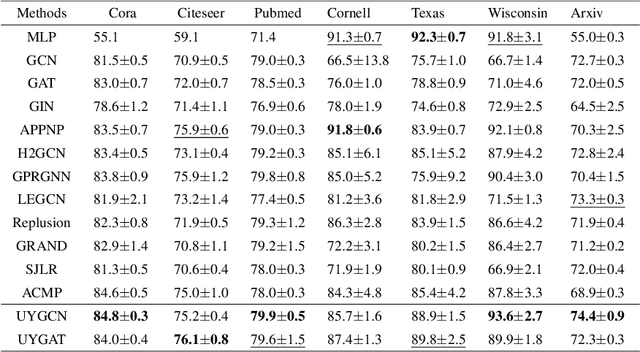
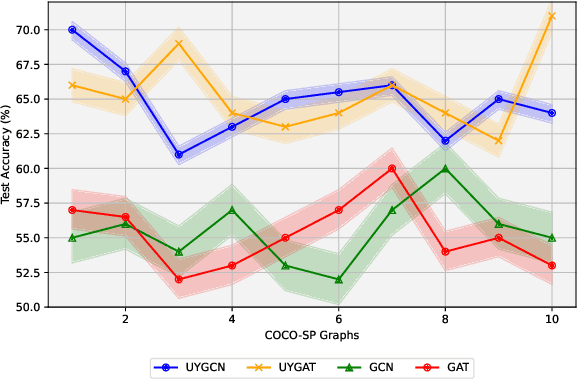
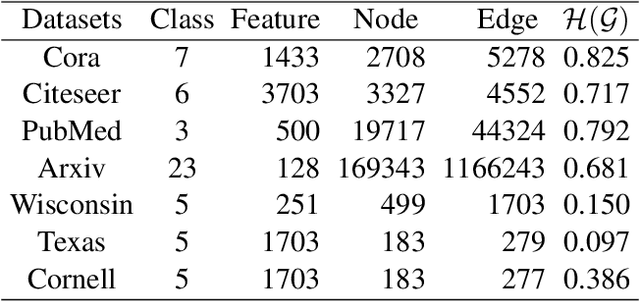
Physics-informed Graph Neural Networks have achieved remarkable performance in learning through graph-structured data by mitigating common GNN challenges such as over-smoothing, over-squashing, and heterophily adaption. Despite these advancements, the development of a simple yet effective paradigm that appropriately integrates previous methods for handling all these challenges is still underway. In this paper, we draw an analogy between the propagation of GNNs and particle systems in physics, proposing a model-agnostic enhancement framework. This framework enriches the graph structure by introducing additional nodes and rewiring connections with both positive and negative weights, guided by node labeling information. We theoretically verify that GNNs enhanced through our approach can effectively circumvent the over-smoothing issue and exhibit robustness against over-squashing. Moreover, we conduct a spectral analysis on the rewired graph to demonstrate that the corresponding GNNs can fit both homophilic and heterophilic graphs. Empirical validations on benchmarks for homophilic, heterophilic graphs, and long-term graph datasets show that GNNs enhanced by our method significantly outperform their original counterparts.

SpecSTG: A Fast Spectral Diffusion Framework for Probabilistic Spatio-Temporal Traffic Forecasting

Traffic forecasting, a crucial application of spatio-temporal graph (STG) learning, has traditionally relied on deterministic models for accurate point estimations. Yet, these models fall short of identifying latent risks of unexpected volatility in future observations. To address this gap, probabilistic methods, especially variants of diffusion models, have emerged as uncertainty-aware solutions. However, existing diffusion methods typically focus on generating separate future time series for individual sensors in the traffic network, resulting in insufficient involvement of spatial network characteristics in the probabilistic learning process. To better leverage spatial dependencies and systematic patterns inherent in traffic data, we propose SpecSTG, a novel spectral diffusion framework. Our method generates the Fourier representation of future time series, transforming the learning process into the spectral domain enriched with spatial information. Additionally, our approach incorporates a fast spectral graph convolution designed for Fourier input, alleviating the computational burden associated with existing models. Numerical experiments show that SpecSTG achieves outstanding performance with traffic flow and traffic speed datasets compared to state-of-the-art baselines. The source code for SpecSTG is available at https://anonymous.4open.science/r/SpecSTG.

Exposition on over-squashing problem on GNNs: Current Methods, Benchmarks and Challenges

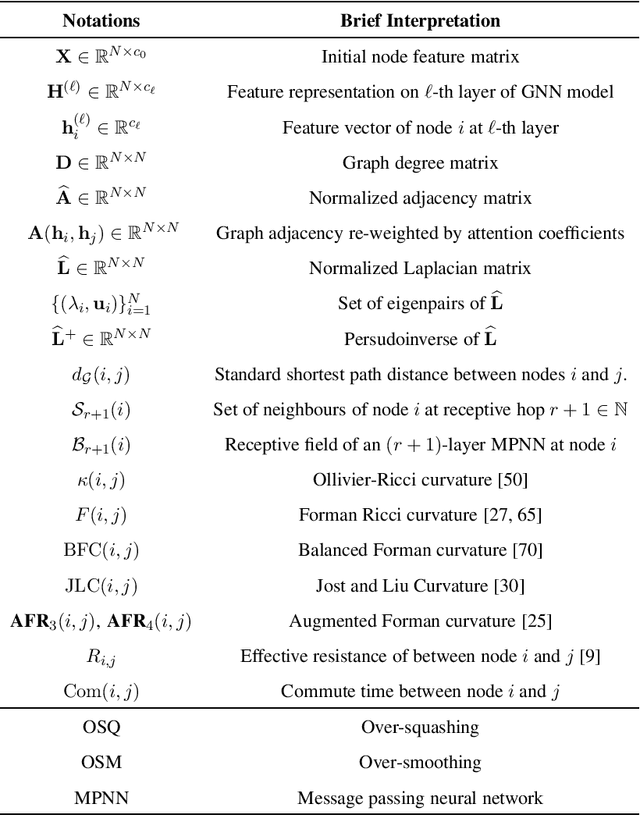
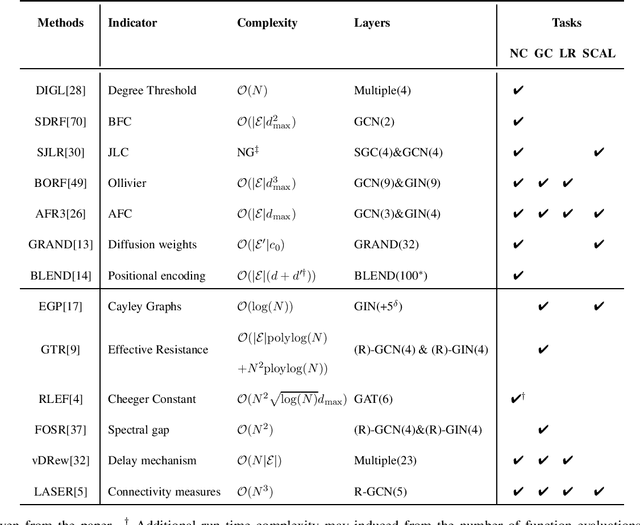
Graph-based message-passing neural networks (MPNNs) have achieved remarkable success in both node and graph-level learning tasks. However, several identified problems, including over-smoothing (OSM), limited expressive power, and over-squashing (OSQ), still limit the performance of MPNNs. In particular, OSQ serves as the latest identified problem, where MPNNs gradually lose their learning accuracy when long-range dependencies between graph nodes are required. In this work, we provide an exposition on the OSQ problem by summarizing different formulations of OSQ from current literature, as well as the three different categories of approaches for addressing the OSQ problem. In addition, we also discuss the alignment between OSQ and expressive power and the trade-off between OSQ and OSM. Furthermore, we summarize the empirical methods leveraged from existing works to verify the efficiency of OSQ mitigation approaches, with illustrations of their computational complexities. Lastly, we list some open questions that are of interest for further exploration of the OSQ problem along with potential directions from the best of our knowledge.

From Continuous Dynamics to Graph Neural Networks: Neural Diffusion and Beyond

Graph neural networks (GNNs) have demonstrated significant promise in modelling relational data and have been widely applied in various fields of interest. The key mechanism behind GNNs is the so-called message passing where information is being iteratively aggregated to central nodes from their neighbourhood. Such a scheme has been found to be intrinsically linked to a physical process known as heat diffusion, where the propagation of GNNs naturally corresponds to the evolution of heat density. Analogizing the process of message passing to the heat dynamics allows to fundamentally understand the power and pitfalls of GNNs and consequently informs better model design. Recently, there emerges a plethora of works that proposes GNNs inspired from the continuous dynamics formulation, in an attempt to mitigate the known limitations of GNNs, such as oversmoothing and oversquashing. In this survey, we provide the first systematic and comprehensive review of studies that leverage the continuous perspective of GNNs. To this end, we introduce foundational ingredients for adapting continuous dynamics to GNNs, along with a general framework for the design of graph neural dynamics. We then review and categorize existing works based on their driven mechanisms and underlying dynamics. We also summarize how the limitations of classic GNNs can be addressed under the continuous framework. We conclude by identifying multiple open research directions.

Bregman Graph Neural Network

Numerous recent research on graph neural networks (GNNs) has focused on formulating GNN architectures as an optimization problem with the smoothness assumption. However, in node classification tasks, the smoothing effect induced by GNNs tends to assimilate representations and over-homogenize labels of connected nodes, leading to adverse effects such as over-smoothing and misclassification. In this paper, we propose a novel bilevel optimization framework for GNNs inspired by the notion of Bregman distance. We demonstrate that the GNN layer proposed accordingly can effectively mitigate the over-smoothing issue by introducing a mechanism reminiscent of the "skip connection". We validate our theoretical results through comprehensive empirical studies in which Bregman-enhanced GNNs outperform their original counterparts in both homophilic and heterophilic graphs. Furthermore, our experiments also show that Bregman GNNs can produce more robust learning accuracy even when the number of layers is high, suggesting the effectiveness of the proposed method in alleviating the over-smoothing issue.

Diffusion Models for Time Series Applications: A Survey

Diffusion models, a family of generative models based on deep learning, have become increasingly prominent in cutting-edge machine learning research. With a distinguished performance in generating samples that resemble the observed data, diffusion models are widely used in image, video, and text synthesis nowadays. In recent years, the concept of diffusion has been extended to time series applications, and many powerful models have been developed. Considering the deficiency of a methodical summary and discourse on these models, we provide this survey as an elementary resource for new researchers in this area and also an inspiration to motivate future research. For better understanding, we include an introduction about the basics of diffusion models. Except for this, we primarily focus on diffusion-based methods for time series forecasting, imputation, and generation, and present them respectively in three individual sections. We also compare different methods for the same application and highlight their connections if applicable. Lastly, we conclude the common limitation of diffusion-based methods and highlight potential future research directions.