Accelerating Multi-Task Temporal Difference Learning under Low-Rank Representation

We study policy evaluation problems in multi-task reinforcement learning (RL) under a low-rank representation setting. In this setting, we are given $N$ learning tasks where the corresponding value function of these tasks lie in an $r$-dimensional subspace, with $r<N$. One can apply the classic temporal-difference (TD) learning method for solving these problems where this method learns the value function of each task independently. In this paper, we are interested in understanding whether one can exploit the low-rank structure of the multi-task setting to accelerate the performance of TD learning. To answer this question, we propose a new variant of TD learning method, where we integrate the so-called truncated singular value decomposition step into the update of TD learning. This additional step will enable TD learning to exploit the dominant directions due to the low rank structure to update the iterates, therefore, improving its performance. Our empirical results show that the proposed method significantly outperforms the classic TD learning, where the performance gap increases as the rank $r$ decreases. From the theoretical point of view, introducing the truncated singular value decomposition step into TD learning might cause an instability on the updates. We provide a theoretical result showing that the instability does not happen. Specifically, we prove that the proposed method converges at a rate $\mathcal{O}(\frac{\ln(t)}{t})$, where $t$ is the number of iterations. This rate matches that of the standard TD learning.

Rapid Grassmannian Averaging with Chebyshev Polynomials

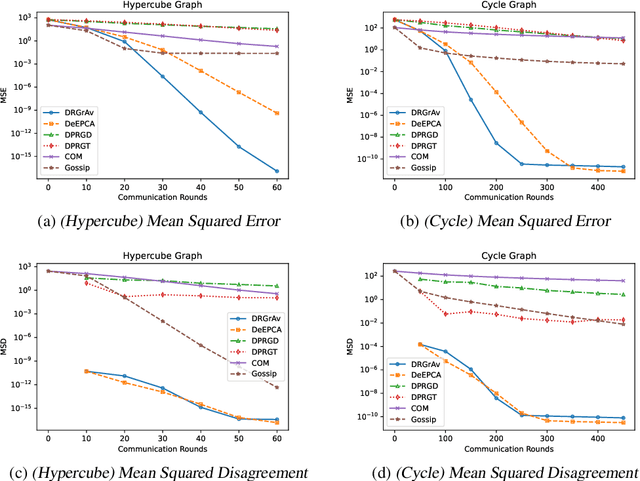
We propose new algorithms to efficiently average a collection of points on a Grassmannian manifold in both the centralized and decentralized settings. Grassmannian points are used ubiquitously in machine learning, computer vision, and signal processing to represent data through (often low-dimensional) subspaces. While averaging these points is crucial to many tasks (especially in the decentralized setting), existing methods unfortunately remain computationally expensive due to the non-Euclidean geometry of the manifold. Our proposed algorithms, Rapid Grassmannian Averaging (RGrAv) and Decentralized Rapid Grassmannian Averaging (DRGrAv), overcome this challenge by leveraging the spectral structure of the problem to rapidly compute an average using only small matrix multiplications and QR factorizations. We provide a theoretical guarantee of optimality and present numerical experiments which demonstrate that our algorithms outperform state-of-the-art methods in providing high accuracy solutions in minimal time. Additional experiments showcase the versatility of our algorithms to tasks such as K-means clustering on video motion data, establishing RGrAv and DRGrAv as powerful tools for generic Grassmannian averaging.

Robust Broadband Beamforming using Bilinear Programming

We introduce a new method for robust beamforming, where the goal is to estimate a signal from array samples when there is uncertainty in the angle of arrival. Our method offers state-of-the-art performance on narrowband signals and is naturally applied to broadband signals. Our beamformer operates by treating the forward model for the array samples as unknown. We show that the "true" forward model lies in the linear span of a small number of fixed linear systems. As a result, we can estimate the forward operator and the signal simultaneously by solving a bilinear inverse problem using least squares. Our numerical experiments show that if the angle of arrival is known to only be within an interval of reasonable size, there is very little loss in estimation performance compared to the case where the angle is known exactly.

Real-time Digital RF Emulation -- II: A Near Memory Custom Accelerator

A near memory hardware accelerator, based on a novel direct path computational model, for real-time emulation of radio frequency systems is demonstrated. Our evaluation of hardware performance uses both application-specific integrated circuits (ASIC) and field programmable gate arrays (FPGA) methodologies: 1). The ASIC testchip implementation, using TSMC 28nm CMOS, leverages distributed autonomous control to extract concurrency in compute as well as low latency. It achieves a $518$ MHz per channel bandwidth in a prototype $4$-node system. The maximum emulation range supported in this paradigm is $9.5$ km with $0.24$ $\mu$s of per-sample emulation latency. 2). The FPGA-based implementation, evaluated on a Xilinx ZCU104 board, demonstrates a $9$-node test case (two Transmitters, one Receiver, and $6$ passive reflectors) with an emulation range of $1.13$ km to $27.3$ km at $215$ MHz bandwidth.

Real-time Digital RF Emulation -- I: The Direct Path Computational Model

In this paper we consider the problem of developing a computational model for emulating an RF channel. The motivation for this is that an accurate and scalable emulator has the potential to minimize the need for field testing, which is expensive, slow, and difficult to replicate. Traditionally, emulators are built using a tapped delay line model where long filters modeling the physical interactions of objects are implemented directly. For an emulation scenario consisting of $M$ objects all interacting with one another, the tapped delay line model's computational requirements scale as $O(M^3)$ per sample: there are $O(M^2)$ channels, each with $O(M)$ complexity. In this paper, we develop a new ``direct path" model that, while remaining physically faithful, allows us to carefully factor the emulator operations, resulting in an $O(M^2)$ per sample scaling of the computational requirements. The impact of this is drastic, a $200$ object scenario sees about a $100\times$ reduction in the number of per sample computations. Furthermore, the direct path model gives us a natural way to distribute the computations for an emulation: each object is mapped to a computational node, and these nodes are networked in a fully connected communication graph. Alongside a discussion of the model and the physical phenomena it emulates, we show how to efficiently parameterize antenna responses and scattering profiles within this direct path framework. To verify the model and demonstrate its viability in hardware, we provide several numerical experiments produced using a cycle level C++ simulator of a hardware implementation of the model.

Precise asymptotics of reweighted least-squares algorithms for linear diagonal networks

The classical iteratively reweighted least-squares (IRLS) algorithm aims to recover an unknown signal from linear measurements by performing a sequence of weighted least squares problems, where the weights are recursively updated at each step. Varieties of this algorithm have been shown to achieve favorable empirical performance and theoretical guarantees for sparse recovery and $\ell_p$-norm minimization. Recently, some preliminary connections have also been made between IRLS and certain types of non-convex linear neural network architectures that are observed to exploit low-dimensional structure in high-dimensional linear models. In this work, we provide a unified asymptotic analysis for a family of algorithms that encompasses IRLS, the recently proposed lin-RFM algorithm (which was motivated by feature learning in neural networks), and the alternating minimization algorithm on linear diagonal neural networks. Our analysis operates in a "batched" setting with i.i.d. Gaussian covariates and shows that, with appropriately chosen reweighting policy, the algorithm can achieve favorable performance in only a handful of iterations. We also extend our results to the case of group-sparse recovery and show that leveraging this structure in the reweighting scheme provably improves test error compared to coordinate-wise reweighting.

Natural Policy Gradient and Actor Critic Methods for Constrained Multi-Task Reinforcement Learning

Multi-task reinforcement learning (RL) aims to find a single policy that effectively solves multiple tasks at the same time. This paper presents a constrained formulation for multi-task RL where the goal is to maximize the average performance of the policy across tasks subject to bounds on the performance in each task. We consider solving this problem both in the centralized setting, where information for all tasks is accessible to a single server, and in the decentralized setting, where a network of agents, each given one task and observing local information, cooperate to find the solution of the globally constrained objective using local communication. We first propose a primal-dual algorithm that provably converges to the globally optimal solution of this constrained formulation under exact gradient evaluations. When the gradient is unknown, we further develop a sampled-based actor-critic algorithm that finds the optimal policy using online samples of state, action, and reward. Finally, we study the extension of the algorithm to the linear function approximation setting.

Subspace Tracking with Dynamical Models on the Grassmannian

Tracking signals in dynamic environments presents difficulties in both analysis and implementation. In this work, we expand on a class of subspace tracking algorithms which utilize the Grassmann manifold -- the set of linear subspaces of a high-dimensional vector space. We design regularized least squares algorithms based on common manifold operations and intuitive dynamical models. We demonstrate the efficacy of the approach for a narrowband beamforming scenario, where the dynamics of multiple signals of interest are captured by motion on the Grassmannian.

Slepian Beamforming: Broadband Beamforming using Streaming Least Squares

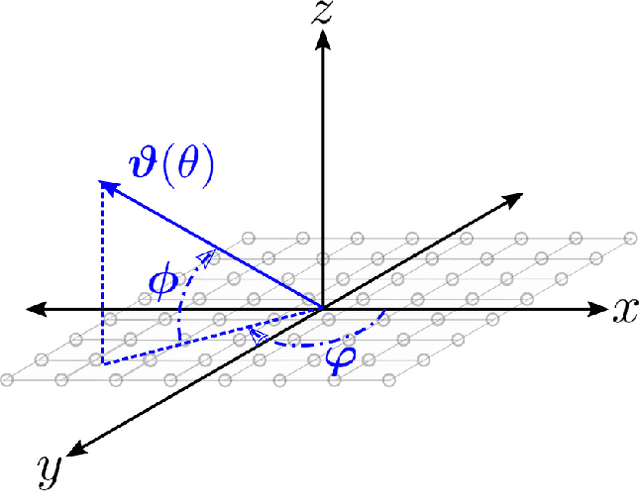
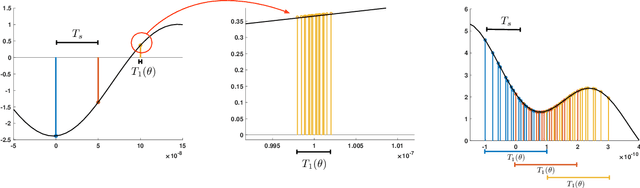
In this paper we revisit the classical problem of estimating a signal as it impinges on a multi-sensor array. We focus on the case where the impinging signal's bandwidth is appreciable and is operating in a broadband regime. Estimating broadband signals, often termed broadband (or wideband) beamforming, is traditionally done through filter and summation, true time delay, or a coupling of the two. Our proposed method deviates substantially from these paradigms in that it requires no notion of filtering or true time delay. We use blocks of samples taken directly from the sensor outputs to fit a robust Slepian subspace model using a least squares approach. We then leverage this model to estimate uniformly spaced samples of the impinging signal. Alongside a careful discussion of this model and how to choose its parameters we show how to fit the model to new blocks of samples as they are received, producing a streaming output. We then go on to show how this method naturally extends to adaptive beamforming scenarios, where we leverage signal statistics to attenuate interfering sources. Finally, we discuss how to use our model to estimate from dimensionality reducing measurements. Accompanying these discussions are extensive numerical experiments establishing that our method outperforms existing filter based approaches while being comparable in terms of computational complexity.

Level set methods for gradient-free optimization of metasurface arrays

Global optimization techniques are increasingly preferred over human-driven methods in the design of electromagnetic structures such as metasurfaces, and careful construction and parameterization of the physical structure is critical in ensuring computational efficiency and convergence of the optimization algorithm to a globally optimal solution. While many design variables in physical systems take discrete values, optimization algorithms often benefit from a continuous design space. This work demonstrates the use of level set functions as a continuous basis for designing material distributions for metasurface arrays and introduces an improved parameterization which is termed the periodic level set function. We explore the use of alternate norms in the definition of the level set function and define a new pseudo-inverse technique for upsampling basis coefficients with these norms. The level set method is compared to the fragmented parameterization and shows improved electromagnetic responses for two dissimilar cost functions: a narrowband objective and a broadband objective. Finally, we manufacture an optimized level set metasurface and measure its scattering parameters to demonstrate real-world performance.