Ilsang Ohn
Nonparametric estimation of a factorizable density using diffusion models
Jan 03, 2025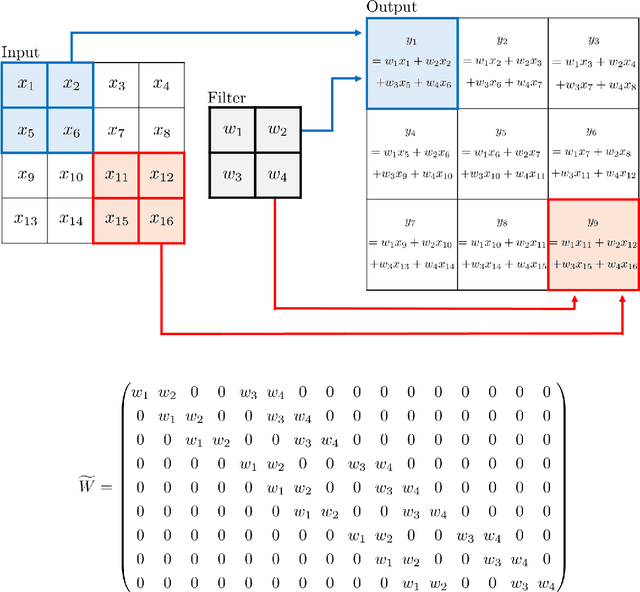
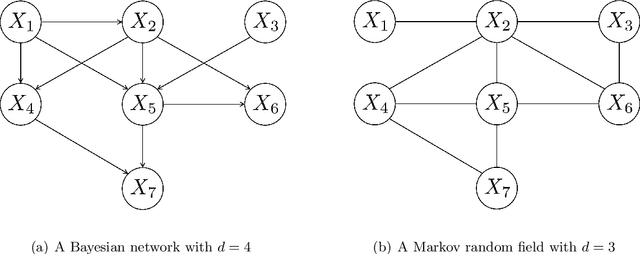
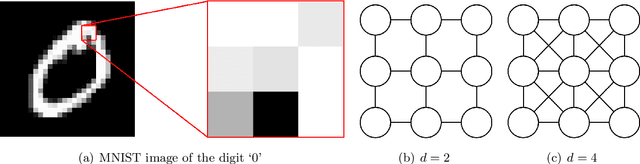
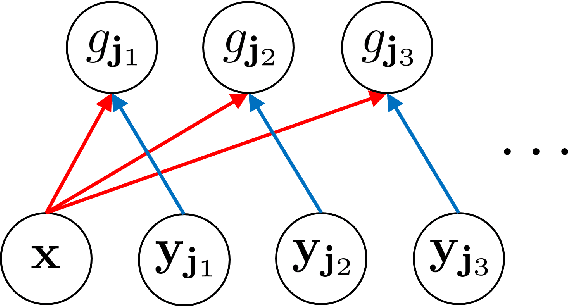
Abstract:In recent years, diffusion models, and more generally score-based deep generative models, have achieved remarkable success in various applications, including image and audio generation. In this paper, we view diffusion models as an implicit approach to nonparametric density estimation and study them within a statistical framework to analyze their surprising performance. A key challenge in high-dimensional statistical inference is leveraging low-dimensional structures inherent in the data to mitigate the curse of dimensionality. We assume that the underlying density exhibits a low-dimensional structure by factorizing into low-dimensional components, a property common in examples such as Bayesian networks and Markov random fields. Under suitable assumptions, we demonstrate that an implicit density estimator constructed from diffusion models adapts to the factorization structure and achieves the minimax optimal rate with respect to the total variation distance. In constructing the estimator, we design a sparse weight-sharing neural network architecture, where sparsity and weight-sharing are key features of practical architectures such as convolutional neural networks and recurrent neural networks.
A Bayesian sparse factor model with adaptive posterior concentration
May 29, 2023Abstract:In this paper, we propose a new Bayesian inference method for a high-dimensional sparse factor model that allows both the factor dimensionality and the sparse structure of the loading matrix to be inferred. The novelty is to introduce a certain dependence between the sparsity level and the factor dimensionality, which leads to adaptive posterior concentration while keeping computational tractability. We show that the posterior distribution asymptotically concentrates on the true factor dimensionality, and more importantly, this posterior consistency is adaptive to the sparsity level of the true loading matrix and the noise variance. We also prove that the proposed Bayesian model attains the optimal detection rate of the factor dimensionality in a more general situation than those found in the literature. Moreover, we obtain a near-optimal posterior concentration rate of the covariance matrix. Numerical studies are conducted and show the superiority of the proposed method compared with other competitors.
Masked Bayesian Neural Networks : Theoretical Guarantee and its Posterior Inference
May 24, 2023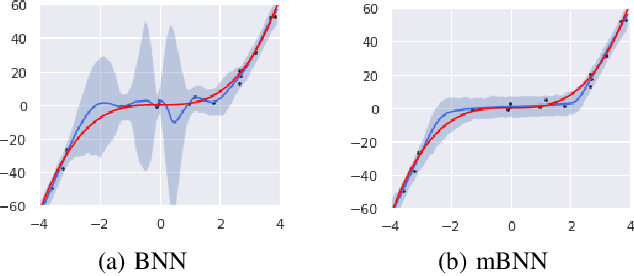
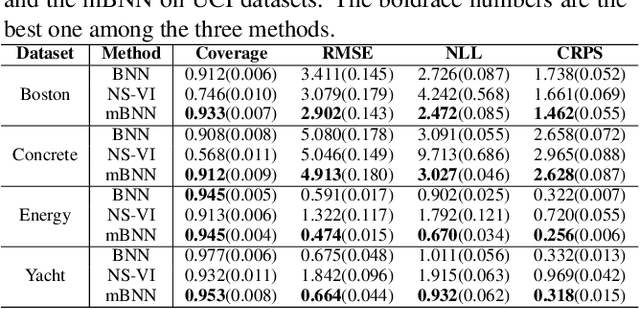
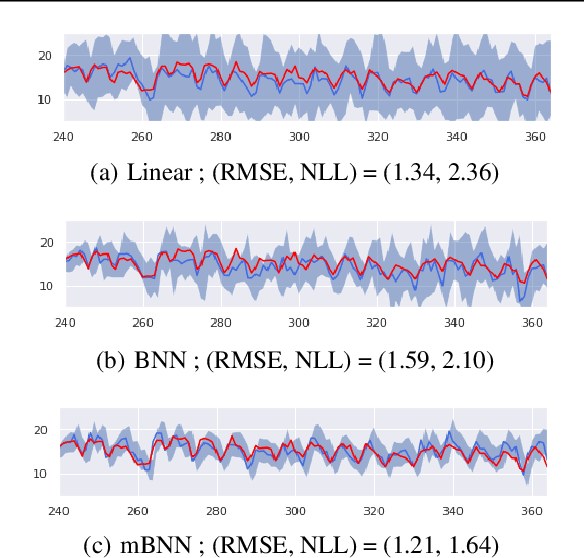
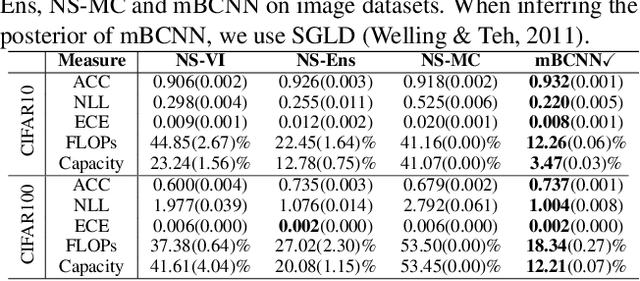
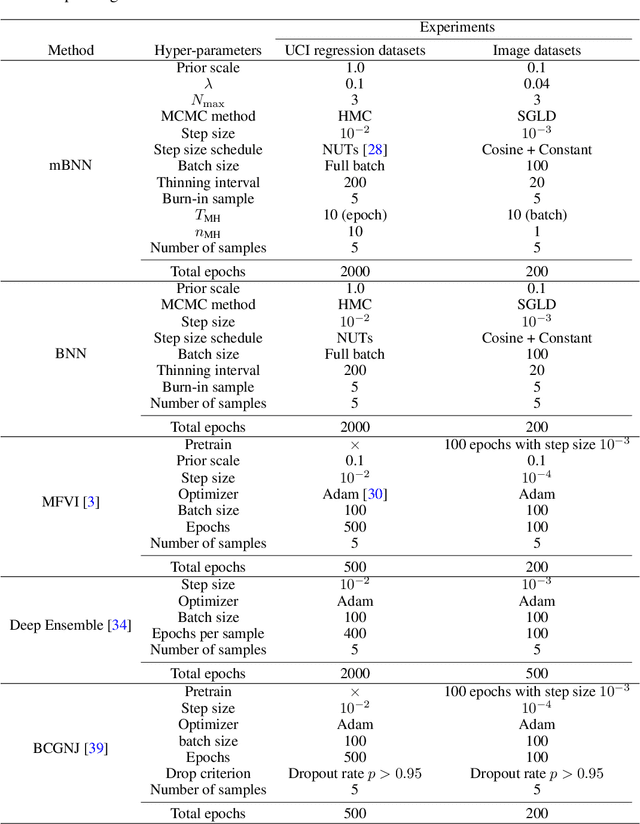
Abstract:Bayesian approaches for learning deep neural networks (BNN) have been received much attention and successfully applied to various applications. Particularly, BNNs have the merit of having better generalization ability as well as better uncertainty quantification. For the success of BNN, search an appropriate architecture of the neural networks is an important task, and various algorithms to find good sparse neural networks have been proposed. In this paper, we propose a new node-sparse BNN model which has good theoretical properties and is computationally feasible. We prove that the posterior concentration rate to the true model is near minimax optimal and adaptive to the smoothness of the true model. In particular the adaptiveness is the first of its kind for node-sparse BNNs. In addition, we develop a novel MCMC algorithm which makes the Bayesian inference of the node-sparse BNN model feasible in practice.
Intrinsic and extrinsic deep learning on manifolds
Feb 16, 2023Abstract:We propose extrinsic and intrinsic deep neural network architectures as general frameworks for deep learning on manifolds. Specifically, extrinsic deep neural networks (eDNNs) preserve geometric features on manifolds by utilizing an equivariant embedding from the manifold to its image in the Euclidean space. Moreover, intrinsic deep neural networks (iDNNs) incorporate the underlying intrinsic geometry of manifolds via exponential and log maps with respect to a Riemannian structure. Consequently, we prove that the empirical risk of the empirical risk minimizers (ERM) of eDNNs and iDNNs converge in optimal rates. Overall, The eDNNs framework is simple and easy to compute, while the iDNNs framework is accurate and fast converging. To demonstrate the utilities of our framework, various simulation studies, and real data analyses are presented with eDNNs and iDNNs.
The convergent Indian buffet process
Jun 16, 2022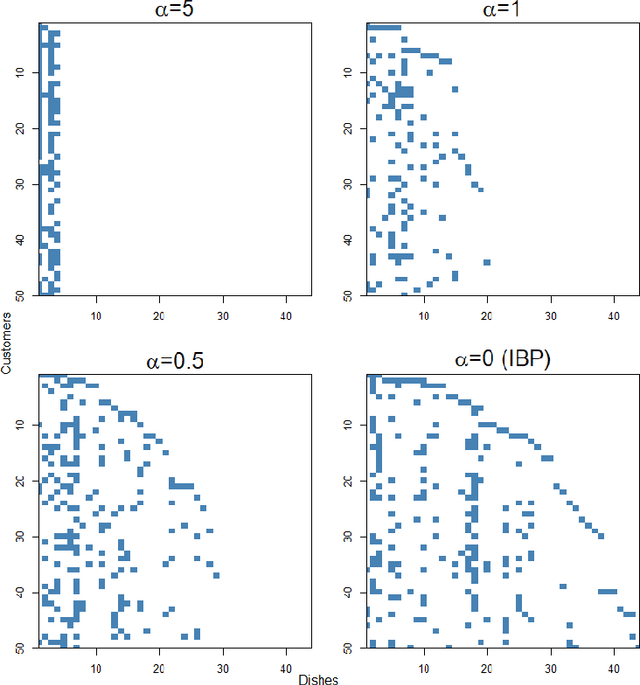
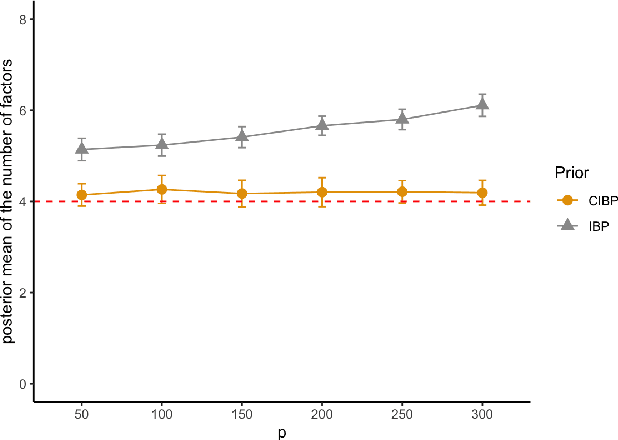
Abstract:We propose a new Bayesian nonparametric prior for latent feature models, which we call the convergent Indian buffet process (CIBP). We show that under the CIBP, the number of latent features is distributed as a Poisson distribution with the mean monotonically increasing but converging to a certain value as the number of objects goes to infinity. That is, the expected number of features is bounded above even when the number of objects goes to infinity, unlike the standard Indian buffet process under which the expected number of features increases with the number of objects. We provide two alternative representations of the CIBP based on a hierarchical distribution and a completely random measure, respectively, which are of independent interest. The proposed CIBP is assessed on a high-dimensional sparse factor model.
Masked Bayesian Neural Networks : Computation and Optimality
Jun 02, 2022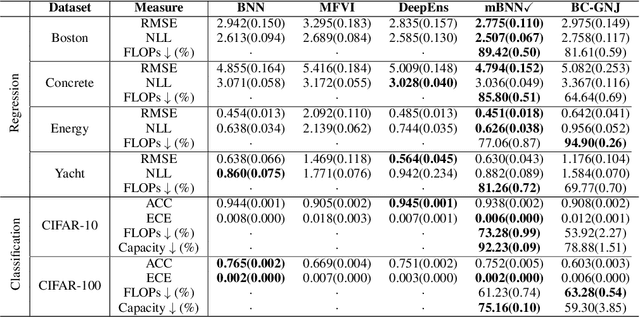
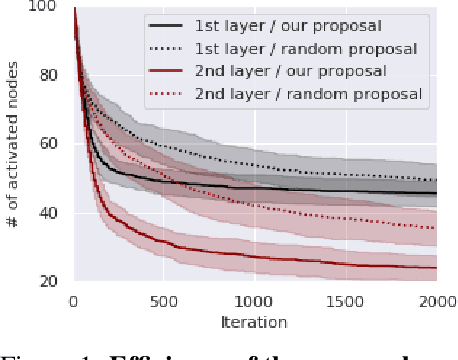
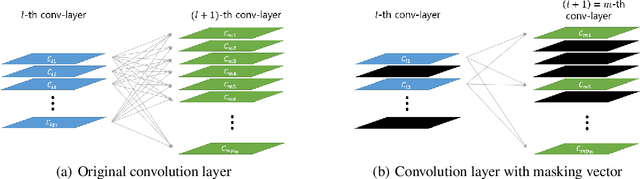
Abstract:As data size and computing power increase, the architectures of deep neural networks (DNNs) have been getting more complex and huge, and thus there is a growing need to simplify such complex and huge DNNs. In this paper, we propose a novel sparse Bayesian neural network (BNN) which searches a good DNN with an appropriate complexity. We employ the masking variables at each node which can turn off some nodes according to the posterior distribution to yield a nodewise sparse DNN. We devise a prior distribution such that the posterior distribution has theoretical optimalities (i.e. minimax optimality and adaptiveness), and develop an efficient MCMC algorithm. By analyzing several benchmark datasets, we illustrate that the proposed BNN performs well compared to other existing methods in the sense that it discovers well condensed DNN architectures with similar prediction accuracy and uncertainty quantification compared to large DNNs.
Learning fair representation with a parametric integral probability metric
Feb 17, 2022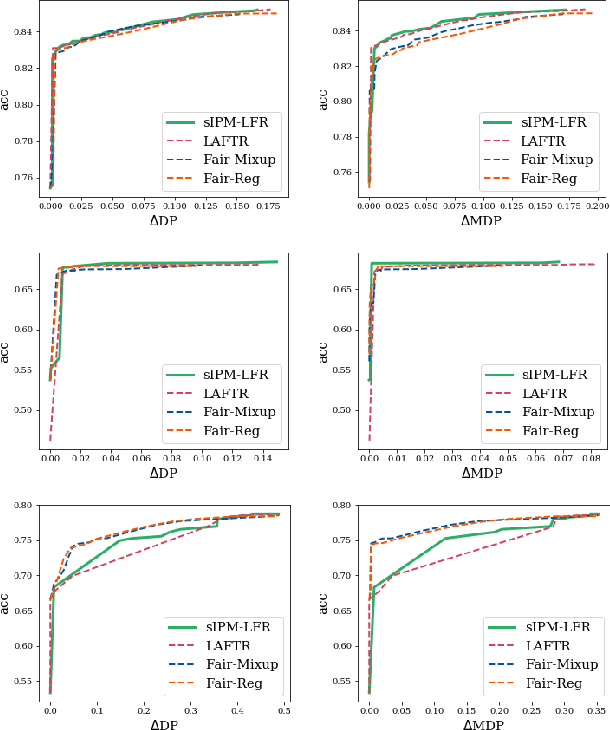
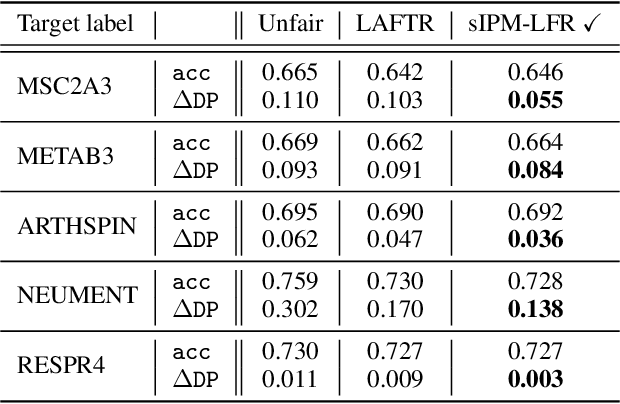
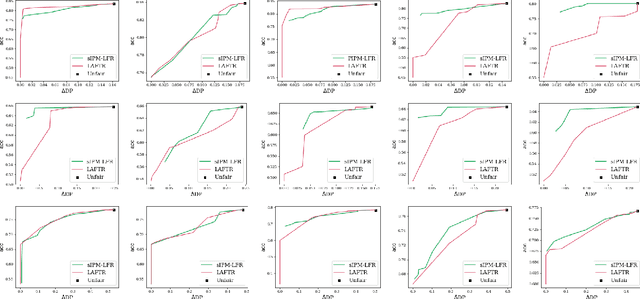
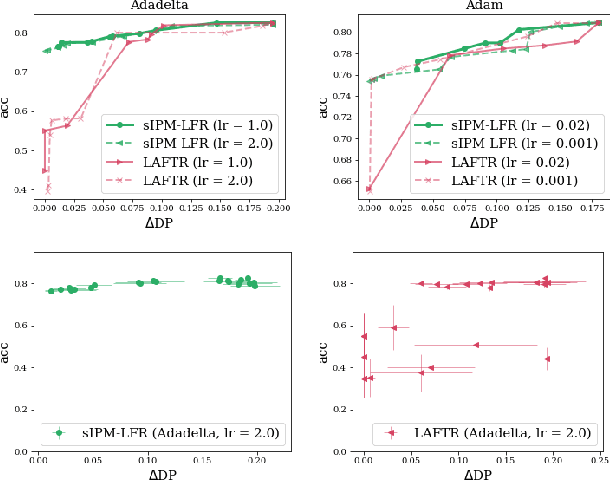
Abstract:As they have a vital effect on social decision-making, AI algorithms should be not only accurate but also fair. Among various algorithms for fairness AI, learning fair representation (LFR), whose goal is to find a fair representation with respect to sensitive variables such as gender and race, has received much attention. For LFR, the adversarial training scheme is popularly employed as is done in the generative adversarial network type algorithms. The choice of a discriminator, however, is done heuristically without justification. In this paper, we propose a new adversarial training scheme for LFR, where the integral probability metric (IPM) with a specific parametric family of discriminators is used. The most notable result of the proposed LFR algorithm is its theoretical guarantee about the fairness of the final prediction model, which has not been considered yet. That is, we derive theoretical relations between the fairness of representation and the fairness of the prediction model built on the top of the representation (i.e., using the representation as the input). Moreover, by numerical experiments, we show that our proposed LFR algorithm is computationally lighter and more stable, and the final prediction model is competitive or superior to other LFR algorithms using more complex discriminators.
SLIDE: a surrogate fairness constraint to ensure fairness consistency
Feb 07, 2022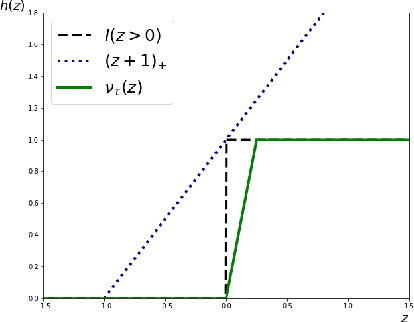
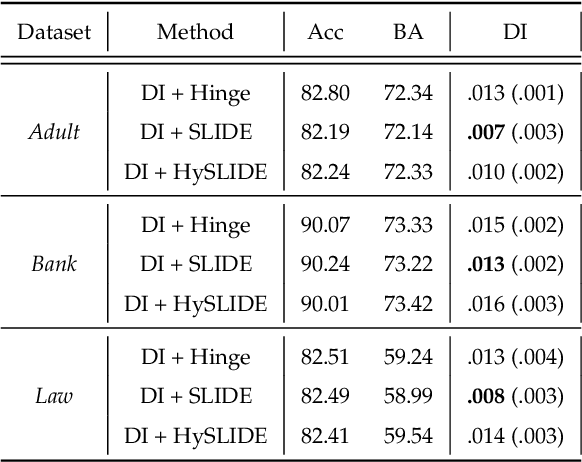
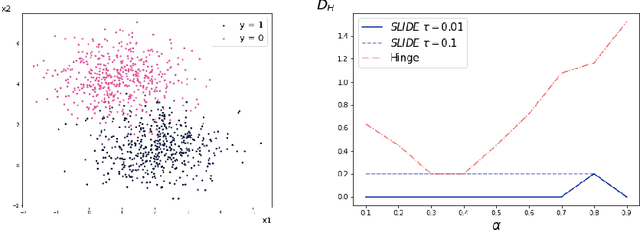
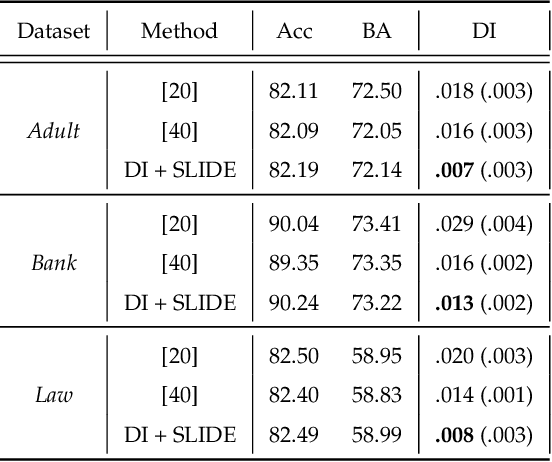
Abstract:As they have a vital effect on social decision makings, AI algorithms should be not only accurate and but also fair. Among various algorithms for fairness AI, learning a prediction model by minimizing the empirical risk (e.g., cross-entropy) subject to a given fairness constraint has received much attention. To avoid computational difficulty, however, a given fairness constraint is replaced by a surrogate fairness constraint as the 0-1 loss is replaced by a convex surrogate loss for classification problems. In this paper, we investigate the validity of existing surrogate fairness constraints and propose a new surrogate fairness constraint called SLIDE, which is computationally feasible and asymptotically valid in the sense that the learned model satisfies the fairness constraint asymptotically and achieves a fast convergence rate. Numerical experiments confirm that the SLIDE works well for various benchmark datasets.
Adaptive variational Bayes: Optimality, computation and applications
Sep 07, 2021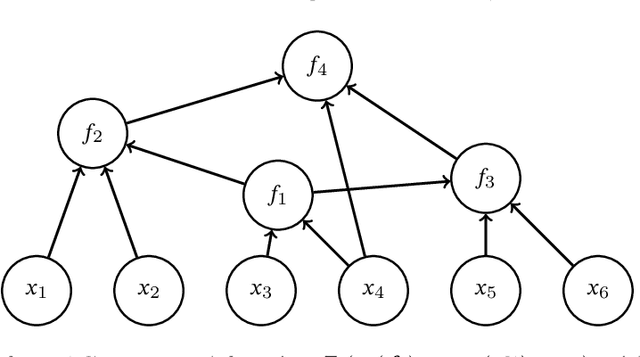
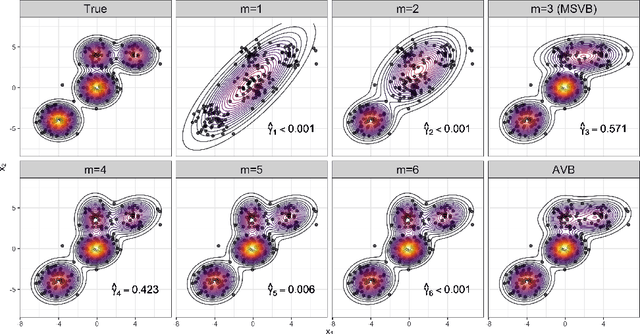
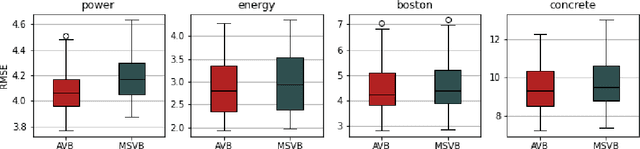
Abstract:In this paper, we explore adaptive inference based on variational Bayes. Although a number of studies have been conducted to analyze contraction properties of variational posteriors, there is still a lack of a general and computationally tractable variational Bayes method that can achieve adaptive optimal contraction of the variational posterior. We propose a novel variational Bayes framework, called adaptive variational Bayes, which can operate on a collection of models with varying dimensions and structures. The proposed framework combines variational posteriors over individual models with certain weights to obtain a variational posterior over the entire model. It turns out that this combined variational posterior minimizes the Kullback-Leibler divergence to the original posterior distribution. We show that the proposed variational posterior achieves optimal contraction rates adaptively under very general conditions and attains model selection consistency when the true model structure exists. We apply the general results obtained for the adaptive variational Bayes to several examples including deep learning models and derive some new and adaptive inference results. Moreover, we consider the use of quasi-likelihood in our framework. We formulate conditions on the quasi-likelihood to ensure the adaptive optimality and discuss specific applications to stochastic block models and nonparametric regression with sub-Gaussian errors.
Nonconvex sparse regularization for deep neural networks and its optimality
Mar 26, 2020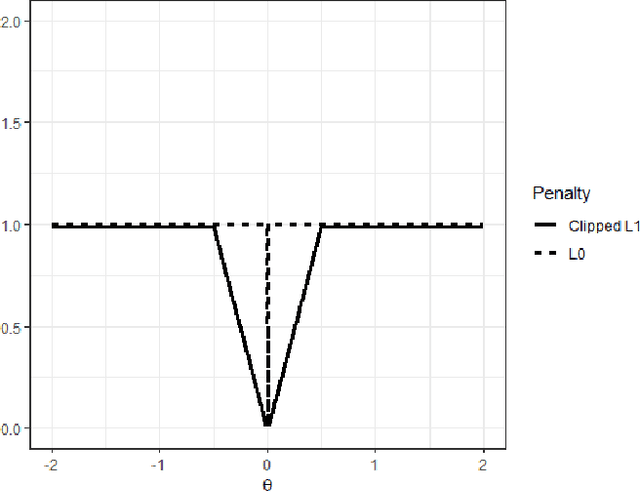

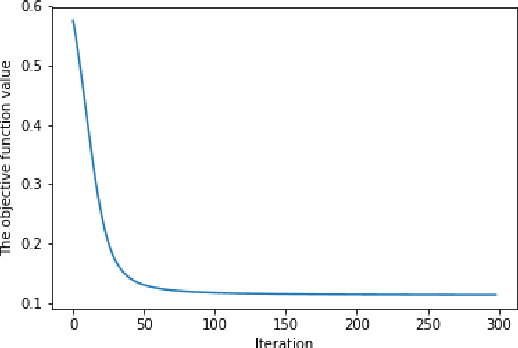
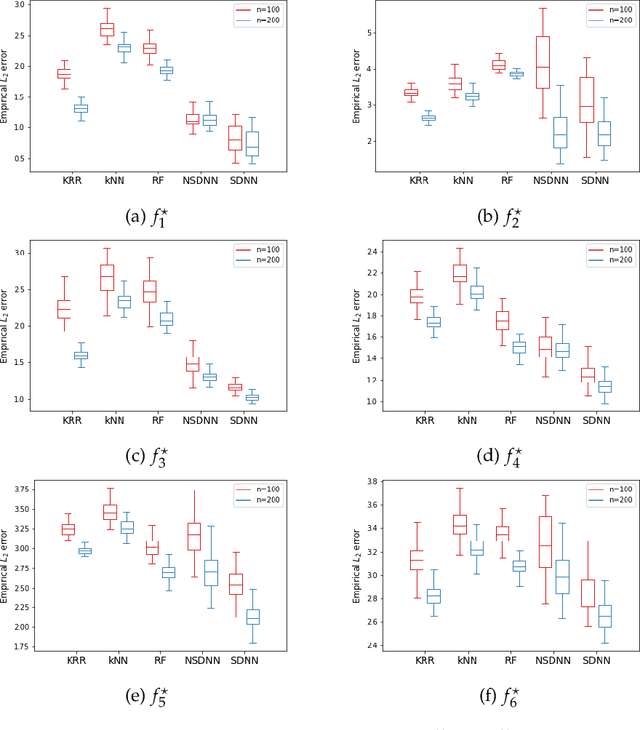
Abstract:Recent theoretical studies proved that deep neural network (DNN) estimators obtained by minimizing empirical risk with a certain sparsity constraint can attain optimal convergence rates for regression and classification problems. However, the sparsity constraint requires to know certain properties of the true model, which are not available in practice. Moreover, computation is difficult due to the discrete nature of the sparsity constraint. In this paper, we propose a novel penalized estimation method for sparse DNNs, which resolves the aforementioned problems existing in the sparsity constraint. We establish an oracle inequality for the excess risk of the proposed sparse-penalized DNN estimator and derive convergence rates for several learning tasks. In particular, we prove that the sparse-penalized estimator can adaptively attain minimax convergence rates for various nonparametric regression problems. For computation, we develop an efficient gradient-based optimization algorithm that guarantees the monotonic reduction of the objective function.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge