Dominik Peters
Proportional Aggregation of Preferences for Sequential Decision Making
Jun 26, 2023
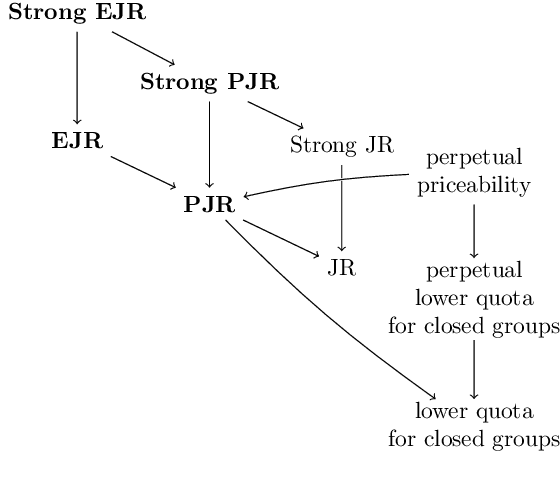
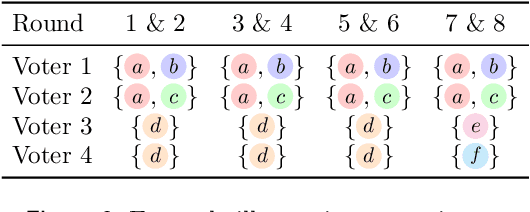
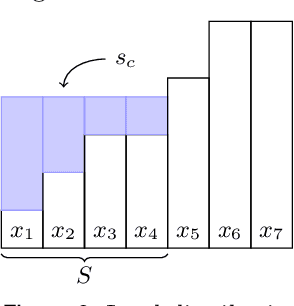
Abstract:We study the problem of fair sequential decision making given voter preferences. In each round, a decision rule must choose a decision from a set of alternatives where each voter reports which of these alternatives they approve. Instead of going with the most popular choice in each round, we aim for proportional representation. We formalize this aim using axioms based on Proportional Justified Representation (PJR), which were proposed in the literature on multi-winner voting and were recently adapted to multi-issue decision making. The axioms require that every group of $\alpha\%$ of the voters, if it agrees in every round (i.e., approves a common alternative), then those voters must approve at least $\alpha\%$ of the decisions. A stronger version of the axioms requires that every group of $\alpha\%$ of the voters that agrees in a $\beta$ fraction of rounds must approve $\beta\cdot\alpha\%$ of the decisions. We show that three attractive voting rules satisfy axioms of this style. One of them (Sequential Phragm\'en) makes its decisions online, and the other two satisfy strengthened versions of the axioms but make decisions semi-online (Method of Equal Shares) or fully offline (Proportional Approval Voting). The first two are polynomial-time computable, and the latter is based on an NP-hard optimization, but it admits a polynomial-time local search algorithm that satisfies the same axiomatic properties. We present empirical results about the performance of these rules based on synthetic data and U.S. political elections. We also run experiments where votes are cast by preference models trained on user responses from the moral machine dataset about ethical dilemmas.
Proportional Rankings
Dec 05, 2016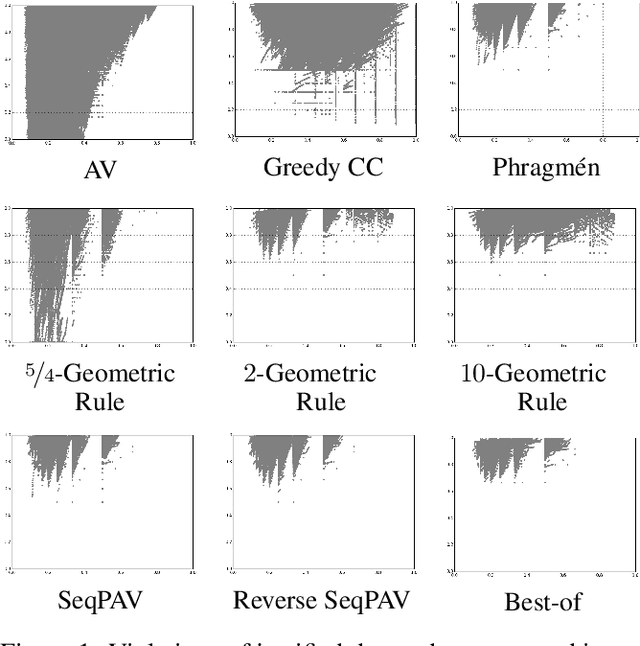
Abstract:In this paper we extend the principle of proportional representation to rankings. We consider the setting where alternatives need to be ranked based on approval preferences. In this setting, proportional representation requires that cohesive groups of voters are represented proportionally in each initial segment of the ranking. Proportional rankings are desirable in situations where initial segments of different lengths may be relevant, e.g., hiring decisions (if it is unclear how many positions are to be filled), the presentation of competing proposals on a liquid democracy platform (if it is unclear how many proposals participants are taking into consideration), or recommender systems (if a ranking has to accommodate different user types). We study the proportional representation provided by several ranking methods and prove theoretical guarantees. Furthermore, we experimentally evaluate these methods and present preliminary evidence as to which methods are most suitable for producing proportional rankings.
Interdependent Scheduling Games
May 31, 2016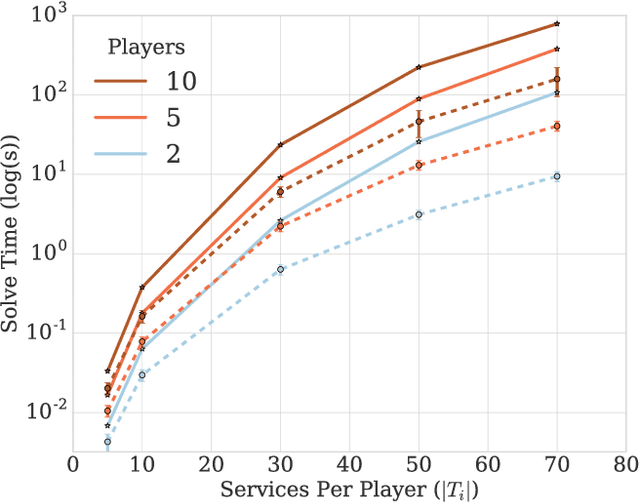
Abstract:We propose a model of interdependent scheduling games in which each player controls a set of services that they schedule independently. A player is free to schedule his own services at any time; however, each of these services only begins to accrue reward for the player when all predecessor services, which may or may not be controlled by the same player, have been activated. This model, where players have interdependent services, is motivated by the problems faced in planning and coordinating large-scale infrastructures, e.g., restoring electricity and gas to residents after a natural disaster or providing medical care in a crisis when different agencies are responsible for the delivery of staff, equipment, and medicine. We undertake a game-theoretic analysis of this setting and in particular consider the issues of welfare maximization, computing best responses, Nash dynamics, and existence and computation of Nash equilibria.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge