Online Reasoning Video Segmentation with Just-in-Time Digital Twins

Reasoning segmentation (RS) aims to identify and segment objects of interest based on implicit text queries. As such, RS is a catalyst for embodied AI agents, enabling them to interpret high-level commands without requiring explicit step-by-step guidance. However, current RS approaches rely heavily on the visual perception capabilities of multimodal large language models (LLMs), leading to several major limitations. First, they struggle with queries that require multiple steps of reasoning or those that involve complex spatial/temporal relationships. Second, they necessitate LLM fine-tuning, which may require frequent updates to maintain compatibility with contemporary LLMs and may increase risks of catastrophic forgetting during fine-tuning. Finally, being primarily designed for static images or offline video processing, they scale poorly to online video data. To address these limitations, we propose an agent framework that disentangles perception and reasoning for online video RS without LLM fine-tuning. Our innovation is the introduction of a just-in-time digital twin concept, where -- given an implicit query -- a LLM plans the construction of a low-level scene representation from high-level video using specialist vision models. We refer to this approach to creating a digital twin as "just-in-time" because the LLM planner will anticipate the need for specific information and only request this limited subset instead of always evaluating every specialist model. The LLM then performs reasoning on this digital twin representation to identify target objects. To evaluate our approach, we introduce a new comprehensive video reasoning segmentation benchmark comprising 200 videos with 895 implicit text queries. The benchmark spans three reasoning categories (semantic, spatial, and temporal) with three different reasoning chain complexity.

Operating Room Workflow Analysis via Reasoning Segmentation over Digital Twins

Analyzing operating room (OR) workflows to derive quantitative insights into OR efficiency is important for hospitals to maximize patient care and financial sustainability. Prior work on OR-level workflow analysis has relied on end-to-end deep neural networks. While these approaches work well in constrained settings, they are limited to the conditions specified at development time and do not offer the flexibility necessary to accommodate the OR workflow analysis needs of various OR scenarios (e.g., large academic center vs. rural provider) without data collection, annotation, and retraining. Reasoning segmentation (RS) based on foundation models offers this flexibility by enabling automated analysis of OR workflows from OR video feeds given only an implicit text query related to the objects of interest. Due to the reliance on large language model (LLM) fine-tuning, current RS approaches struggle with reasoning about semantic/spatial relationships and show limited generalization to OR video due to variations in visual characteristics and domain-specific terminology. To address these limitations, we first propose a novel digital twin (DT) representation that preserves both semantic and spatial relationships between the various OR components. Then, building on this foundation, we propose ORDiRS (Operating Room Digital twin representation for Reasoning Segmentation), an LLM-tuning-free RS framework that reformulates RS into a "reason-retrieval-synthesize" paradigm. Finally, we present ORDiRS-Agent, an LLM-based agent that decomposes OR workflow analysis queries into manageable RS sub-queries and generates responses by combining detailed textual explanations with supporting visual evidence from RS. Experimental results on both an in-house and a public OR dataset demonstrate that our ORDiRS achieves a cIoU improvement of 6.12%-9.74% compared to the existing state-of-the-arts.

Boosting the Local Invariance for Better Adversarial Transferability

Transfer-based attacks pose a significant threat to real-world applications by directly targeting victim models with adversarial examples generated on surrogate models. While numerous approaches have been proposed to enhance adversarial transferability, existing works often overlook the intrinsic relationship between adversarial perturbations and input images. In this work, we find that adversarial perturbation often exhibits poor translation invariance for a given clean image and model, which is attributed to local invariance. Through empirical analysis, we demonstrate that there is a positive correlation between the local invariance of adversarial perturbations w.r.t. the input image and their transferability across different models. Based on this finding, we propose a general adversarial transferability boosting technique called Local Invariance Boosting approach (LI-Boost). Extensive experiments on the standard ImageNet dataset demonstrate that LI-Boost could significantly boost various types of transfer-based attacks (e.g., gradient-based, input transformation-based, model-related, advanced objective function, ensemble, etc.) on CNNs, ViTs, and defense mechanisms. Our approach presents a promising direction for future research in improving adversarial transferability across different models.

Towards Distributed Backdoor Attacks with Network Detection in Decentralized Federated Learning

Distributed backdoor attacks (DBA) have shown a higher attack success rate than centralized attacks in centralized federated learning (FL). However, it has not been investigated in the decentralized FL. In this paper, we experimentally demonstrate that, while directly applying DBA to decentralized FL, the attack success rate depends on the distribution of attackers in the network architecture. Considering that the attackers can not decide their location, this paper aims to achieve a high attack success rate regardless of the attackers' location distribution. Specifically, we first design a method to detect the network by predicting the distance between any two attackers on the network. Then, based on the distance, we organize the attackers in different clusters. Lastly, we propose an algorithm to \textit{dynamically} embed local patterns decomposed from a global pattern into the different attackers in each cluster. We conduct a thorough empirical investigation and find that our method can, in benchmark datasets, outperform both centralized attacks and naive DBA in different decentralized frameworks.

A Survey of Lottery Ticket Hypothesis

The Lottery Ticket Hypothesis (LTH) states that a dense neural network model contains a highly sparse subnetwork (i.e., winning tickets) that can achieve even better performance than the original model when trained in isolation. While LTH has been proved both empirically and theoretically in many works, there still are some open issues, such as efficiency and scalability, to be addressed. Also, the lack of open-source frameworks and consensual experimental setting poses a challenge to future research on LTH. We, for the first time, examine previous research and studies on LTH from different perspectives. We also discuss issues in existing works and list potential directions for further exploration. This survey aims to provide an in-depth look at the state of LTH and develop a duly maintained platform to conduct experiments and compare with the most updated baselines.

Segmentation of Mediastinal Lymph Nodes in CT with Anatomical Priors

Purpose: Lymph nodes (LNs) in the chest have a tendency to enlarge due to various pathologies, such as lung cancer or pneumonia. Clinicians routinely measure nodal size to monitor disease progression, confirm metastatic cancer, and assess treatment response. However, variations in their shapes and appearances make it cumbersome to identify LNs, which reside outside of most organs. Methods: We propose to segment LNs in the mediastinum by leveraging the anatomical priors of 28 different structures (e.g., lung, trachea etc.) generated by the public TotalSegmentator tool. The CT volumes from 89 patients available in the public NIH CT Lymph Node dataset were used to train three 3D nnUNet models to segment LNs. The public St. Olavs dataset containing 15 patients (out-of-training-distribution) was used to evaluate the segmentation performance. Results: For the 15 test patients, the 3D cascade nnUNet model obtained the highest Dice score of 72.2 +- 22.3 for mediastinal LNs with short axis diameter $\geq$ 8mm and 54.8 +- 23.8 for all LNs respectively. These results represent an improvement of 10 points over a current approach that was evaluated on the same test dataset. Conclusion: To our knowledge, we are the first to harness 28 distinct anatomical priors to segment mediastinal LNs, and our work can be extended to other nodal zones in the body. The proposed method has immense potential for improved patient outcomes through the identification of enlarged nodes in initial staging CT scans.

Successfully Applying Lottery Ticket Hypothesis to Diffusion Model

Despite the success of diffusion models, the training and inference of diffusion models are notoriously expensive due to the long chain of the reverse process. In parallel, the Lottery Ticket Hypothesis (LTH) claims that there exists winning tickets (i.e., aproperly pruned sub-network together with original weight initialization) that can achieve performance competitive to the original dense neural network when trained in isolation. In this work, we for the first time apply LTH to diffusion models. We empirically find subnetworks at sparsity 90%-99% without compromising performance for denoising diffusion probabilistic models on benchmarks (CIFAR-10, CIFAR-100, MNIST). Moreover, existing LTH works identify the subnetworks with a unified sparsity along different layers. We observe that the similarity between two winning tickets of a model varies from block to block. Specifically, the upstream layers from two winning tickets for a model tend to be more similar than the downstream layers. Therefore, we propose to find the winning ticket with varying sparsity along different layers in the model. Experimental results demonstrate that our method can find sparser sub-models that require less memory for storage and reduce the necessary number of FLOPs. Codes are available at https://github.com/osier0524/Lottery-Ticket-to-DDPM.

UrbanIR: Large-Scale Urban Scene Inverse Rendering from a Single Video

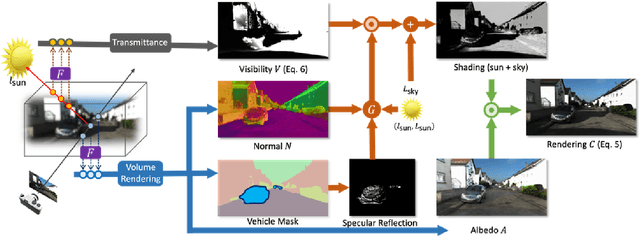
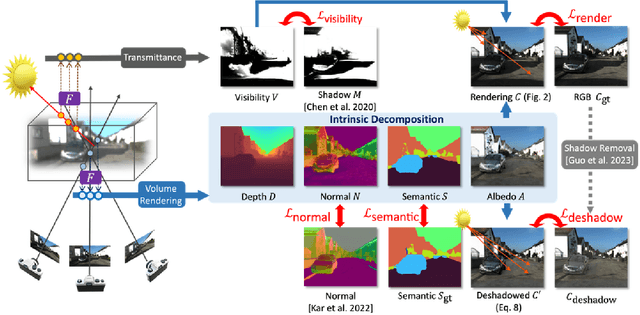
We show how to build a model that allows realistic, free-viewpoint renderings of a scene under novel lighting conditions from video. Our method -- UrbanIR: Urban Scene Inverse Rendering -- computes an inverse graphics representation from the video. UrbanIR jointly infers shape, albedo, visibility, and sun and sky illumination from a single video of unbounded outdoor scenes with unknown lighting. UrbanIR uses videos from cameras mounted on cars (in contrast to many views of the same points in typical NeRF-style estimation). As a result, standard methods produce poor geometry estimates (for example, roofs), and there are numerous ''floaters''. Errors in inverse graphics inference can result in strong rendering artifacts. UrbanIR uses novel losses to control these and other sources of error. UrbanIR uses a novel loss to make very good estimates of shadow volumes in the original scene. The resulting representations facilitate controllable editing, delivering photorealistic free-viewpoint renderings of relit scenes and inserted objects. Qualitative evaluation demonstrates strong improvements over the state-of-the-art.

Random Subspace Learning Approach to High-Dimensional Outliers Detection

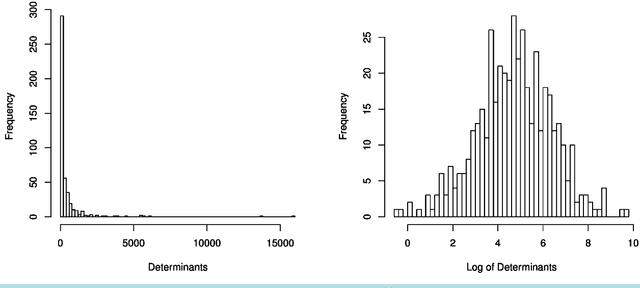
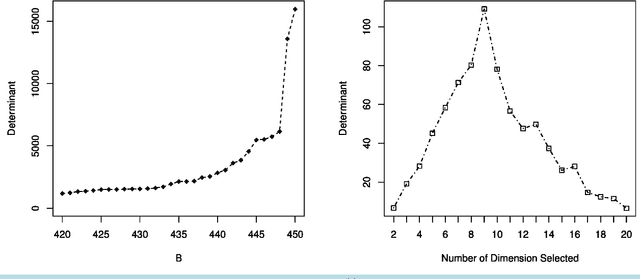
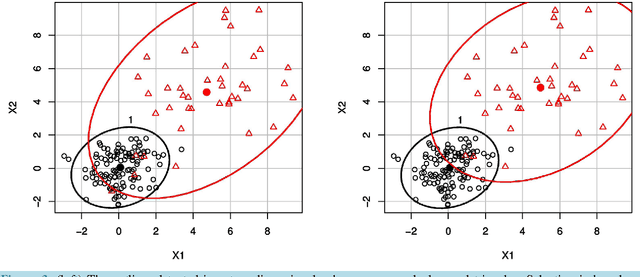
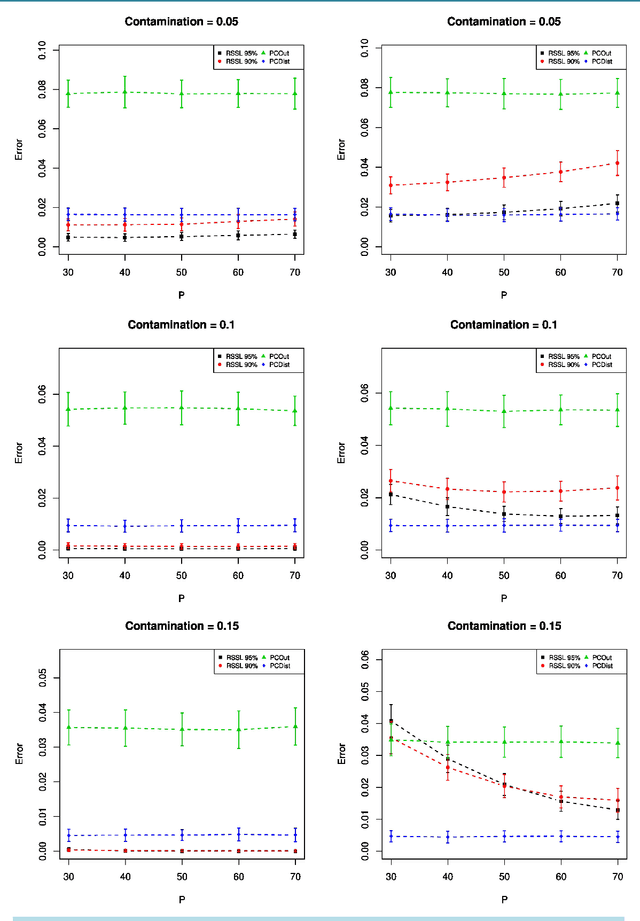
We introduce and develop a novel approach to outlier detection based on adaptation of random subspace learning. Our proposed method handles both high-dimension low-sample size and traditional low-dimensional high-sample size datasets. Essentially, we avoid the computational bottleneck of techniques like minimum covariance determinant (MCD) by computing the needed determinants and associated measures in much lower dimensional subspaces. Both theoretical and computational development of our approach reveal that it is computationally more efficient than the regularized methods in high-dimensional low-sample size, and often competes favorably with existing methods as far as the percentage of correct outlier detection is concerned.