DDPM Score Matching and Distribution Learning

Score estimation is the backbone of score-based generative models (SGMs), especially denoising diffusion probabilistic models (DDPMs). A key result in this area shows that with accurate score estimates, SGMs can efficiently generate samples from any realistic data distribution (Chen et al., ICLR'23; Lee et al., ALT'23). This distribution learning result, where the learned distribution is implicitly that of the sampler's output, does not explain how score estimation relates to classical tasks of parameter and density estimation. This paper introduces a framework that reduces score estimation to these two tasks, with various implications for statistical and computational learning theory: Parameter Estimation: Koehler et al. (ICLR'23) demonstrate that a score-matching variant is statistically inefficient for the parametric estimation of multimodal densities common in practice. In contrast, we show that under mild conditions, denoising score-matching in DDPMs is asymptotically efficient. Density Estimation: By linking generation to score estimation, we lift existing score estimation guarantees to $(\epsilon,\delta)$-PAC density estimation, i.e., a function approximating the target log-density within $\epsilon$ on all but a $\delta$-fraction of the space. We provide (i) minimax rates for density estimation over H\"older classes and (ii) a quasi-polynomial PAC density estimation algorithm for the classical Gaussian location mixture model, building on and addressing an open problem from Gatmiry et al. (arXiv'24). Lower Bounds for Score Estimation: Our framework offers the first principled method to prove computational lower bounds for score estimation across general distributions. As an application, we establish cryptographic lower bounds for score estimation in general Gaussian mixture models, conceptually recovering Song's (NeurIPS'24) result and advancing his key open problem.

Can SGD Select Good Fishermen? Local Convergence under Self-Selection Biases and Beyond

We revisit the problem of estimating $k$ linear regressors with self-selection bias in $d$ dimensions with the maximum selection criterion, as introduced by Cherapanamjeri, Daskalakis, Ilyas, and Zampetakis [CDIZ23, STOC'23]. Our main result is a $\operatorname{poly}(d,k,1/\varepsilon) + {k}^{O(k)}$ time algorithm for this problem, which yields an improvement in the running time of the algorithms of [CDIZ23] and [GM24, arXiv]. We achieve this by providing the first local convergence algorithm for self-selection, thus resolving the main open question of [CDIZ23]. To obtain this algorithm, we reduce self-selection to a seemingly unrelated statistical problem called coarsening. Coarsening occurs when one does not observe the exact value of the sample but only some set (a subset of the sample space) that contains the exact value. Inference from coarse samples arises in various real-world applications due to rounding by humans and algorithms, limited precision of instruments, and lag in multi-agent systems. Our reduction to coarsening is intuitive and relies on the geometry of the self-selection problem, which enables us to bypass the limitations of previous analytic approaches. To demonstrate its applicability, we provide a local convergence algorithm for linear regression under another self-selection criterion, which is related to second-price auction data. Further, we give the first polynomial time local convergence algorithm for coarse Gaussian mean estimation given samples generated from a convex partition. Previously, only a sample-efficient algorithm was known due to Fotakis, Kalavasis, Kontonis, and Tzamos [FKKT21, COLT'21].

Does Generation Require Memorization? Creative Diffusion Models using Ambient Diffusion

There is strong empirical evidence that the state-of-the-art diffusion modeling paradigm leads to models that memorize the training set, especially when the training set is small. Prior methods to mitigate the memorization problem often lead to a decrease in image quality. Is it possible to obtain strong and creative generative models, i.e., models that achieve high generation quality and low memorization? Despite the current pessimistic landscape of results, we make significant progress in pushing the trade-off between fidelity and memorization. We first provide theoretical evidence that memorization in diffusion models is only necessary for denoising problems at low noise scales (usually used in generating high-frequency details). Using this theoretical insight, we propose a simple, principled method to train the diffusion models using noisy data at large noise scales. We show that our method significantly reduces memorization without decreasing the image quality, for both text-conditional and unconditional models and for a variety of data availability settings.

Characterizations of Language Generation With Breadth

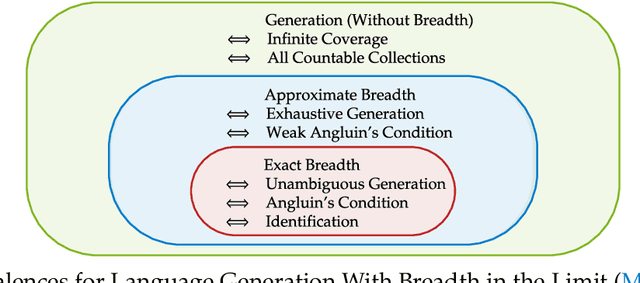
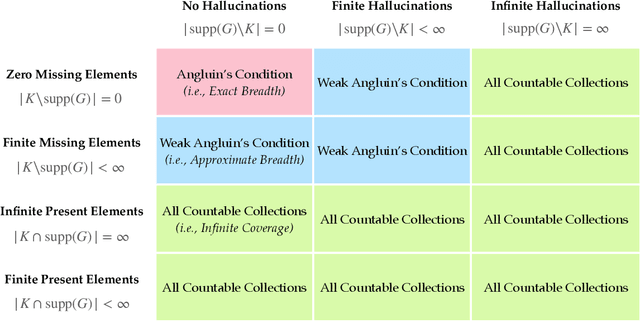
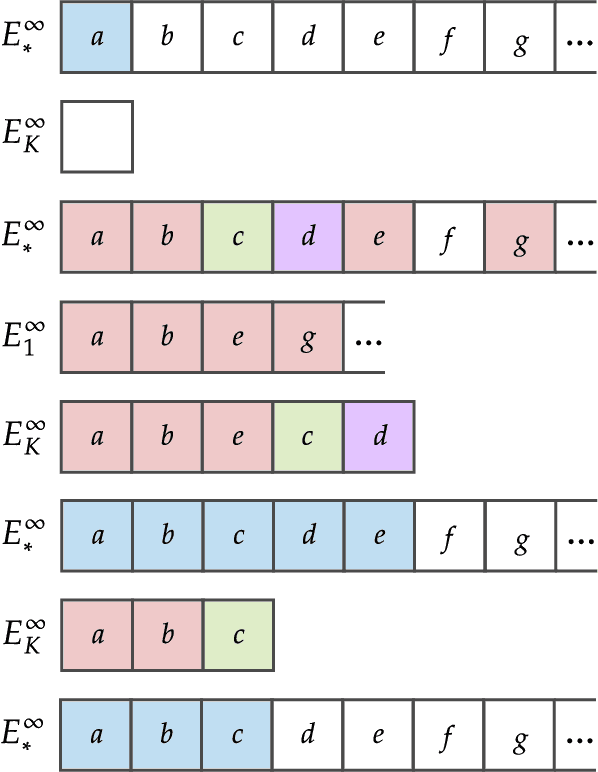
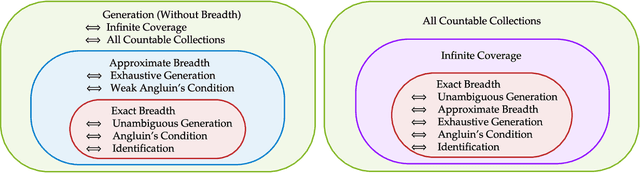
We study language generation in the limit, introduced by Kleinberg and Mullainathan [KM24], building on classical works of Gold [Gol67] and Angluin [Ang79]. [KM24] proposed an algorithm that generates strings from any countable language collection in the limit. While their algorithm eventually outputs strings from the target language $K$, it sacrifices breadth, i.e., the ability to generate all strings in $K$. A key open question in [KM24] is whether this trade-off between consistency and breadth is inherrent. Recent works proposed different notions of consistent generation with breadth. Kalavasis, Mehrotra, and Velegkas [KVM24] introduced three definitions: generation with exact breadth, approximate breadth, and unambiguous generation. Concurrently and independently, Charikar and Pabbaraju [CP24a] proposed exhaustive generation. Both works examined when generation with these notions of breadth is possible. Building on [CP24a, KVM24], we fully characterize language generation for these notions and their natural combinations. For exact breadth, we provide an unconditional lower bound, removing a technical condition from [KVM24] and extending the result of [CP24a] that holds for specific collections of languages. We show that generation with exact breadth is characterized by Angluin's condition for identification. We further introduce a weaker version of Angluin's condition that tightly characterizes both approximate breadth and exhaustive generation, proving their equivalence. Additionally, we show that unambiguous generation is also characterized by Angluin's condition as a special case of a broader result. Finally, we strengthen [KVM24] by giving unconditional lower bounds for stable generators, showing that Angluin's condition characterizes the previous breadth notions for stable generators. This shows a separation between stable and unstable generation with approximate breadth.

On the Limits of Language Generation: Trade-Offs Between Hallucination and Mode Collapse

Specifying all desirable properties of a language model is challenging, but certain requirements seem essential. Given samples from an unknown language, the trained model should produce valid strings not seen in training and be expressive enough to capture the language's full richness. Otherwise, outputting invalid strings constitutes "hallucination," and failing to capture the full range leads to "mode collapse." We ask if a language model can meet both requirements. We investigate this within a statistical language generation setting building on Gold and Angluin. Here, the model receives random samples from a distribution over an unknown language K, which belongs to a possibly infinite collection of languages. The goal is to generate unseen strings from K. We say the model generates from K with consistency and breadth if, as training size increases, its output converges to all unseen strings in K. Kleinberg and Mullainathan [KM24] asked if consistency and breadth in language generation are possible. We answer this negatively: for a large class of language models, including next-token prediction models, this is impossible for most collections of candidate languages. This contrasts with [KM24]'s result, showing consistent generation without breadth is possible for any countable collection of languages. Our finding highlights that generation with breadth fundamentally differs from generation without breadth. As a byproduct, we establish near-tight bounds on the number of samples needed for generation with or without breadth. Finally, our results offer hope: consistent generation with breadth is achievable for any countable collection of languages when negative examples (strings outside K) are available alongside positive ones. This suggests that post-training feedback, which encodes negative examples, can be crucial in reducing hallucinations while limiting mode collapse.

Barriers to Welfare Maximization with No-Regret Learning

A celebrated result in the interface of online learning and game theory guarantees that the repeated interaction of no-regret players leads to a coarse correlated equilibrium (CCE) -- a natural game-theoretic solution concept. Despite the rich history of this foundational problem and the tremendous interest it has received in recent years, a basic question still remains open: how many iterations are needed for no-regret players to approximate an equilibrium? In this paper, we establish the first computational lower bounds for that problem in two-player (general-sum) games under the constraint that the CCE reached approximates the optimal social welfare (or some other natural objective). From a technical standpoint, our approach revolves around proving lower bounds for computing a near-optimal $T$-sparse CCE -- a mixture of $T$ product distributions, thereby circumscribing the iteration complexity of no-regret learning even in the centralized model of computation. Our proof proceeds by extending a classical reduction of Gilboa and Zemel [1989] for optimal Nash to sparse (approximate) CCE. In particular, we show that the inapproximability of maximum clique precludes attaining any non-trivial sparsity in polynomial time. Moreover, we strengthen our hardness results to apply in the low-precision regime as well via the planted clique conjecture.

Computational Lower Bounds for Regret Minimization in Normal-Form Games

A celebrated connection in the interface of online learning and game theory establishes that players minimizing swap regret converge to correlated equilibria (CE) -- a seminal game-theoretic solution concept. Despite the long history of this problem and the renewed interest it has received in recent years, a basic question remains open: how many iterations are needed to approximate an equilibrium under the usual normal-form representation? In this paper, we provide evidence that existing learning algorithms, such as multiplicative weights update, are close to optimal. In particular, we prove lower bounds for the problem of computing a CE that can be expressed as a uniform mixture of $T$ product distributions -- namely, a uniform $T$-sparse CE; such lower bounds immediately circumscribe (computationally bounded) regret minimization algorithms in games. Our results are obtained in the algorithmic framework put forward by Kothari and Mehta (STOC 2018) in the context of computing Nash equilibria, which consists of the sum-of-squares (SoS) relaxation in conjunction with oracle access to a verification oracle; the goal in that framework is to lower bound either the degree of the SoS relaxation or the number of queries to the verification oracle. Here, we obtain two such hardness results, precluding computing i) uniform $\text{log }n$-sparse CE when $\epsilon =\text{poly}(1/\text{log }n)$ and ii) uniform $n^{1 - o(1)}$-sparse CE when $\epsilon = \text{poly}(1/n)$.

Smaller Confidence Intervals From IPW Estimators via Data-Dependent Coarsening

Inverse propensity-score weighted (IPW) estimators are prevalent in causal inference for estimating average treatment effects in observational studies. Under unconfoundedness, given accurate propensity scores and $n$ samples, the size of confidence intervals of IPW estimators scales down with $n$, and, several of their variants improve the rate of scaling. However, neither IPW estimators nor their variants are robust to inaccuracies: even if a single covariate has an $\varepsilon>0$ additive error in the propensity score, the size of confidence intervals of these estimators can increase arbitrarily. Moreover, even without errors, the rate with which the confidence intervals of these estimators go to zero with $n$ can be arbitrarily slow in the presence of extreme propensity scores (those close to 0 or 1). We introduce a family of Coarse IPW (CIPW) estimators that captures existing IPW estimators and their variants. Each CIPW estimator is an IPW estimator on a coarsened covariate space, where certain covariates are merged. Under mild assumptions, e.g., Lipschitzness in expected outcomes and sparsity of extreme propensity scores, we give an efficient algorithm to find a robust estimator: given $\varepsilon$-inaccurate propensity scores and $n$ samples, its confidence interval size scales with $\varepsilon+1/\sqrt{n}$. In contrast, under the same assumptions, existing estimators' confidence interval sizes are $\Omega(1)$ irrespective of $\varepsilon$ and $n$. Crucially, our estimator is data-dependent and we show that no data-independent CIPW estimator can be robust to inaccuracies.

Injecting Undetectable Backdoors in Deep Learning and Language Models

As ML models become increasingly complex and integral to high-stakes domains such as finance and healthcare, they also become more susceptible to sophisticated adversarial attacks. We investigate the threat posed by undetectable backdoors in models developed by insidious external expert firms. When such backdoors exist, they allow the designer of the model to sell information to the users on how to carefully perturb the least significant bits of their input to change the classification outcome to a favorable one. We develop a general strategy to plant a backdoor to neural networks while ensuring that even if the model's weights and architecture are accessible, the existence of the backdoor is still undetectable. To achieve this, we utilize techniques from cryptography such as cryptographic signatures and indistinguishability obfuscation. We further introduce the notion of undetectable backdoors to language models and extend our neural network backdoor attacks to such models based on the existence of steganographic functions.

On the Computational Landscape of Replicable Learning

We study computational aspects of algorithmic replicability, a notion of stability introduced by Impagliazzo, Lei, Pitassi, and Sorrell [2022]. Motivated by a recent line of work that established strong statistical connections between replicability and other notions of learnability such as online learning, private learning, and SQ learning, we aim to understand better the computational connections between replicability and these learning paradigms. Our first result shows that there is a concept class that is efficiently replicably PAC learnable, but, under standard cryptographic assumptions, no efficient online learner exists for this class. Subsequently, we design an efficient replicable learner for PAC learning parities when the marginal distribution is far from uniform, making progress on a question posed by Impagliazzo et al. [2022]. To obtain this result, we design a replicable lifting framework inspired by Blanc, Lange, Malik, and Tan [2023] that transforms in a black-box manner efficient replicable PAC learners under the uniform marginal distribution over the Boolean hypercube to replicable PAC learners under any marginal distribution, with sample and time complexity that depends on a certain measure of the complexity of the distribution. Finally, we show that any pure DP learner can be transformed to a replicable one in time polynomial in the accuracy, confidence parameters and exponential in the representation dimension of the underlying hypothesis class.