EOCSA: Predicting Prognosis of Epithelial Ovarian Cancer with Whole Slide Histopathological Images

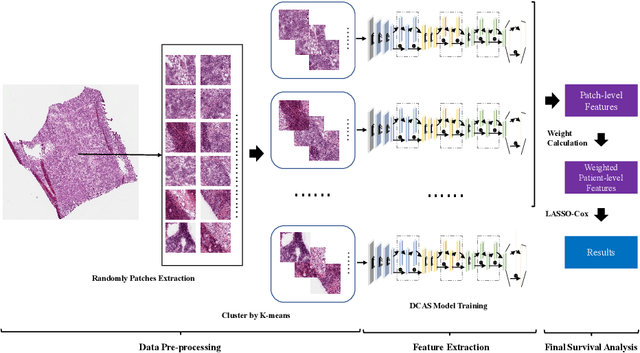
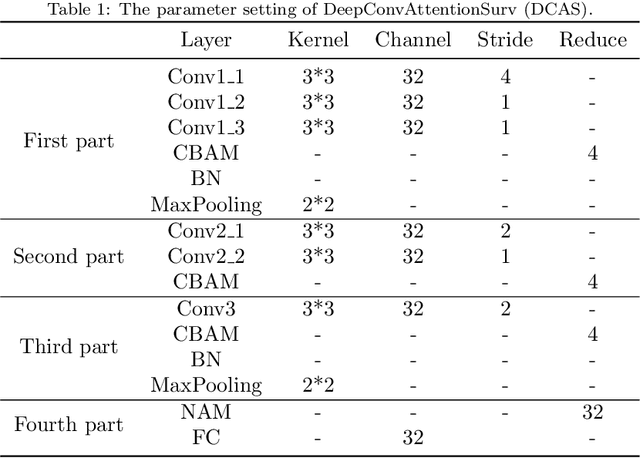
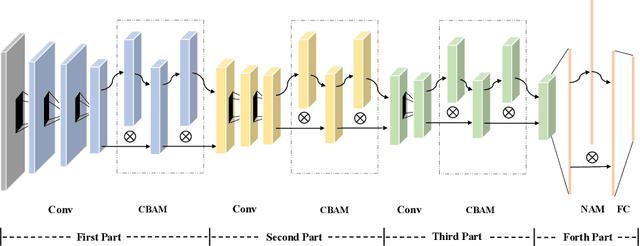
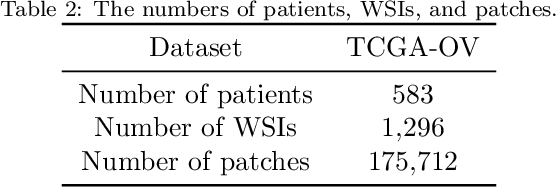
Ovarian cancer is one of the most serious cancers that threaten women around the world. Epithelial ovarian cancer (EOC), as the most commonly seen subtype of ovarian cancer, has rather high mortality rate and poor prognosis among various gynecological cancers. Survival analysis outcome is able to provide treatment advices to doctors. In recent years, with the development of medical imaging technology, survival prediction approaches based on pathological images have been proposed. In this study, we designed a deep framework named EOCSA which analyzes the prognosis of EOC patients based on pathological whole slide images (WSIs). Specifically, we first randomly extracted patches from WSIs and grouped them into multiple clusters. Next, we developed a survival prediction model, named DeepConvAttentionSurv (DCAS), which was able to extract patch-level features, removed less discriminative clusters and predicted the EOC survival precisely. Particularly, channel attention, spatial attention, and neuron attention mechanisms were used to improve the performance of feature extraction. Then patient-level features were generated from our weight calculation method and the survival time was finally estimated using LASSO-Cox model. The proposed EOCSA is efficient and effective in predicting prognosis of EOC and the DCAS ensures more informative and discriminative features can be extracted. As far as we know, our work is the first to analyze the survival of EOC based on WSIs and deep neural network technologies. The experimental results demonstrate that our proposed framework has achieved state-of-the-art performance of 0.980 C-index. The implementation of the approach can be found at https://github.com/RanSuLab/EOCprognosis.

Machine Discovery of Partial Differential Equations from Spatiotemporal Data

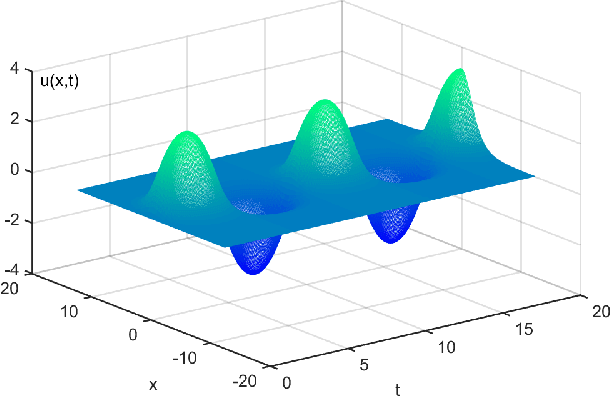
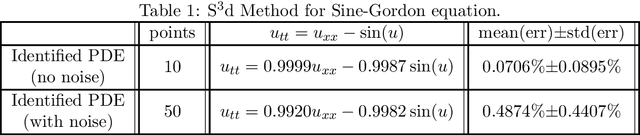
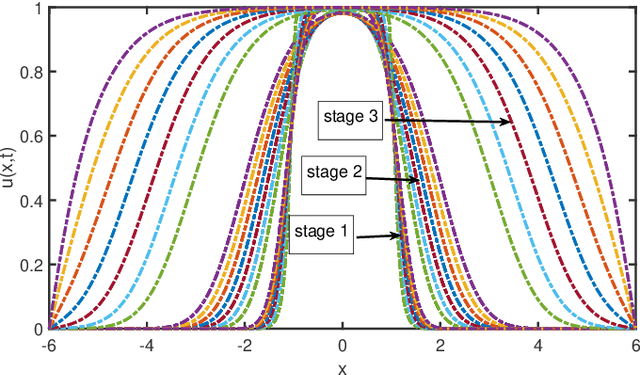
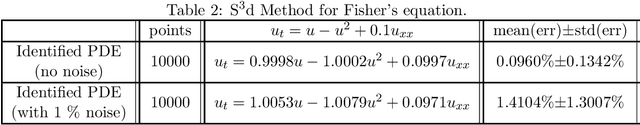
The study presents a general framework for discovering underlying Partial Differential Equations (PDEs) using measured spatiotemporal data. The method, called Sparse Spatiotemporal System Discovery ($\text{S}^3\text{d}$), decides which physical terms are necessary and which can be removed (because they are physically negligible in the sense that they do not affect the dynamics too much) from a pool of candidate functions. The method is built on the recent development of Sparse Bayesian Learning; which enforces the sparsity in the to-be-identified PDEs, and therefore can balance the model complexity and fitting error with theoretical guarantees. Without leveraging prior knowledge or assumptions in the discovery process, we use an automated approach to discover ten types of PDEs, including the famous Navier-Stokes and sine-Gordon equations, from simulation data alone. Moreover, we demonstrate our data-driven discovery process with the Complex Ginzburg-Landau Equation (CGLE) using data measured from a traveling-wave convection experiment. Our machine discovery approach presents solutions that has the potential to inspire, support and assist physicists for the establishment of physical laws from measured spatiotemporal data, especially in notorious fields that are often too complex to allow a straightforward establishment of physical law, such as biophysics, fluid dynamics, neuroscience or nonlinear optics.

Data-driven Discovery of Cyber-Physical Systems

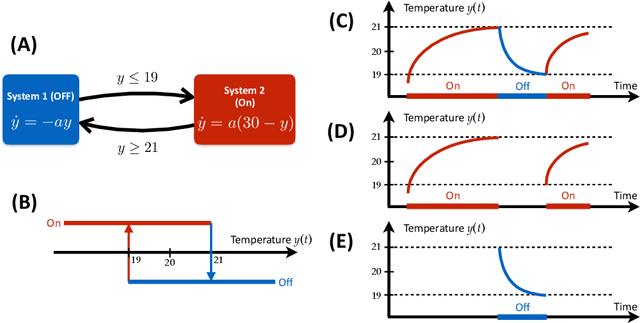
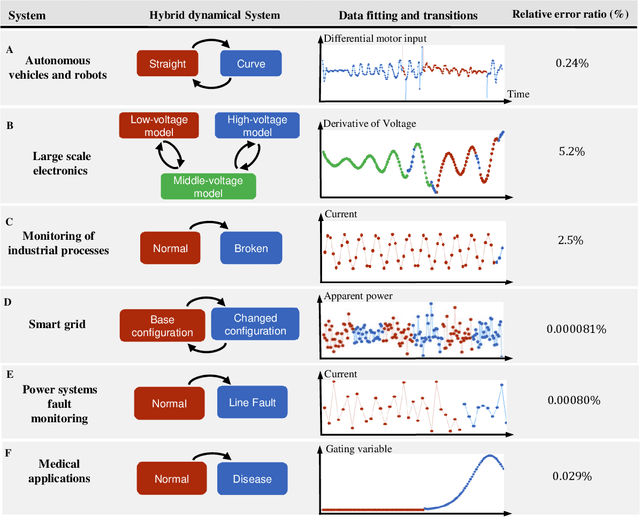
Cyber-physical systems (CPSs) embed software into the physical world. They appear in a wide range of applications such as smart grids, robotics, intelligent manufacture and medical monitoring. CPSs have proved resistant to modeling due to their intrinsic complexity arising from the combination of physical components and cyber components and the interaction between them. This study proposes a general framework for reverse engineering CPSs directly from data. The method involves the identification of physical systems as well as the inference of transition logic. It has been applied successfully to a number of real-world examples ranging from mechanical and electrical systems to medical applications. The novel framework seeks to enable researchers to make predictions concerning the trajectory of CPSs based on the discovered model. Such information has been proven essential for the assessment of the performance of CPS, the design of failure-proof CPS and the creation of design guidelines for new CPSs.