The Indirect Method for Generating Libraries of Optimal Periodic Trajectories and Its Application to Economical Bipedal Walking

Trajectory optimization is an essential tool for generating efficient and dynamically consistent gaits in legged locomotion. This paper explores the indirect method of trajectory optimization, emphasizing its application in creating optimal periodic gaits for legged systems and contrasting it with the more commonly used direct method. While the direct method provides considerable flexibility in its implementation, it is limited by its input space parameterization. In contrast, the indirect method improves accuracy by defining control inputs as functions of the system's states and costates. We tackle the convergence challenges associated with indirect shooting methods, particularly through the systematic development of gait libraries by utilizing numerical continuation methods. Our contributions include: (1) the formalization of a general periodic trajectory optimization problem that extends existing first-order necessary conditions for a broader range of cost functions and operating conditions; (2) a methodology for efficiently generating libraries of optimal trajectories (gaits) utilizing a single shooting approach combined with numerical continuation methods, including a novel approach for reconstructing Lagrange multipliers and costates from passive gaits; and (3) a comparative analysis of the indirect and direct shooting methods using a compass-gait walker as a case study, demonstrating the former's superior accuracy in generating optimal gaits. The findings underscore the potential of the indirect method for generating families of optimal gaits, thereby advancing the field of trajectory optimization in legged robotics.

Swing-Up of a Weakly Actuated Double Pendulum via Nonlinear Normal Modes

We identify the nonlinear normal modes spawning from the stable equilibrium of a double pendulum under gravity, and we establish their connection to homoclinic orbits through the unstable upright position as energy increases. This result is exploited to devise an efficient swing-up strategy for a double pendulum with weak, saturating actuators. Our approach involves stabilizing the system onto periodic orbits associated with the nonlinear modes while gradually injecting energy. Since these modes are autonomous system evolutions, the required control effort for stabilization is minimal. Even with actuator limitations of less than 1% of the maximum gravitational torque, the proposed method accomplishes the swing-up of the double pendulum by allowing sufficient time.

An Approach for Generating Families of Energetically Optimal Gaits from Passive Dynamic Walking Gaits

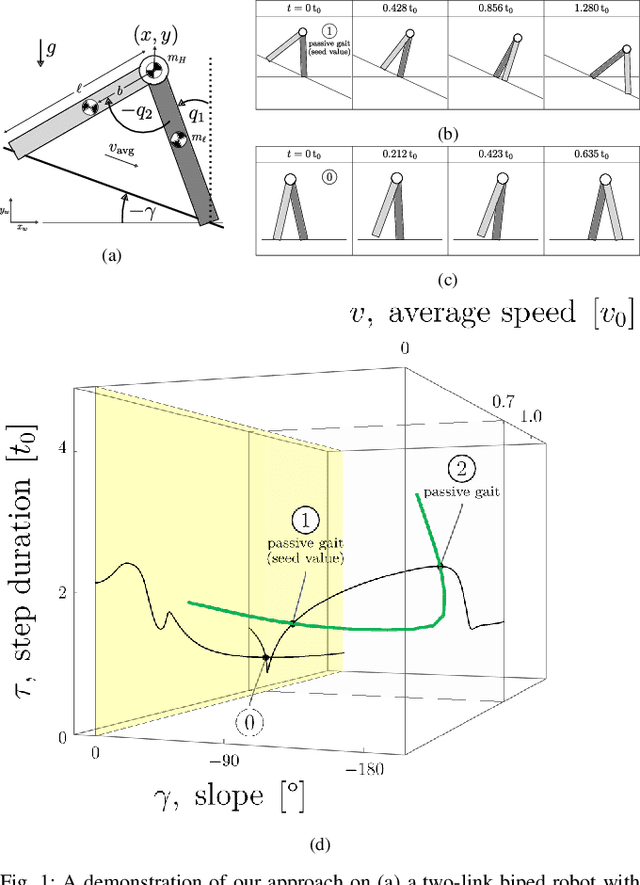
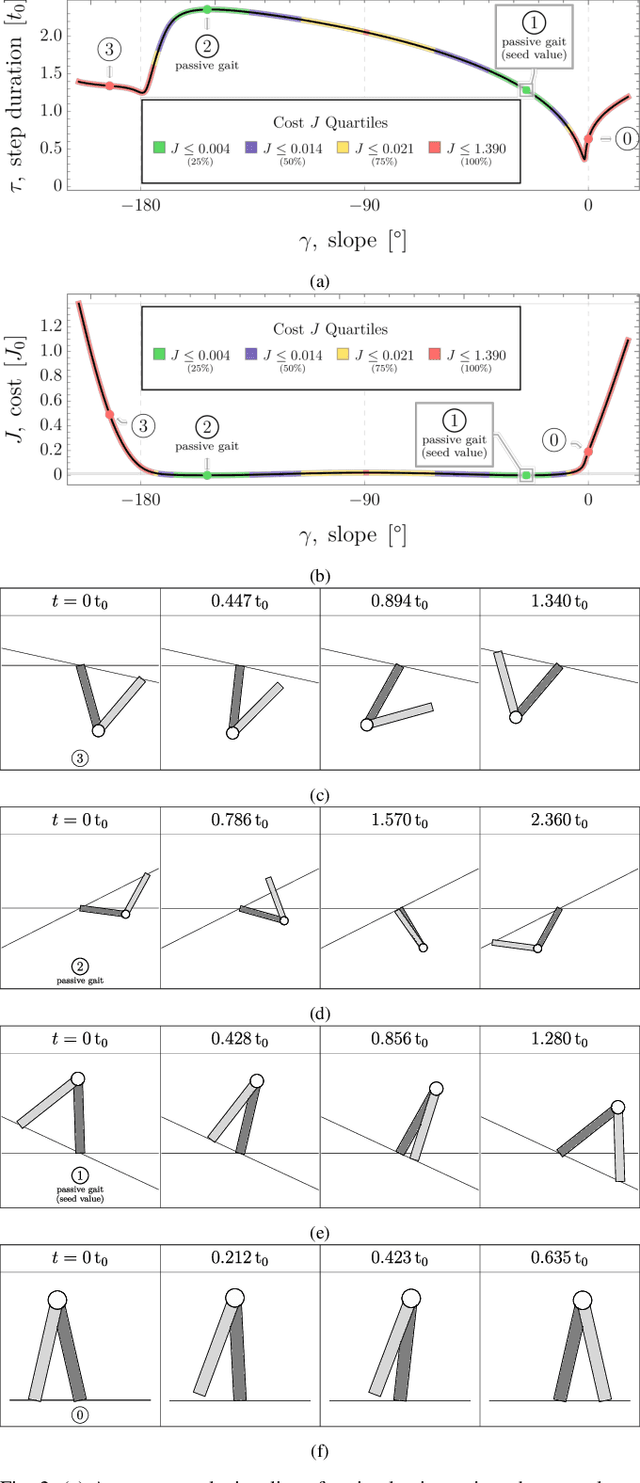
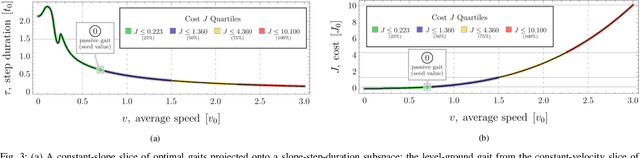
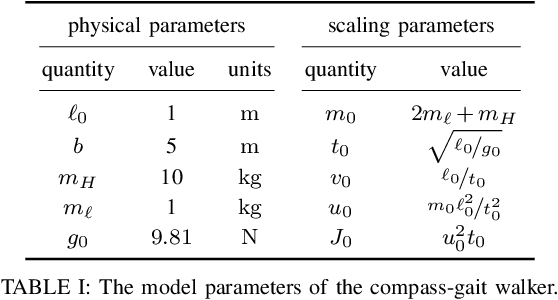
For a class of biped robots with impulsive dynamics and a non-empty set of passive gaits (unactuated, periodic motions of the biped model), we present a method for computing continuous families of locally optimal gaits with respect to a class of commonly used energetic cost functions (e.g., the integral of torque-squared). We compute these families using only the passive gaits of the biped, which are globally optimal gaits with respect to these cost functions. Our approach fills in an important gap in the literature when computing a library of locally optimal gaits, which often do not make use of these globally optimal solutions as seed values. We demonstrate our approach on a well-studied two-link biped model.

Connecting Gaits in Energetically Conservative Legged Systems

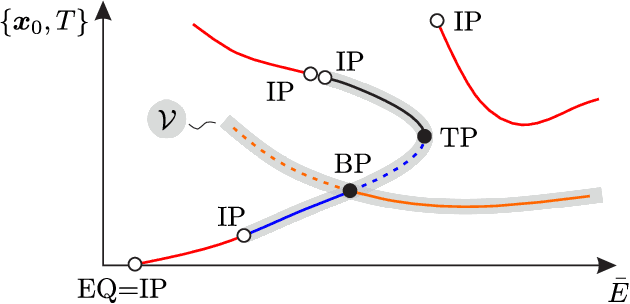
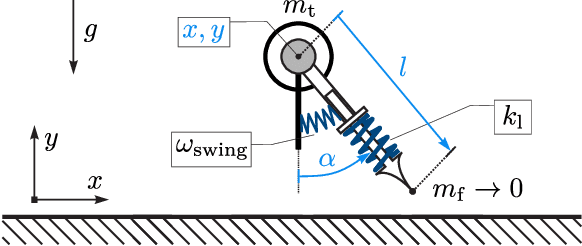
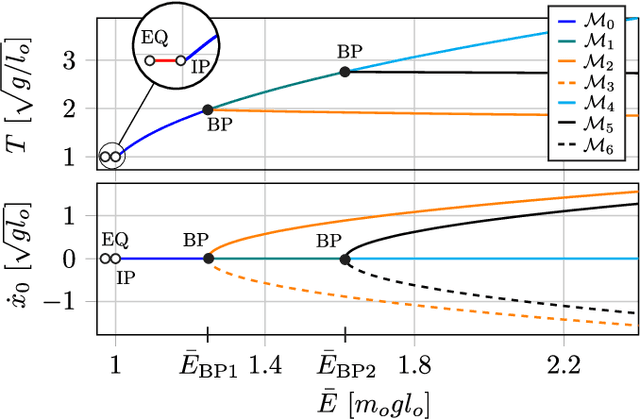
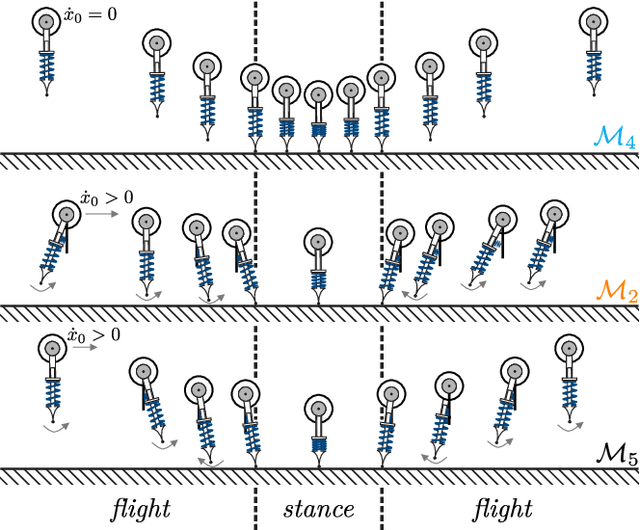
In this work, we present a nonlinear dynamics perspective on generating and connecting gaits for energetically conservative models of legged systems. In particular, we show that the set of conservative gaits constitutes a connected space of locally defined 1D submanifolds in the gait space. These manifolds are coordinate-free parameterized by energy level. We present algorithms for identifying such families of gaits through the use of numerical continuation methods, generating sets and bifurcation points. To this end, we also introduce several details for the numerical implementation. Most importantly, we establish the necessary condition for the Delassus' matrix to preserve energy across impacts. An important application of our work is with simple models of legged locomotion that are often able to capture the complexity of legged locomotion with just a few degrees of freedom and a small number of physical parameters. We demonstrate the efficacy of our framework on a one-legged hopper with four degrees of freedom.