Distributionally Robust Coreset Selection under Covariate Shift

Coreset selection, which involves selecting a small subset from an existing training dataset, is an approach to reducing training data, and various approaches have been proposed for this method. In practical situations where these methods are employed, it is often the case that the data distributions differ between the development phase and the deployment phase, with the latter being unknown. Thus, it is challenging to select an effective subset of training data that performs well across all deployment scenarios. We therefore propose Distributionally Robust Coreset Selection (DRCS). DRCS theoretically derives an estimate of the upper bound for the worst-case test error, assuming that the future covariate distribution may deviate within a defined range from the training distribution. Furthermore, by selecting instances in a way that suppresses the estimate of the upper bound for the worst-case test error, DRCS achieves distributionally robust training instance selection. This study is primarily applicable to convex training computation, but we demonstrate that it can also be applied to deep learning under appropriate approximations. In this paper, we focus on covariate shift, a type of data distribution shift, and demonstrate the effectiveness of DRCS through experiments.

Distributionally Robust Safe Sample Screening

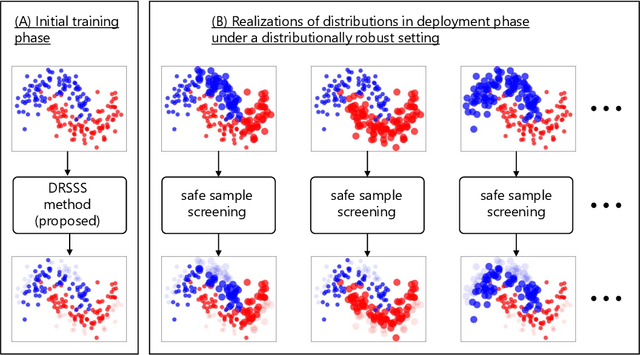
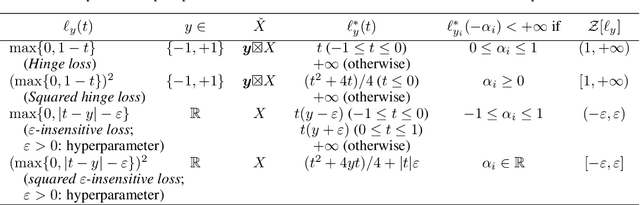
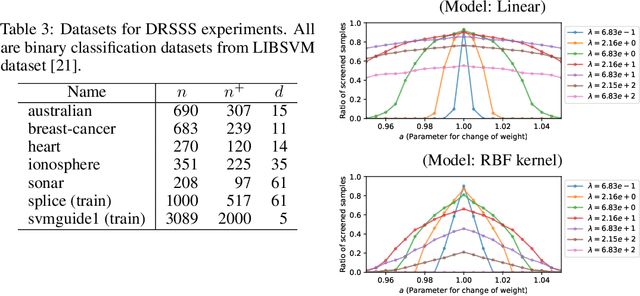
In this study, we propose a machine learning method called Distributionally Robust Safe Sample Screening (DRSSS). DRSSS aims to identify unnecessary training samples, even when the distribution of the training samples changes in the future. To achieve this, we effectively combine the distributionally robust (DR) paradigm, which aims to enhance model robustness against variations in data distribution, with the safe sample screening (SSS), which identifies unnecessary training samples prior to model training. Since we need to consider an infinite number of scenarios regarding changes in the distribution, we applied SSS because it does not require model training after the change of the distribution. In this paper, we employed the covariate shift framework to represent the distribution of training samples and reformulated the DR covariate-shift problem as a weighted empirical risk minimization problem, where the weights are subject to uncertainty within a predetermined range. By extending the existing SSS technique to accommodate this weight uncertainty, the DRSSS method is capable of reliably identifying unnecessary samples under any future distribution within a specified range. We provide a theoretical guarantee for the DRSSS method and validate its performance through numerical experiments on both synthetic and real-world datasets.

$f$-Cal: Calibrated aleatoric uncertainty estimation from neural networks for robot perception

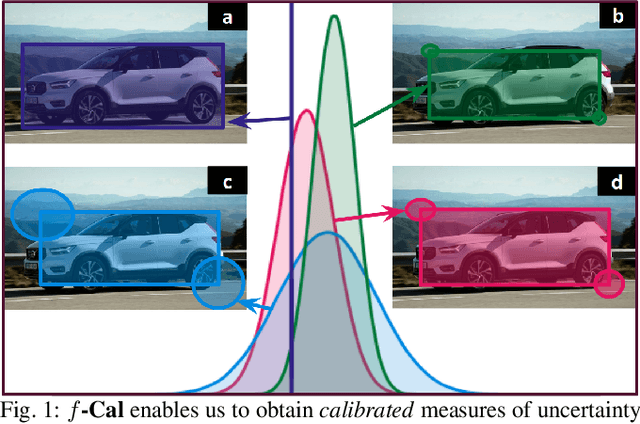
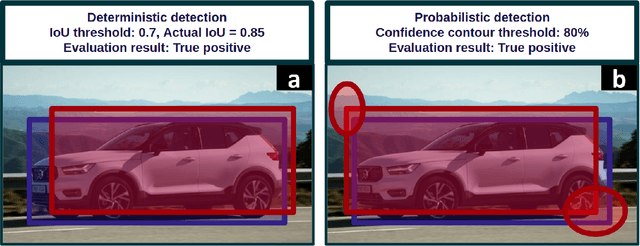
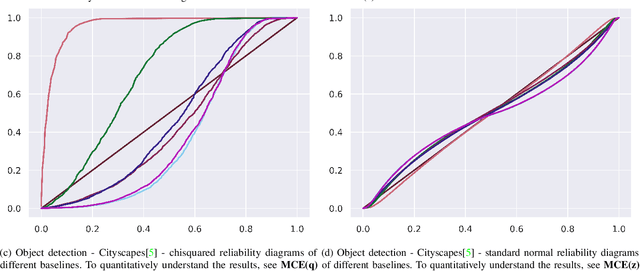
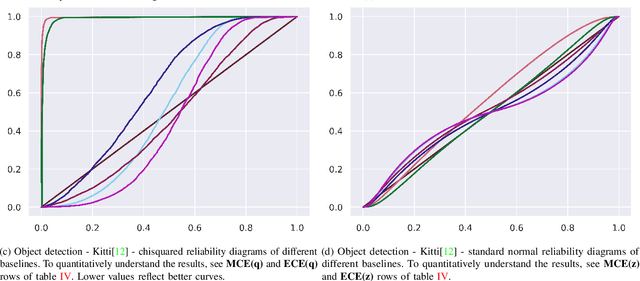
While modern deep neural networks are performant perception modules, performance (accuracy) alone is insufficient, particularly for safety-critical robotic applications such as self-driving vehicles. Robot autonomy stacks also require these otherwise blackbox models to produce reliable and calibrated measures of confidence on their predictions. Existing approaches estimate uncertainty from these neural network perception stacks by modifying network architectures, inference procedure, or loss functions. However, in general, these methods lack calibration, meaning that the predictive uncertainties do not faithfully represent the true underlying uncertainties (process noise). Our key insight is that calibration is only achieved by imposing constraints across multiple examples, such as those in a mini-batch; as opposed to existing approaches which only impose constraints per-sample, often leading to overconfident (thus miscalibrated) uncertainty estimates. By enforcing the distribution of outputs of a neural network to resemble a target distribution by minimizing an $f$-divergence, we obtain significantly better-calibrated models compared to prior approaches. Our approach, $f$-Cal, outperforms existing uncertainty calibration approaches on robot perception tasks such as object detection and monocular depth estimation over multiple real-world benchmarks.