TaskMet: Task-Driven Metric Learning for Model Learning

Deep learning models are often deployed in downstream tasks that the training procedure may not be aware of. For example, models solely trained to achieve accurate predictions may struggle to perform well on downstream tasks because seemingly small prediction errors may incur drastic task errors. The standard end-to-end learning approach is to make the task loss differentiable or to introduce a differentiable surrogate that the model can be trained on. In these settings, the task loss needs to be carefully balanced with the prediction loss because they may have conflicting objectives. We propose take the task loss signal one level deeper than the parameters of the model and use it to learn the parameters of the loss function the model is trained on, which can be done by learning a metric in the prediction space. This approach does not alter the optimal prediction model itself, but rather changes the model learning to emphasize the information important for the downstream task. This enables us to achieve the best of both worlds: a prediction model trained in the original prediction space while also being valuable for the desired downstream task. We validate our approach through experiments conducted in two main settings: 1) decision-focused model learning scenarios involving portfolio optimization and budget allocation, and 2) reinforcement learning in noisy environments with distracting states. The source code to reproduce our experiments is available at https://github.com/facebookresearch/taskmet

$f$-Cal: Calibrated aleatoric uncertainty estimation from neural networks for robot perception

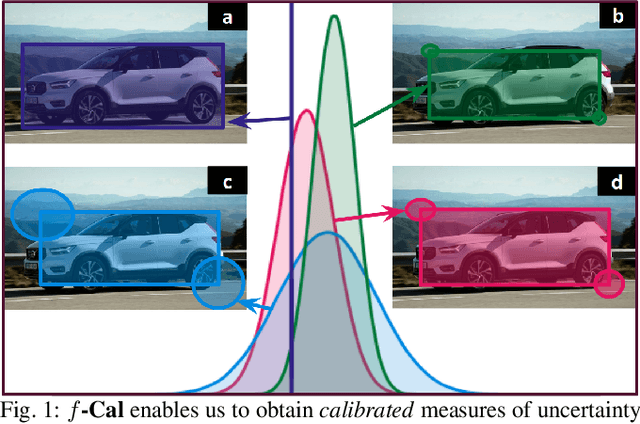
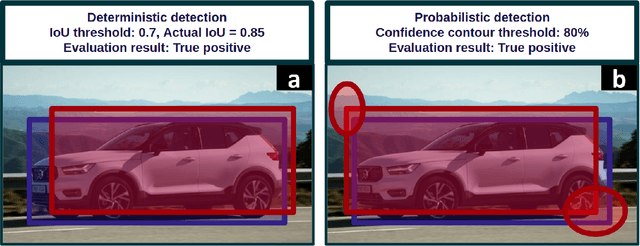
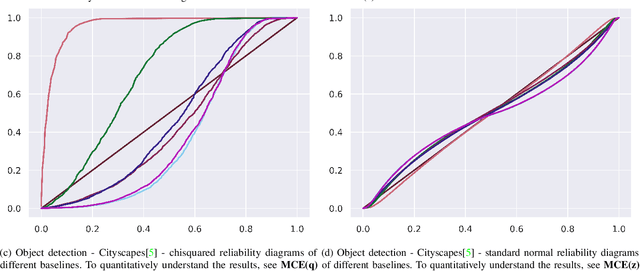
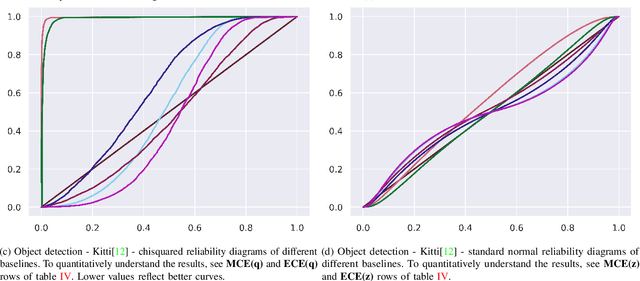
While modern deep neural networks are performant perception modules, performance (accuracy) alone is insufficient, particularly for safety-critical robotic applications such as self-driving vehicles. Robot autonomy stacks also require these otherwise blackbox models to produce reliable and calibrated measures of confidence on their predictions. Existing approaches estimate uncertainty from these neural network perception stacks by modifying network architectures, inference procedure, or loss functions. However, in general, these methods lack calibration, meaning that the predictive uncertainties do not faithfully represent the true underlying uncertainties (process noise). Our key insight is that calibration is only achieved by imposing constraints across multiple examples, such as those in a mini-batch; as opposed to existing approaches which only impose constraints per-sample, often leading to overconfident (thus miscalibrated) uncertainty estimates. By enforcing the distribution of outputs of a neural network to resemble a target distribution by minimizing an $f$-divergence, we obtain significantly better-calibrated models compared to prior approaches. Our approach, $f$-Cal, outperforms existing uncertainty calibration approaches on robot perception tasks such as object detection and monocular depth estimation over multiple real-world benchmarks.