Slice-100K: A Multimodal Dataset for Extrusion-based 3D Printing

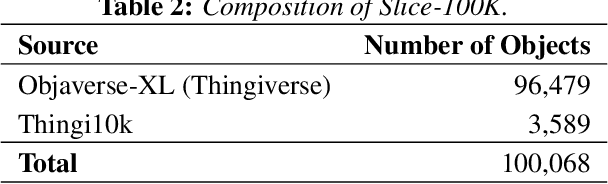
G-code (Geometric code) or RS-274 is the most widely used computer numerical control (CNC) and 3D printing programming language. G-code provides machine instructions for the movement of the 3D printer, especially for the nozzle, stage, and extrusion of material for extrusion-based additive manufacturing. Currently there does not exist a large repository of curated CAD models along with their corresponding G-code files for additive manufacturing. To address this issue, we present SLICE-100K, a first-of-its-kind dataset of over 100,000 G-code files, along with their tessellated CAD model, LVIS (Large Vocabulary Instance Segmentation) categories, geometric properties, and renderings. We build our dataset from triangulated meshes derived from Objaverse-XL and Thingi10K datasets. We demonstrate the utility of this dataset by finetuning GPT-2 on a subset of the dataset for G-code translation from a legacy G-code format (Sailfish) to a more modern, widely used format (Marlin). SLICE-100K will be the first step in developing a multimodal foundation model for digital manufacturing.

Evaluating NeRFs for 3D Plant Geometry Reconstruction in Field Conditions

We evaluate different Neural Radiance Fields (NeRFs) techniques for reconstructing (3D) plants in varied environments, from indoor settings to outdoor fields. Traditional techniques often struggle to capture the complex details of plants, which is crucial for botanical and agricultural understanding. We evaluate three scenarios with increasing complexity and compare the results with the point cloud obtained using LiDAR as ground truth data. In the most realistic field scenario, the NeRF models achieve a 74.65% F1 score with 30 minutes of training on the GPU, highlighting the efficiency and accuracy of NeRFs in challenging environments. These findings not only demonstrate the potential of NeRF in detailed and realistic 3D plant modeling but also suggest practical approaches for enhancing the speed and efficiency of the 3D reconstruction process.

Latent Diffusion Models for Structural Component Design

Recent advances in generative modeling, namely Diffusion models, have revolutionized generative modeling, enabling high-quality image generation tailored to user needs. This paper proposes a framework for the generative design of structural components. Specifically, we employ a Latent Diffusion model to generate potential designs of a component that can satisfy a set of problem-specific loading conditions. One of the distinct advantages our approach offers over other generative approaches, such as generative adversarial networks (GANs), is that it permits the editing of existing designs. We train our model using a dataset of geometries obtained from structural topology optimization utilizing the SIMP algorithm. Consequently, our framework generates inherently near-optimal designs. Our work presents quantitative results that support the structural performance of the generated designs and the variability in potential candidate designs. Furthermore, we provide evidence of the scalability of our framework by operating over voxel domains with resolutions varying from $32^3$ to $128^3$. Our framework can be used as a starting point for generating novel near-optimal designs similar to topology-optimized designs.

Towards Foundational AI Models for Additive Manufacturing: Language Models for G-Code Debugging, Manipulation, and Comprehension

3D printing or additive manufacturing is a revolutionary technology that enables the creation of physical objects from digital models. However, the quality and accuracy of 3D printing depend on the correctness and efficiency of the G-code, a low-level numerical control programming language that instructs 3D printers how to move and extrude material. Debugging G-code is a challenging task that requires a syntactic and semantic understanding of the G-code format and the geometry of the part to be printed. In this paper, we present the first extensive evaluation of six state-of-the-art foundational large language models (LLMs) for comprehending and debugging G-code files for 3D printing. We design effective prompts to enable pre-trained LLMs to understand and manipulate G-code and test their performance on various aspects of G-code debugging and manipulation, including detection and correction of common errors and the ability to perform geometric transformations. We analyze their strengths and weaknesses for understanding complete G-code files. We also discuss the implications and limitations of using LLMs for G-code comprehension.

ZeroForge: Feedforward Text-to-Shape Without 3D Supervision

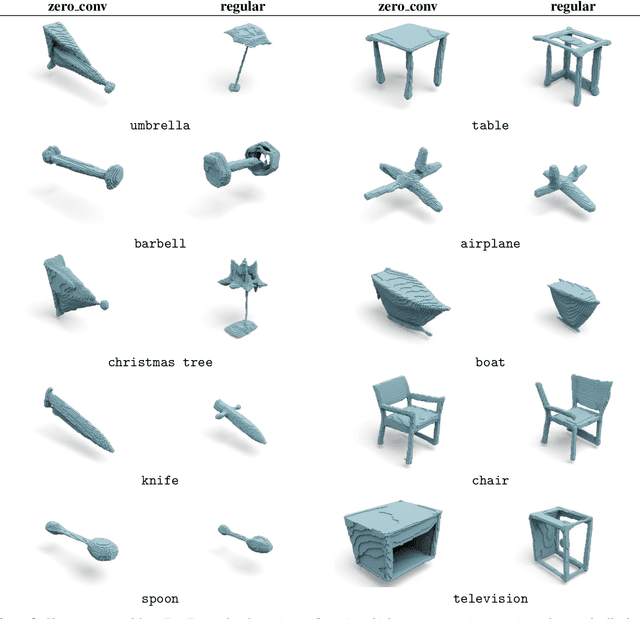
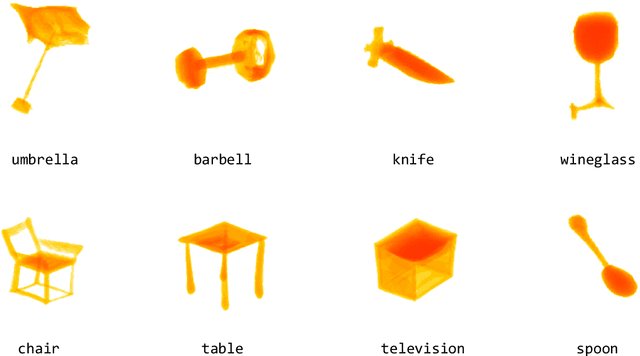
Current state-of-the-art methods for text-to-shape generation either require supervised training using a labeled dataset of pre-defined 3D shapes, or perform expensive inference-time optimization of implicit neural representations. In this work, we present ZeroForge, an approach for zero-shot text-to-shape generation that avoids both pitfalls. To achieve open-vocabulary shape generation, we require careful architectural adaptation of existing feed-forward approaches, as well as a combination of data-free CLIP-loss and contrastive losses to avoid mode collapse. Using these techniques, we are able to considerably expand the generative ability of existing feed-forward text-to-shape models such as CLIP-Forge. We support our method via extensive qualitative and quantitative evaluations

Neural PDE Solvers for Irregular Domains

Neural network-based approaches for solving partial differential equations (PDEs) have recently received special attention. However, the large majority of neural PDE solvers only apply to rectilinear domains, and do not systematically address the imposition of Dirichlet/Neumann boundary conditions over irregular domain boundaries. In this paper, we present a framework to neurally solve partial differential equations over domains with irregularly shaped (non-rectilinear) geometric boundaries. Our network takes in the shape of the domain as an input (represented using an unstructured point cloud, or any other parametric representation such as Non-Uniform Rational B-Splines) and is able to generalize to novel (unseen) irregular domains; the key technical ingredient to realizing this model is a novel approach for identifying the interior and exterior of the computational grid in a differentiable manner. We also perform a careful error analysis which reveals theoretical insights into several sources of error incurred in the model-building process. Finally, we showcase a wide variety of applications, along with favorable comparisons with ground truth solutions.