The Comparison of Riemannian Geometric Matrix-CFAR Signal Detectors

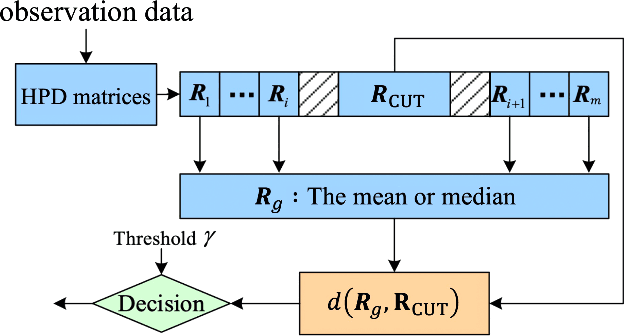
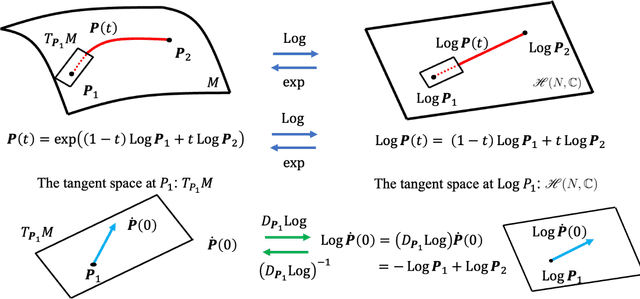
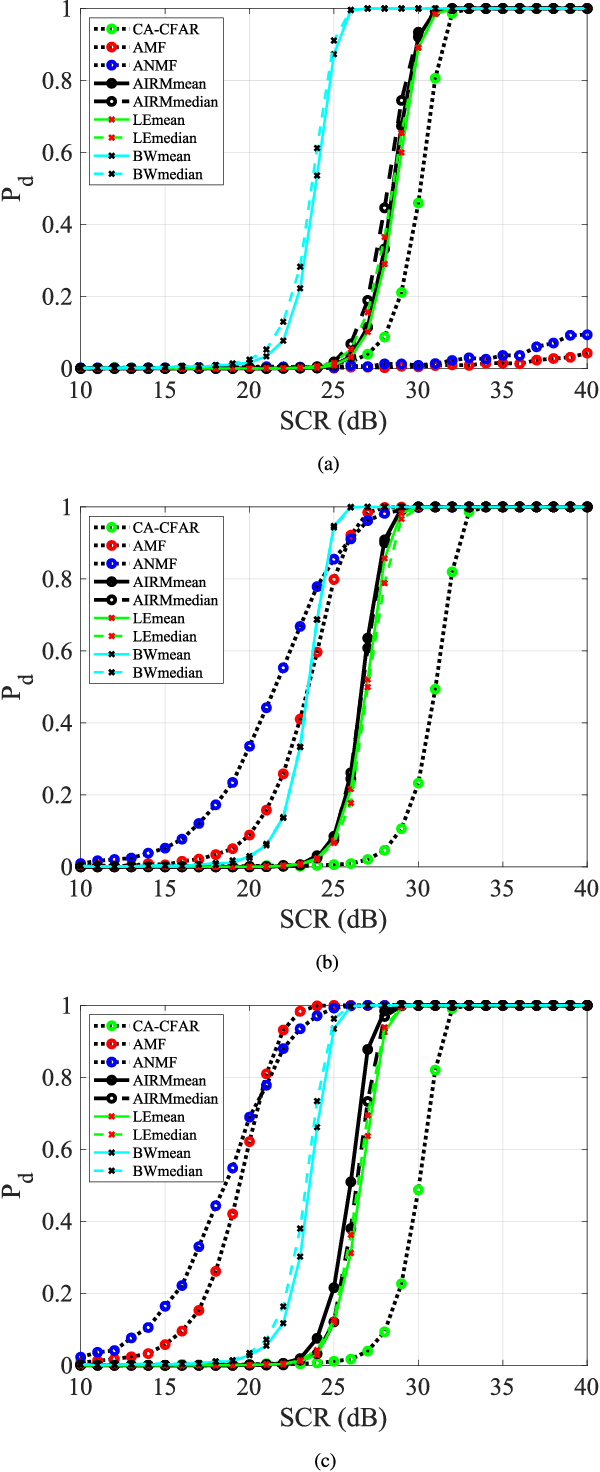
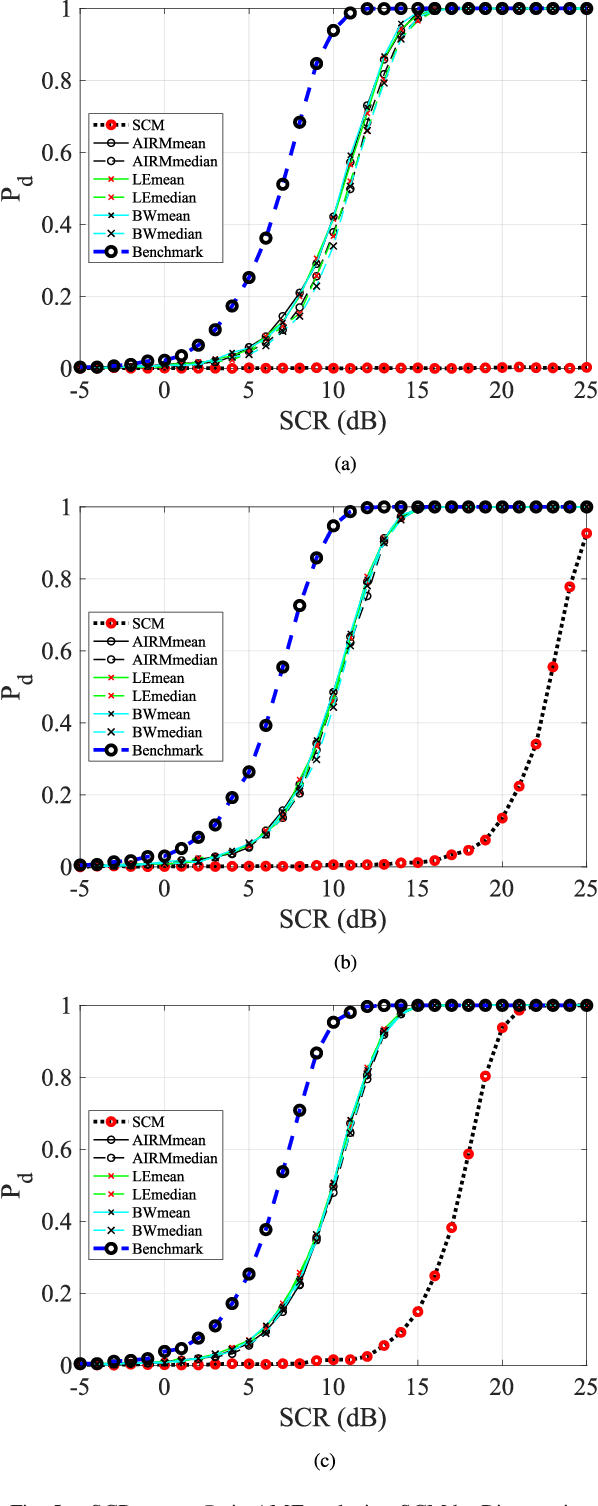
Essential characteristics of signal data can be captured by the autocovariance matrix, which, in the stationary scenarios, is Toeplitz Hermitian positive definite (HPD). In this paper, several well-known Riemannian geometric structures of HPD matrix manifolds are applied to signal detection, including the affine invariant Riemannian metric, the log-Euclidean metric, and the Bures--Wasserstein (BW) metric, the last of which was recently extended to HPD manifolds. Riemannian gradient descent algorithms are proposed to solve the corresponding geometric means and medians, that play fundamental roles in the detection process. Simulations within the scenario using the ideal steering vector as the target signal provide compelling evidence that the BW detectors outperform the other geometric detectors as well as the conventional adaptive matched filter and adaptive normalized matched filter when observation data are limited. Further simulations demonstrate that the matrix-CFAR is robust in scenarios where the signal is mismatched. In addition to detection performances, robustness of the geometric detectors to outliers and computational complexity of the algorithms are analysed.

LDA-MIG Detectors for Maritime Targets in Nonhomogeneous Sea Clutter

This paper deals with the problem of detecting maritime targets embedded in nonhomogeneous sea clutter, where limited number of secondary data is available due to the heterogeneity of sea clutter. A class of linear discriminant analysis (LDA)-based matrix information geometry (MIG) detectors is proposed in the supervised scenario. As customary, Hermitian positive-definite (HPD) matrices are used to model the observational sample data, and the clutter covariance matrix of received dataset is estimated as geometric mean of the secondary HPD matrices. Given a set of training HPD matrices with class labels, that are elements of a higher-dimensional HPD matrix manifold, the LDA manifold projection learns a mapping from the higher-dimensional HPD matrix manifold to a lower-dimensional one subject to maximum discrimination. In the current study, the LDA manifold projection, with the cost function maximizing between-class distance while minimizing within-class distance, is formulated as an optimization problem in the Stiefel manifold. Four robust LDA-MIG detectors corresponding to different geometric measures are proposed. Numerical results based on both simulated radar clutter with interferences and real IPIX radar data show the advantage of the proposed LDA-MIG detectors against their counterparts without using LDA as well as the state-of-art maritime target detection methods in nonhomogeneous sea clutter.

Towards a median signal detector through the total Bregman divergence and its robust analysis

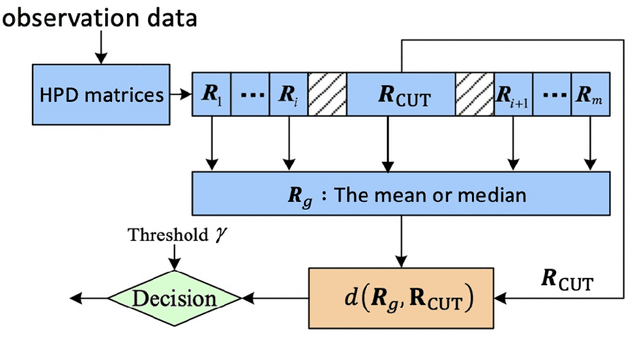
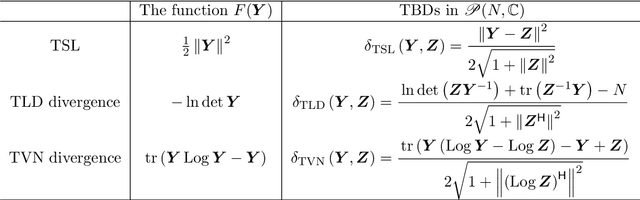
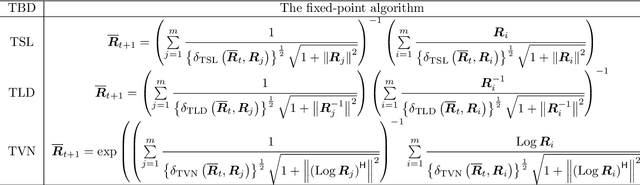
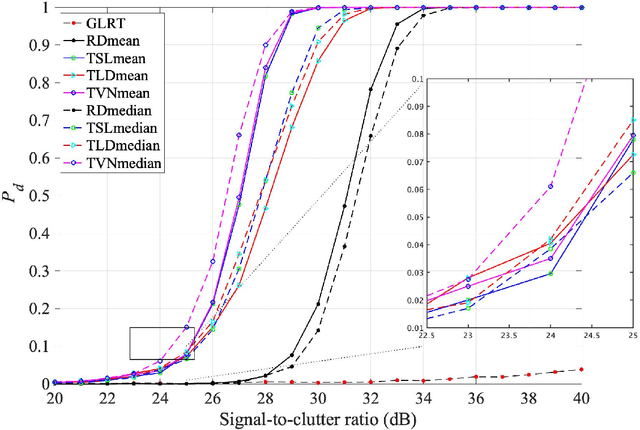
A novel family of geometric signal detectors are proposed through medians of the total Bregman divergence (TBD), which are shown advantageous over the conventional methods and their mean counterparts. By interpreting the observation data as Hermitian positive-definite (HPD) matrices, their mean or median play an essential role in signal detection. As is difficult to be solved analytically, we propose numerical solutions through Riemannian gradient descent algorithms or fixed-point algorithms. Beside detection performance, robustness of a detector to outliers is also of vital importance, which can often be analyzed via the influence functions. Introducing an orthogonal basis for Hermitian matrices, we are able to compute the corresponding influence functions analytically and exactly by solving a linear system, which is transformed from the governing matrix equation. Numerical simulations show that the TBD medians are more robust than their mean counterparts.

Unsupervised Learning Discriminative MIG Detectors in Nonhomogeneous Clutter

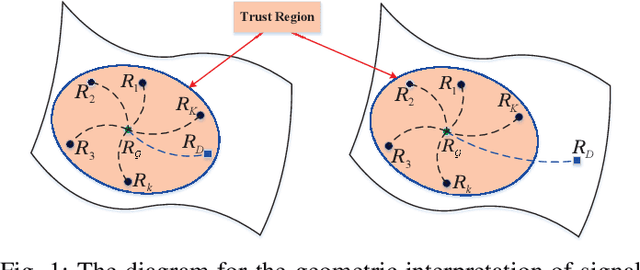
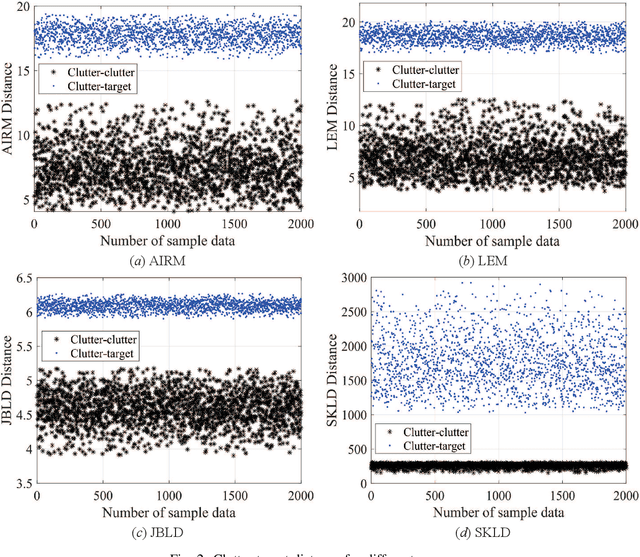
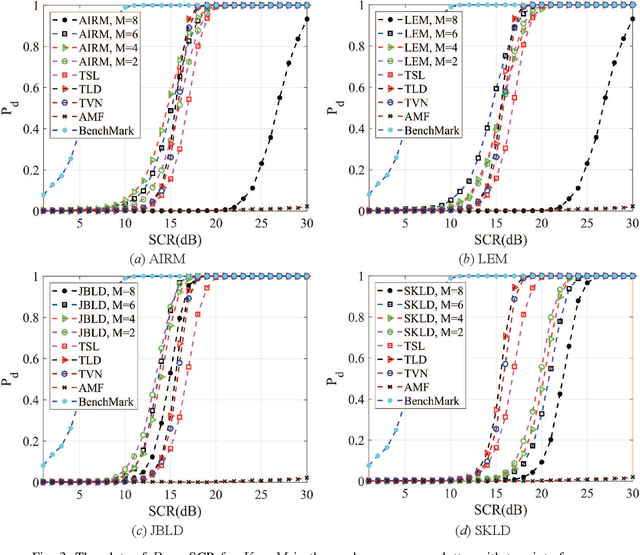
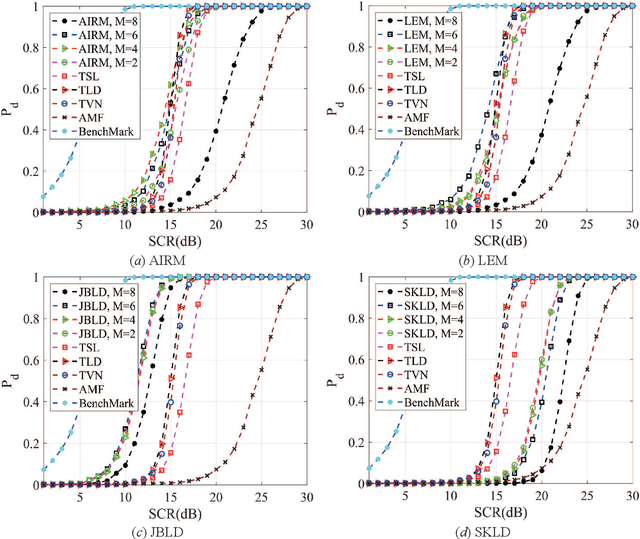
Principal component analysis (PCA) is a common used pattern analysis method that maps high-dimensional data into a lower-dimensional space maximizing the data variance, that results in the promotion of separability of data. Inspired by the principle of PCA, a novel type of learning discriminative matrix information geometry (MIG) detectors in the unsupervised scenario are developed, and applied to signal detection in nonhomogeneous environments. Hermitian positive-definite (HPD) matrices can be used to model the sample data, while the clutter covariance matrix is estimated by the geometric mean of a set of secondary HPD matrices. We define a projection that maps the HPD matrices in a high-dimensional manifold to a low-dimensional and more discriminative one to increase the degree of separation of HPD matrices by maximizing the data variance. Learning a mapping can be formulated as a two-step mini-max optimization problem in Riemannian manifolds, which can be solved by the Riemannian gradient descent algorithm. Three discriminative MIG detectors are illustrated with respect to different geometric measures, i.e., the Log-Euclidean metric, the Jensen--Bregman LogDet divergence and the symmetrized Kullback--Leibler divergence. Simulation results show that performance improvements of the novel MIG detectors can be achieved compared with the conventional detectors and their state-of-the-art counterparts within nonhomogeneous environments.

MIG Median Detectors with Manifold Filter

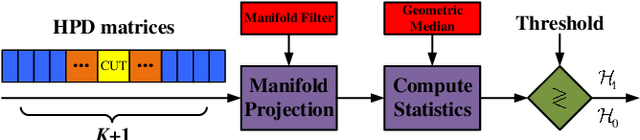
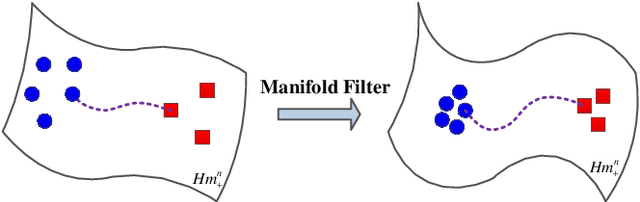
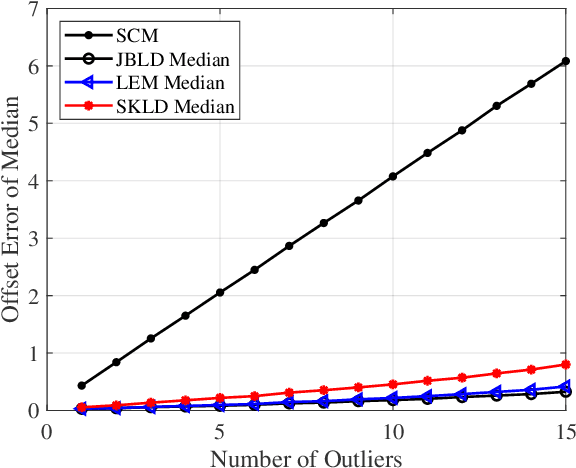
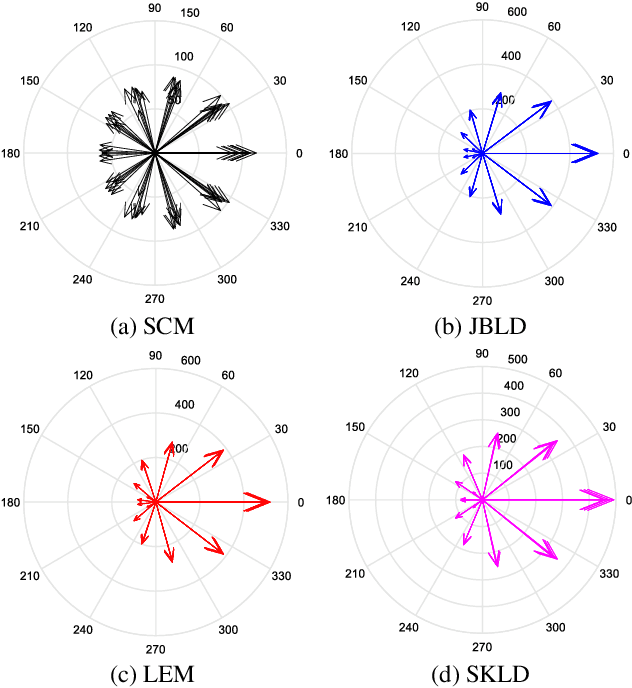
In this paper, we propose a class of median-based matrix information geometry (MIG) detectors with a manifold filter and apply them to signal detection in nonhomogeneous environments. As customary, the sample data is assumed to be modeled as Hermitian positive-definite (HPD) matrices, and the geometric median of a set of HPD matrices is interpreted as an estimate of the clutter covariance matrix (CCM). Then, the problem of signal detection can be reformulated as discriminating two points on the manifold of HPD matrices, one of which is the HPD matrix in the cell under test while the other represents the CCM. By manifold filter, we map a set of HPD matrices to another set of HPD matrices by weighting them, that consequently improves the discriminative power by reducing the intra-class distances while increasing the inter-class distances. Three MIG median detectors are designed by resorting to three geometric measures on the matrix manifold, and the corresponding geometric medians are shown to be robust to outliers. Numerical simulations show the advantage of the proposed MIG median detectors in comparison with their state-of-the-art counterparts as well as the conventional detectors in nonhomogeneous environments.

Target Detection within Nonhomogeneous Clutter via Total Bregman Divergence-Based Matrix Information Geometry Detectors

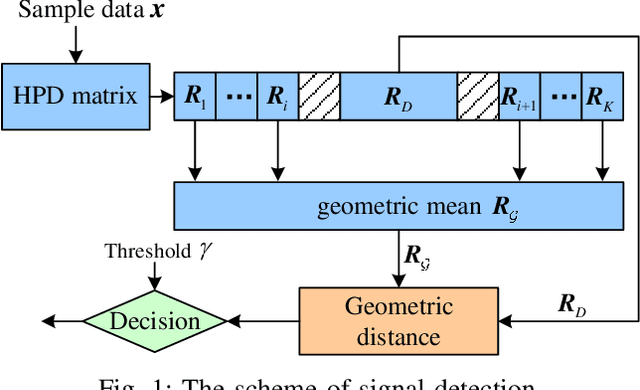
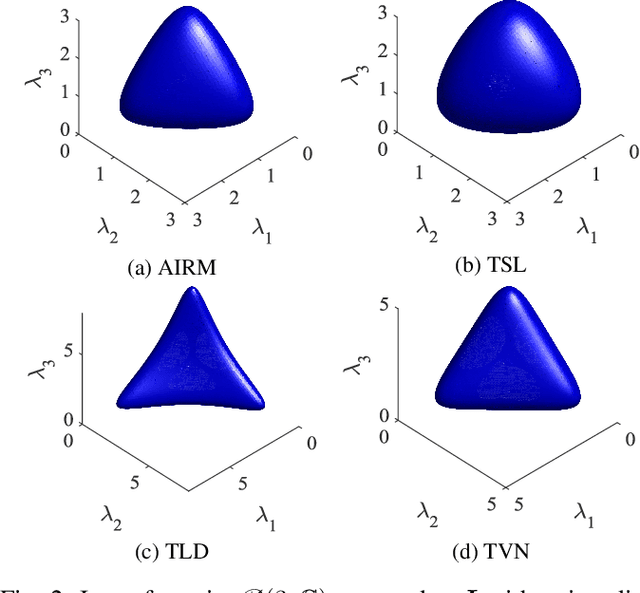
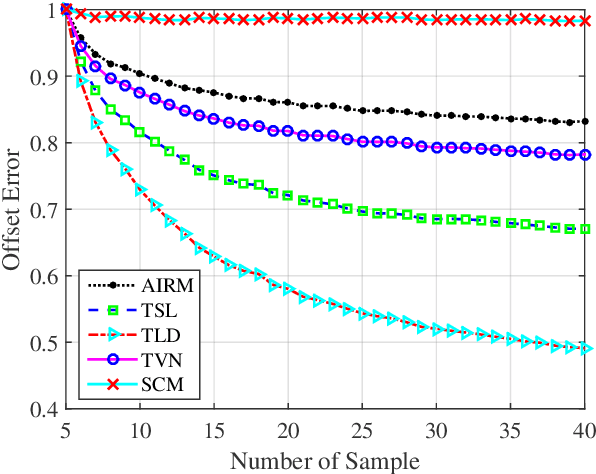
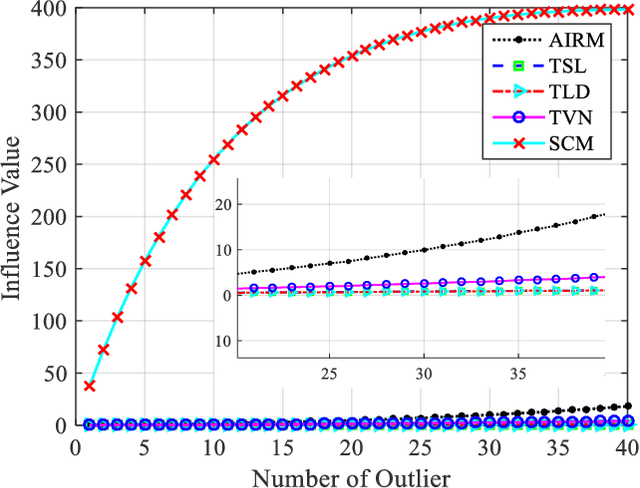
Information divergences are commonly used to measure the dissimilarity of two elements on a statistical manifold. Differentiable manifolds endowed with different divergences may possess different geometric properties, which can result in totally different performances in many practical applications. In this paper, we propose a total Bregman divergence-based matrix information geometry (TBD-MIG) detector and apply it to detect targets emerged into nonhomogeneous clutter. In particular, each sample data is assumed to be modeled as a Hermitian positive-definite (HPD) matrix and the clutter covariance matrix is estimated by the TBD mean of a set of secondary HPD matrices. We then reformulate the problem of signal detection as discriminating two points on the HPD matrix manifold. Three TBD-MIG detectors, referred to as the total square loss, the total log-determinant and the total von Neumann MIG detectors, are proposed, and they can achieve great performances due to their power of discrimination and robustness to interferences. Simulations show the advantage of the proposed TBD-MIG detectors in comparison with the geometric detector using an affine invariant Riemannian metric as well as the adaptive matched filter in nonhomogeneous clutter.