On Moving Object Segmentation from Monocular Video with Transformers

Moving object detection and segmentation from a single moving camera is a challenging task, requiring an understanding of recognition, motion and 3D geometry. Combining both recognition and reconstruction boils down to a fusion problem, where appearance and motion features need to be combined for classification and segmentation. In this paper, we present a novel fusion architecture for monocular motion segmentation - M3Former, which leverages the strong performance of transformers for segmentation and multi-modal fusion. As reconstructing motion from monocular video is ill-posed, we systematically analyze different 2D and 3D motion representations for this problem and their importance for segmentation performance. Finally, we analyze the effect of training data and show that diverse datasets are required to achieve SotA performance on Kitti and Davis.

DROID-Splat: Combining end-to-end SLAM with 3D Gaussian Splatting

Recent progress in scene synthesis makes standalone SLAM systems purely based on optimizing hyperprimitives with a Rendering objective possible \cite{monogs}. However, the tracking performance still lacks behind traditional \cite{orbslam} and end-to-end SLAM systems \cite{droid}. An optimal trade-off between robustness, speed and accuracy has not yet been reached, especially for monocular video. In this paper, we introduce a SLAM system based on an end-to-end Tracker and extend it with a Renderer based on recent 3D Gaussian Splatting techniques. Our framework \textbf{DroidSplat} achieves both SotA tracking and rendering results on common SLAM benchmarks. We implemented multiple building blocks of modern SLAM systems to run in parallel, allowing for fast inference on common consumer GPU's. Recent progress in monocular depth prediction and camera calibration allows our system to achieve strong results even on in-the-wild data without known camera intrinsics. Code will be available at \url{https://github.com/ChenHoy/DROID-Splat}.

Sigma Flows for Image and Data Labeling and Learning Structured Prediction

This paper introduces the sigma flow model for the prediction of structured labelings of data observed on Riemannian manifolds, including Euclidean image domains as special case. The approach combines the Laplace-Beltrami framework for image denoising and enhancement, introduced by Sochen, Kimmel and Malladi about 25 years ago, and the assignment flow approach introduced and studied by the authors. The sigma flow arises as Riemannian gradient flow of generalized harmonic energies and thus is governed by a nonlinear geometric PDE which determines a harmonic map from a closed Riemannian domain manifold to a statistical manifold, equipped with the Fisher-Rao metric from information geometry. A specific ingredient of the sigma flow is the mutual dependency of the Riemannian metric of the domain manifold on the evolving state. This makes the approach amenable to machine learning in a specific way, by realizing this dependency through a mapping with compact time-variant parametrization that can be learned from data. Proof of concept experiments demonstrate the expressivity of the sigma flow model and prediction performance. Structural similarities to transformer network architectures and networks generated by the geometric integration of sigma flows are pointed out, which highlights the connection to deep learning and, conversely, may stimulate the use of geometric design principles for structured prediction in other areas of scientific machine learning.

Learning Distances from Data with Normalizing Flows and Score Matching

Density-based distances (DBDs) offer an elegant solution to the problem of metric learning. By defining a Riemannian metric which increases with decreasing probability density, shortest paths naturally follow the data manifold and points are clustered according to the modes of the data. We show that existing methods to estimate Fermat distances, a particular choice of DBD, suffer from poor convergence in both low and high dimensions due to i) inaccurate density estimates and ii) reliance on graph-based paths which are increasingly rough in high dimensions. To address these issues, we propose learning the densities using a normalizing flow, a generative model with tractable density estimation, and employing a smooth relaxation method using a score model initialized from a graph-based proposal. Additionally, we introduce a dimension-adapted Fermat distance that exhibits more intuitive behavior when scaled to high dimensions and offers better numerical properties. Our work paves the way for practical use of density-based distances, especially in high-dimensional spaces.

Generative Assignment Flows for Representing and Learning Joint Distributions of Discrete Data

We introduce a novel generative model for the representation of joint probability distributions of a possibly large number of discrete random variables. The approach uses measure transport by randomized assignment flows on the statistical submanifold of factorizing distributions, which also enables to sample efficiently from the target distribution and to assess the likelihood of unseen data points. The embedding of the flow via the Segre map in the meta-simplex of all discrete joint distributions ensures that any target distribution can be represented in principle, whose complexity in practice only depends on the parametrization of the affinity function of the dynamical assignment flow system. Our model can be trained in a simulation-free manner without integration by conditional Riemannian flow matching, using the training data encoded as geodesics in closed-form with respect to the e-connection of information geometry. By projecting high-dimensional flow matching in the meta-simplex of joint distributions to the submanifold of factorizing distributions, our approach has strong motivation from first principles of modeling coupled discrete variables. Numerical experiments devoted to distributions of structured image labelings demonstrate the applicability to large-scale problems, which may include discrete distributions in other application areas. Performance measures show that our approach scales better with the increasing number of classes than recent related work.

The Central Spanning Tree Problem

Spanning trees are an important primitive in many data analysis tasks, when a data set needs to be summarized in terms of its "skeleton", or when a tree-shaped graph over all observations is required for downstream processing. Popular definitions of spanning trees include the minimum spanning tree and the optimum distance spanning tree, a.k.a. the minimum routing cost tree. When searching for the shortest spanning tree but admitting additional branching points, even shorter spanning trees can be realized: Steiner trees. Unfortunately, both minimum spanning and Steiner trees are not robust with respect to noise in the observations; that is, small perturbations of the original data set often lead to drastic changes in the associated spanning trees. In response, we make two contributions when the data lies in a Euclidean space: on the theoretical side, we introduce a new optimization problem, the "(branched) central spanning tree", which subsumes all previously mentioned definitions as special cases. On the practical side, we show empirically that the (branched) central spanning tree is more robust to noise in the data, and as such is better suited to summarize a data set in terms of its skeleton. We also propose a heuristic to address the NP-hard optimization problem, and illustrate its use on single cell RNA expression data from biology and 3D point clouds of plants.

Generative Modeling of Discrete Joint Distributions by E-Geodesic Flow Matching on Assignment Manifolds

This paper introduces a novel generative model for discrete distributions based on continuous normalizing flows on the submanifold of factorizing discrete measures. Integration of the flow gradually assigns categories and avoids issues of discretizing the latent continuous model like rounding, sample truncation etc. General non-factorizing discrete distributions capable of representing complex statistical dependencies of structured discrete data, can be approximated by embedding the submanifold into a the meta-simplex of all joint discrete distributions and data-driven averaging. Efficient training of the generative model is demonstrated by matching the flow of geodesics of factorizing discrete distributions. Various experiments underline the approach's broad applicability.

On the Universality of Coupling-based Normalizing Flows

We present a novel theoretical framework for understanding the expressive power of coupling-based normalizing flows such as RealNVP. Despite their prevalence in scientific applications, a comprehensive understanding of coupling flows remains elusive due to their restricted architectures. Existing theorems fall short as they require the use of arbitrarily ill-conditioned neural networks, limiting practical applicability. Additionally, we demonstrate that these constructions inherently lead to volume-preserving flows, a property which we show to be a fundamental constraint for expressivity. We propose a new distributional universality theorem for coupling-based normalizing flows, which overcomes several limitations of prior work. Our results support the general wisdom that the coupling architecture is expressive and provide a nuanced view for choosing the expressivity of coupling functions, bridging a gap between empirical results and theoretical understanding.

Quantum State Assignment Flows

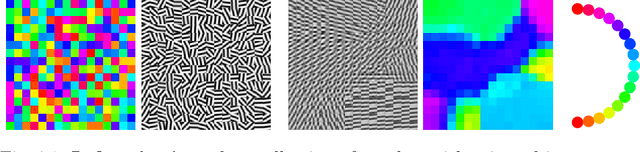
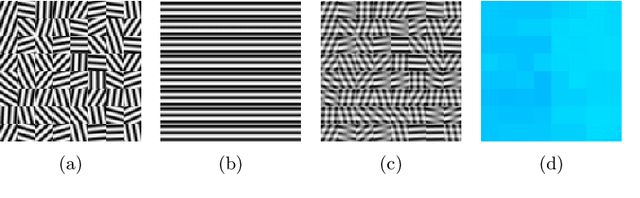
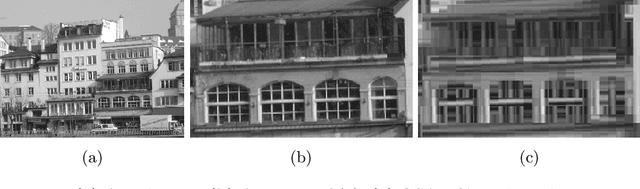
This paper introduces assignment flows for density matrices as state spaces for representing and analyzing data associated with vertices of an underlying weighted graph. Determining an assignment flow by geometric integration of the defining dynamical system causes an interaction of the non-commuting states across the graph, and the assignment of a pure (rank-one) state to each vertex after convergence. Adopting the Riemannian Bogoliubov-Kubo-Mori metric from information geometry leads to closed-form local expressions which can be computed efficiently and implemented in a fine-grained parallel manner. Restriction to the submanifold of commuting density matrices recovers the assignment flows for categorial probability distributions, which merely assign labels from a finite set to each data point. As shown for these flows in our prior work, the novel class of quantum state assignment flows can also be characterized as Riemannian gradient flows with respect to a non-local non-convex potential, after proper reparametrization and under mild conditions on the underlying weight function. This weight function generates the parameters of the layers of a neural network, corresponding to and generated by each step of the geometric integration scheme. Numerical results indicates and illustrate the potential of the novel approach for data representation and analysis, including the representation of correlations of data across the graph by entanglement and tensorization.

On the Convergence Rate of Gaussianization with Random Rotations

Gaussianization is a simple generative model that can be trained without backpropagation. It has shown compelling performance on low dimensional data. As the dimension increases, however, it has been observed that the convergence speed slows down. We show analytically that the number of required layers scales linearly with the dimension for Gaussian input. We argue that this is because the model is unable to capture dependencies between dimensions. Empirically, we find the same linear increase in cost for arbitrary input $p(x)$, but observe favorable scaling for some distributions. We explore potential speed-ups and formulate challenges for further research.