Sigma Flows for Image and Data Labeling and Learning Structured Prediction

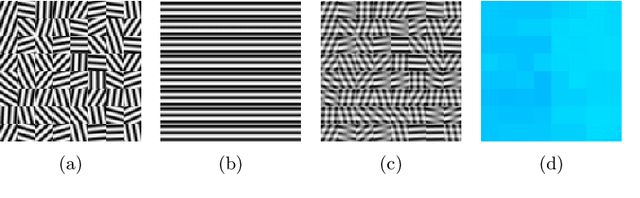
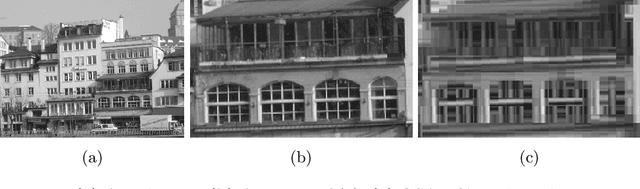
This paper introduces the sigma flow model for the prediction of structured labelings of data observed on Riemannian manifolds, including Euclidean image domains as special case. The approach combines the Laplace-Beltrami framework for image denoising and enhancement, introduced by Sochen, Kimmel and Malladi about 25 years ago, and the assignment flow approach introduced and studied by the authors. The sigma flow arises as Riemannian gradient flow of generalized harmonic energies and thus is governed by a nonlinear geometric PDE which determines a harmonic map from a closed Riemannian domain manifold to a statistical manifold, equipped with the Fisher-Rao metric from information geometry. A specific ingredient of the sigma flow is the mutual dependency of the Riemannian metric of the domain manifold on the evolving state. This makes the approach amenable to machine learning in a specific way, by realizing this dependency through a mapping with compact time-variant parametrization that can be learned from data. Proof of concept experiments demonstrate the expressivity of the sigma flow model and prediction performance. Structural similarities to transformer network architectures and networks generated by the geometric integration of sigma flows are pointed out, which highlights the connection to deep learning and, conversely, may stimulate the use of geometric design principles for structured prediction in other areas of scientific machine learning.

Quantum State Assignment Flows

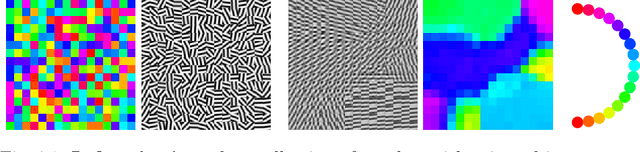
This paper introduces assignment flows for density matrices as state spaces for representing and analyzing data associated with vertices of an underlying weighted graph. Determining an assignment flow by geometric integration of the defining dynamical system causes an interaction of the non-commuting states across the graph, and the assignment of a pure (rank-one) state to each vertex after convergence. Adopting the Riemannian Bogoliubov-Kubo-Mori metric from information geometry leads to closed-form local expressions which can be computed efficiently and implemented in a fine-grained parallel manner. Restriction to the submanifold of commuting density matrices recovers the assignment flows for categorial probability distributions, which merely assign labels from a finite set to each data point. As shown for these flows in our prior work, the novel class of quantum state assignment flows can also be characterized as Riemannian gradient flows with respect to a non-local non-convex potential, after proper reparametrization and under mild conditions on the underlying weight function. This weight function generates the parameters of the layers of a neural network, corresponding to and generated by each step of the geometric integration scheme. Numerical results indicates and illustrate the potential of the novel approach for data representation and analysis, including the representation of correlations of data across the graph by entanglement and tensorization.