Cyclic Supports in Recursive Bipolar Argumentation Frameworks: Semantics and LP Mapping

Dung's Abstract Argumentation Framework (AF) has emerged as a key formalism for argumentation in Artificial Intelligence. It has been extended in several directions, including the possibility to express supports, leading to the development of the Bipolar Argumentation Framework (BAF), and recursive attacks and supports, resulting in the Recursive BAF (Rec-BAF). Different interpretations of supports have been proposed, whereas for Rec-BAF (where the target of attacks and supports may also be attacks and supports) even different semantics for attacks have been defined. However, the semantics of these frameworks have either not been defined in the presence of support cycles, or are often quite intricate in terms of the involved definitions. We encompass this limitation and present classical semantics for general BAF and Rec-BAF and show that the semantics for specific BAF and Rec-BAF frameworks can be defined by very simple and intuitive modifications of that defined for the case of AF. This is achieved by providing a modular definition of the sets of defeated and acceptable elements for each AF-based framework. We also characterize, in an elegant and uniform way, the semantics of general BAF and Rec-BAF in terms of logic programming and partial stable model semantics.

Counterfactual and Semifactual Explanations in Abstract Argumentation: Formal Foundations, Complexity and Computation

Explainable Artificial Intelligence and Formal Argumentation have received significant attention in recent years. Argumentation-based systems often lack explainability while supporting decision-making processes. Counterfactual and semifactual explanations are interpretability techniques that provide insights into the outcome of a model by generating alternative hypothetical instances. While there has been important work on counterfactual and semifactual explanations for Machine Learning models, less attention has been devoted to these kinds of problems in argumentation. In this paper, we explore counterfactual and semifactual reasoning in abstract Argumentation Framework. We investigate the computational complexity of counterfactual- and semifactual-based reasoning problems, showing that they are generally harder than classical argumentation problems such as credulous and skeptical acceptance. Finally, we show that counterfactual and semifactual queries can be encoded in weak-constrained Argumentation Framework, and provide a computational strategy through ASP solvers.

Even-if Explanations: Formal Foundations, Priorities and Complexity

EXplainable AI has received significant attention in recent years. Machine learning models often operate as black boxes, lacking explainability and transparency while supporting decision-making processes. Local post-hoc explainability queries attempt to answer why individual inputs are classified in a certain way by a given model. While there has been important work on counterfactual explanations, less attention has been devoted to semifactual ones. In this paper, we focus on local post-hoc explainability queries within the semifactual `even-if' thinking and their computational complexity among different classes of models, and show that both linear and tree-based models are strictly more interpretable than neural networks. After this, we introduce a preference-based framework that enables users to personalize explanations based on their preferences, both in the case of semifactuals and counterfactuals, enhancing interpretability and user-centricity. Finally, we explore the complexity of several interpretability problems in the proposed preference-based framework and provide algorithms for polynomial cases.

Proceedings 36th International Conference on Logic Programming (Technical Communications)

Since the first conference held in Marseille in 1982, ICLP has been the premier international event for presenting research in logic programming. Contributions are solicited in all areas of logic programming and related areas, including but not restricted to: - Foundations: Semantics, Formalisms, Answer-Set Programming, Non-monotonic Reasoning, Knowledge Representation. - Declarative Programming: Inference engines, Analysis, Type and mode inference, Partial evaluation, Abstract interpretation, Transformation, Validation, Verification, Debugging, Profiling, Testing, Logic-based domain-specific languages, constraint handling rules. - Related Paradigms and Synergies: Inductive and Co-inductive Logic Programming, Constraint Logic Programming, Interaction with SAT, SMT and CSP solvers, Logic programming techniques for type inference and theorem proving, Argumentation, Probabilistic Logic Programming, Relations to object-oriented and Functional programming, Description logics, Neural-Symbolic Machine Learning, Hybrid Deep Learning and Symbolic Reasoning. - Implementation: Concurrency and distribution, Objects, Coordination, Mobility, Virtual machines, Compilation, Higher Order, Type systems, Modules, Constraint handling rules, Meta-programming, Foreign interfaces, User interfaces. - Applications: Databases, Big Data, Data Integration and Federation, Software Engineering, Natural Language Processing, Web and Semantic Web, Agents, Artificial Intelligence, Bioinformatics, Education, Computational life sciences, Education, Cybersecurity, and Robotics.

On the Semantics of Abstract Argumentation Frameworks: A Logic Programming Approach

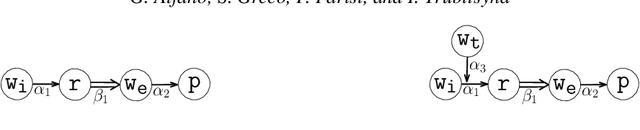
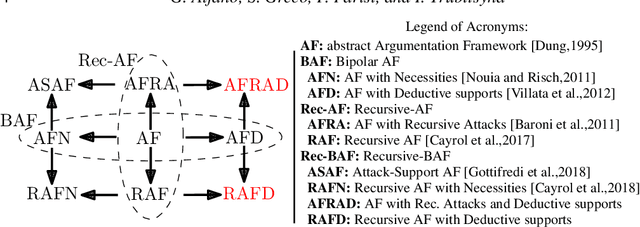
Recently there has been an increasing interest in frameworks extending Dung's abstract Argumentation Framework (AF). Popular extensions include bipolar AFs and AFs with recursive attacks and necessary supports. Although the relationships between AF semantics and Partial Stable Models (PSMs) of logic programs has been deeply investigated, this is not the case for more general frameworks extending AF. In this paper we explore the relationships between AF-based frameworks and PSMs. We show that every AF-based framework $\Delta$ can be translated into a logic program $P_\Delta$ so that the extensions prescribed by different semantics of $\Delta$ coincide with subsets of the PSMs of $P_\Delta$. We provide a logic programming approach that characterizes, in an elegant and uniform way, the semantics of several AF-based frameworks. This result allows also to define the semantics for new AF-based frameworks, such as AFs with recursive attacks and recursive deductive supports. Under consideration for publication in Theory and Practice of Logic Programming.

Using Linear Constraints for Logic Program Termination Analysis

It is widely acknowledged that function symbols are an important feature in answer set programming, as they make modeling easier, increase the expressive power, and allow us to deal with infinite domains. The main issue with their introduction is that the evaluation of a program might not terminate and checking whether it terminates or not is undecidable. To cope with this problem, several classes of logic programs have been proposed where the use of function symbols is restricted but the program evaluation termination is guaranteed. Despite the significant body of work in this area, current approaches do not include many simple practical programs whose evaluation terminates. In this paper, we present the novel classes of rule-bounded and cycle-bounded programs, which overcome different limitations of current approaches by performing a more global analysis of how terms are propagated from the body to the head of rules. Results on the correctness, the complexity, and the expressivity of the proposed approach are provided.

Greedy Algorithms in Datalog

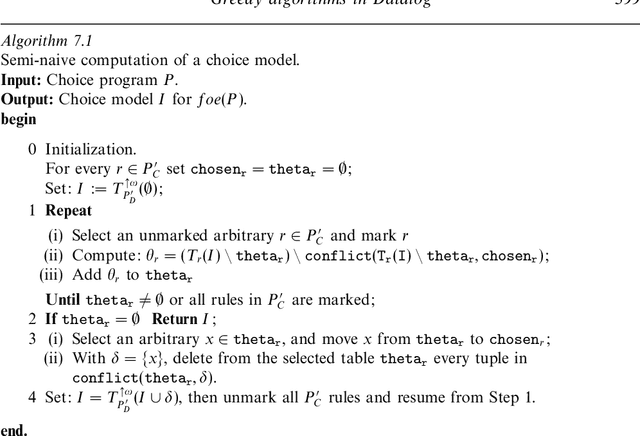
In the design of algorithms, the greedy paradigm provides a powerful tool for solving efficiently classical computational problems, within the framework of procedural languages. However, expressing these algorithms within the declarative framework of logic-based languages has proven a difficult research challenge. In this paper, we extend the framework of Datalog-like languages to obtain simple declarative formulations for such problems, and propose effective implementation techniques to ensure computational complexities comparable to those of procedural formulations. These advances are achieved through the use of the "choice" construct, extended with preference annotations to effect the selection of alternative stable-models and nondeterministic fixpoints. We show that, with suitable storage structures, the differential fixpoint computation of our programs matches the complexity of procedural algorithms in classical search and optimization problems.

Minimal founded semantics for disjunctive logic programs and deductive databases

In this paper, we propose a variant of stable model semantics for disjunctive logic programming and deductive databases. The semantics, called minimal founded, generalizes stable model semantics for normal (i.e. non disjunctive) programs but differs from disjunctive stable model semantics (the extension of stable model semantics for disjunctive programs). Compared with disjunctive stable model semantics, minimal founded semantics seems to be more intuitive, it gives meaning to programs which are meaningless under stable model semantics and is no harder to compute. More specifically, minimal founded semantics differs from stable model semantics only for disjunctive programs having constraint rules or rules working as constraints. We study the expressive power of the semantics and show that for general disjunctive datalog programs it has the same power as disjunctive stable model semantics.