Changhwa Lee
HOReeNet: 3D-aware Hand-Object Grasping Reenactment
Nov 11, 2022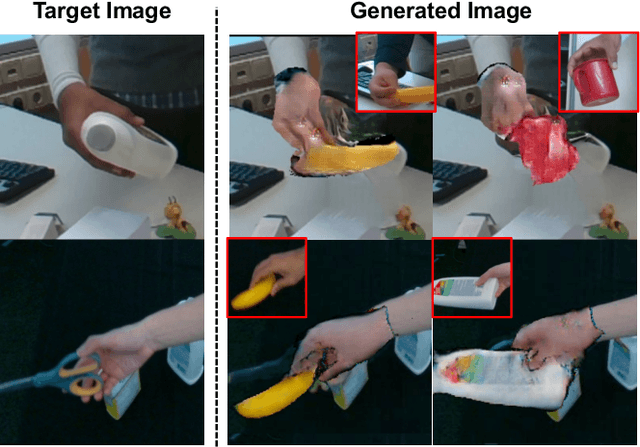
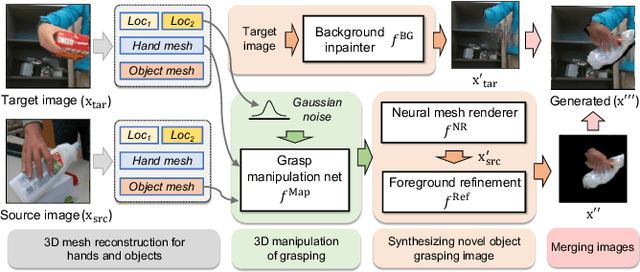

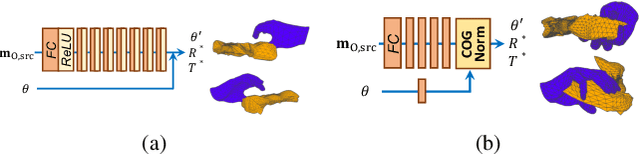
Abstract:We present HOReeNet, which tackles the novel task of manipulating images involving hands, objects, and their interactions. Especially, we are interested in transferring objects of source images to target images and manipulating 3D hand postures to tightly grasp the transferred objects. Furthermore, the manipulation needs to be reflected in the 2D image space. In our reenactment scenario involving hand-object interactions, 3D reconstruction becomes essential as 3D contact reasoning between hands and objects is required to achieve a tight grasp. At the same time, to obtain high-quality 2D images from 3D space, well-designed 3D-to-2D projection and image refinement are required. Our HOReeNet is the first fully differentiable framework proposed for such a task. On hand-object interaction datasets, we compared our HOReeNet to the conventional image translation algorithms and reenactment algorithm. We demonstrated that our approach could achieved the state-of-the-art on the proposed task.
Moment Multicalibration for Uncertainty Estimation
Aug 18, 2020Abstract:We show how to achieve the notion of "multicalibration" from H\'ebert-Johnson et al. [2018] not just for means, but also for variances and other higher moments. Informally, it means that we can find regression functions which, given a data point, can make point predictions not just for the expectation of its label, but for higher moments of its label distribution as well-and those predictions match the true distribution quantities when averaged not just over the population as a whole, but also when averaged over an enormous number of finely defined subgroups. It yields a principled way to estimate the uncertainty of predictions on many different subgroups-and to diagnose potential sources of unfairness in the predictive power of features across subgroups. As an application, we show that our moment estimates can be used to derive marginal prediction intervals that are simultaneously valid as averaged over all of the (sufficiently large) subgroups for which moment multicalibration has been obtained.
Fair Prediction with Endogenous Behavior
Feb 18, 2020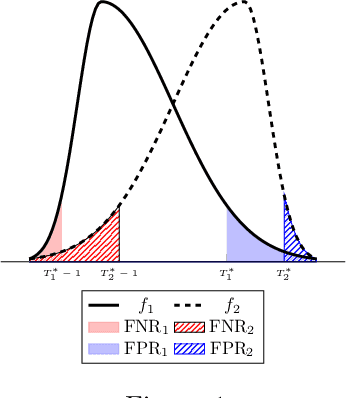
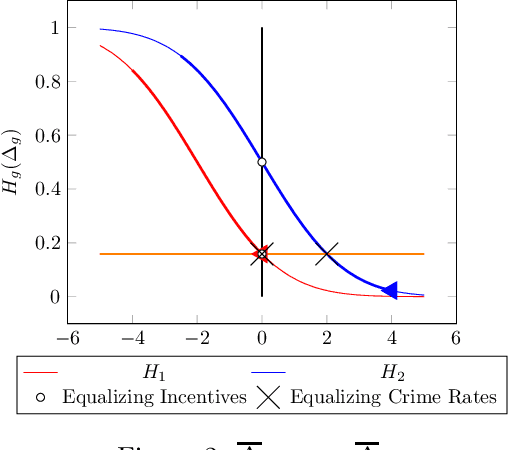
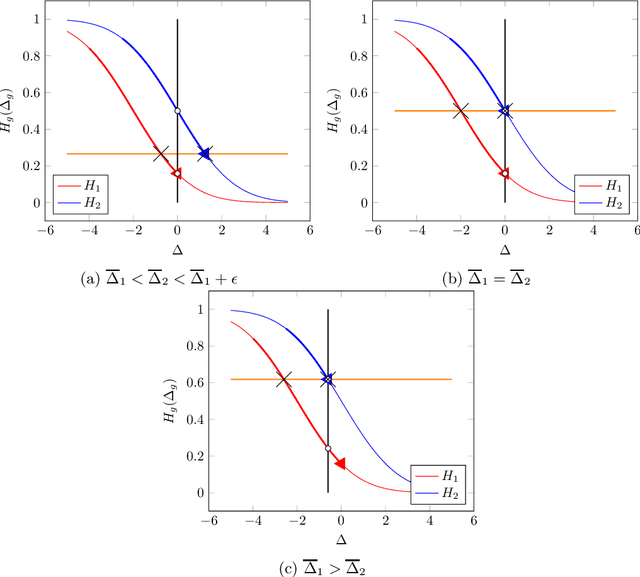
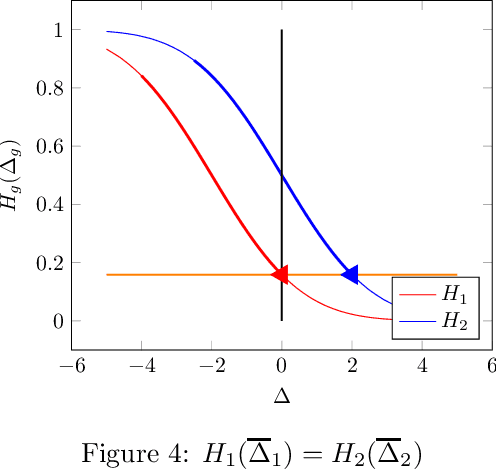
Abstract:There is increasing regulatory interest in whether machine learning algorithms deployed in consequential domains (e.g. in criminal justice) treat different demographic groups "fairly." However, there are several proposed notions of fairness, typically mutually incompatible. Using criminal justice as an example, we study a model in which society chooses an incarceration rule. Agents of different demographic groups differ in their outside options (e.g. opportunity for legal employment) and decide whether to commit crimes. We show that equalizing type I and type II errors across groups is consistent with the goal of minimizing the overall crime rate; other popular notions of fairness are not.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge