Persistent de Rham-Hodge Laplacians in the Eulerian representation

Recently, topological data analysis (TDA) has become a trending topic in data science and engineering. However, the key technique of TDA, i.e., persistent homology, is defined on point cloud data, which restricts its scope. In this work, we propose persistent de Rham-Hodge Laplacian, or persistent Hodge Laplacian (PHL) for abbreviation, for the TDA on manifolds with boundaries, or volumetric data. Specifically, we extended the evolutionary de Rham-Hodge theory from the Lagrangian formulation to the Eulerian formulation via structure-persevering Cartesian grids, and extended the persistent Laplacian on point clouds to persistent (de Rham-)Hodge Laplacian on nested families of manifolds with appropriate boundary conditions. The proposed PHL facilitates the machine learning and deep learning prediction of volumetric data. For a proof-of-principle application of the proposed PHL, we propose a persistent Hodge Laplacian learning (PHLL) algorithm for data on manifolds or volumetric data. To this end, we showcase the PHLL prediction of protein-ligand binding affinities in two benchmark datasets. Our numerical experiments highlight the power and promise of PHLL.

INFAMOUS-NeRF: ImproviNg FAce MOdeling Using Semantically-Aligned Hypernetworks with Neural Radiance Fields

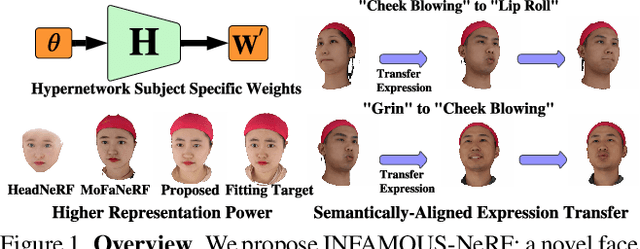
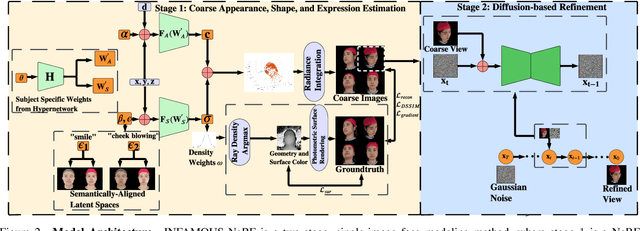
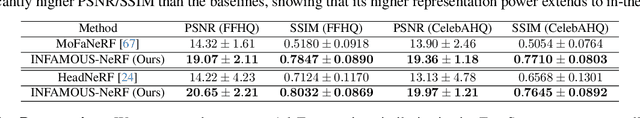
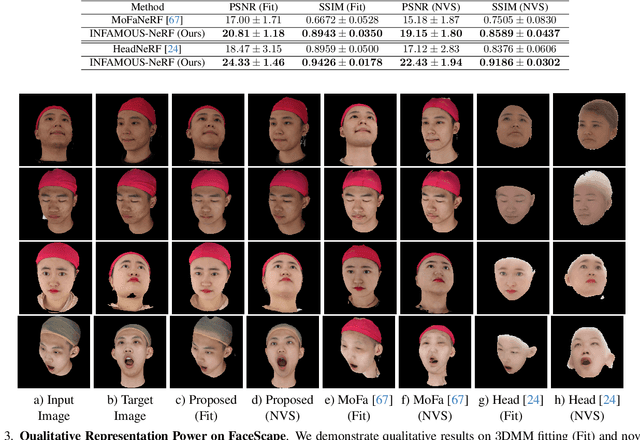
We propose INFAMOUS-NeRF, an implicit morphable face model that introduces hypernetworks to NeRF to improve the representation power in the presence of many training subjects. At the same time, INFAMOUS-NeRF resolves the classic hypernetwork tradeoff of representation power and editability by learning semantically-aligned latent spaces despite the subject-specific models, all without requiring a large pretrained model. INFAMOUS-NeRF further introduces a novel constraint to improve NeRF rendering along the face boundary. Our constraint can leverage photometric surface rendering and multi-view supervision to guide surface color prediction and improve rendering near the surface. Finally, we introduce a novel, loss-guided adaptive sampling method for more effective NeRF training by reducing the sampling redundancy. We show quantitatively and qualitatively that our method achieves higher representation power than prior face modeling methods in both controlled and in-the-wild settings. Code and models will be released upon publication.

Face Relighting with Geometrically Consistent Shadows

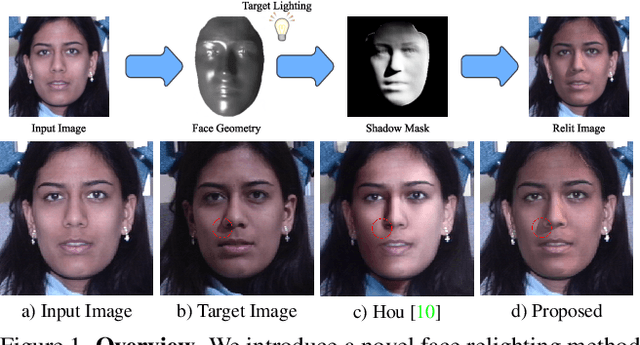
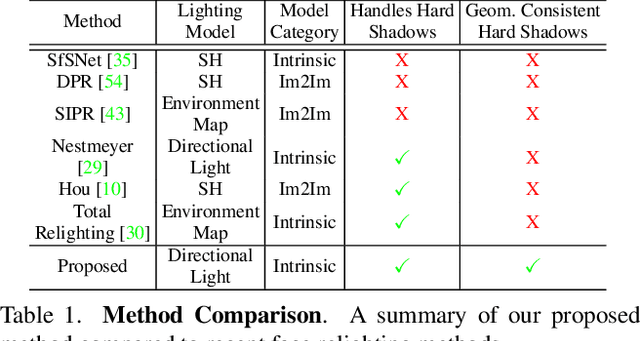
Most face relighting methods are able to handle diffuse shadows, but struggle to handle hard shadows, such as those cast by the nose. Methods that propose techniques for handling hard shadows often do not produce geometrically consistent shadows since they do not directly leverage the estimated face geometry while synthesizing them. We propose a novel differentiable algorithm for synthesizing hard shadows based on ray tracing, which we incorporate into training our face relighting model. Our proposed algorithm directly utilizes the estimated face geometry to synthesize geometrically consistent hard shadows. We demonstrate through quantitative and qualitative experiments on Multi-PIE and FFHQ that our method produces more geometrically consistent shadows than previous face relighting methods while also achieving state-of-the-art face relighting performance under directional lighting. In addition, we demonstrate that our differentiable hard shadow modeling improves the quality of the estimated face geometry over diffuse shading models.

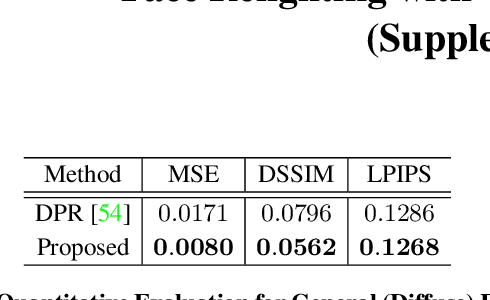
Towards High Fidelity Face Relighting with Realistic Shadows

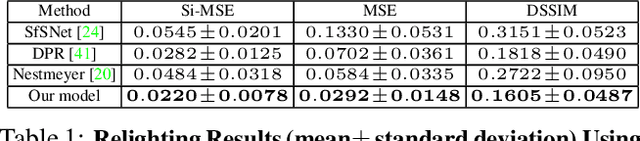
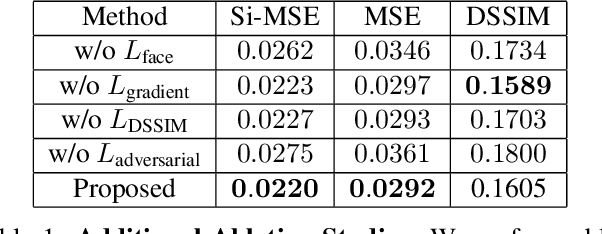
Existing face relighting methods often struggle with two problems: maintaining the local facial details of the subject and accurately removing and synthesizing shadows in the relit image, especially hard shadows. We propose a novel deep face relighting method that addresses both problems. Our method learns to predict the ratio (quotient) image between a source image and the target image with the desired lighting, allowing us to relight the image while maintaining the local facial details. During training, our model also learns to accurately modify shadows by using estimated shadow masks to emphasize on the high-contrast shadow borders. Furthermore, we introduce a method to use the shadow mask to estimate the ambient light intensity in an image, and are thus able to leverage multiple datasets during training with different global lighting intensities. With quantitative and qualitative evaluations on the Multi-PIE and FFHQ datasets, we demonstrate that our proposed method faithfully maintains the local facial details of the subject and can accurately handle hard shadows while achieving state-of-the-art face relighting performance.

Parallel Transport Unfolding: A Connection-based Manifold Learning Approach

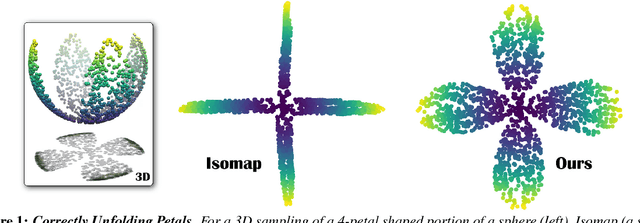
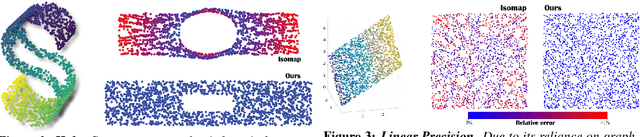
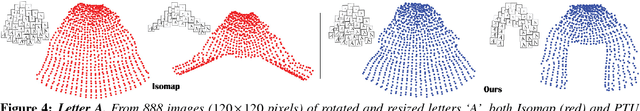
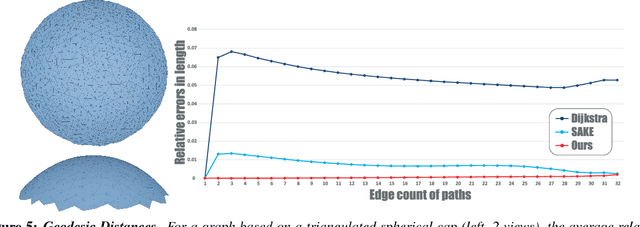
Manifold learning offers nonlinear dimensionality reduction of high-dimensional datasets. In this paper, we bring geometry processing to bear on manifold learning by introducing a new approach based on metric connection for generating a quasi-isometric, low-dimensional mapping from a sparse and irregular sampling of an arbitrary manifold embedded in a high-dimensional space. Geodesic distances of discrete paths over the input pointset are evaluated through "parallel transport unfolding" (PTU) to offer robustness to poor sampling and arbitrary topology. Our new geometric procedure exhibits the same strong resilience to noise as one of the staples of manifold learning, the Isomap algorithm, as it also exploits all pairwise geodesic distances to compute a low-dimensional embedding. While Isomap is limited to geodesically-convex sampled domains, parallel transport unfolding does not suffer from this crippling limitation, resulting in an improved robustness to irregularity and voids in the sampling. Moreover, it involves only simple linear algebra, significantly improves the accuracy of all pairwise geodesic distance approximations, and has the same computational complexity as Isomap. Finally, we show that our connection-based distance estimation can be used for faster variants of Isomap such as L-Isomap.