Towards free-response paradigm: a theory on decision-making in spiking neural networks

The energy-efficient and brain-like information processing abilities of Spiking Neural Networks (SNNs) have attracted considerable attention, establishing them as a crucial element of brain-inspired computing. One prevalent challenge encountered by SNNs is the trade-off between inference speed and accuracy, which requires sufficient time to achieve the desired level of performance. Drawing inspiration from animal behavior experiments that demonstrate a connection between decision-making reaction times, task complexity, and confidence levels, this study seeks to apply these insights to SNNs. The focus is on understanding how SNNs make inferences, with a particular emphasis on untangling the interplay between signal and noise in decision-making processes. The proposed theoretical framework introduces a new optimization objective for SNN training, highlighting the importance of not only the accuracy of decisions but also the development of predictive confidence through learning from past experiences. Experimental results demonstrate that SNNs trained according to this framework exhibit improved confidence expression, leading to better decision-making outcomes. In addition, a strategy is introduced for efficient decision-making during inference, which allows SNNs to complete tasks more quickly and can use stopping times as indicators of decision confidence. By integrating neuroscience insights with neuromorphic computing, this study opens up new possibilities to explore the capabilities of SNNs and advance their application in complex decision-making scenarios.

Learn to integrate parts for whole through correlated neural variability

Sensory perception originates from the responses of sensory neurons, which react to a collection of sensory signals linked to various physical attributes of a singular perceptual object. Unraveling how the brain extracts perceptual information from these neuronal responses is a pivotal challenge in both computational neuroscience and machine learning. Here we introduce a statistical mechanical theory, where perceptual information is first encoded in the correlated variability of sensory neurons and then reformatted into the firing rates of downstream neurons. Applying this theory, we illustrate the encoding of motion direction using neural covariance and demonstrate high-fidelity direction recovery by spiking neural networks. Networks trained under this theory also show enhanced performance in classifying natural images, achieving higher accuracy and faster inference speed. Our results challenge the traditional view of neural covariance as a secondary factor in neural coding, highlighting its potential influence on brain function.

Probabilistic Computation with Emerging Covariance: Towards Efficient Uncertainty Quantification

Building robust, interpretable, and secure artificial intelligence system requires some degree of quantifying and representing uncertainty via a probabilistic perspective, as it allows to mimic human cognitive abilities. However, probabilistic computation presents significant challenges due to its inherent complexity. In this paper, we develop an efficient and interpretable probabilistic computation framework by truncating the probabilistic representation up to its first two moments, i.e., mean and covariance. We instantiate the framework by training a deterministic surrogate of a stochastic network that learns the complex probabilistic representation via combinations of simple activations, encapsulating the non-linearities coupling of the mean and covariance. We show that when the mean is supervised for optimizing the task objective, the unsupervised covariance spontaneously emerging from the non-linear coupling with the mean faithfully captures the uncertainty associated with model predictions. Our research highlights the inherent computability and simplicity of probabilistic computation, enabling its wider application in large-scale settings.

Toward spike-based stochastic neural computing

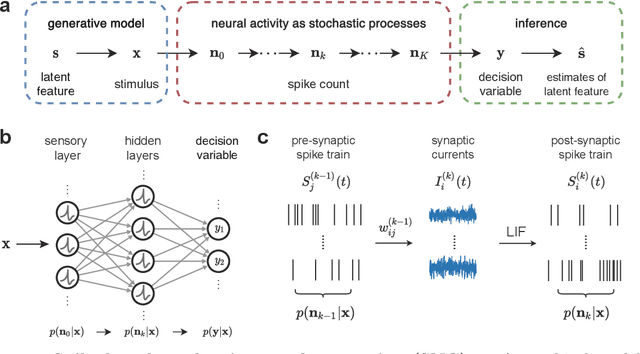
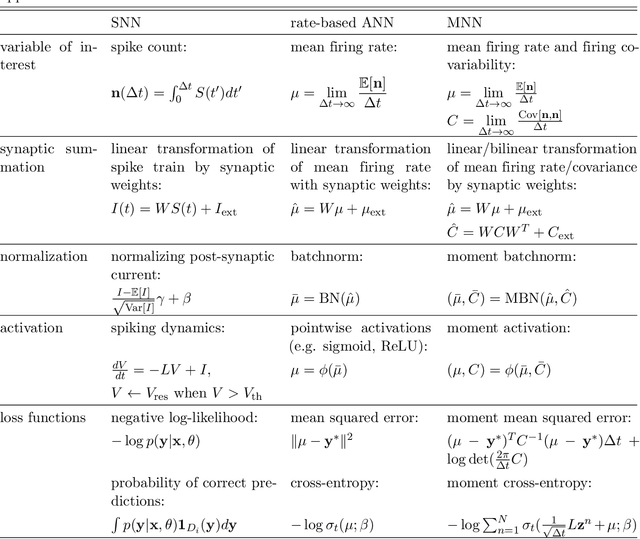
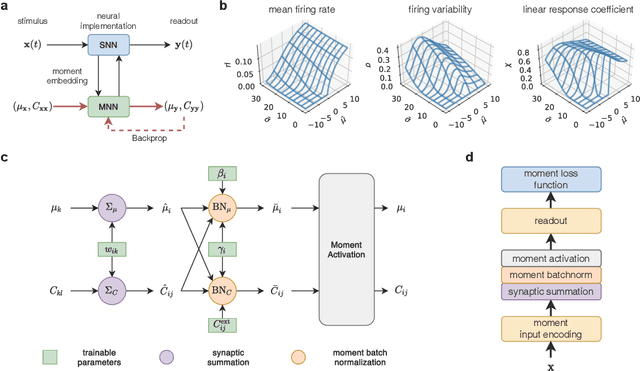
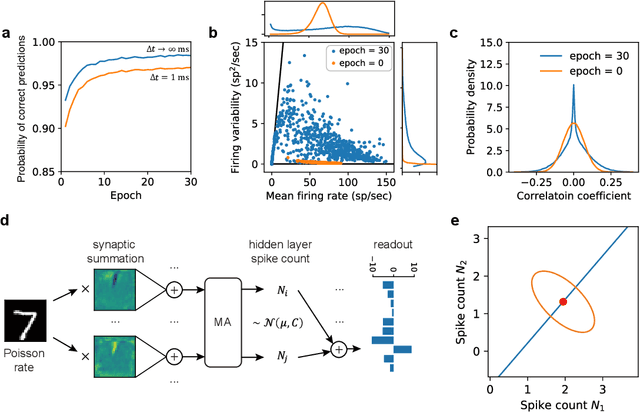
Inspired by the highly irregular spiking activity of cortical neurons, stochastic neural computing is an attractive theory for explaining the operating principles of the brain and the ability to represent uncertainty by intelligent agents. However, computing and learning with high-dimensional joint probability distributions of spiking neural activity across large populations of neurons present as a major challenge. To overcome this, we develop a novel moment embedding approach to enable gradient-based learning in spiking neural networks accounting for the propagation of correlated neural variability. We show under the supervised learning setting a spiking neural network trained this way is able to learn the task while simultaneously minimizing uncertainty, and further demonstrate its application to neuromorphic hardware. Built on the principle of spike-based stochastic neural computing, the proposed method opens up new opportunities for developing machine intelligence capable of computing uncertainty and for designing unconventional computing architectures.

Sub-Nyquist Sampling with Optical Pulses for Photonic Blind Source Separation

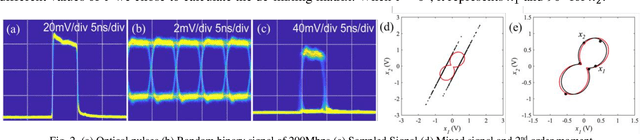
We proposed and demonstrated an optical pulse sampling method for photonic blind source separation. It can separate large bandwidth of mixed signals by small sampling frequency, which can reduce the workload of digital signal processing.

Photonic Interference Cancellation with Hybrid Free Space Optical Communication and MIMO Receiver

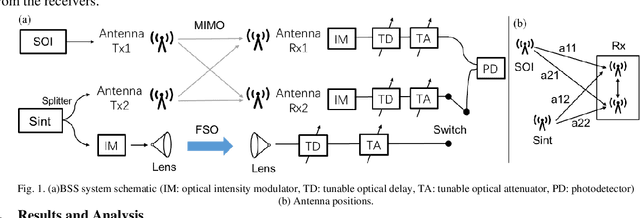
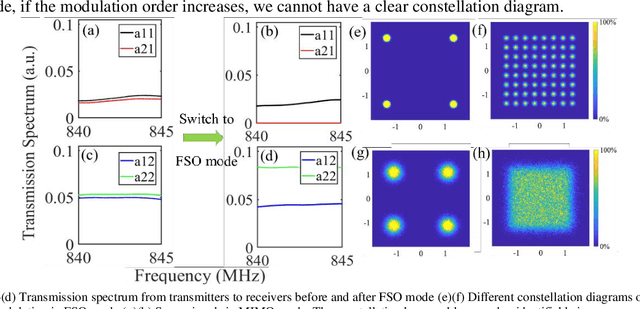
We proposed and demonstrated a hybrid blind source separation system which can switch between multiple-input and multi-output mode and free space optical communication mode depends on different situation to get best condition for separation.

Radio Frequency Interference Management with Free-Space Optical Communication and Photonic Signal Processing

We design and experimentally demonstrate a radio frequency interference management system with free-space optical communication and photonic signal processing. The system provides real-time interference cancellation in 6 GHz wide bandwidth.

Wideband photonic interference cancellation based on free space optical communication

We propose and experimentally demonstrate an interference management system that removes wideband wireless interference by using photonic signal processing and free space optical communication. The receiver separates radio frequency interferences by upconverting the mixed signals to optical frequencies and processing the signals with the photonic circuits. Signals with GHz bandwidth are processed and separated in real-time. The reference signals for interference cancellation are transmitted in a free space optical communication link, which provides large bandwidth for multi-band operation and accelerates the mixed signal separation process by reducing the dimensions of the un-known mixing matrix. Experimental results show that the system achieves 30dB real-time cancellation depth with over 6GHz bandwidth. Multiple radio frequency bands can be processed at the same time with a single system. In addition, multiple radio frequency bands can be processed at the same time with a single system.

Wideband photonic blind source separation with optical pulse sampling

We propose and experimentally demonstrate an optical pulse sampling method for photonic blind source separation. The photonic system processes and separates wideband signals based on the statistical information of the mixed signals and thus the sampling frequency can be orders of magnitude lower than the bandwidth of the signals. The ultra-fast optical pulse functions as a tweezer that collects samples of the signals at very low sampling rates, and each sample is short enough to maintain the statistical properties of the signals. The low sampling frequency reduces the workloads of the analog to digital conversion and digital signal processing systems. In the meantime, the short pulse sampling maintains the accuracy of the sampled signals, so the statistical properties of the undersampling signals are the same as the statistical properties of the original signals. With the optical pulses generated from a mode-locked laser, the optical pulse sampling system is able to process and separate mixed signals with bandwidth over 100GHz and achieves a dynamic range of 30dB.

Best k-layer neural network approximations

We investigate the geometry of the empirical risk minimization problem for $k$-layer neural networks. We will provide examples showing that for the classical activation functions $\sigma(x)= 1/\bigl(1 + \exp(-x)\bigr)$ and $\sigma(x)=\tanh(x)$, there exists a positive-measured subset of target functions that do not have best approximations by a fixed number of layers of neural networks. In addition, we study in detail the properties of shallow networks, classifying cases when a best $k$-layer neural network approximation always exists or does not exist for the ReLU activation $\sigma=\max(0,x)$. We also determine the dimensions of shallow ReLU-activated networks.