Data-Driven Model Selections of Second-Order Particle Dynamics via Integrating Gaussian Processes with Low-Dimensional Interacting Structures

In this paper, we focus on the data-driven discovery of a general second-order particle-based model that contains many state-of-the-art models for modeling the aggregation and collective behavior of interacting agents of similar size and body type. This model takes the form of a high-dimensional system of ordinary differential equations parameterized by two interaction kernels that appraise the alignment of positions and velocities. We propose a Gaussian Process-based approach to this problem, where the unknown model parameters are marginalized by using two independent Gaussian Process (GP) priors on latent interaction kernels constrained to dynamics and observational data. This results in a nonparametric model for interacting dynamical systems that accounts for uncertainty quantification. We also develop acceleration techniques to improve scalability. Moreover, we perform a theoretical analysis to interpret the methodology and investigate the conditions under which the kernels can be recovered. We demonstrate the effectiveness of the proposed approach on various prototype systems, including the selection of the order of the systems and the types of interactions. In particular, we present applications to modeling two real-world fish motion datasets that display flocking and milling patterns up to 248 dimensions. Despite the use of small data sets, the GP-based approach learns an effective representation of the nonlinear dynamics in these spaces and outperforms competitor methods.

Learning Transition Operators From Sparse Space-Time Samples

We consider the nonlinear inverse problem of learning a transition operator $\mathbf{A}$ from partial observations at different times, in particular from sparse observations of entries of its powers $\mathbf{A},\mathbf{A}^2,\cdots,\mathbf{A}^{T}$. This Spatio-Temporal Transition Operator Recovery problem is motivated by the recent interest in learning time-varying graph signals that are driven by graph operators depending on the underlying graph topology. We address the nonlinearity of the problem by embedding it into a higher-dimensional space of suitable block-Hankel matrices, where it becomes a low-rank matrix completion problem, even if $\mathbf{A}$ is of full rank. For both a uniform and an adaptive random space-time sampling model, we quantify the recoverability of the transition operator via suitable measures of incoherence of these block-Hankel embedding matrices. For graph transition operators these measures of incoherence depend on the interplay between the dynamics and the graph topology. We develop a suitable non-convex iterative reweighted least squares (IRLS) algorithm, establish its quadratic local convergence, and show that, in optimal scenarios, no more than $\mathcal{O}(rn \log(nT))$ space-time samples are sufficient to ensure accurate recovery of a rank-$r$ operator $\mathbf{A}$ of size $n \times n$. This establishes that spatial samples can be substituted by a comparable number of space-time samples. We provide an efficient implementation of the proposed IRLS algorithm with space complexity of order $O(r n T)$ and per-iteration time complexity linear in $n$. Numerical experiments for transition operators based on several graph models confirm that the theoretical findings accurately track empirical phase transitions, and illustrate the applicability and scalability of the proposed algorithm.

Data-driven discovery of interacting particle systems using Gaussian processes

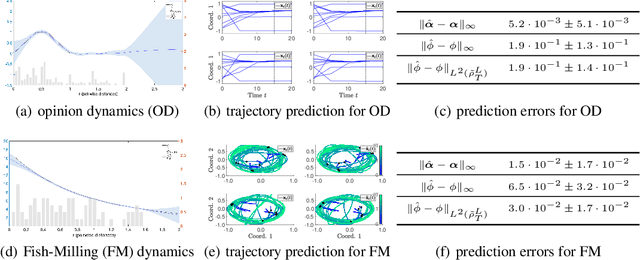
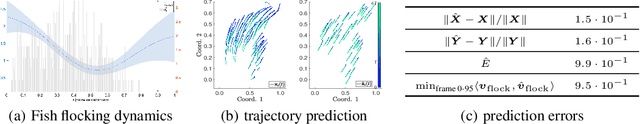
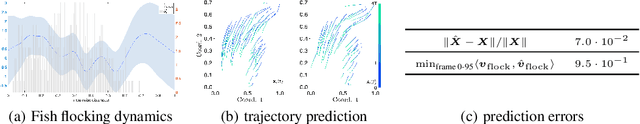
Interacting particle or agent systems that display a rich variety of collection motions are ubiquitous in science and engineering. A fundamental and challenging goal is to understand the link between individual interaction rules and collective behaviors. In this paper, we study the data-driven discovery of distance-based interaction laws in second-order interacting particle systems. We propose a learning approach that models the latent interaction kernel functions as Gaussian processes, which can simultaneously fulfill two inference goals: one is the nonparametric inference of interaction kernel function with the pointwise uncertainty quantification, and the other one is the inference of unknown parameters in the non-collective forces of the system. We formulate learning interaction kernel functions as a statistical inverse problem and provide a detailed analysis of recoverability conditions, establishing that a coercivity condition is sufficient for recoverability. We provide a finite-sample analysis, showing that our posterior mean estimator converges at an optimal rate equal to the one in the classical 1-dimensional Kernel Ridge regression. Numerical results on systems that exhibit different collective behaviors demonstrate efficient learning of our approach from scarce noisy trajectory data.

Learning Theory for Inferring Interaction Kernels in Second-Order Interacting Agent Systems

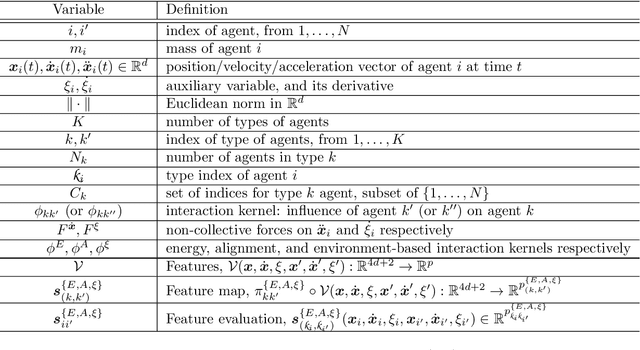
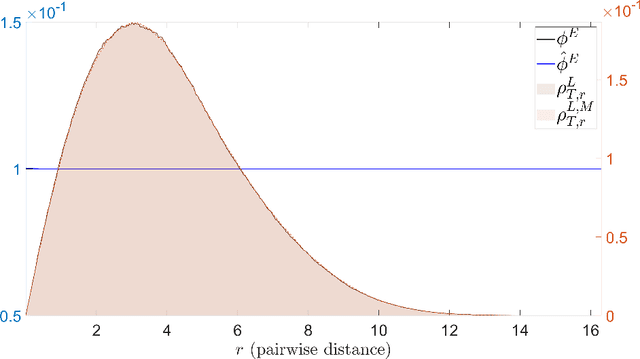
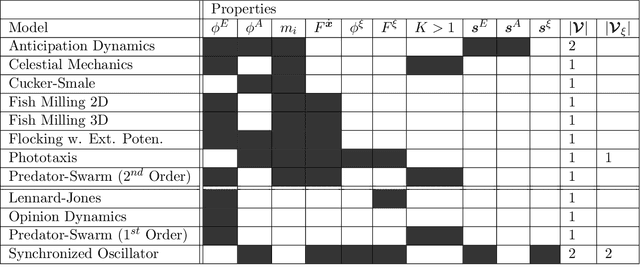
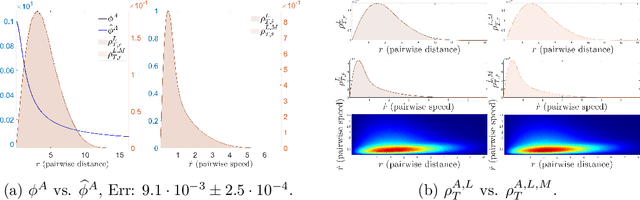
Modeling the complex interactions of systems of particles or agents is a fundamental scientific and mathematical problem that is studied in diverse fields, ranging from physics and biology, to economics and machine learning. In this work, we describe a very general second-order, heterogeneous, multivariable, interacting agent model, with an environment, that encompasses a wide variety of known systems. We describe an inference framework that uses nonparametric regression and approximation theory based techniques to efficiently derive estimators of the interaction kernels which drive these dynamical systems. We develop a complete learning theory which establishes strong consistency and optimal nonparametric min-max rates of convergence for the estimators, as well as provably accurate predicted trajectories. The estimators exploit the structure of the equations in order to overcome the curse of dimensionality and we describe a fundamental coercivity condition on the inverse problem which ensures that the kernels can be learned and relates to the minimal singular value of the learning matrix. The numerical algorithm presented to build the estimators is parallelizable, performs well on high-dimensional problems, and is demonstrated on complex dynamical systems.

Learning interaction kernels in stochastic systems of interacting particles from multiple trajectories

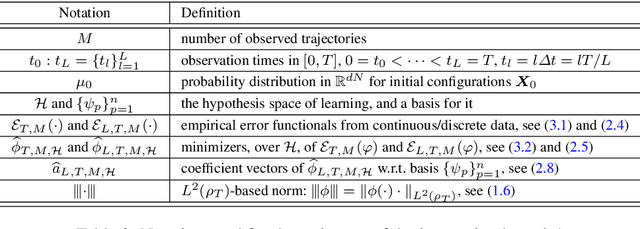
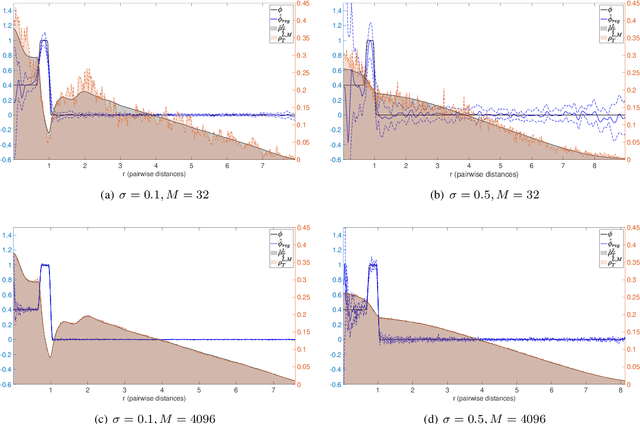
We consider stochastic systems of interacting particles or agents, with dynamics determined by an interaction kernel which only depends on pairwise distances. We study the problem of inferring this interaction kernel from observations of the positions of the particles, in either continuous or discrete time, along multiple independent trajectories. We introduce a nonparametric inference approach to this inverse problem, based on a regularized maximum likelihood estimator constrained to suitable hypothesis spaces adaptive to data. We show that a coercivity condition enables us to control the condition number of this problem and prove the consistency of our estimator, and that in fact it converges at a near-optimal learning rate, equal to the min-max rate of $1$-dimensional non-parametric regression. In particular, this rate is independent of the dimension of the state space, which is typically very high. We also analyze the discretization errors in the case of discrete-time observations, showing that it is of order $1/2$ in terms of the time gaps between observations. This term, when large, dominates the sampling error and the approximation error, preventing convergence of the estimator. Finally, we exhibit an efficient parallel algorithm to construct the estimator from data, and we demonstrate the effectiveness of our algorithm with numerical tests on prototype systems including stochastic opinion dynamics and a Lennard-Jones model.

Learning interaction kernels in heterogeneous systems of agents from multiple trajectories

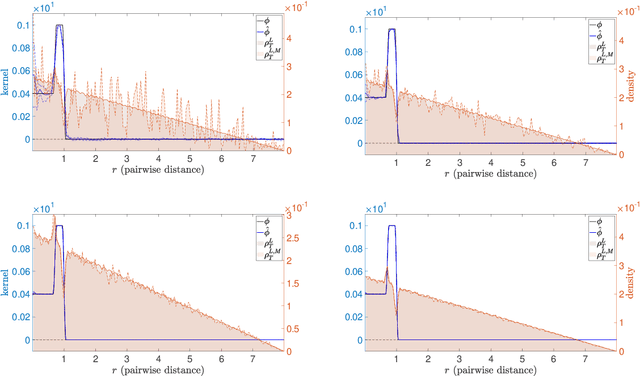
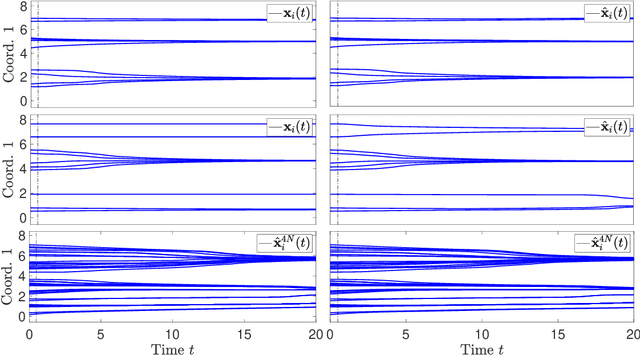
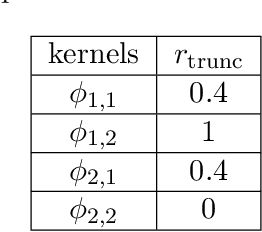
Systems of interacting particles or agents have wide applications in many disciplines such as Physics, Chemistry, Biology and Economics. These systems are governed by interaction laws, which are often unknown: estimating them from observation data is a fundamental task that can provide meaningful insights and accurate predictions of the behaviour of the agents. In this paper, we consider the inverse problem of learning interaction laws given data from multiple trajectories, in a nonparametric fashion, when the interaction kernels depend on pairwise distances. We establish a condition for learnability of interaction kernels, and construct estimators that are guaranteed to converge in a suitable $L^2$ space, at the optimal min-max rate for 1-dimensional nonparametric regression. We propose an efficient learning algorithm based on least squares, which can be implemented in parallel for multiple trajectories and is therefore well-suited for the high dimensional, big data regime. Numerical simulations on a variety examples, including opinion dynamics, predator-swarm dynamics and heterogeneous particle dynamics, suggest that the learnability condition is satisfied in models used in practice, and the rate of convergence of our estimator is consistent with the theory. These simulations also suggest that our estimators are robust to noise in the observations, and produce accurate predictions of dynamics in relative large time intervals, even when they are learned from data collected in short time intervals.

Nonparametric inference of interaction laws in systems of agents from trajectory data

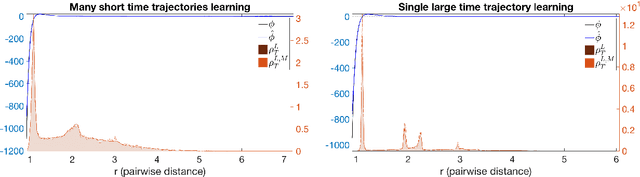
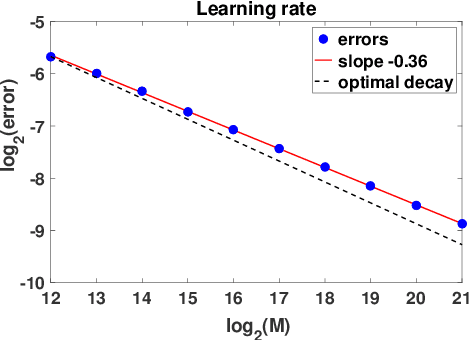
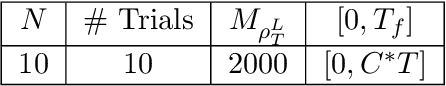
Inferring the laws of interaction between particles and agents in complex dynamical systems from observational data is a fundamental challenge in a wide variety of disciplines. We propose a non-parametric statistical learning approach to estimate the governing laws of distance-based interactions, with no reference or assumption about their analytical form, from data consisting trajectories of interacting agents. We demonstrate the effectiveness of our learning approach both by providing theoretical guarantees, and by testing the approach on a variety of prototypical systems in various disciplines. These systems include homogeneous and heterogeneous agents systems, ranging from particle systems in fundamental physics to agent-based systems modeling opinion dynamics under the social influence, prey-predator dynamics, flocking and swarming, and phototaxis in cell dynamics.