Electromagnetic Modeling and Capacity Analysis of Rydberg Atom-Based MIMO System

Rydberg atom-based antennas exploit the quantum properties of highly excited Rydberg atoms, providing unique advantages over classical antennas, such as high sensitivity, broad frequency range, and compact size. Despite the increasing interests in their applications in antenna and communication engineering, two key properties, involving the lack of polarization multiplexing and isotropic reception without mutual coupling, remain unexplored in the analysis of Rydberg atom-based spatial multiplexing, i.e., multiple-input and multiple-output (MIMO), communications. Generally, the design considerations for any antenna, even for atomic ones, can be extracted to factors such as radiation patterns, efficiency, and polarization, allowing them to be seamlessly integrated into existing system models. In this letter, we extract the antenna properties from relevant quantum characteristics, enabling electromagnetic modeling and capacity analysis of Rydberg MIMO systems in both far-field and near-field scenarios. By employing ray-based method for far-field analysis and dyadic Green's function for near-field calculation, our results indicate that Rydberg atom-based antenna arrays offer specific advantages over classical dipole-type arrays in single-polarization MIMO communications.

Electromagnetic Normalization of Channel Matrix for Holographic MIMO Communications

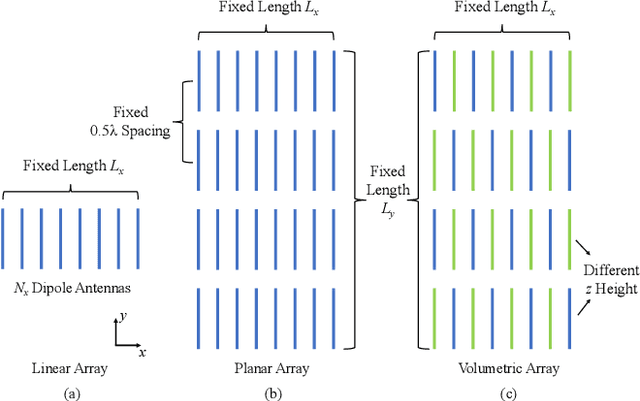
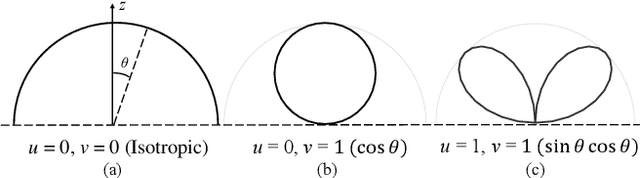
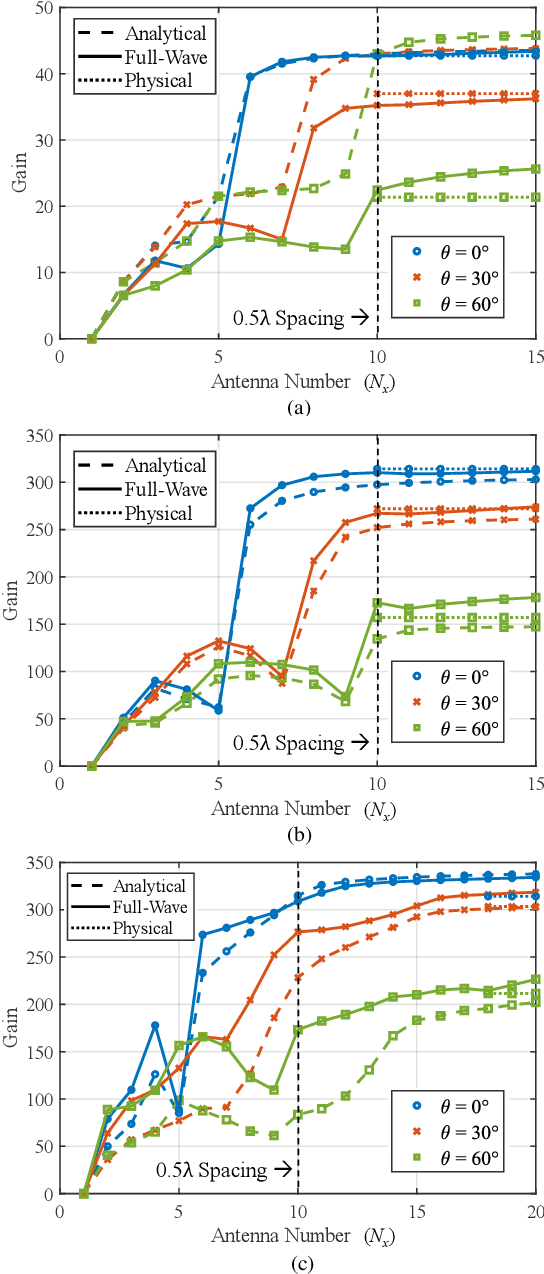
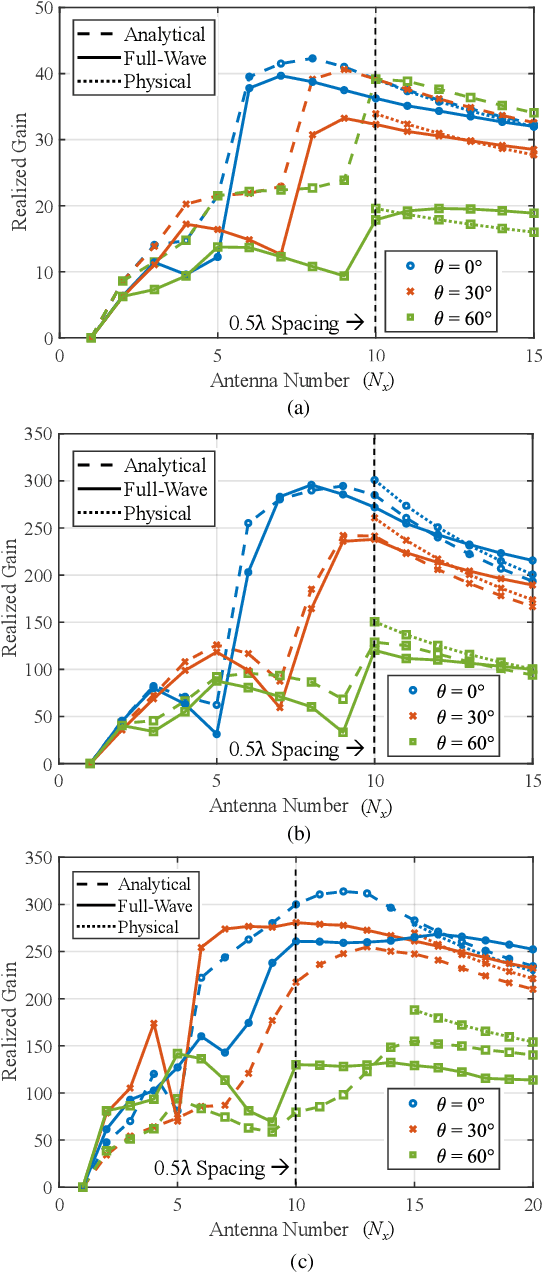
Holographic multiple-input and multiple-output (MIMO) communications introduce innovative antenna array configurations, such as dense arrays and volumetric arrays, which offer notable advantages over conventional planar arrays with half-wavelength element spacing. However, accurately assessing the performance of these new holographic MIMO systems necessitates careful consideration of channel matrix normalization, as it is influenced by array gain, which, in turn, depends on the array topology. Traditional normalization methods may be insufficient for assessing these advanced array topologies, potentially resulting in misleading or inaccurate evaluations. In this study, we propose electromagnetic normalization approaches for the channel matrix that accommodate arbitrary array topologies, drawing on the array gains from analytical, physical, and full-wave methods. Additionally, we introduce a normalization method for near-field MIMO channels based on a rigorous dyadic Green's function approach, which accounts for potential losses of gain at near field. Finally, we perform capacity analyses under quasi-static, ergodic, and near-field conditions, through adopting the proposed normalization techniques. Our findings indicate that channel matrix normalization should reflect the realized gains of the antenna array in target directions. Failing to accurately normalize the channel matrix can result in errors when evaluating the performance limits and benefits of unconventional holographic array topologies, potentially compromising the optimal design of holographic MIMO systems.

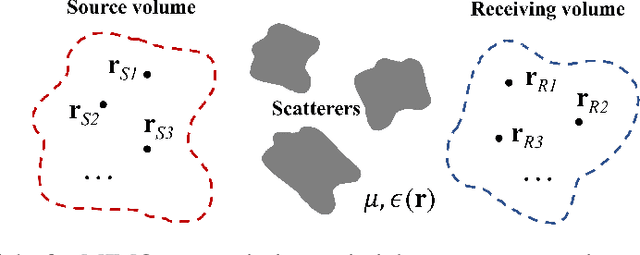
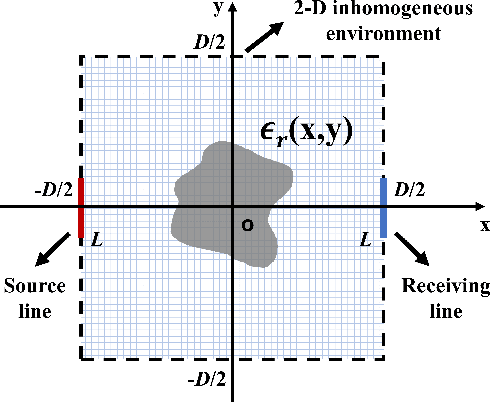
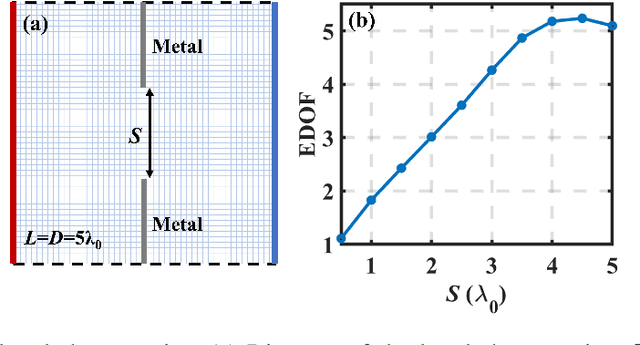
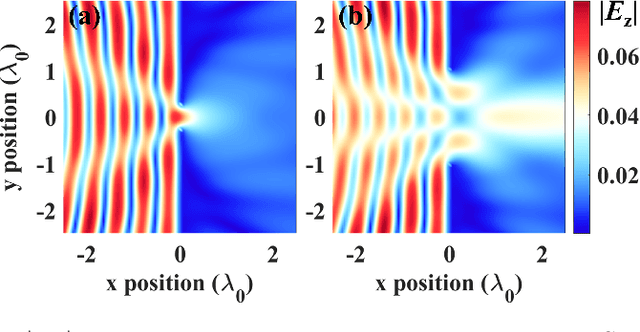
Electromagnetic Effective-Degree-of-Freedom Limit of a MIMO System in 2-D Inhomogeneous Environment

Compared with a single-input-single-output (SISO) wireless communication system, the benefit of multiple-input-multiple-output (MIMO) technology originates from its extra degree of freedom (DOF), also referred as scattering channels or spatial electromagnetic (EM) modes, brought by spatial multiplexing. When the physical sizes of transmitting and receiving arrays are fixed, and there are sufficient antennas (typically with half-wavelength spacings), the DOF limit is only dependent on the propagating environment. Analytical methods can be used to estimate this limit in free space, and some approximate models are adopted in stochastic environments, such as Clarke's model and Ray-tracing methods. However, this DOF limit in an certain inhomogeneous environment has not been well discussed with rigorous full-wave numerical methods. In this work, volume integral equation (VIE) is implemented for investigating the limit of MIMO effective degree of freedom (EDOF) in three representative two-dimensional (2-D) inhomogeneous environments. Moreover, we clarify the relation between the performance of a MIMO system and the scattering characteristics of its propagating environment.

Electromagnetic Effective Degree of Freedom of a MIMO System in Free Space

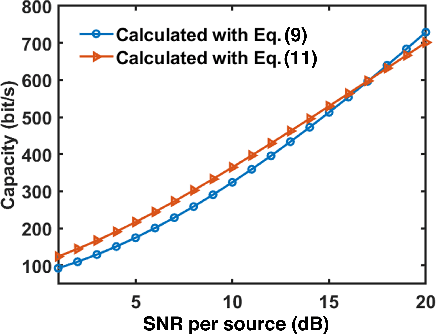
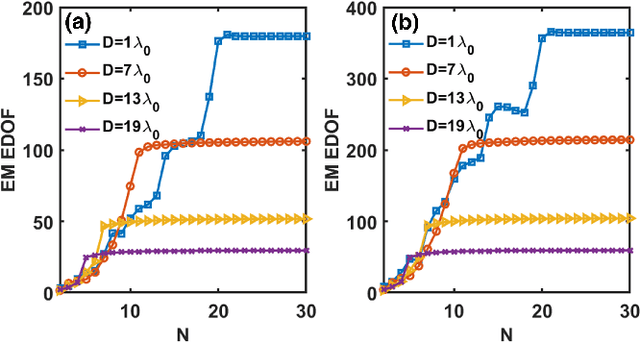
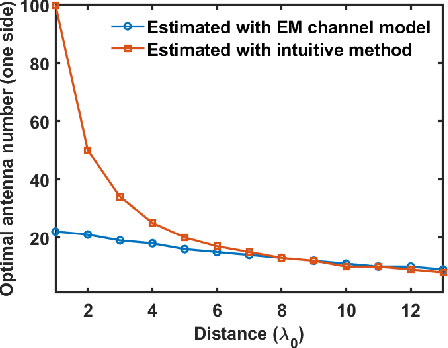
Effective degree of freedom (EDOF) of a multiple-input-multiple-output (MIMO) system represents its equivalent number of independent single-input-single-output (SISO) systems, which directly characterizes the communication performance. Traditional EDOF only considers single polarization, where the full polarized components degrade into two independent transverse components under the far-field approximation. However, the traditional model is not applicable to complex scenarios especially for the near-field region. Based on an electromagnetic (EM) channel model built from the dyadic Green's function, we first calculate the EM EDOF to estimate the performance of an arbitrary MIMO system with full polarizations in free space. Then, we clarify the relations between the limit of EDOF and the optimal number of sources/receivers. Finally, potential benefits of near-field MIMO communications are demonstrated with the EM EDOF, in which the contribution of the longitudinally polarized source is taken into account. This work establishes a fundamental EM framework for MIMO wireless communications.