Reconfigurable Codebook-Based Beamforming for RDARS-Aided mmWave MU-MIMO Systems

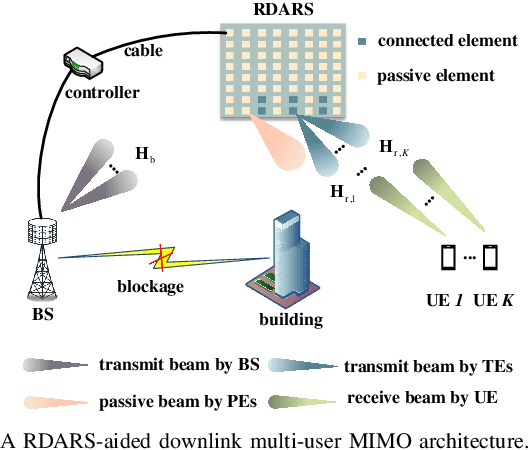
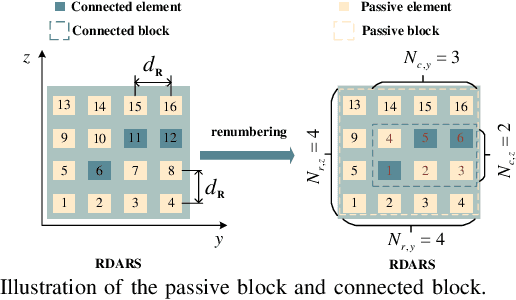
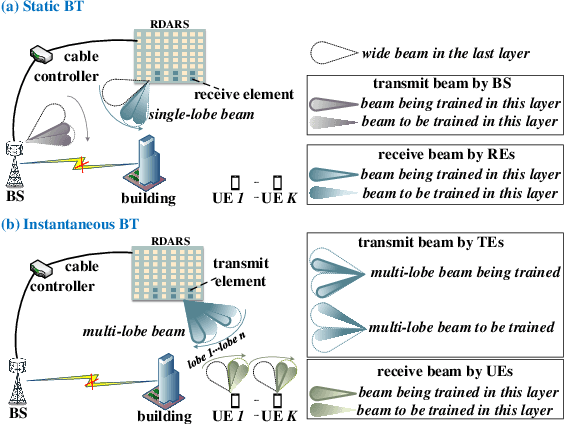
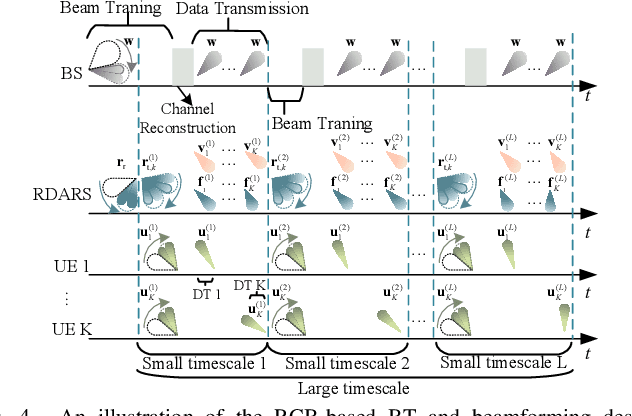
Reconfigurable distributed antenna and reflecting surface (RDARS) is a new architecture for the sixth-generation (6G) millimeter wave (mmWave) communications. In RDARS-aided mmWave systems, the active and passive beamforming design and working mode configuration for reconfigurable elements are crucial for system performance. In this paper, we aim to maximize the weighted sum rate (WSR) in the RDARS-aided mmWave system. To take advantage of RDARS, we first design a reconfigurable codebook (RCB) in which the number and dimension of the codeword can be flexibly adjusted. Then, a low overhead beam training scheme based on hierarchical search is proposed. Accordingly, the active and passive beamforming for data transmission is designed to achieve the maximum WSR for both space-division multiple access (SDMA) and time-division multiple access (TDMA) schemes. For the TDMA scheme, the optimal number of RDARS transmit elements and the allocated power budget for WSR maximization are derived in closed form. Besides, the superiority of the RDARS is verified and the conditions under which RDARS outperforms RIS and DAS are given. For the SDMA scheme, we characterize the relationship between the number of RDARS connected elements and the user distribution, followed by the derivation of the optimal placement positions of the RDARS transmit elements. High-quality beamforming design solutions are derived to minimize the inter-user interference (IUI) at the base station and RDARS side respectively, which nearly leads to the maximal WSR. Finally, simulation results confirm our theoretical findings and the superiority of the proposed schemes.

Joint Size and Placement Optimization for IRS-Aided Communications with Active and Passive Elements

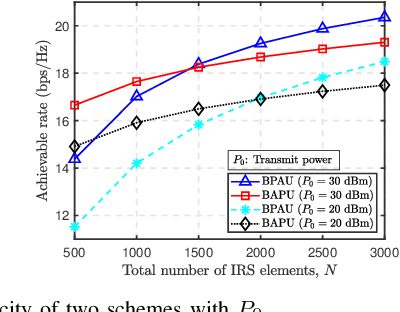
Different types of intelligent reflecting surfaces (IRS) are exploited for assisting wireless communications. The joint use of passive IRS (PIRS) and active IRS (AIRS) emerges as a promising solution owing to their complementary advantages. They can be integrated into a single hybrid active-passive IRS (HIRS) or deployed in a distributed manner, which poses challenges in determining the IRS element allocation and placement for rate maximization. In this paper, we investigate the capacity of an IRS-aided wireless communication system with both active and passive elements. Specifically, we consider three deployment schemes: 1) base station (BS)-HIRS-user (BHU); 2) BS-AIRS-PIRS-user (BAPU); 3) BS-PIRS-AIRS-user (BPAU). Under the line-of-sight channel model, we formulate a rate maximization problem via a joint optimization of the IRS element allocation and placement. We first derive the optimized number of active and passive elements for BHU, BAPU, and BPAU schemes, respectively. Then, low-complexity HIRS/AIRS placement strategies are provided. To obtain more insights, we characterize the system capacity scaling orders for the three schemes with respect to the large total number of IRS elements, amplification power budget, and BS transmit power. Finally, simulation results are presented to validate our theoretical findings and show the performance difference among the BHU, BAPU, and BPAU schemes with the proposed joint design under various system setups.

Intelligent Reflecting Surfaces Aided Wireless Network: Deployment Architectures and Solutions

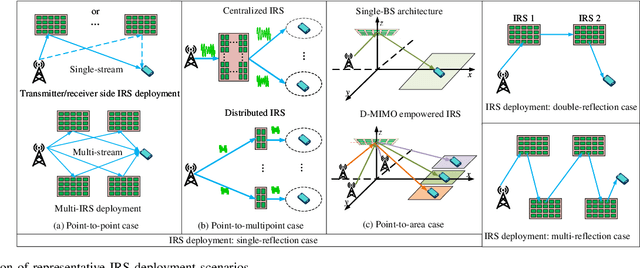
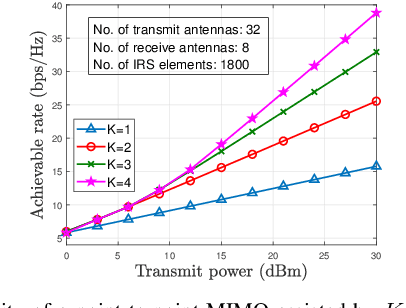
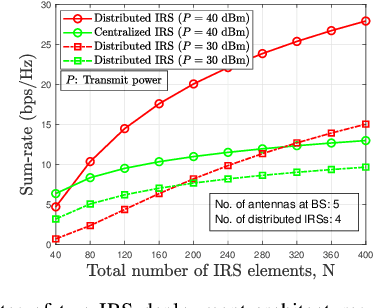
Intelligent reflecting surfaces (IRSs) have emerged as a transformative technology for wireless networks by improving coverage, capacity, and energy efficiency through intelligent manipulation of wireless propagation environments. This paper provides a comprehensive study on the deployment and coordination of IRSs for wireless networks. By addressing both single- and multi-reflection IRS architectures, we examine their deployment strategies across diverse scenarios, including point-to-point, point-to-multipoint, and point-to-area setups. For the single-reflection case, we highlight the trade-offs between passive and active IRS architectures in terms of beamforming gain, coverage extension, and spatial multiplexing. For the multi-reflection case, we discuss practical strategies to optimize IRS deployment and element allocation, balancing cooperative beamforming gains and path loss. The paper further discusses practical challenges in IRS implementation, including environmental conditions, system compatibility, and hardware limitations. Numerical results and field tests validate the effectiveness of IRS-aided wireless networks and demonstrate their capacity and coverage improvements. Lastly, promising research directions, including movable IRSs, near-field deployments, and network-level optimization, are outlined to guide future investigations.

Semi-Passive Intelligent Reflecting Surface Enabled Sensing Systems

Intelligent reflecting surface (IRS) has garnered growing interest and attention due to its potential for facilitating and supporting wireless communications and sensing. This paper studies a semi-passive IRS-enabled sensing system, where an IRS consists of both passive reflecting elements and active sensors. Our goal is to minimize the Cram\'{e}r-Rao bound (CRB) for parameter estimation under both point and extended target cases. Towards this goal, we begin by deriving the CRB for the direction-of-arrival (DoA) estimation in closed-form and then theoretically analyze the IRS reflecting elements and sensors allocation design based on the CRB under the point target case with a single-antenna base station (BS). To efficiently solve the corresponding optimization problem for the case with a multi-antenna BS, we propose an efficient algorithm by jointly optimizing the IRS phase shifts and the BS beamformers. Under the extended target case, the CRB for the target response matrix (TRM) estimation is minimized via the optimization of the BS transmit beamformers. Moreover, we explore the influence of various system parameters on the CRB and compare these effects to those observed under the point target case. Simulation results show the effectiveness of the semi-passive IRS and our proposed beamforming design for improving the performance of the sensing system.

Hybrid Active-Passive IRS Assisted Energy-Efficient Wireless Communication

Deploying active reflecting elements at the intelligent reflecting surface (IRS) increases signal amplification capability but incurs higher power consumption. Therefore, it remains a challenging and open problem to determine the optimal number of active/passive elements for maximizing energy efficiency (EE). To answer this question, we consider a hybrid active-passive IRS (H-IRS) assisted wireless communication system, where the H-IRS consists of both active and passive reflecting elements.Specifically, we study the optimization of the number of active/passive elements at the H-IRS to maximize EE. To this end, we first derive the closed-form expression for a near-optimal solution under the line-of-sight (LoS) channel case and obtain its optimal solution under the Rayleigh fading channel case. Then, an efficient algorithm is employed to obtain a high-quality sub-optimal solution for the EE maximization under the general Rician channel case. Simulation results demonstrate the effectiveness of the H-IRS for maximizing EE under different Rician factors and IRS locations.