Benchmarking Constraint-Based Bayesian Structure Learning Algorithms: Role of Network Topology

Modeling the associations between real world entities from their multivariate cross-sectional profiles can provide cues into the concerted working of these entities as a system. Several techniques have been proposed for deciphering these associations including constraint-based Bayesian structure learning (BSL) algorithms that model them as directed acyclic graphs. Benchmarking these algorithms have typically focused on assessing the variation in performance measures such as sensitivity as a function of the dimensionality represented by the number of nodes in the DAG, and sample size. The present study elucidates the importance of network topology in benchmarking exercises. More specifically, it investigates variations in sensitivity across distinct network topologies while constraining the nodes, edges, and sample-size to be identical, eliminating these as potential confounders. Sensitivity of three popular constraint-based BSL algorithms (Peter-Clarke, Grow-Shrink, Incremental Association Markov Blanket) in learning the network structure from multivariate cross-sectional profiles sampled from network models with sub-linear, linear, and super-linear DAG topologies generated using preferential attachment is investigated. Results across linear and nonlinear models revealed statistically significant $(\alpha=0.05)$ decrease in sensitivity estimates from sub-linear to super-linear topology constitutively across the three algorithms. These results are demonstrated on networks with nodes $(N_{nods}=48,64)$, noise strengths $(\sigma =3,6)$ and sample size $(N = 2^{10})$. The findings elucidate the importance of accommodating the network topology in constraint-based BSL benchmarking exercises.

Entropy and the Kullback-Leibler Divergence for Bayesian Networks: Computational Complexity and Efficient Implementation

Bayesian networks (BNs) are a foundational model in machine learning and causal inference. Their graphical structure can handle high-dimensional problems, divide-and-conquering them into a sparse collection of smaller ones; underlies Judea Pearl's causality; and determines their explainability and interpretability. Despite their popularity, there are few resources in the literature on how to compute Shannon's entropy and the Kullback-Leibler (KL) divergence for BNs under their most common distributional assumptions. In this paper, we provide computationally efficient algorithms for both by leveraging BNs' graphical structure, and we illustrate them with a complete set of numerical examples. In the process, we show it is possible to reduce the computational complexity of KL from cubic to quadratic for Gaussian BNs.

Towards a Transportable Causal Network Model Based on Observational Healthcare Data

Over the last decades, many prognostic models based on artificial intelligence techniques have been used to provide detailed predictions in healthcare. Unfortunately, the real-world observational data used to train and validate these models are almost always affected by biases that can strongly impact the outcomes validity: two examples are values missing not-at-random and selection bias. Addressing them is a key element in achieving transportability and in studying the causal relationships that are critical in clinical decision making, going beyond simpler statistical approaches based on probabilistic association. In this context, we propose a novel approach that combines selection diagrams, missingness graphs, causal discovery and prior knowledge into a single graphical model to estimate the cardiovascular risk of adolescent and young females who survived breast cancer. We learn this model from data comprising two different cohorts of patients. The resulting causal network model is validated by expert clinicians in terms of risk assessment, accuracy and explainability, and provides a prognostic model that outperforms competing machine learning methods.

Learning Bayesian Networks with Heterogeneous Agronomic Data Sets via Mixed-Effect Models and Hierarchical Clustering

Research involving diverse but related data sets, where associations between covariates and outcomes may vary, is prevalent in various fields including agronomic studies. In these scenarios, hierarchical models, also known as multilevel models, are frequently employed to assimilate information from different data sets while accommodating their distinct characteristics. However, their structure extend beyond simple heterogeneity, as variables often form complex networks of causal relationships. Bayesian networks (BNs) provide a powerful framework for modelling such relationships using directed acyclic graphs to illustrate the connections between variables. This study introduces a novel approach that integrates random effects into BN learning. Rooted in linear mixed-effects models, this approach is particularly well-suited for handling hierarchical data. Results from a real-world agronomic trial suggest that employing this approach enhances structural learning, leading to the discovery of new connections and the improvement of improved model specification. Furthermore, we observe a reduction in prediction errors from 28% to 17%. By extending the applicability of BNs to complex data set structures, this approach contributes to the effective utilisation of BNs for hierarchical agronomic data. This, in turn, enhances their value as decision-support tools in the field.

Analyzing Complex Systems with Cascades Using Continuous-Time Bayesian Networks

Interacting systems of events may exhibit cascading behavior where events tend to be temporally clustered. While the cascades themselves may be obvious from the data, it is important to understand which states of the system trigger them. For this purpose, we propose a modeling framework based on continuous-time Bayesian networks (CTBNs) to analyze cascading behavior in complex systems. This framework allows us to describe how events propagate through the system and to identify likely sentry states, that is, system states that may lead to imminent cascading behavior. Moreover, CTBNs have a simple graphical representation and provide interpretable outputs, both of which are important when communicating with domain experts. We also develop new methods for knowledge extraction from CTBNs and we apply the proposed methodology to a data set of alarms in a large industrial system.

Causal Discovery with Missing Data in a Multicentric Clinical Study

Causal inference for testing clinical hypotheses from observational data presents many difficulties because the underlying data-generating model and the associated causal graph are not usually available. Furthermore, observational data may contain missing values, which impact the recovery of the causal graph by causal discovery algorithms: a crucial issue often ignored in clinical studies. In this work, we use data from a multi-centric study on endometrial cancer to analyze the impact of different missingness mechanisms on the recovered causal graph. This is achieved by extending state-of-the-art causal discovery algorithms to exploit expert knowledge without sacrificing theoretical soundness. We validate the recovered graph with expert physicians, showing that our approach finds clinically-relevant solutions. Finally, we discuss the goodness of fit of our graph and its consistency from a clinical decision-making perspective using graphical separation to validate causal pathways.

Risk Assessment of Lymph Node Metastases in Endometrial Cancer Patients: A Causal Approach

Assessing the pre-operative risk of lymph node metastases in endometrial cancer patients is a complex and challenging task. In principle, machine learning and deep learning models are flexible and expressive enough to capture the dynamics of clinical risk assessment. However, in this setting we are limited to observational data with quality issues, missing values, small sample size and high dimensionality: we cannot reliably learn such models from limited observational data with these sources of bias. Instead, we choose to learn a causal Bayesian network to mitigate the issues above and to leverage the prior knowledge on endometrial cancer available from clinicians and physicians. We introduce a causal discovery algorithm for causal Bayesian networks based on bootstrap resampling, as opposed to the single imputation used in related works. Moreover, we include a context variable to evaluate whether selection bias results in learning spurious associations. Finally, we discuss the strengths and limitations of our findings in light of the presence of missing data that may be missing-not-at-random, which is common in real-world clinical settings.

fairml: A Statistician's Take on Fair Machine Learning Modelling

The adoption of machine learning in applications where it is crucial to ensure fairness and accountability has led to a large number of model proposals in the literature, largely formulated as optimisation problems with constraints reducing or eliminating the effect of sensitive attributes on the response. While this approach is very flexible from a theoretical perspective, the resulting models are somewhat black-box in nature: very little can be said about their statistical properties, what are the best practices in their applied use, and how they can be extended to problems other than those they were originally designed for. Furthermore, the estimation of each model requires a bespoke implementation involving an appropriate solver which is less than desirable from a software engineering perspective. In this paper, we describe the fairml R package which implements our previous work (Scutari, Panero, and Proissl 2022) and related models in the literature. fairml is designed around classical statistical models (generalised linear models) and penalised regression results (ridge regression) to produce fair models that are interpretable and whose properties are well-known. The constraint used to enforce fairness is orthogonal to model estimation, making it possible to mix-and-match the desired model family and fairness definition for each application. Furthermore, fairml provides facilities for model estimation, model selection and validation including diagnostic plots.

Using Mixed-Effect Models to Learn Bayesian Networks from Related Data Sets

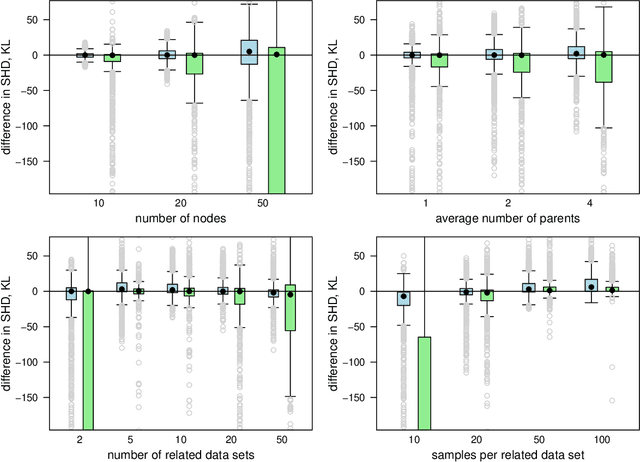
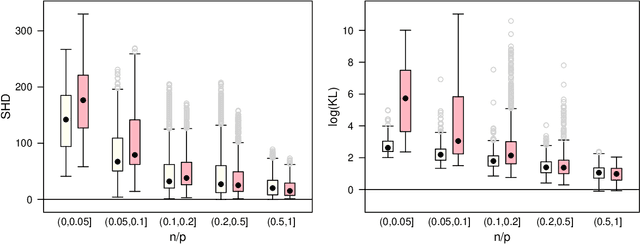
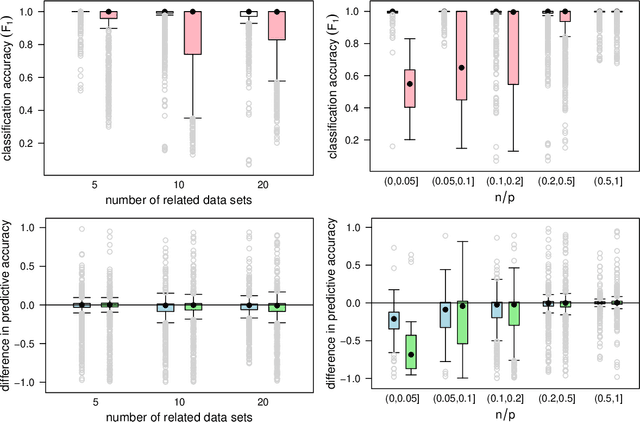
We commonly assume that data are a homogeneous set of observations when learning the structure of Bayesian networks. However, they often comprise different data sets that are related but not homogeneous because they have been collected in different ways or from different populations. In our previous work (Azzimonti, Corani and Scutari, 2021), we proposed a closed-form Bayesian Hierarchical Dirichlet score for discrete data that pools information across related data sets to learn a single encompassing network structure, while taking into account the differences in their probabilistic structures. In this paper, we provide an analogous solution for learning a Bayesian network from continuous data using mixed-effects models to pool information across the related data sets. We study its structural, parametric, predictive and classification accuracy and we show that it outperforms both conditional Gaussian Bayesian networks (that do not perform any pooling) and classical Gaussian Bayesian networks (that disregard the heterogeneous nature of the data). The improvement is marked for low sample sizes and for unbalanced data sets.

Comments on: "Hybrid Semiparametric Bayesian Networks"

Invited discussion on the paper "Hybrid Semiparametric Bayesian Networks" by David Atienza, Pedro Larranaga and Concha Bielza (TEST, 2022).