Deep learning-based modularized loading protocol for parameter estimation of Bouc-Wen class models

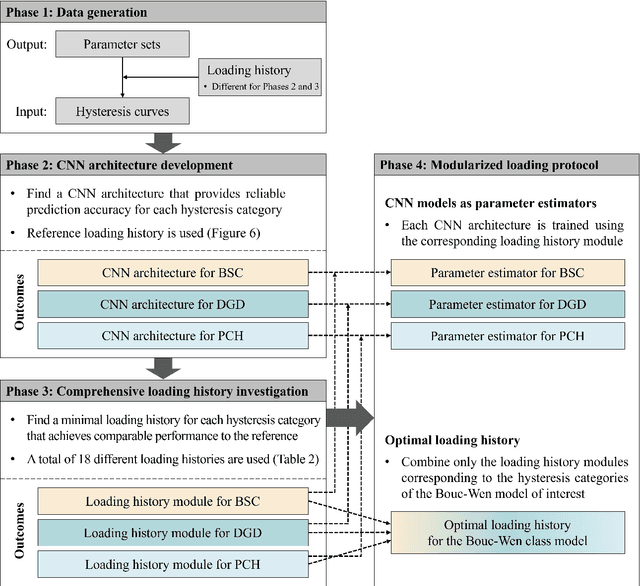
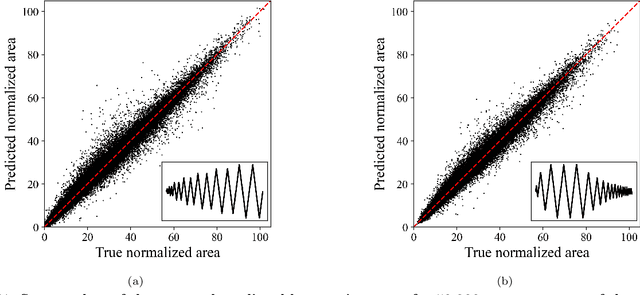
This study proposes a modularized deep learning-based loading protocol for optimal parameter estimation of Bouc-Wen (BW) class models. The protocol consists of two key components: optimal loading history construction and CNN-based rapid parameter estimation. Each component is decomposed into independent sub-modules tailored to distinct hysteretic behaviors-basic hysteresis, structural degradation, and pinching effect-making the protocol adaptable to diverse hysteresis models. Three independent CNN architectures are developed to capture the path-dependent nature of these hysteretic behaviors. By training these CNN architectures on diverse loading histories, minimal loading sequences, termed \textit{loading history modules}, are identified and then combined to construct an optimal loading history. The three CNN models, trained on the respective loading history modules, serve as rapid parameter estimators. Numerical evaluation of the protocol, including nonlinear time history analysis of a 3-story steel moment frame and fragility curve construction for a 3-story reinforced concrete frame, demonstrates that the proposed protocol significantly reduces total analysis time while maintaining or improving estimation accuracy. The proposed protocol can be extended to other hysteresis models, suggesting a systematic approach for identifying general hysteresis models.

Foundation Models for Electrocardiograms

Foundation models, enhanced by self-supervised learning (SSL) techniques, represent a cutting-edge frontier in biomedical signal analysis, particularly for electrocardiograms (ECGs), crucial for cardiac health monitoring and diagnosis. This study conducts a comprehensive analysis of foundation models for ECGs by employing and refining innovative SSL methodologies - namely, generative and contrastive learning - on a vast dataset of over 1.1 million ECG samples. By customizing these methods to align with the intricate characteristics of ECG signals, our research has successfully developed foundation models that significantly elevate the precision and reliability of cardiac diagnostics. These models are adept at representing the complex, subtle nuances of ECG data, thus markedly enhancing diagnostic capabilities. The results underscore the substantial potential of SSL-enhanced foundation models in clinical settings and pave the way for extensive future investigations into their scalable applications across a broader spectrum of medical diagnostics. This work sets a benchmark in the ECG field, demonstrating the profound impact of tailored, data-driven model training on the efficacy and accuracy of medical diagnostics.

MEMTO: Memory-guided Transformer for Multivariate Time Series Anomaly Detection

Detecting anomalies in real-world multivariate time series data is challenging due to complex temporal dependencies and inter-variable correlations. Recently, reconstruction-based deep models have been widely used to solve the problem. However, these methods still suffer from an over-generalization issue and fail to deliver consistently high performance. To address this issue, we propose the MEMTO, a memory-guided Transformer using a reconstruction-based approach. It is designed to incorporate a novel memory module that can learn the degree to which each memory item should be updated in response to the input data. To stabilize the training procedure, we use a two-phase training paradigm which involves using K-means clustering for initializing memory items. Additionally, we introduce a bi-dimensional deviation-based detection criterion that calculates anomaly scores considering both input space and latent space. We evaluate our proposed method on five real-world datasets from diverse domains, and it achieves an average anomaly detection F1-score of 95.74%, significantly outperforming the previous state-of-the-art methods. We also conduct extensive experiments to empirically validate the effectiveness of our proposed model's key components.

Probabilistic Performance-Pattern Decomposition (PPPD): analysis framework and applications to stochastic mechanical systems

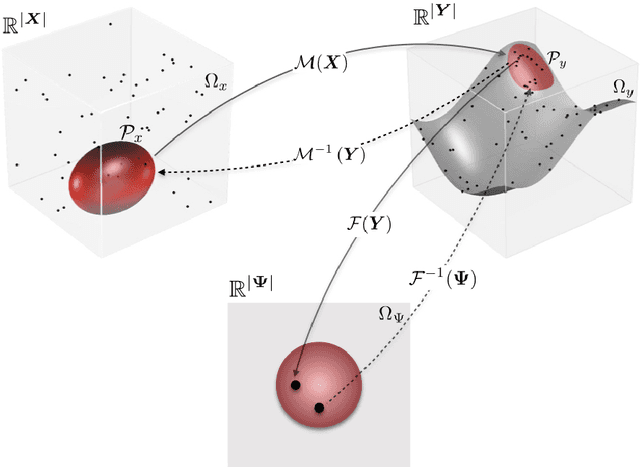
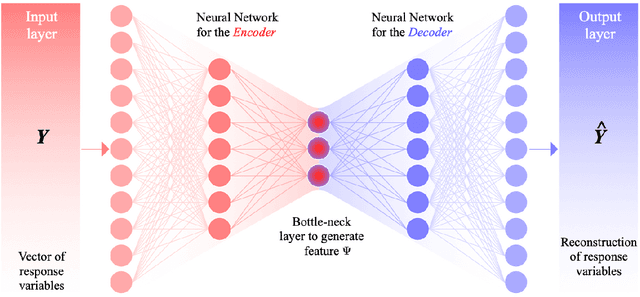
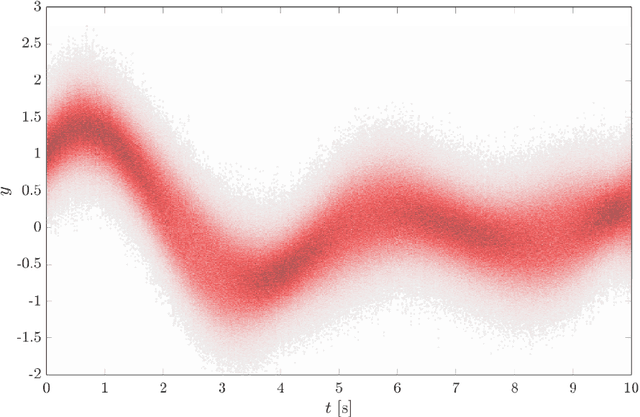
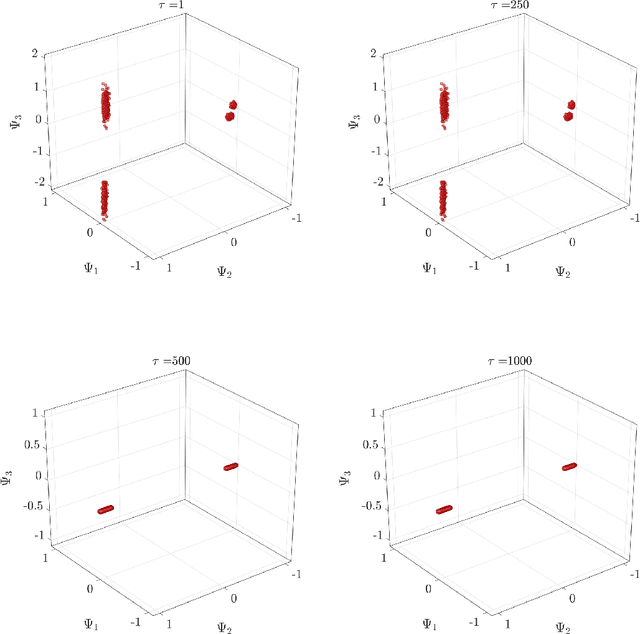
Since the early 1900s, numerous research efforts have been devoted to developing quantitative solutions to stochastic mechanical systems. In general, the problem is perceived as solved when a complete or partial probabilistic description on the quantity of interest (QoI) is determined. However, in the presence of complex system behavior, there is a critical need to go beyond mere probabilistic descriptions. In fact, to gain a full understanding of the system, it is crucial to extract physical characterizations from the probabilistic structure of the QoI, especially when the QoI solution is obtained in a data-driven fashion. Motivated by this perspective, the paper proposes a framework to obtain structuralized characterizations on behaviors of stochastic systems. The framework is named Probabilistic Performance-Pattern Decomposition (PPPD). PPPD analysis aims to decompose complex response behaviors, conditional to a prescribed performance state, into meaningful patterns in the space of system responses, and to investigate how the patterns are triggered in the space of basic random variables. To illustrate the application of PPPD, the paper studies three numerical examples: 1) an illustrative example with hypothetical stochastic processes input and output; 2) a stochastic Lorenz system with periodic as well as chaotic behaviors; and 3) a simplified shear-building model subjected to a stochastic ground motion excitation.