Heterogeneous Causal Discovery of Repeated Undesirable Health Outcomes

Understanding factors triggering or preventing undesirable health outcomes across patient subpopulations is essential for designing targeted interventions. While randomized controlled trials and expert-led patient interviews are standard methods for identifying these factors, they can be time-consuming and infeasible. Causal discovery offers an alternative to conventional approaches by generating cause-and-effect hypotheses from observational data. However, it often relies on strong or untestable assumptions, which can limit its practical application. This work aims to make causal discovery more practical by considering multiple assumptions and identifying heterogeneous effects. We formulate the problem of discovering causes and effect modifiers of an outcome, where effect modifiers are contexts (e.g., age groups) with heterogeneous causal effects. Then, we present a novel, end-to-end framework that incorporates an ensemble of causal discovery algorithms and estimation of heterogeneous effects to discover causes and effect modifiers that trigger or inhibit the outcome. We demonstrate that the ensemble approach improves robustness by enhancing recall of causal factors while maintaining precision. Our study examines the causes of repeat emergency room visits for diabetic patients and hospital readmissions for ICU patients. Our framework generates causal hypotheses consistent with existing literature and can help practitioners identify potential interventions and patient subpopulations to focus on.

Learning Exposure Mapping Functions for Inferring Heterogeneous Peer Effects

In causal inference, interference refers to the phenomenon in which the actions of peers in a network can influence an individual's outcome. Peer effect refers to the difference in counterfactual outcomes of an individual for different levels of peer exposure, the extent to which an individual is exposed to the treatments, actions, or behaviors of peers. Estimating peer effects requires deciding how to represent peer exposure. Typically, researchers define an exposure mapping function that aggregates peer treatments and outputs peer exposure. Most existing approaches for defining exposure mapping functions assume peer exposure based on the number or fraction of treated peers. Recent studies have investigated more complex functions of peer exposure which capture that different peers can exert different degrees of influence. However, none of these works have explicitly considered the problem of automatically learning the exposure mapping function. In this work, we focus on learning this function for the purpose of estimating heterogeneous peer effects, where heterogeneity refers to the variation in counterfactual outcomes for the same peer exposure but different individual's contexts. We develop EgoNetGNN, a graph neural network (GNN)-based method, to automatically learn the appropriate exposure mapping function allowing for complex peer influence mechanisms that, in addition to peer treatments, can involve the local neighborhood structure and edge attributes. We show that GNN models that use peer exposure based on the number or fraction of treated peers or learn peer exposure naively face difficulty accounting for such influence mechanisms. Our comprehensive evaluation on synthetic and semi-synthetic network data shows that our method is more robust to different unknown underlying influence mechanisms when estimating heterogeneous peer effects when compared to state-of-the-art baselines.

See Me and Believe Me: Causality and Intersectionality in Testimonial Injustice in Healthcare

In medical settings, it is critical that all who are in need of care are correctly heard and understood. When this is not the case due to prejudices a listener has, the speaker is experiencing \emph{testimonial injustice}, which, building upon recent work, we quantify by the presence of several categories of unjust vocabulary in medical notes. In this paper, we use FCI, a causal discovery method, to study the degree to which certain demographic features could lead to marginalization (e.g., age, gender, and race) by way of contributing to testimonial injustice. To achieve this, we review physicians' notes for each patient, where we identify occurrences of unjust vocabulary, along with the demographic features present, and use causal discovery to build a Structural Causal Model (SCM) relating those demographic features to testimonial injustice. We analyze and discuss the resulting SCMs to show the interaction of these factors and how they influence the experience of injustice. Despite the potential presence of some confounding variables, we observe how one contributing feature can make a person more prone to experiencing another contributor of testimonial injustice. There is no single root of injustice and thus intersectionality cannot be ignored. These results call for considering more than singular or equalized attributes of who a person is when analyzing and improving their experiences of bias and injustice. This work is thus a first foray at using causal discovery to understand the nuanced experiences of patients in medical settings, and its insights could be used to guide design principles throughout healthcare, to build trust and promote better patient care.

Cascade-based Randomization for Inferring Causal Effects under Diffusion Interference

The presence of interference, where the outcome of an individual may depend on the treatment assignment and behavior of neighboring nodes, can lead to biased causal effect estimation. Current approaches to network experiment design focus on limiting interference through cluster-based randomization, in which clusters are identified using graph clustering, and cluster randomization dictates the node assignment to treatment and control. However, cluster-based randomization approaches perform poorly when interference propagates in cascades, whereby the response of individuals to treatment propagates to their multi-hop neighbors. When we have knowledge of the cascade seed nodes, we can leverage this interference structure to mitigate the resulting causal effect estimation bias. With this goal, we propose a cascade-based network experiment design that initiates treatment assignment from the cascade seed node and propagates the assignment to their multi-hop neighbors to limit interference during cascade growth and thereby reduce the overall causal effect estimation error. Our extensive experiments on real-world and synthetic datasets demonstrate that our proposed framework outperforms the existing state-of-the-art approaches in estimating causal effects in network data.

Bridging or Breaking: Impact of Intergroup Interactions on Religious Polarization

While exposure to diverse viewpoints may reduce polarization, it can also have a backfire effect and exacerbate polarization when the discussion is adversarial. Here, we examine the question whether intergroup interactions around important events affect polarization between majority and minority groups in social networks. We compile data on the religious identity of nearly 700,000 Indian Twitter users engaging in COVID-19-related discourse during 2020. We introduce a new measure for an individual's group conformity based on contextualized embeddings of tweet text, which helps us assess polarization between religious groups. We then use a meta-learning framework to examine heterogeneous treatment effects of intergroup interactions on an individual's group conformity in the light of communal, political, and socio-economic events. We find that for political and social events, intergroup interactions reduce polarization. This decline is weaker for individuals at the extreme who already exhibit high conformity to their group. In contrast, during communal events, intergroup interactions can increase group conformity. Finally, we decompose the differential effects across religious groups in terms of emotions and topics of discussion. The results show that the dynamics of religious polarization are sensitive to the context and have important implications for understanding the role of intergroup interactions.

Leveraging heterogeneous spillover effects in maximizing contextual bandit rewards

Recommender systems relying on contextual multi-armed bandits continuously improve relevant item recommendations by taking into account the contextual information. The objective of these bandit algorithms is to learn the best arm (i.e., best item to recommend) for each user and thus maximize the cumulative rewards from user engagement with the recommendations. However, current approaches ignore potential spillover between interacting users, where the action of one user can impact the actions and rewards of other users. Moreover, spillover may vary for different people based on their preferences and the closeness of ties to other users. This leads to heterogeneity in the spillover effects, i.e., the extent to which the action of one user can impact the action of another. Here, we propose a framework that allows contextual multi-armed bandits to account for such heterogeneous spillovers when choosing the best arm for each user. By experimenting on several real-world datasets using prominent linear and non-linear contextual bandit algorithms, we observe that our proposed method leads to significantly higher rewards than existing solutions that ignore spillover.

Mitigating Cold-start Forecasting using Cold Causal Demand Forecasting Model

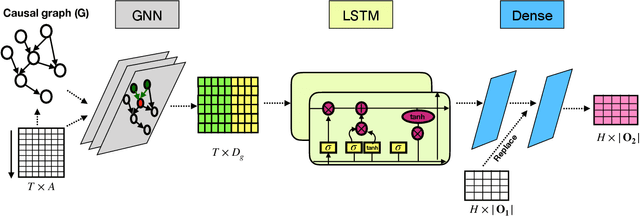
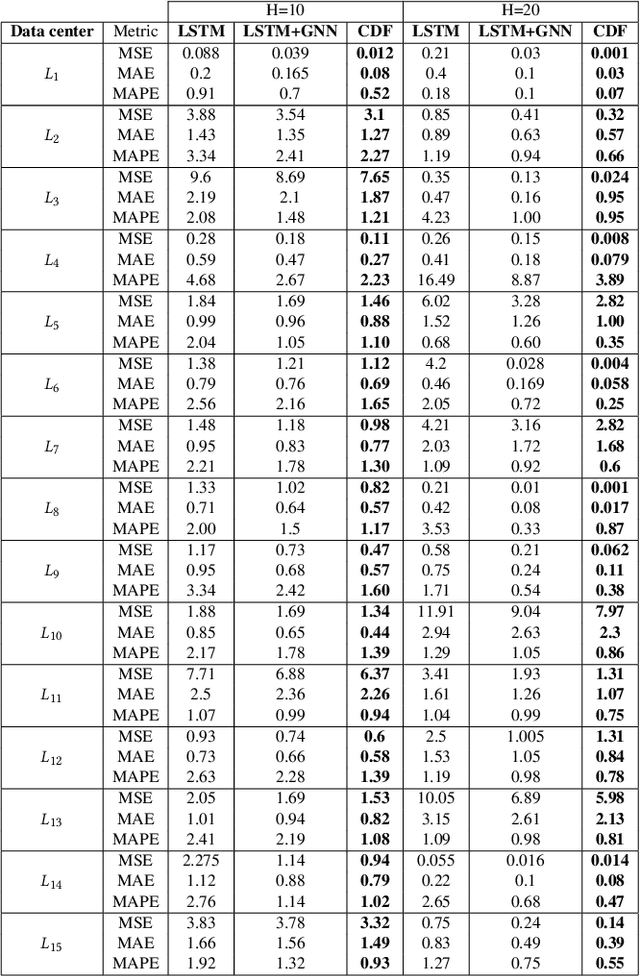
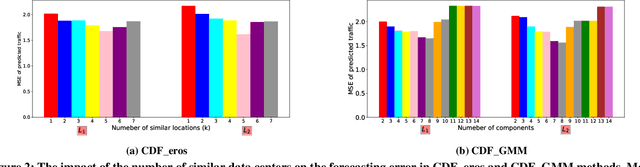
Forecasting multivariate time series data, which involves predicting future values of variables over time using historical data, has significant practical applications. Although deep learning-based models have shown promise in this field, they often fail to capture the causal relationship between dependent variables, leading to less accurate forecasts. Additionally, these models cannot handle the cold-start problem in time series data, where certain variables lack historical data, posing challenges in identifying dependencies among variables. To address these limitations, we introduce the Cold Causal Demand Forecasting (CDF-cold) framework that integrates causal inference with deep learning-based models to enhance the forecasting accuracy of multivariate time series data affected by the cold-start problem. To validate the effectiveness of the proposed approach, we collect 15 multivariate time-series datasets containing the network traffic of different Google data centers. Our experiments demonstrate that the CDF-cold framework outperforms state-of-the-art forecasting models in predicting future values of multivariate time series data.

Contagion Effect Estimation Using Proximal Embeddings

Contagion effect refers to the causal effect of peers' behavior on the outcome of an individual in social networks. While prominent methods for estimating contagion effects in observational studies often assume that there are no unmeasured confounders, contagion can be confounded due to latent homophily: nodes in a homophilous network tend to have ties to peers with similar attributes and can behave similarly without influencing one another. One way to account for latent homophily is by considering proxies for the unobserved confounders. However, in the presence of high-dimensional proxies, proxy-based methods can lead to substantially biased estimation of contagion effects, as we demonstrate in this paper. To tackle this issue, we introduce the novel Proximal Embeddings (ProEmb), a framework which integrates Variational Autoencoders (VAEs) and adversarial networks to generate balanced low-dimensional representations of high-dimensional proxies for different treatment groups and identifies contagion effects in the presence of unobserved network confounders. We empirically show that our method significantly increases the accuracy of contagion effect estimation in observational network data compared to state-of-the-art methods.

Inferring Causal Effects Under Heterogeneous Peer Influence

Causal inference in networks should account for interference, which occurs when a unit's outcome is influenced by treatments or outcomes of peers. There can be heterogeneous peer influence between units when a unit's outcome is subjected to variable influence from different peers based on their attributes and relationships, or when each unit has a different susceptibility to peer influence. Existing solutions to causal inference under interference consider either homogeneous influence from peers or specific heterogeneous influence mechanisms (e.g., based on local neighborhood structure). This paper presents a methodology for estimating individual causal effects in the presence of heterogeneous peer influence due to arbitrary mechanisms. We propose a structural causal model for networks that can capture arbitrary assumptions about network structure, interference conditions, and causal dependence. We identify potential heterogeneous contexts using the causal model and propose a novel graph neural network-based estimator to estimate individual causal effects. We show that existing state-of-the-art methods for individual causal effect estimation produce biased results in the presence of heterogeneous peer influence, and that our proposed estimator is robust.

Data-Driven Estimation of Heterogeneous Treatment Effects

Estimating how a treatment affects different individuals, known as heterogeneous treatment effect estimation, is an important problem in empirical sciences. In the last few years, there has been a considerable interest in adapting machine learning algorithms to the problem of estimating heterogeneous effects from observational and experimental data. However, these algorithms often make strong assumptions about the observed features in the data and ignore the structure of the underlying causal model, which can lead to biased estimation. At the same time, the underlying causal mechanism is rarely known in real-world datasets, making it hard to take it into consideration. In this work, we provide a survey of state-of-the-art data-driven methods for heterogeneous treatment effect estimation using machine learning, broadly categorizing them as methods that focus on counterfactual prediction and methods that directly estimate the causal effect. We also provide an overview of a third category of methods which rely on structural causal models and learn the model structure from data. Our empirical evaluation under various underlying structural model mechanisms shows the advantages and deficiencies of existing estimators and of the metrics for measuring their performance.