Bo Yang Hsueh
Accelerating Sparse DNN Models without Hardware-Support via Tile-Wise Sparsity
Aug 29, 2020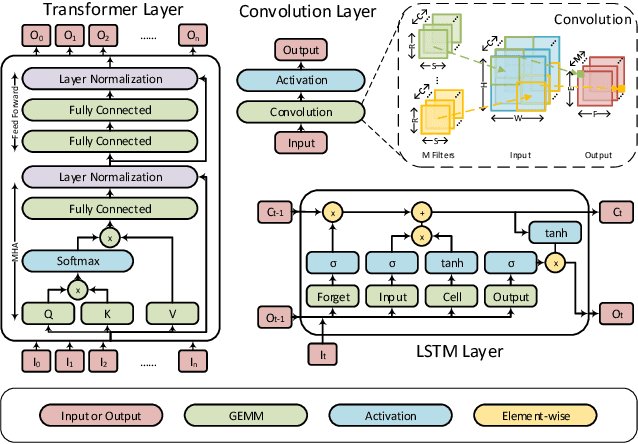
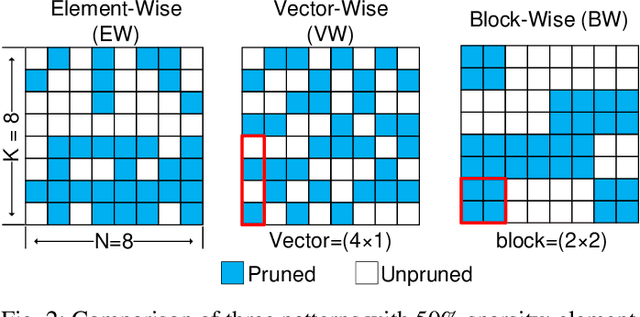
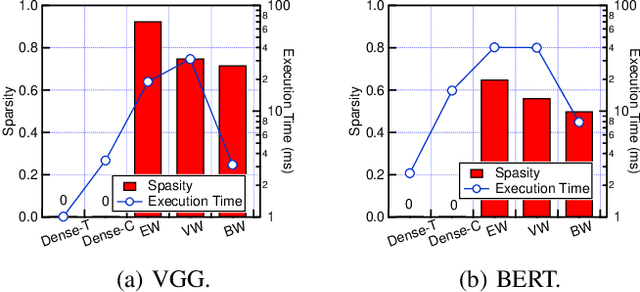
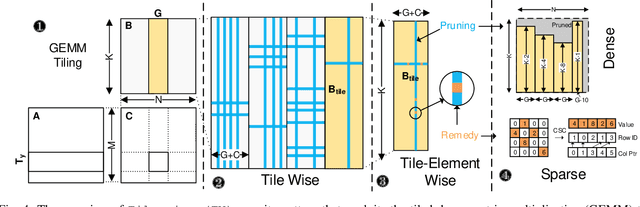
Abstract:Network pruning can reduce the high computation cost of deep neural network (DNN) models. However, to maintain their accuracies, sparse models often carry randomly-distributed weights, leading to irregular computations. Consequently, sparse models cannot achieve meaningful speedup on commodity hardware (e.g., GPU) built for dense matrix computations. As such, prior works usually modify or design completely new sparsity-optimized architectures for exploiting sparsity. We propose an algorithm-software co-designed pruning method that achieves latency speedups on existing dense architectures. Our work builds upon the insight that the matrix multiplication generally breaks the large matrix into multiple smaller tiles for parallel execution. We propose a tiling-friendly "tile-wise" sparsity pattern, which maintains a regular pattern at the tile level for efficient execution but allows for irregular, arbitrary pruning at the global scale to maintain the high accuracy. We implement and evaluate the sparsity pattern on GPU tensor core, achieving a 1.95x speedup over the dense model.
Stochastic Gradient Descent with Hyperbolic-Tangent Decay
Jun 05, 2018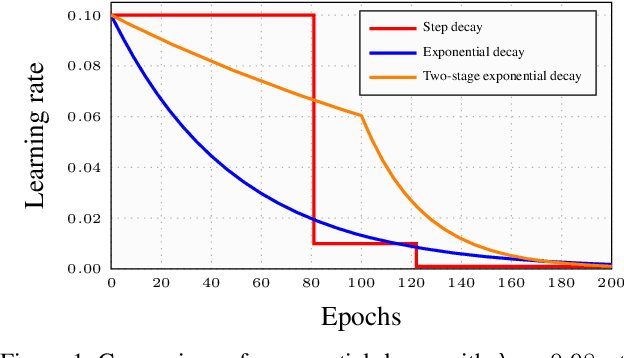
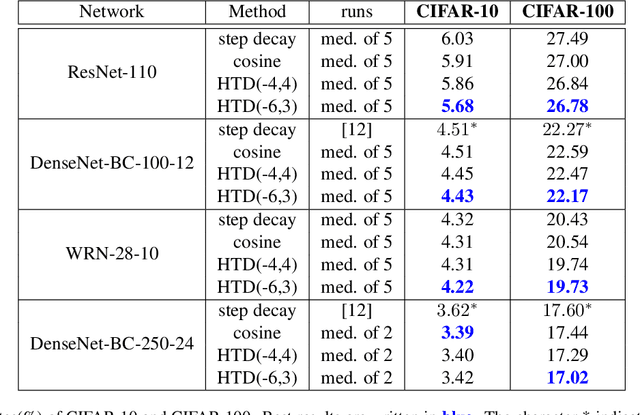
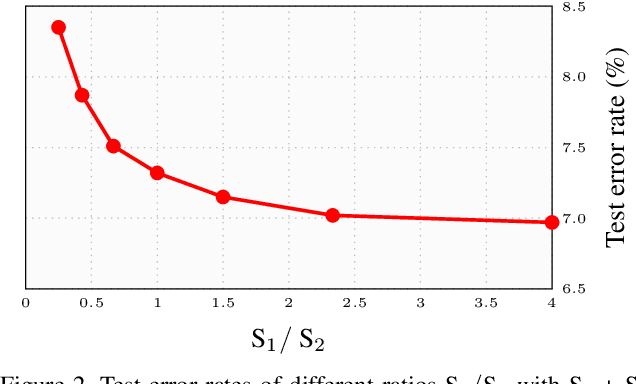
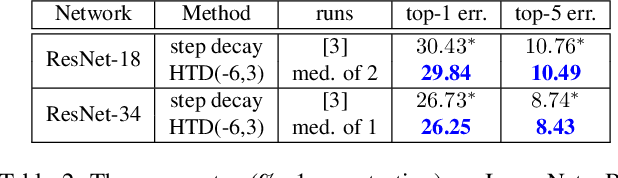
Abstract:Learning rate scheduler has been a critical issue in the deep neural network training. Several schedulers and methods have been proposed, including step decay scheduler, adaptive method, cosine scheduler and cyclical scheduler. This paper proposes a new scheduling method, named hyperbolic-tangent decay (HTD). We run experiments on several benchmarks such as: ResNet, Wide ResNet and DenseNet for CIFAR-10 and CIFAR-100 datasets, LSTM for PAMAP2 dataset, ResNet on ImageNet and Fashion-MNIST datasets. In our experiments, HTD outperforms step decay and cosine scheduler in nearly all cases, while requiring less hyperparameters than step decay, and more flexible than cosine scheduler.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge