Scalable Deep Generative Modeling for Sparse Graphs

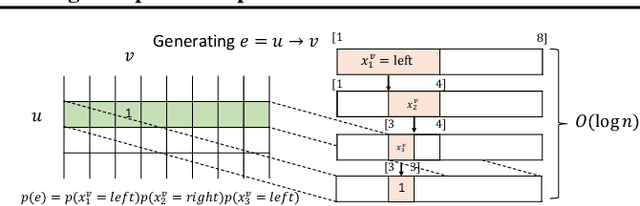
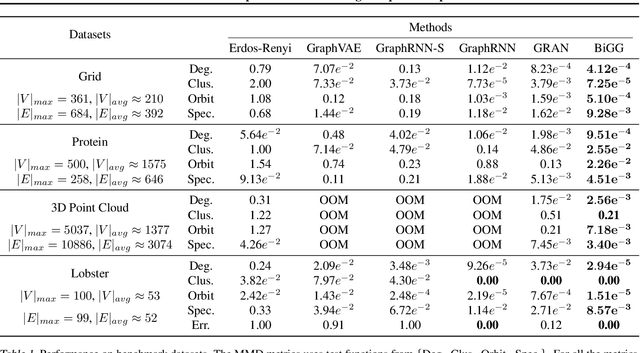
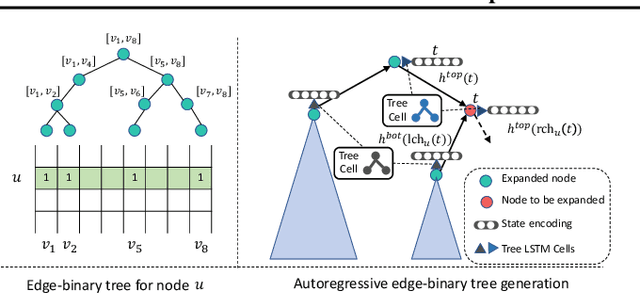
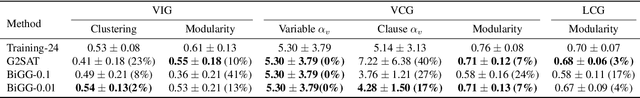
Learning graph generative models is a challenging task for deep learning and has wide applicability to a range of domains like chemistry, biology and social science. However current deep neural methods suffer from limited scalability: for a graph with $n$ nodes and $m$ edges, existing deep neural methods require $\Omega(n^2)$ complexity by building up the adjacency matrix. On the other hand, many real world graphs are actually sparse in the sense that $m\ll n^2$. Based on this, we develop a novel autoregressive model, named BiGG, that utilizes this sparsity to avoid generating the full adjacency matrix, and importantly reduces the graph generation time complexity to $O((n + m)\log n)$. Furthermore, during training this autoregressive model can be parallelized with $O(\log n)$ synchronization stages, which makes it much more efficient than other autoregressive models that require $\Omega(n)$. Experiments on several benchmarks show that the proposed approach not only scales to orders of magnitude larger graphs than previously possible with deep autoregressive graph generative models, but also yields better graph generation quality.

Chip Placement with Deep Reinforcement Learning

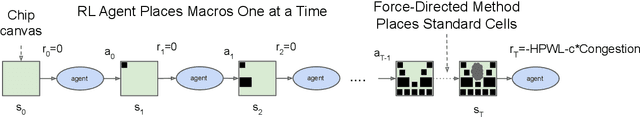
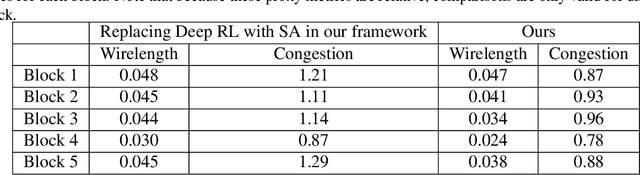
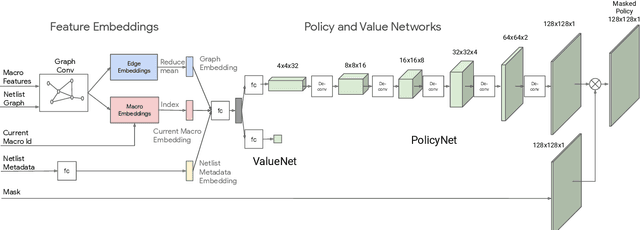
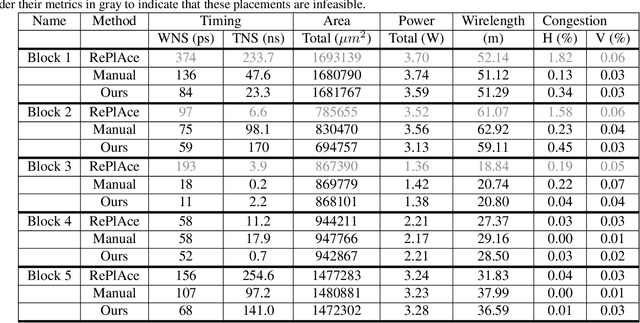
In this work, we present a learning-based approach to chip placement, one of the most complex and time-consuming stages of the chip design process. Unlike prior methods, our approach has the ability to learn from past experience and improve over time. In particular, as we train over a greater number of chip blocks, our method becomes better at rapidly generating optimized placements for previously unseen chip blocks. To achieve these results, we pose placement as a Reinforcement Learning (RL) problem and train an agent to place the nodes of a chip netlist onto a chip canvas. To enable our RL policy to generalize to unseen blocks, we ground representation learning in the supervised task of predicting placement quality. By designing a neural architecture that can accurately predict reward across a wide variety of netlists and their placements, we are able to generate rich feature embeddings of the input netlists. We then use this architecture as the encoder of our policy and value networks to enable transfer learning. Our objective is to minimize PPA (power, performance, and area), and we show that, in under 6 hours, our method can generate placements that are superhuman or comparable on modern accelerator netlists, whereas existing baselines require human experts in the loop and take several weeks.

Generalized Clustering by Learning to Optimize Expected Normalized Cuts

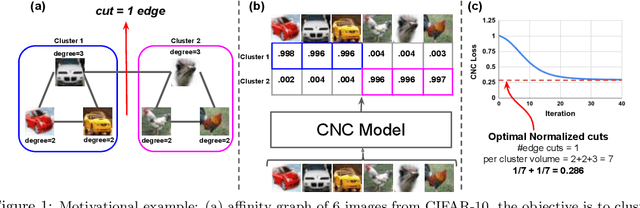
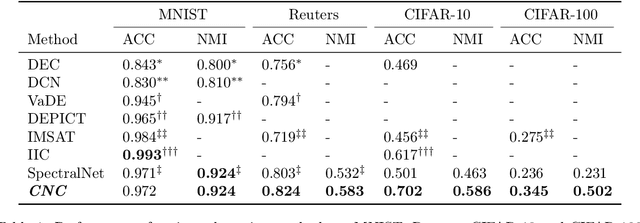
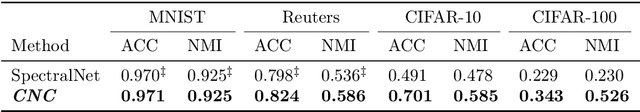
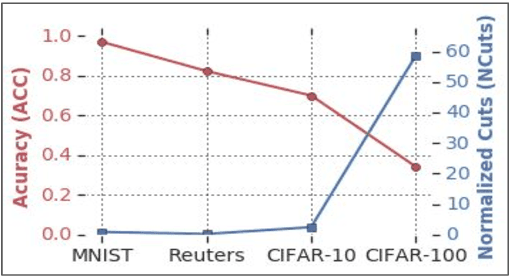
We introduce a novel end-to-end approach for learning to cluster in the absence of labeled examples. Our clustering objective is based on optimizing normalized cuts, a criterion which measures both intra-cluster similarity as well as inter-cluster dissimilarity. We define a differentiable loss function equivalent to the expected normalized cuts. Unlike much of the work in unsupervised deep learning, our trained model directly outputs final cluster assignments, rather than embeddings that need further processing to be usable. Our approach generalizes to unseen datasets across a wide variety of domains, including text, and image. Specifically, we achieve state-of-the-art results on popular unsupervised clustering benchmarks (e.g., MNIST, Reuters, CIFAR-10, and CIFAR-100), outperforming the strongest baselines by up to 10.9%. Our generalization results are superior (by up to 21.9%) to the recent top-performing clustering approach with the ability to generalize.

GAP: Generalizable Approximate Graph Partitioning Framework

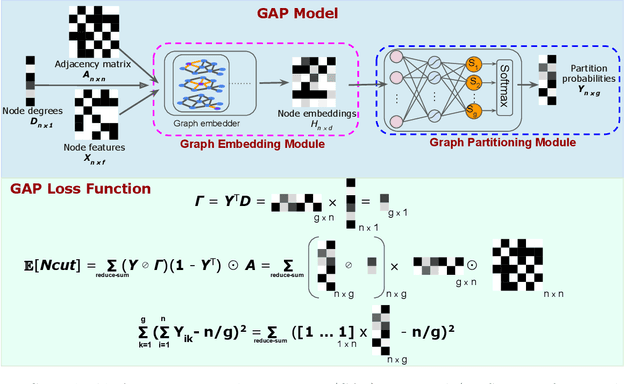
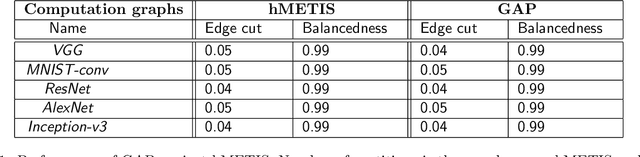
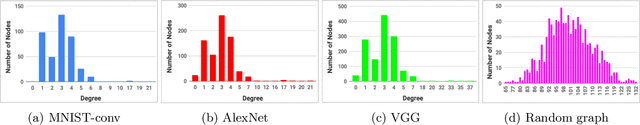
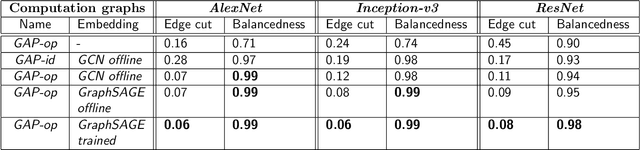
Graph partitioning is the problem of dividing the nodes of a graph into balanced partitions while minimizing the edge cut across the partitions. Due to its combinatorial nature, many approximate solutions have been developed, including variants of multi-level methods and spectral clustering. We propose GAP, a Generalizable Approximate Partitioning framework that takes a deep learning approach to graph partitioning. We define a differentiable loss function that represents the partitioning objective and use backpropagation to optimize the network parameters. Unlike baselines that redo the optimization per graph, GAP is capable of generalization, allowing us to train models that produce performant partitions at inference time, even on unseen graphs. Furthermore, because we learn the representation of the graph while jointly optimizing for the partitioning loss function, GAP can be easily tuned for a variety of graph structures. We evaluate the performance of GAP on graphs of varying sizes and structures, including graphs of widely used machine learning models (e.g., ResNet, VGG, and Inception-V3), scale-free graphs, and random graphs. We show that GAP achieves competitive partitions while being up to 100 times faster than the baseline and generalizes to unseen graphs.